
PROTEIN FOLDING, MOLECULAR DOCKING, DRUG DESIGN
The Role of the Derivative “Drift” in Complex Systems Dynamics
Corrado Giannantoni
ENEA - Casaccia Research Center - Via Anguillarese km 1,300 - 00060 S.Maria di Galeria, Rome, Italy
Keywords: Protein folding, Complex systems dynamics, Traditional Differential Calculus (TDC), Incipient Differential
Calculus (IDC), Molecular docking, Drug design.
Abstract: The relevance of Protein Folding is widely recognized. It is also well-known, however, that it is one of the
dynamic problems in TDC considered as being intractable. In addition, even in the case of solutions
obtainable in reasonable computation time, these always present a “drift” between the foreseen behavior of
the biological system analyzed and the corresponding experimental results. A drift which is much more
marked as the order of the system increases.
Both the “intractability” of the problem and the above-mentioned “drifts”, as well as the insolubility of the
problem in explicit terms (or at least in a closed form), can be overcome by starting from a different
gnoseological approach. This suggests a new definition of derivative, the “incipient” derivative.
The solution to the “Three-body Problem” obtained by means of IDC, and its extension to any number of
bodies, allows us to assert that the folding of even a macroscopic protein, such as dystrophin for example,
made up of about 100,000 atoms, can be carried out in a few minutes, when the model is run on next
generation computers (1 Petaflop).
The same methodology can also be applied to both Molecular Docking and computer-aided Drug Design.
1 INTRODUCTION
Mathematical models of Complex Systems
sometimes result as being intrinsically insoluble,
such as the famous “Three-body Problem”
(Poincaré, 1889).
In other cases they may result as being
insolvable “in practice” or, as usually referred to, as
being “intractable” (such as, for instance, Protein
Folding). This is because, although these problems
are thought of as being theoretically solvable in
principle, the time required in practice to be solved
may range from hundreds to some thousands of
years, even when run on the most updated
computers. Furthermore, even if they were solvable
in reasonable time, they would always present a
“drift” between the foreseen behavior of the system
analyzed and the corresponding experimental
results. A characteristic which is shared by all the
other mathematical models which result as being
solvable both in theory and in practice. A drift
which, in addition, is generally much more marked
as the order of the system increases.
This substantially depends on the fact that
mathematical models of Complex Systems are
generally formulated in terms of Traditional
Differential Calculus (TDC), that is by means of
linear and non-linear differential equations based on
the well-known concept of derivative. TDC,
however, often shows its limits, particularly when
describing biological Systems and, even more, social
Systems.
These in fact present such a richness of
characteristics that are, in the majority of cases,
much wider than the description capabilities of the
usual differential equations. In particular, because all
these Systems are habitually modeled as they were
mere “mechanisms”.
Such an aspect became particularly evident
during the research (Giannantoni, 2001b, 2002) for
an appropriate formulation of Odum’s Maximum
Em
ergy-Power Principle (proposed by the same
Author as a possible Fourth Thermodynamic
Principle (Odum, 1994a,b,c)).
The original concept of Emergy, in fact,
introduces a profound novelty in Thermodynamics,
that is: there are processes which cannot be
193
Giannantoni C. (2010).
PROTEIN FOLDING, MOLECULAR DOCKING, DRUG DESIGN - The Role of the Derivative “Drift” in Complex Systems Dynamics.
In Proceedings of the First International Conference on Bioinformatics, pages 193-199
DOI: 10.5220/0002763401930199
Copyright
c
SciTePress

considered as being pure “mechanisms” (Odum,
1988). This is equivalent to say that they are not
describable in mere functional terms, because their
outputs show an unexpected “excess” with respect to
their inputs. “Excess” that can be termed as Quality
(with a capital Q) exactly because it is no longer
understood as a simple “property” or a
“characteristic” of a given phenomenon, but it is
recognized as being any emerging “property” (from
the considered process) never ever reducible to its
phenomenological premises or to our traditional
mental categories.
This evidently suggests a different gnoseological
approach with a correspondingly associated new
formal language, now represented by the definition
of a new concept of derivative, the “incipient”
derivative (see Appendix).
This enabled us to reformulate the Maximum
Em-Power Principle in a more general form, that is,
as the Maximum Ordinality Principle.
The successful application of such a Principle to
some decisively “critical points” of various
Disciplines, now enables us to assert that both the
“intractability” of the problem and related “drifts”,
as well as the insolvability of the problem in explicit
terms (or at least in a closed form), can be overcome
by starting from the above-mentioned new
gnoseological approach.
2 THE M. EM-P. PRINCIPLE
The Maximum Em-Power Principle asserts that:
“Every System reaches its Maximum Organization
when maximizing the flow of processed Emergy,
including that of its surrounding habitat”.
It thus suggests we focus our attention on those
processes which can be considered as more
specifically generative. Among them (as the same
Odum points out) there are three fundamental
processes (Co-production, Inter-action, Feed-back)
in which such an aspect is particularly evident.
These processes, in fact, when analyzed under
steady state conditions, can more appropriately be
described by means of a particular non-conservative
Algebra (Brown and Herendeen, 1996). This leads to
the introduction of the concept of Transformity,
which allows us to define Emergy as
Emergy = Energy Quality (Tr ) x Energy quantity (Ex )
(1)
that is as the product of a given quantity of available
Energy (represented by Exergy), by the product of
its corresponding Quality (expressed by
Transfomity).
The M. Em-P. Principle, through the introduction
of the new concept of derivative (given in
Appendix), can be reformulated in an extremely
more general form, by replacing the concepts of
Emergy and Transformity with the concept of
Ordinality (Giannantoni, 2008a).
The corresponding verbal enunciation then
becomes: “Every System tends to Maximize its own
Ordinality, including that of the surrounding
habitat”. In formal terms, this can be expressed as
0}{)/(
)/(
=
∼∼∼
rtdd
nm
Maxnm →)/(
(2)
where:
)/( nm = the Ordinality of the System,
which represents the Structural Organization of the
System in terms of Co-productions, Inter-actions,
Feed-backs, while
}{
∼
r = the proper Space of the
System.
Such a more general formulation was thus
assumed as the preferential guide to recognize the
most profound physical nature of the basic processes
which particularly characterize self-organizing
Systems (such as Co-production, Inter-action, Feed-
back).
In such a perspective, we can now consider the
solutions to the “Two-body Problem”, to the “Three-
body Problem”, and to the more general “N-body
Problem”, respectively, in order to apply the latter
solution to the case of Protein Folding.
3 THE TWO-BODY PROBLEM
The new concept of derivative appeared as rather
surprising from the very beginning. In fact, although
originating from the description of self-organizing
systems, it seemed also valid when describing non-
living systems, such as those analyzed in Celestial
Mechanics. Let us think of, for example, Mercury’s
Precessions, the Three-body Problem, etc..
The initial idea of adopting IDC, to reconsider
such problems in a new light, originated from the
subsisting difference between the derivatives of the
exponential function
)(t
e
α
obtained on the basis of
the two distinct concepts of derivative (see Table 1).
In this respect it is worth noting that the assumption
of the exponential function as a reference function
does not represent a limitation, because any
function
)(tf can always be structured in the form
)()(ln
)(
ttf
eetf
α
==
(3)
BIOINFORMATICS 2010 - International Conference on Bioinformatics
194
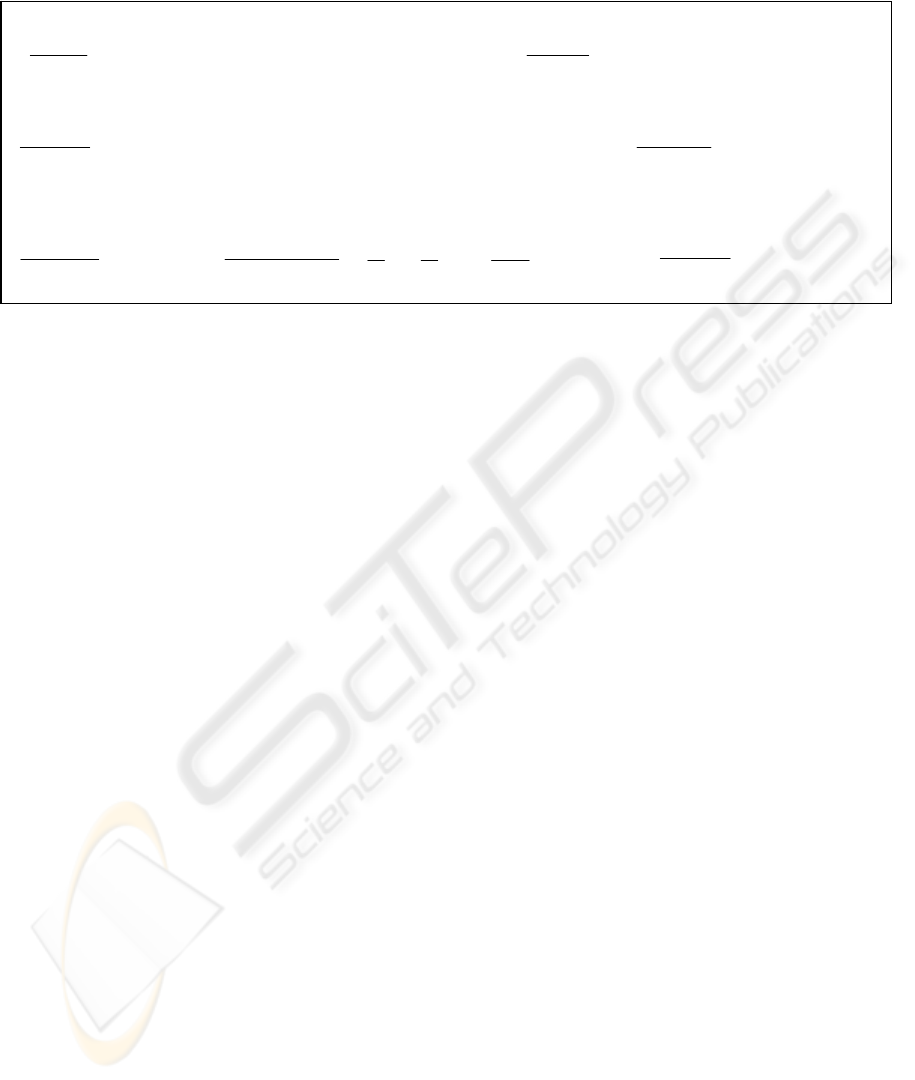
Table 1: Comparison between traditional and incipient derivates for the exponential function
)(t
e
α
, where the traditional
derivative of order n is expressed by Faà di Bruno’s formula (Oldham & Spanier, 1974, p. 37).
Such a choice, in addition, simplifies the
exposition of the basic concepts we are going to
present.
As Table 1 clearly shows, the traditional
derivatives present “additional” terms (from the
second order on) with respect to the incipient
derivatives. Such a specific “difference” suggested
the possibility of re-interpreting, by means of
incipient derivatives, the “failure” of Classical
Mechanics in foreseeing Mercury’s Precessions,
without modifying, in any form, the space-time
concepts, as vice versa happens in General
Relativity (Giannantoni, 2004b).
In fact the “Two-body Problem”, as traditionally
modeled in Classical Mechanics, is strictly
equivalent to solving a second order homogeneous
differential equation with variable coefficients
(Landau and Lifchitz, 1969, p. 46). At the same time
it is also well known that Classical
Mechanics underestimates the value of Mercury’s
Precessions, by foreseeing an angular anomaly of
“zero”, with respect to 42.6 ± 0.9 sec/cy, obtained by
astronomical measurements (ib.). It was precisely
this “discrepancy” which led us to think that such an
effect could be directly related to the “drift” of the
second order traditional derivative with respect to
the corresponding second order “incipient”
derivative. In fact, we obtained an estimation of the
angular anomaly of 42.45 sec/cy, which represents a
satisfactory agreement with the most recent
available data (Giannantoni, 2004b).
4 THE THREE-BODY PROLEM
The Three-body Problem was proved to be
intrinsically insoluble in Classical Mechanics
(Poincaré, 1899). In fact it is described by an 18th-
order system of ordinary differential equations
which, however, admits only 2 first order closed
form integrals (energy and areas). The concept of
“integral”, in this case, is not understood according
to the traditional sense of “solution”, but as a
“function of solutions” (ib., p. 8) structured in the
form
ttxtxtxF
ni
cos)](),....(),([
21
=
(4)
where
),(
1
tx
)(),....(
2
txtx
n
represent the generic
unknown variables of the considered problem. (ib. ,
vol. 1, p. 253).
Vice versa, when faced in terms of incipient
derivatives, the problem becomes perfectly solvable,
in the sense that: i) there exists at least one solution
in a closed form, as explicitly desired by Poincaré
(ib.); ii) such a solution, in addition, can be obtained
(always in a closed form) at there different
hierarchical levels of Ordinality, according to the
initial model adopted (Giannantoni 2007b, pp. 49-
60): a) as System made up of three distinct bodies;
b) as System made up of three “binary-duet ” sub-
systems, c) as one sole “ternary ” System made up of
three “binary-duet ” sub-systems (see also
Appendix).
The fact that the “Three-body Problem”, even in
its most general form, admits at least one solution in
a closed form when reformulated in IDC, is
substantially due to the intrinsic and specific
properties of the incipient derivatives (see
Appendix). In fact such a solution can be obtained
on the basis of the following: i) the Fundamental
Theorem of the Solving Kernel (Giannantoni, 1995),
which gives the general solution of any linear
differential equation with variable coefficients in
)(
)(
)(
t
t
et
dt
de
α
α
α
⋅=
)(
)(
)(
t
t
et
td
ed
α
α
α
⋅=
∼
∼
D
)()(2
2
)(2
)()]([
tt
t
etet
dt
ed
αα
α
αα
⋅+⋅=
)(2
2
)(2
)]([
t
t
et
td
ed
α
α
α
⋅=
∼
∼
D
∑
⋅⋅=
!!...!
!
21
)(
)(
n
t
n
tn
kkk
n
e
dt
ed
α
α
n
k
n
kk
n
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
⋅⋅
⎟
⎠
⎞
⎜
⎝
⎛
⎟
⎠
⎞
⎜
⎝
⎛
!!2!1
)(
21
ααα
)(
)(
)]([
tn
tn
et
t
d
ed
α
α
α
⋅=
∼
∼
D
PROTEIN FOLDING, MOLECULAR DOCKING, DRUG DESIGN - The Role of the Derivative "Drift" in Complex
Systems Dynamics
195

terms of the sole Solving Kernel; ii) such a solution,
in particular, is already structured in a closed form
(according to Poincaré’s definition) and can directly
be transposed to the case of incipient derivatives
(Giannantoni 2007a, ch. 5); iii) in addition, since the
Solving Kernel is generally a function of function,
such a transposition can be directly obtained by
means of Faà di Bruno’s formula (ib., ch. 3); iv) this
in fact, being in turn structured in a closed form, can
directly be transposed to the derivatives of functions
of function when the latter are expressed in incipient
terms (the only difference is that, in such a case,
there are no longer “partitions” and, consequently,
related “sums”); v) finally, any traditional non-linear
differential equation in TDC can always be
transformed into a linear Ordinal differential
equation in IDC, with the same methodology as
already shown, for example, with reference to
Riccati’s Equation (ib. ch. 2).
On the other hand, such a general procedure,
already adopted in other papers and books (e.g.,
Giannantoni, 2004a,b, 2006a), is the same which
enabled us to sustain the general validity of a
Differential Calculus (namely IDC), which
contemporaneously operates in terms of Ordinality
and cardinality (Giannantoni 2007a, ch. 3).
These solutions, however, are still affected by
another form of “drift”, related to the supposed
independence of the space variables (x,y,z) from
each other. A hypothesis which, in reality, is merely
an aprioristic assumption about the geometrical
nature of the proper Space of the System.
If, vice versa, the proper Space of the System is
considered as being essentially “unum”, that is to
say the three coordinates (x,y,z) are so strictly
related to each other so as to form one sole thing of
Ordinal nature (ib., ch. 6), the problem admits an
extremely elegant solution in explicit terms and in
an Ordinal exponential form (ib.).
In such a case, the cardinal structure of the
System is nothing but the formal reflex of its Ordinal
nature, and it can be obtained through an adherent
reduction process (ib.).
5 THE N-BODY PROBLEM
The results obtained in the case of the Three-body
Problem can easily be generalized to the “N-body
Problem” (Giannantoni, 2008b, ch. 22), so as to get
an explicit solution in an Ordinal exponential form
(ib.). This solution is not affected by any form of
“drift”, either due to the “step by step” derivation or
to the supposed reciprocal independence of
coordinates, because the System analyzed is always
referred to its proper Space of generative nature.
On the other hand, such an extension can easily
be understood on the basis of what has been
previously said: any non-linear differential equation,
written in terms of incipient derivatives, can always
be transformed into a linear differential equation
(Giannantoni, 2007a, ch. 2).
This enables us to assert that the simulation of
Protein Folding, even in the case of a macroscopic
protein, such as dystrophin (made up of about
100,000 atoms), can be obtained in a few minutes,
when run on the next generation computers (1
Petaflop).
6 CONCLUSIONS
The solution to the “Three-body Problem”, thought
of as being a self-organizing system, and thus
modeled in the light of the Maximum Ordinality
Principle, after having been successfully extended to
the case of “N-body Problem”, enables us to assert
that any Protein Folding can be modeled as a
“tractable” problem. This can be solved by means of
appropriate computer code (in progress), to firstly
analyze biological systems made up of a limited
number of atoms (e.g, sugars and their mono-
chirality), before modeling the smallest proteins
(bout 2.000 atoms).
The computer time strictly required for its
solution (some minutes) evidently refers to the sole
availability of the three-dimensional space
configuration of the considered protein, given by an
appropriate set of data in the computer memory. The
subsequent analysis of the various parts of the folded
protein, however, could require much longer times.
In all cases, the analysis will always result as being
feasible and practicable.
What has been previously said about Protein
Folding can clearly be applied to Molecular
Docking, as well as to computer-aided Drug Design.
In this respect we have also analyzed the
possibility of adopting the same methodology when
analyzing the so-called “Phase 0”, recently defined
by US Food and Drug Administration (Shivaani K.
et al. 2009).
Clearly it is worth pointing out that the
mathematical procedure [code] here proposed
should not be considered as being reducible to a
mere mathematical “tool”, that is as simply being
able to solve the above-mentioned problems in a
more efficient way. This is because it represents a
radically new methodology, precisely because it is
BIOINFORMATICS 2010 - International Conference on Bioinformatics
196

based on IDC. This new differential calculus, in fact,
“translates” into an adherent formal language a
gnoseological approach which is completely
different from the traditional one. This difference
resides on the three new basic presuppositions:
Generative Causality, Adherent Logic, Ordinal
Relationships (see also Appendix).
This an entirely different approach also enabled
us to recognize the reason for the mono-chirality of
proteins (Giannantoni, 2007a., ch. 18). That very
aspect which, really surprisingly, is ever present,
even in non-living Systems. For example, in the
motion of the planets in the Solar System. Albeit
mono-chirality is characterized in this case by some
“genetic” properties which, by keeping “memory” of
the generative process of the System, always reveal
the different nature of mono-chirality with respect to
biological systems (such as proteins, for instance)
(ib.).
REFERENCES
Brown M. T. and Herendeen R. A., 1996. Embodied
Energy Analysis and Emergy analysis: a comparative
view. Ecological Economics 19 (1996), 219-235.
Giannantoni C., 1995. Linear Differential Equations with
Variable Coefficients. Fundamental Theorem of the
Solving Kernel. ENEA, Rome, RT/ERG/95/07.
Giannantoni C., 2001a. The Problem of the Initial
Conditions and Their Physical Meaning in Linear
Differential Equations of Fractional Order. Applied
Mathematics and Computation 141 (2003) 87-102.
Giannantoni C., 2001b. Mathematical Formulation of the
Maximum Em-Power Principle. Second Biennial
International Emergy Conference. Gainesville
(Florida, USA), September 20-22, 2001, pp. 15-33.
Giannantoni C., 2002. The Maximum Em-Power Principle
as the basis for Thermodynamics of Quality. Ed.
S.G.E., Padua, ISBN 88-86281-76-5.
Giannantoni C., 2004a. Differential Bases of Emergy
Algebra. Third Emergy Evaluation and Research
Conference. Gainesville (Florida, USA), January 29-
31, 2004.
Giannantoni C., 2004b. Mathematics for Generative
Processes: Living and Non-Living Systems. 11
th
International Congress on Computational and Applied
Mathematics, Leuven, July 26-30, 2004. Applied
Mathematics and Computation 189 (2006) 324-340.
Giannantoni C., 2006. Emergy Analysis as the First
Ordinal Theory of Complex Systems. Proceedings of
the Fourth Emergy Conference 2006. Gainesville,
Florida, USA, January 17-22, pp. 15.1-15.14.
Giannantoni C., 2007a. Armonia delle Scienze (vol. I). La
Leggerezza della Qualità. Ed. Sigraf, Pescara (Italy),
ISBN 978-88-95566-00-9.
Giannantoni C., 2007b. Ordinal Benefits vs Economic
Benefits as a Reference Guide for Policy Decision
Making. The Case of Hydrogen Technologies.
Submitted to Energy (February 2008). In press.
Giannantoni C., 2008a. From Transformity to Ordinality,
or better: from Generative Transformity to Ordinal
Generativity. Proceedings of the Fifth Emergy
Conference. Gainesville, Florida, USA, January 31-
February 2, 2008.
Giannantoni C., 2008b. Armonia delle Scienze (vol. II).
L’Ascendenza della Qualità. Edizioni Sigraf, Pescara
(Italy), ISBN 978-88-95566-18-4.
Landau L. and Lifchitz E., 1969. Mécanique. Ed. MIR,
Moscow.
Odum H. T., 1988. Self-Organization, Transformity and
Information Science, v. 242, pp. 1132-1139,
November 25.
Odum H. T., 1994a. Ecological and General Systems. An
Introduction to Systems Ecology. Re. Edition.
University Press Colorado.
Odum H. T., 1994b. Environmental Accounting. Environ.
Engineering Sciences. University of Florida.
Odum H. T., 1994c. Self Organization and Maximum
Power. Environ. Engineering Sciences. University of
Florida.
Oldham K. B. and Spanier J., 1974. The Fractional
Calculus. Theory and Applications of Differentiation
and Integration to Arbitrary Order. Academic Press,
Inc., London.
Poincaré H., 1889. Les Méthodes Nouvelles de la
Mécanique Céleste. Ed. Librerie Scientifique et
Technique A. Blachard. Vol. I, II, III, Paris, 1987.
Shivaani K. et al. 2009. Phase 0 Clinical Trial of the Poly
(ADP-Ribose) Polymerase Inhibitor ABT-888 in
Patients with Advanced Malignancies. Journal of
Clinical Oncology. Published Ahead of Print on April
13, 2009 as 10.1200/JCO.2008.19.7681.
APPENDIX
The analysis of Generative Processes under dynamic
conditions suggests the introduction of a new
concept of “derivative”. This is because the same
adoption of the traditional derivative (d/dt) is
nothing but the formal reflex of three fundamental
pre-assumptions when describing physical-
biological-social systems: i) efficient causality; ii)
necessary logic; iii) functional relationships.
It is then evident that such an aprioristic
perspective excludes, from its basic foundation, the
possibility that any process output might ever show
anything “extra”, with respect to its corresponding
input, as a consequence of the intrinsic (supposedly)
necessary, efficient and functional dynamics of the
system analyzed.
Consequently, such a theoretical approach will
never see any “output excess”, exactly because it has
PROTEIN FOLDING, MOLECULAR DOCKING, DRUG DESIGN - The Role of the Derivative "Drift" in Complex
Systems Dynamics
197

already excluded from the very beginning (but only
aprioristically) that there might be “any”. In this
sense it is possible to say that such an approach
describes all the phenomena as they were mere
“mechanisms”.
Generative Processes, on the contrary, suggest
we think of a different form of “causality”, precisely
because their outputs always show something in
“excess” with respect to their inputs. This
“causality” may be termed as “generative” causality
or “spring” causality or whatsoever. In all cases the
basic concept is rather clear. In fact, any term
adopted is simply finalized at indicating that it is
worth supposing a form of “causality” which is
capable of giving rise to something “extra” with
respect to what it is usually foreseen (and expected)
by the traditional approach.
The same happens for Logic. In fact, a different
Logic is correspondently needed in order to
contemplate the possibility of coming to conclusions
much richer than their corresponding premises. This
new form of Logic, in turn, could correspondently
be termed as “adherent” Logic, because its
conclusions are always faithfully conform to the
premises. The conclusions, however, could even be
well-beyond what is strictly foreseen by the same
premises when interpreted in strictly necessary
terms.
As an adherent consequence of both previous
concepts, the relationships between phenomena
cannot be reduced to mere “functional” relationships
between the corresponding cardinal quantities. In
fact, they always “vehicle” something else, which
leads us to term those relationships as “Ordinal”
relationships. The term “Ordinal”, which might
appear as being simply adopted only to make a
difference with respect to its corresponding
“cardinal” concept, has in reality a much more
profound meaning (as shown later on).
At this stage we can clearly assert that the new
concept of derivative is nothing but the adherent
“translation”, in formal terms, of the three new
gnoseological concepts: Generative Causality,
Adherent Logic, Ordinal Relationships.
Such a new derivative was termed as “incipient”
(or prior derivative) because it describes the
processes in their generating activity or, preferably,
because it focuses on their pertinent outputs in their
specific act of being born. Its mathematical
definition is substantially based on the reverse
priority of the order of the three elements that
constitute the traditional definition:
()
tf
t
t
Δ
Δ
→Δ 0
lim
(5)
that is: i) the concept of function (which is assumed
to be a primary concept); ii) the incremental ratio (of
the supposedly known function); iii) the operation of
limit (referred to the result of the previous two
steps). It is thus defined as follows (for further
details see also (Giannantoni, 2001a, 2002)):
() ()
tf
t
Limtf
td
d
q
t
q
q
DD
∼
∼
∼
∼
→Δ
∼
∼
⎟
⎟
⎟
⎠
⎞
⎜
⎜
⎜
⎝
⎛
Δ
−
=
+
∼
1
00:
δ
(6)
where: i) the symbol
∼
Lim now represents a sort of
“window” or “threshold” (= Limen in Latin), from
which we observe and describe the considered
phenomenon, whereas
+
∼
→Δ 00:t indicates not
only the initial time of our registration, but also the
proper “origin” (in its etymological sense) of
something new which is being born; ii) the
“operator”
∼
δ
registers the variation of the property
(
)
tf
analyzed, not only in terms of quantity, but
also, and especially, in terms of Quality (as indicated
by the symbol “tilde” specifically adopted); iii) thus
the ratio (
t
∼
∼
Δ
−1
δ
) indicates not only a quantitative
variation in time, but both the variation in Quality
and quantity. That is, the Generativity of the
considered process or, in other terms, the output
“excess” (per unit time) characterized by both its
Ordinality and its related cardinality; iv) the
sequence of symbols in Eq. (6) is consequently
interpreted according to a direct priority (from left to
right); v) the sequence is also interpreted as a
generative inter-action (represented by the symbol
“
D
”) between the three considered concepts; vi) the
definition is valid for any fractional number
q . This
enables us to represent the three basic processes
(Co-production, Inter-action, Feed-back) in terms of
fractional derivatives of order 1/2, 2, and {2/2}
respectively. In such a case the order of derivation is
termed as Ordinality, because the corresponding
resulting “functions” (“binary”, “duet”, and “binary-
duet” functions, respectively) are structured in such
a way as to show an “excess” of Information, which
is never ever reducible to its sole phenomenological
BIOINFORMATICS 2010 - International Conference on Bioinformatics
198

premises or to our traditional mental categories
(Giannantoni, 2004b).
On the basis of such a definition, by always
referring to a generic function structured in the
exponential form
)(t
e
α
(for the same reason
previously specified), the incipient derivative of
order
n is given by
)()(
)]([)(
tntn
ete
td
d
αα
α
⋅=
∼
∼
D
(7)
where
)(t
D
α
represents the first order incipient
derivative of the function
)(t
α
. The symbol of a
little circle adopted to denote the incipient derivative
was evidently chosen in analogy to classical
Newton’s “dot” notation, which usually indicates a
first-order derivative.
The different symbology is here justified by the
fact that the former should now remind us the
conceptual difference between the incipient
derivative and the traditional one.
In fact, even if
)(t
D
α
and )(t
α
coincide from a
pure cardinal point of view, they are, on the
contrary, radically different from a Generative point
of view. The former in fact represents the specific
exit of a Generative Process, whereas the latter is
always understood as the result of a necessary
process (thought of as being a “mechanism” or a set
of “mechanisms”).
Such a quantitative coincidence, however, is
strictly valid only for
1=n . The right hand side of
Eq. (7), in fact, reveals an extremely important
property: a sort of “persistence of form”, which is
even more marked when the derivative is of
fractional order (
nm / ). This is precisely because it
represents an “adherent” consequence of a
Generative Process, characterized by specific
generation modalities. In other words, any
“generating process” (modeled by the left hand side
of Eq. (7)) gives origin to an output which
corresponds to a multiple structure functions
(multiple “binary” functions or multiple “duet”
functions (or both)), characterized by the Ordinality
)/( nm and described by the right hand side of Eq.
(7). (Giannantoni, 2006, 2007b). These functions are
similar to harmonic evolutions always in
“resonance” (as in a “musical chord”) with the
original function and at the same time with each
other, and they reach their maximum harmony in the
case of a perfect Ordinal Feed-back
}/{ nn . (ib.)
Such a more general modeling capacity of
incipient derivatives, associated with the afore-
mentioned property that any Ordinal dynamic model
always presents a solution (at least) in a closed form
(Giannantoni 2007a, ch. 5), confers to the Incipient
Differential Calculus much wider potentialities with
respect to the Traditional Differential Calculus, both
of integer and fractional order (ib.). This is also
confirmed by the fact that such a new mathematical
approach not only led us to the solution of the
famous “Three-body Problem” (ib.), but also paves
the way to the solution to the well-known “Three-
good Problem” which, on the other hand, remains
still unsolved in Neo-Classical Economics.
PROTEIN FOLDING, MOLECULAR DOCKING, DRUG DESIGN - The Role of the Derivative "Drift" in Complex
Systems Dynamics
199
