
SYNAPTIC TRANSMISSION
AND FOKKER-PLANCK EQUATION
Elso Drigo Filho and Marcelo T. Araujo
Department of Physics, State University of São Paulo - UNESP, Cristóvão Colombo, 2265, São José do Rio Preto, Brazil
Keywords: Fokker-Planck equation, Synaptic transmission, Escape rate.
Abstract: An important neurologic process consists in a time dependent transmission of the electric signal between
neurons. The description of such signal is the objective of this work. In this way, the Fokker-Planck
equation with a term of control which depends on time is used. The applied force is characterized by the
existence of a barrier that increases with time and reduces the diffusion of particles. The solution of the
equation is obtained by an ansatz that satisfies the initial conditions of the problem. Numerical examples of
the time evolution of the found solutions are analyzed by calculating the escape rate and the time necessary
to across the barrier for different values of diffusion constant.
1 INTRODUCTION
The process related to the flux of charge in the
region between two neurons (synapse) is
fundamental to the function of the nervous system.
Thus, the understanding and description of ion
transport in the synapses have a prominent place in
modern neuroscience. This process occurs through
fast picks of electrical currents between neurons
(Hille, 1992, Ramakrishnan, 2011). Studies
involving the synapse are very important
considering that few neurological diseases have an
effective treatment (Fassio, 2011). This fact has
encouraged studies in this area, aiming to develop
techniques that can bring greater understanding of
the biological processes involved (Joshi, 2011
England, 2010, Guo, 2010, Fallon, 2011) and thus,
develop more effective treatment methods for
different types of neurological diseases.
A quantitative description of processes involving
stochastic components can be made by using the
Fokker-Planck equation (FPE). This equation has
wide application in many branches of physics,
chemistry and biology (Coffey, 2004), such as
protein folding (Curtis, 1997) or ion transport across
membranes (Lee, 2002). In some cases, the Fokker-
Planck equation can be solved analytically as, for
instance, for the linear and stationary problems
involving one variable (Risken, 1989).
The usual form of EFP is
[]
2
2
(,) () (,) (,).Pxt f xPxt Q Pxt
tx
x
∂∂ ∂
=− +
∂∂
∂
(1)
where t represents the time variable and the variable
x can be identified, for example, with the velocity
(Reichl, 1988). The function f(x) is known as the
external force acting on the system. However, this
terminology is only appropriate when x represents
the velocity. Q this is related to the diffusion
constant, and P(x,t) is the probability distribution.
Different methods have been proposed to
determine and analyze the solution of the Fokker-
Planck equation (1), as the use of the numerical
method based on finite differences (Ames, 1992),
Adomian polynomial method (Tatari, 2007) and the
mapping of FPE in equation type Schroedinger
(Risken, 1989).
In terms of synapse transmission, the potential to
be used should reflect the transitory nature of the
signal. Thus, it is suggested a potential which is
initially metastable and converges to a harmonic
potential for large value of time (Figure 1). Then,
initially the particles in the region of minimum of
potential can escape out this region. However, the
system becomes confined for the stationary regime.
The solution of the Fokker-Planck equation in this
case is obtained through an ansatz.
Studies involving kinetics of reaction, studied for
more than 70 years (Hänggir, 1990), are still based
59
Drigo Filho E. and Araujo M..
SYNAPTIC TRANSMISSION AND FOKKER-PLANCK EQUATION.
DOI: 10.5220/0003757100590063
In Proceedings of the 1st International Conference on Operations Research and Enterprise Systems (ICORES-2012), pages 59-63
ISBN: 978-989-8425-97-3
Copyright
c
2012 SCITEPRESS (Science and Technology Publications, Lda.)
on the description of the system in quasi-stationary
regime. Thus, the information related to the system
outside that regime is lost. On the other hand, the
solution of the Fokker-Planck equation (Risken,
1989) makes possible the analysis of physical and
chemical processes from the beginning, when the
system is in the no equilibrium condition.
In the section 2, the FPE and the probability
distribution are presented. In section 3, the dynamic
properties, the escape rates and a characteristic time,
of the system are determined. In section 4, numerical
examples are discussed. Finally, the conclusions are
presented in Section 5.
2 THE FOKKER-PLANCK
EQUATION
The potential studied in this work is:
(
)
23
12
() ,Vx kx kt
x
=−
(2)
where k
1
is a constant and k
2
(t) is a time function. In
order to describe the flux of ions through the
synapse, the function k
2
(t) must go to a constant
value for a large value of time (k
2
(t→∞)→constant)
and the anharmonic term become small comparing
the harmonic part. Thus, for large values of time, the
barrier of potential to be overcome for occurs
diffusion of particles from the region of minimum
becomes very large. In this condition the system is
governed by a quasi harmonic potential. The explicit
form adopted for k
2
(t) in this work is:
()
1
3/2
4
22,0 1
() 2 .
kt
kt k k e
λλ
−
−
⎡⎤
=+−
⎣⎦
(3)
where k
2,0
is a constant. This expression attends the
properties described above and allows an analytical
solution for EFP (1) with the function f(x) given by
derivative of potential (2),
2
12
() 2 3
f
xkxkx=− +
(4)
The solution of the equation (1), for f(x) given by
(4), can be found assuming that P(x,t) is written as
3
2
2
2
()
1
(,) exp
()
2()
ktx
x
Pxt
tQ
Qt
λ
φ
φ
⎧⎫
⎪⎪
=−+
⎨⎬
⎪⎪
⎩⎭
(5)
where λ is a constant defined from the normalization
of probability and the function
φ
(t) is given by
1
4
2
11
() 1
22
kt
te
kk
λλ
φ
−
⎛⎞
=+−
⎜⎟
⎝⎠
(6)
The initial condition adopted is that all particles
are initially in the region of minimum in x = 0.
Then, in the initial time (t = 0) the distribution of
probability has value equal 1 in the position x = 0.
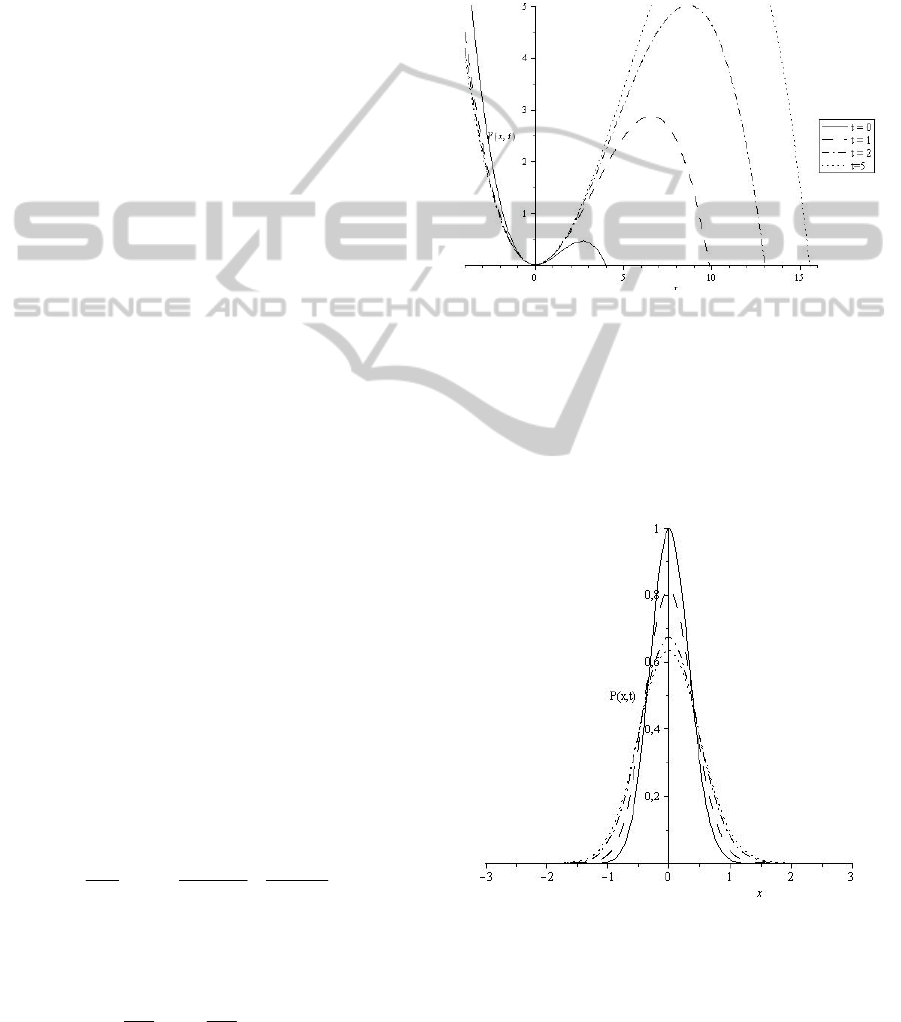
The figure 1 shows a numerical representation of
the potential (2) in function of position for different
values of time, with k
2
(t) given by (3) and the
parameters k
1
= 0.2, λ = 1 and k
2,0
= 0.05.
Figure 1: Curves of the potential V(x,t) defined in
equation (2) for different values of time, k
1
= 0.2, λ = 1
and k
2,0
= 0.05.
The distribution of probabilities, equation (5), is
showed in the figure 2 for different values of time
and diffusion constant. The values of k
1
, λ and k
2,0
are the same used in the figure 1 (k
1
= 0.2, λ = 1 e
k
2,0
= 0.05).
a)
Figure 2: Distribution of probability for different times
with a) Q = 0.1 and b) Q = 1.
ICORES 2012 - 1st International Conference on Operations Research and Enterprise Systems
60

b)
Figure 2: Distribution of probability for different times
with a) Q = 0.1 and b) Q = 1. (Cont.)
3 DYNAMIC PROPERTIES
OF THE SYSTEM
Important results for the transmission at synapses are
the diffusion rate (r) and or time of passage through
the barrier (τ). In order to compute these quantities,
one consider the initial potential metastable (2) (t =
0), as showed in figure 3.
Figure 3: Representation of a metastable potential.
The figure 3 shows a maximum peak in x
max
and
a point of local minimum in the region I (x
min
). The
points x
1
and x
2
refer to a region I around the
minimum x
min
and A referes to a point in the second
region, region II, after the barrier. The escape rate of
particles through the barrier can be calculated from
the relation,
,
J
r
w
=
(7)
where J is the current probability or particle flow
through a particular region and w represents the
population within the region of minimum. They are
defined, respectively, as
2
1min
( , ) ( , ) e ( , ) ( , ) .
x
A
xx
wxt Pxtdx Jxt jxtdx==
∫∫
(8)
The function j(x,t) is obtained from the Fokker-
Planck equation, such that,
(,) () (,) (,).
j
xt f xPxt Q Pxt
x
∂
=−
∂
(9)
Then, the rate r can be rewritten as
2
min 1
() ( , ) ( , ) .
x
A
xx
rt jxtdx Pxtdx=
∫∫
(10)
This literal definition allows obtaining the escape
rate in the various situations of potential.
Another important quantity to characterize the
diffusion through the barrier is the first passage time
or particle escape time (τ) (Lenzi, 2001). This time
corresponds to the inverse of the escape rate, i.e.:
2
1min
1
( ) ( , ) ( , )
x
A
xx
rt Pxtdx jxtdx
τ
τ
=⇒=
∫∫
(11)
4 NUMERICAL RESULTS
Using the solution for the Fokker-Planck equation
(5), the curves obtained for the escape rate of
particles (equation (10)) for different values of
diffusion (Q) are presented in figure 4. The constants
values are adopted as k
1
= 0.2, λ = 1 and k
2,0
= 0.05.
Figure 4: Curves of the rate escape (r) versus t for
different values of Q with k
1
= 0.2, λ = 1 and k
2,0
= 0.05.
Time: t = 0.0; t = 0.5; t = 2.0;
t = 5.0
SYNAPTIC TRANSMISSION AND FOKKER-PLANCK EQUATION
61

In the figure 4 it is showed that the rate of
diffusion of particles decays with time until the
value zero. This behavior is associated with the
potential that is not constant on time. For large value
of time, the potential presents a high and large
barrier. The result is the confinement of the
particles.
This phenomenon is also observed by the first
passage time (τ) of the particles through the barrier,
equation (11). In the figure 5 the value of τ as a
function of the time is plotted. Initially, the time is
short featuring a fast passage of particles through the
barrier, but this value increase due to the increased
and diverges (τ → ∞) for large values of time.
Figure 5: Escape time (τ) versus t for different values of
Q, with k
1
= 0.2, λ = 1 and k
2,0
= 0.05.
From the curves in figure 5, it is possible to
observe that for large values of the Q the diffusion
through the barrier become more fast and effective.
In figure 4 we note that for a time t = 4 there is a
small diffusion of particles when Q equal to 2, but
for a low value of the diffusion coefficient (for
instance, Q = 0.1) the diffusion is null for this time.
Following the same reasoning, it is observed that for
a low value of the diffusion coefficient, the time of
first passage (figure 5) becomes long for large Q
values.
5 CONCLUSIONS
The results obtained from the solution of the Fokker-
Planck equation for the potential suggested (2)
exhibit a behavior consistent with that expected from
the electrical transmission at synapses. The flow of
charge is most intense at the beginning of the signal
and tends to vanish for large values of time. This
result combined with the construction of the
potential allows concluding that this approach can be
effective to describe the dynamics of the physical
process that occurs at synapses.
The models proposed in literature usually
consider the diffusion through a fixed barrier of
energy on time and the escape rate is calculated
using only the results of the system at the stationary
state (rate of Kramer (Risken, 1989, Hänggir,
1990)), which limits quite the applicability of these
models. Then, the potential proposed here can be
useful to build more realistic models.
ACKNOWLEDGEMENTS
The authors thank CNPq, FAPESP and
FUNDUNESP (Brazilian agencies) for partial
financial support.
REFERENCES
Hille, B., 1992. Ionic Channels of Excitable Membranes,
Sinauer Associates INC., Sunderland, 2
nd
edition.
Ramakrishnan, S., Hasler, P. E., Gordon, C., 2011.
Floating Gate Synapses With Spike-Time-Dependent
Plasticity, IEEE Trans. Biomed. Circuits Syst., v. 5
244-252.
Fassio, A., et. al., 2011. Synapsins: From synapse to
network hyper excitability and epilepsy, Semin. Cell
Dev. Biol., 8p.
Joshi, J., et. al., 2011. A Biomimetic Fabricated Carbon
Nanotube Synapse for Prosthetic Applications, Life
Science Systems and Applications Workshop, 139-
142.
England, P. M., 2010. Bridging the Gaps between
Synapses, Circuits, and Behavior, Chem. & Biol. 17,
607-615.
Guo, D., Li, C., 2010. Signal propagation in feed forward
neuronal networks with unreliable synapses, J.
Comput. Neurosci., v. 30, 567–587.
Fallon, J. R., 2011. Calcium channels put synapses in their
place, Nat. Neurosci., v. 14, 536 – 538.
Coffey, W., 2004. The Langevin equation: with
applications to stochastic problems in physics,
chemistry, and electrical engineering, River
Edge: World Scientific, 2
nd
edition.
Curtiss, C. F., Bird, R. B., 1997. Fokker–Planck equation
for the one-molecule distribution function in polymer
mixtures and its solution, Jour. Chem. Phys., v. 106, n.
23.
Lee, K., Sung, W., 2002. Ion transport and channel
transition in biomembranes, Phys. A, v. 315 79 - 97.
Risken, H., 1989. The Fokker-Planck Equation, Springer,
2
nd
edition.
Reichl, L. E., 1988. A Modern Course in Statistical
Physics, Wiley-Interscience, 2
nd
edition.
ICORES 2012 - 1st International Conference on Operations Research and Enterprise Systems
62

Ames, W. F., 1992. Numerical methods for partial
differential equations, Academic Press INC., San
Diego.
Tatari, M., Dehghan, M., Razzaghi, M., 2007. Application
of the Adomian decomposition method for the Fokker-
Planck equation, Math. and Comp. Model., v. 45,
639–650.
Hänggir, P., Talkner, P., Borkovec, M., 1990. Reaction-
rate theory: fifty years later Kramers, Rev. Mod.
Phys., v. 62.
Lenzi, E. K., Anteneodo, C., Borland, L., 2001. Escape
time in anomalous diffusive media, Phys. Rev. E, v.
63, 051109 (1-5).
SYNAPTIC TRANSMISSION AND FOKKER-PLANCK EQUATION
63
