STATE OBSERVER FOR NONLINEAR SYSTEMS:

APPLICATION TO GRINDING PROCESS CONTROL

Seraphin C. Abou & Thien-My Dao

Mechanical / Manufacturing Engineering Department

Ecole de Technologie Supérieure

1100, rue Notre-Dame West

Montreal, (Quebec), H3C 1K3, Canada

Keywords: Process control; Mineral process; Nonlinear systems; Nonlinear observer; Grinding

Abstract: Due to the measurement problems encountered in mineral processes, observers are appropriate ingredients

of advanced model based control algorithm. The measurement problem can be solved by designing

nonlinear observer. This paper discusses the way in which a state observer may be designed to control a

special class of nonlinear systems. Focus is put on the pertinent applicability of the scope of these

techniques, to control the dynamics of mills in mineral processes. The approach uses a small number of

parameters to control the mill power draw affected by sudden changes within the system. It provides with

principles and ability of the system to adapt to changing circumstances due to intermittent disturbances (like

for instance changes in hardness of the raw material). Performance and stability analysis was developed.

Using a generalised similarity transformation for the error dynamics, it is shown that under boundedness

condition the proposed observer guarantees the global exponential convergence of the estimation error. This

way, the nominal performance of the process is improved but the robust stability is not guaranteed to fully

avoid the mill plugging.

1 INTRODUCTION

Grinding plants never operate at steady state but

rather at perpetual transient states due to a variety of

disturbances. The mathematical model was

addressed in the way that combines disturbance

parameters with material physical properties. It

satisfies sufficient conditions which lead to

determine the system at any instant in time.

In mineral processes, the application of modern

model based control algorithms is hampered by the

lack of accurate and cheap on-line sensors. The

design of state observers, which reconstruct states

out of a limited set of measurements, is a possible

approach for dealing with the measurement problem.

Due to the (time varying) nonlinear behaviour of

grinding systems, the measurement problem can

only be solved by designing nonlinear observers.

In general, observers design methodologies are

based on (i) exact linearisation, (ii) local

linearisation in original coordinates, (iii) local

linearisation in observer coordinates, and (iv) high

gain methods are considered (Misawa, 1989). Due to

the process uncertainty, inherent in mineral

processing, applicability and robustness analysis of

the nonlinear observers have been performed. The

stability properties analysed are with respect to zero,

which is equilibrium for the proposed system. In this

sense, our main restriction on the nominal system is

that the subsystem be globally stable with variable

viewed as a virtual control input. As a case study,

wet grinding in continuous and fed-batch operation

mode considered is described in Section 2. In

Section 3 observer design is discussed in general

while simulation results are presented in Section 4.

The observer performance analysis is discussed in

general in Section 5.

2 SYSTEM DESCRIPTION

A wet grinding shown in Fig.1 or dry grinding

(cement processing) has been developed with the

objective of studying the effects of many variables

on particle size reduction in continuous grinding

processes. Detailed phenomenological model that

describes the charge behaviour has been developed

and validated against real data (Abou, 1998).

Indeed, mineral processes present non-linear/chaotic

dynamic behaviour. Considerable efforts have been

developed in controlling such system, (Abou, 1997),

(Weller, 1980). In (Abou, 1998), a comprehensive

310

C. Abou S. and Dao T. (2004).

STATE OBSERVER FOR NONLINEAR SYSTEMS: APPLICATION TO GRINDING PROCESS CONTROL.

In Proceedings of the First International Conference on Informatics in Control, Automation and Robotics, pages 312-319

DOI: 10.5220/0001131803120319

Copyright

c

 SciTePress

model integrates the physical mechanisms governing

mineral processes and a fundamental understanding

of the charge behaviour was expressed. It was

pointed out that grinding media collisions and

impacts on lifters induced non-linearity in materials

breakage process. Due to inappropriate control of

the motor charge, important engineering conclusions

derived from the charge motion studies (Abou,

1997), recommend a focused study of the influence

of the wear of both the grinding media and the lifters

on the material size reduction quality. Further

Investigation reveals that, an important factor of the

poor quality of fine grinding is due to lacks of an

appropriate control of the power draw of the mill.

This causes increase of energy consumption, and

production cost, (Austin, 1990).

To address practical results which could be

transferred to industrial level, the key is the

development of a practically an accurate grinding

circuit control. That is to maximise the

manoeuvrability at the low and the high speed

rotating stability of ball mills when the material

hardness and size or the slurry concentration change.

Figure.1: Schema of ball mill powering system

3 GRINDING PROCESS

MODELLING

Grinding systems are power-intensive, and even the

simplest ones; exhibit complex bifurcation

behaviour in going from periodic motion to chaos.

Such a complex behaviour has been noticed in the

analyses of the dynamics of the charge of ball mill

(Abou, 1998). It appears that, simple but nonlinear

models are necessary to describe such a system. The

main goal is to minimise the consumption energy,

avoid strong impact which causes wear of lifters,

and rotate the charge with optimal speed for required

fine particle quality. Using the cross section of the

ball mill shown in Fig.1, the mill action could be

shown graphically by considering the change in

position of the centre of gravity of ball and particle

charge with increasing speed of rotation, Fig.2.

η

z

η

y

ω

k <

ω

j <

ω

0

Impact

zone 1

r

j

R

ω

0

ω

j

Trajectories

of balls

ω

k

Impact

zone 2

Figure 2: Ball movement with various rotation speed

Notice that the motor load is influenced by the

filling percentage, the speed, the mill geometry and

other relevant material properties such as stiffness

and the coefficient of friction, etc… As shown in

Fig.2, theoretical position of the charge at different

rotation speed was first derived by (Davis, 1919)

based on force balance.

Most research (Austin, 1990), have developed first

order model to describe the system. However, their

use in practical solutions context has a lack of their

dependence on the physical parameters of the

system. Since the problem is to develop the grinding

process model for control purpose, the main

objective in an advanced mathematical model

formulation could base on the following basic

control flowchart structure, Fig.3 to develop the

process behaviour.

Moto

r

Ball Mill

Definition of control

objectives

Process advanced

mathematical modelling

(,)

x

fxu

=

&

Controller

Figure 3: Control system design procedure

Notice that, besides in batch mode, grinding circuit

can operate in continuous or fed-batch mode. Based

on the interpretation of the Fig.3, we are interested

in the constitutive characteristics of the charge

motion defined by the function

(

,

)

f

xu

, focusing on

the specific parameters that better describe

continuous grinding phenomena relationship. From a

macroscopic standpoint, the internal breakage model

can be formulated taking in account the specifics

phenomena of particle transport and size reductions:

STATE OBSERVER FOR NONLINEAR SYSTEMS: APPLICATION TO GRINDING PROCESS CONTROL

311

() () ()

..

i

nn

m

.

i

x

m

tzz

∂

∂∂

∂∂∂

⎡⎤

=Ψ −Ψ⎡⎤

⎢

⎣⎦

⎣

⎥

⎦

(1)

where,

[kg], is particle mass of size i

i

m

The left side term of equation (Abou, 1998)

expresses the rate of mineral production, while the

term at the right side indicates fine particle transport

phenomena. In such a process with distributed

parameters, function Ψ

n

(.) that characterises the

particle size reduction, depends on many variables

which are absolutely linked to system performance

reliability. Therefore, without lacking for the

physical sense for the process, we can write:

(

)

(

)

.,

nn

x

uΨ=Ψ

(2)

Thus, we note the variation of the volume V of the

charge is important to the breakage mechanism as

much as it is to the transport phenomena, but from a

volumetric point of view both phenomena could be

treated in a different way. Therefore, the fraction of

the total mass broken within a tiny volume of the

charge is assumed to be

()t

σ

:

()

c

V

tad

σρ

=

∫∫∫

V

(3)

where

c

ρ

is the charge bulk density, is defined as

a mass volume of material of classes

i, so that the

flow rate of particle is:

a

()

c

VV

a

dd

dV a

dt t dt

∂ρ

σ

ρ

∂

=+

∫∫∫ ∫∫∫

dV

(4)

In worse case, where we associate to the breakage

process, the flux due to the absolute motion of the

particle, we could define the flux associate to the

fluid. However, as the mass could not be transferred

by conduction phenomena, the mass flux therefore,

vanishes, so that we could write:

i

FV

d

p

J

dF dV

dt

σ

ϑσ

=− ⋅ +

∫∫ ∫∫∫

rr

(5)

where,

i

J

r

:longitudinal diffusion flux of the mass in

class

i. ;

ϑ

:piecewise parameter;

σ

p

: local fine

particle.

Based on equation (5) for the observer design, we

assume that the mixing mechanism of powders in

ball mills can be well described by a diffusion model

and many factors such as the screen plate gape, balls

quantity and energy consumed. We deduced that, the

process could be defined as a multi-input multi-

output nonlinear system of the form:

(, ())

()

x

Fxut

ygx

=

&

(0)

o

x

x= (6)

where

is the state,

x∈Ω

r

uR

∈

is the control input:

m

yR∈

is the output; (0)

x

is the initial state.

It is assumed that,

∀

t the state trajectory ()

x

t is

defined. In addition, the function

(.)

F

is

continuously differentiable nonlinear function which

represents the dynamics of the process and the

disturbances.

We consider four state variables:

-

the material grinding rate,

1

()xt

-

the charge grindability,

2

()xt

-

the material fineness ,

3

()xt

-

the raw material hardness,

4

()xt

The output is set as follows:

1000

() ()

0010

yt xt

⎡⎤

=

⎢⎥

⎣⎦

(7)

Interactions of these parameters are not easily

identified.

Assumption 1. As the proposed function (.)

F

in (6)

is assumed to be C

1

, there exists a C

1

function

()x

ζ

such that

(, ())

x

Fx x

ζ

=

&

(8a)

Is globally asymptotically stable.

As result the system (6) could be designed in

parameterised nonlinear mapping form as follows:

(,,) (,,)xfxyuhxyu

=

+

&

(8b)

4 NONLINEAR OBSERVER

DESIGN

For a linear dynamical system in equation (9), a

well-known Luenburger basic linear observer theory

is given as follows:

() () ()

() ()

x

tAxtBut

y t Cx t

=

+

=

&

()

oo

x

tx

=

(9)

where

dim ; dim ; ; dim

x

n y m with n m u r

=

=>=

ˆˆ ˆ

() () () ( () ())

x

tAxtButKytCxt=++ −

&

(10)

The error dynamic equation is:

() ()et Aet=

%

&

As results, if the following conditions are satisfied:

Conditions

1.

matrix C has

rank m n<

2.

the pair

{

}

,CA

is completely observable

3.

a x transformation matrix T exists

so that

()nm−

n

K

CTAAT=−

%

4.

eigenvalues of the state matrix

A

%

have

negative real parts.

ICINCO 2004 - SIGNAL PROCESSING, SYSTEMS MODELING AND CONTROL

312

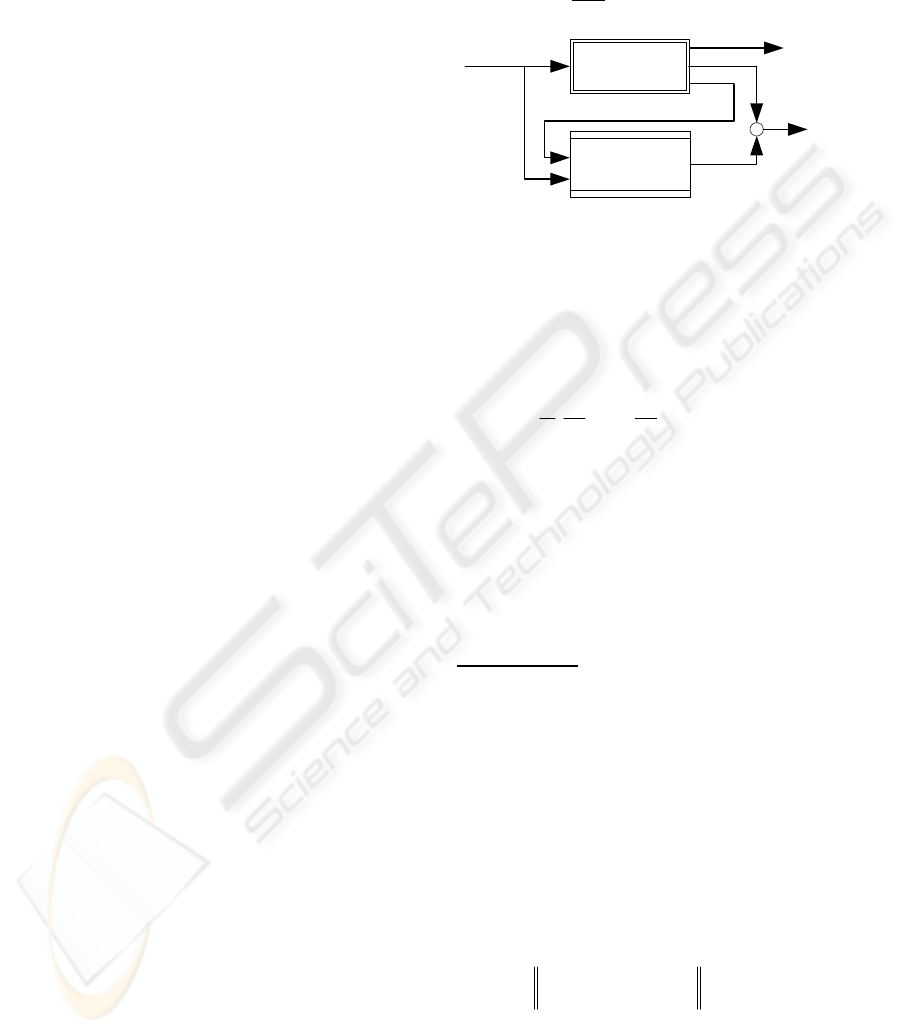
such that

(

is stable, then the state error

converges to zero. In addition we have:

)AKC−

ˆ

exx

=−

{

}

{

Re ( ) Re ( )

ji

}

A

αϕ

<

%

(11)

In nonlinear system as described in (6), stability

would not suffice as for Luenberger observers to

guaranty its applicability. Complete controllability is

required.

Even though the use of the nonlinear functions can

make the observer more efficient, treating the

system in the form as in (6) is a challenge. The

Jacobian matrices of

and in (6) with

respect to

x taken at α is used to design a

matrix

(,)Fxt (,)gxt

(,)t

α

Γ

which is a full rank, ( ) and

satisfies assumptions below. The system described

in (1)-(5) is highly nonlinear, clearly, it is difficult to

verify in practice assumptions that

,

and their respective time derivatives

are bounded. Therefore, there is a real incentive for

finding possible ways to lessen the complexity of the

computation of

.

n

R

α

∈

(,)/Fxt x∂∂

∂(,)/gxt x∂

1

ˆ

(,)xt

−

Γ

Therefore, by eliminating some redundant terms, we

are seeking an improvement of the proposed

observer design for a special class of the system

described in (6) using (8a) and (8b), for which

assumptions 1 hold. Proceeding by analogy to the

classical observer design approach in linear case for

SISO, it is possible to extend the high gain observer

design to MIMO cases, fig.4.

Keeping to the fact that the model described by

equations (5) and (6) are exactly the same as another

and have theoretical importance, the system could be

treated as a special class of nonlinear system when

unknown inputs are considered. In this sense, to

avoid our investigations becoming extremely

restricted circumstances where deficiencies become

apparent, we introduced the following representation

class to fairly well match the mill behaviour.

(,,)

x

Ax h x y u

yCx

=+

=

&

(12)

Equation (12) is valid for each state of the system.

The sufficient and necessary conditions that

characterise the function

may be found in

(Misawa, 1989). Therefore the following conditions

are assumed.

(,,)hxyu

Assumption 2: The observer state converges

asymptotically to the state of the system, so that the

state error is in the neighbourhood of zero.

Therefore, the unmodeled dynamics subsystems

have relative degree zero.

Assumption 3: The partial derivatives of

with respect to

(,,)hxyu

x

and their respective time

derivatives are bounded for all

x

and , so that:

u

(,,)

h

i

xyu

ij

x

j

∂

Ν=

∂

(13)

Process

Observer

u

y

e

-

+

ˆ

y

Figure 4: Nonlinear observer structure

We assumed

o

x

is an equilibrium point

corresponding to zero input and output,

i.e.,

()0;()0

oo

fx hx

=

= Functions (.) (.)

f

and h are

smooth.

We denote by

i

θ

δ

a diagonal matrix and A the

constant matrix in Brunowsky form:

2

11 1

( , ,........, )

i

k

ii i

diag

θ

δ

θ

θθ

=

(14)

01...0

001 0

0. ....

0 0 0 ....

A

i

⎡

⎤

⎢

⎥

⎢

⎥

=

⎢

⎥

⎢

⎥

⎣

⎦

(15)

The design of parameters

i

θ

δ

must be large enough

to compensate the system nonlinearity. Thus we

shall assume:

Assumptions 4:

a. Matrix (, ,)

x

yu

i

Γ

is full rank and

is defined as follow :

(,,)

.....

1

(,,)

C

i

Cxyu

ii

xyu

i

n

Cxy

ii

u

⎡

⎤

⎢

⎥

Ψ

⎢

⎥

Γ=

⎢

⎥

⎢

⎥

−

⎢

⎥

Ψ

⎣

⎦

(16)

where

(,,) (,,)

x

yu A xyu

iiij

Ψ=+Ν

b.

There exists a positive

constant

γ

which is independent of

θ

and

satisfies condition 4. such that:

{

}

1

sup ( , , )xyu

ii i

δ

γ

θθ

−

Γ

≤

&

(17)

STATE OBSERVER FOR NONLINEAR SYSTEMS: APPLICATION TO GRINDING PROCESS CONTROL

313

c.

(18)

1

0.0

000

(,,)

. ...

000

n

xyu

ϕ

−

⎡⎤

⎢⎥

Λ=

⎢⎥

⎣⎦

A high gain observer design for the class of

nonlinear systems in equation (12) can be stated as

follows:

1

ˆˆˆ

() () (, , ) ( )

iii

ˆ

i

x

tAxthxyu KyCx

θ

δ

−

=+ + −

&

(19)

We define the estimation error as:

ˆ

() () ()et xt xt=−

(20)

One major problem in updating the gain of observer

in equation (19) lies in the computation of the

symbolic inverse of the matrix

(, ,)

x

yu

i

Γ . In many

cases, this may become very complicated depending

on the nonlinearities involved in the system. More

precisely, at times, the matrix

ˆ

(,,)

x

yu

i

Γ may

contain excessive number of terms and

consequently, the real time implementation of the

observer may become tedious (Iwasaki, 1999). This

in turn will bring considerable simplification to the

expression of the observer’s gain.

To this end,

(,,)

x

yu

i

Γ is consider lower triangular

and non singular for all

x

and .

u

[]

12

, , .....,

T

n

x

xx x=

(21)

Based on equation (5) to express the system

described in equation (12), the improvement of the

observer in equation (19) is related to the

simplification of the gain of the observer by

elimination of the redundant terms.

For the grinding system, it is known that the motor

load depends on the load within the mill that is

tightly related to the input feed (raw material

physical properties, tailings flow rates, energy...) and

the output (flow rate, particle distribution ...). The

evolution of the charge within the mill, (the hold-up)

reproduces some unstable behaviour and is

formulated as follows:

11 11

22 212

12

(,)

(, ,)

....................

( , ,..., , )

nn n n

xAxhxu

xAxhxxu

x

Ax h x x x u

=+

⎧

⎪

=+

⎪

=

⎨

⎪

=+

⎩

&

(22)

11

22

.......

nn

yCx

y

yCx

=

⎧

⎪

=

⎪

=

⎨

⎪

=

⎩

(23)

Based on equations (8), the function

is as

follows:

(,,)hxyu

11 1

2

212 12 2

3123 23

41234 34

(,)

(, ,)

( , , , ) exp( )

(, , , ,)

hxu xu

hxxu xx xu

hxxxu xx u

hxxxxu xx u

ε

=

⎧

⎪

=+

⎪

⎨

=

⎪

=+

⎩

(24)

Based on equation (15) the matrix

(,,)

x

yu

i

Λ is

chosen such as (,,) (,,)

x

yu L xyuC

ii

Λ=

i

Similar to (, ,)

x

yu

i

Γ

we choose the

matrix (,,)Qxyu

i

as follows:

(,,)

....

1

(,,)

C

i

CA xyu

ii

Qxyu

i

n

CA xyu

ii

⎛⎞

⎜⎟

⎜

=

⎜

⎜⎟

−

⎜⎟

⎝⎠

%

⎟

(25)

where

(,,) (,,)

A

xyu A xyu

iii

=+Λ

%

Further the similarity matrix transformation for the

error dynamics is:

1

(,,) (,,) (,,)

M

xyu Q xyu xyu

iii

−

=Λ

(26)

Based on the following theorem, [4.] equation (26)

is valid.

Theorem:

Assume that system (19) satisfies assumptions

a, b,

c

.

There exist

0

o

θ

> such that

o

θ

∀≥ we have, for

all

ˆ

(0)

n

x

R∈

;

ˆ

(0) (0)xx

λ

−

≤ where

λ

is positive

[4.]. Therefore equation (19) becomes as follows:

11

ˆˆˆ

() () ( , , ) ( )

iiii

ˆ

i

x

tAxthxyuM KyCx

θ

δ

−−

=+ + −

&

(27)

Then the error dynamics is:

11

ˆ

() (,,) (, ,)

ˆ

(,,) (, ,)

i

ii

et Ae hxyu hxyu

ii

M

xyu Lxyu K Ce

θ

δ

−−

=+ −

⎡⎤

−+

⎣⎦

&

(28)

Note that the gain matrix

K

i

is chosen such that

matrices

A

KC

iii

−

is Hurwitz, i.e. (all the

eigenvalues of have negative real parts).

5 SIMULATION RESULTS

From the above equations the material grinding rate

within the mill,

; the charge grindability (i.e.,

total of material grinded per unity of energy),

;

the material fineness ,

and the raw material

hardness,

are presented as below.

1

()xt

2

()xt

3

()xt

4

()xt

ICINCO 2004 - SIGNAL PROCESSING, SYSTEMS MODELING AND CONTROL

314

By an easy manipulation of non linear equations we

could choose conveniently the steady-state values.

Others values are imposed from the model

(Nijmeijer, 1990). Practical problem observed on

industrial milling circuit is that, large changes of the

material feed hardness causes instability in the

system controlling. The values of the various

coefficients in the model have been tuned in such a

way that the model step responses fit with

experimental step responses. Thirteen tonnes of

material was initially loaded in the mill.

0

1 2

3 4

5

6

7

8

4

4.5

5.0

5.5

Fine particle

Time [h]

[%]

x

3

Figure 5: Percentage of fine particle

0

1 2

3

4

5

6

7

8

0

0.2

0.4

0.6

0.8

1

Material hardness

Time [h]

x

4

Figure 6: Material hardness variation

0

2

4

6

8

10

12

1

0

1

2

1

3

1

4

Rate of change of mass fraction of material

in 30x40 Mesh size fraction

Loading rate [t/h]

[t/h]

0

2

4

6

8

10

12

0

2

4

6

8

10

12

Material hold

-

up within the mill

Loading rate [t/h]

[t/h]

a

).

b)

.

Figure 7: Illustration of loading rate dependence of the

grinding performance

a)

Effect of solid flow rate on grinding rate

b)

Effect of solid flow rate on hold-up

Instead of trying to find a mathematical expression

of disturbances, a state observer in equation (27) can

be used to estimate it and compensate for it in real

time.

As a result, for the system in equations (22)-(24),

using equation (27) the estimation of the material

grinding rate,

; the charge portion that is under

going grinding per unity of input energy,

; the

material fineness ,

and the raw material

hardness,

is as follows:

1

ˆ

()xt

2

ˆ

()xt

3

ˆ

()xt

4

ˆ

()xt

1

2

3

4

ˆ

0100

ˆ

11 0 0

ˆ

0001

ˆ

00 11

x

Ax

x

⎡

⎤

⎡⎤

⎢

⎥

⎢⎥

−

⎢

⎥

⎢⎥

=×

⎢

⎥

⎢⎥

⎢

⎥

⎢⎥

−

⎣⎦

⎣

⎦

(29)

1

2

3

4

ˆ

000

ˆ

000

ˆ

(,,)

ˆ

00 exp()0 0

ˆ

00 0 1

x

uo

x

ad

hxyu u

x

bu

x

c

ε

⎡⎤

⎡

⎤⎡

⎢⎥

⎤

⎢

⎥⎢

−

⎢⎥

⎥

⎢

⎥⎢

=×

⎢⎥

⎥

+

⎢

⎥⎢

⎢⎥

⎥

⎢

⎥⎢

−

⎥

⎣

⎦⎣

⎣⎦

⎦

(30)

ˆ

i

y

yCx

=

−

%

(31)

11

22

33

44

ˆ

1000 1000

ˆ

1000 1000

ˆ

0100 0010

ˆ

0100 0010

yx

y

yx

⎡

⎤⎡

⎡⎤⎡⎤

⎤

⎢

⎥⎢

⎢⎥⎢⎥

⎥

⎢

⎥⎢

⎢⎥⎢⎥

=×−

⎥

×

⎢

⎥⎢

⎢⎥⎢⎥

⎥

⎢

⎥⎢

⎢⎥⎢⎥

⎣⎦⎣⎦

⎥

⎣

⎦⎣

%

⎦

i

(32)

11

ii

M

K

θ

δ

−−

ϒ=

(33)

2

1111

1

42 2

1 1 1111 12 111 1122

2

3

32 221

42

4

3 2 21 3 3 4 2 22 21 2

ˆ

1

ˆˆ ˆ

2(0.5 0.5 )

ˆˆ

exp( ) 1

ˆˆ

(2 ) 2 ( )

xku

x

xkxxxkk

xx u k

xkxxxkk

θε

θθθ

εθ

θθθ

⎡

⎤

−++ +

ϒ

⎡⎤

⎢

⎥

⎢⎥

−++ −++

ϒ

⎢

⎥

⎢⎥

=

⎢

⎥

⎢⎥

ϒ

−+ ++

⎢

⎥

⎢⎥

⎢

⎥

ϒ

⎣⎦

−+ − + +

⎣

⎦

(34)

In figures 8, 9 are shown the simulation results for

the observer and the system, while the tracking error

is shown in fig.10, 11.

STATE OBSERVER FOR NONLINEAR SYSTEMS: APPLICATION TO GRINDING PROCESS CONTROL

315

0

0.5

1

1.5

2

2.5

3

3.5

4

4.5

5

0

2

4

6

8

Rate of change of mass fraction x1

(

solid: s

y

stem

)

;

(

dashed: observer

)

tim e [h]

Grinding

rate [t/

h]

0

0.5

1

1.5

2

2.5

3

3.5

4

4.5

5

0.05

0.1

0.15

0.2

0.25

Grindability x2

(solid: system ); (dashed: observer)

tim e [h]

[t/Kwh]

a.)

b.)

Figure 8: Output response of the system and the observer

a.)

Rate of change of mass fraction

b.)

Grindability

0

0.5

1

1.5

2

2.5

3

3.5

4

4.5 5

0

5

10

15

20

Percentage of fine particle x3,

(solid: system); (dashed: observer)

time [h]

[%]

0

0.5

1

1.5

2

2.5

3

3.5

4

4.5 5

0

0.2

0.4

0.6

0.8

1

Material hardness x4,

(solid: system); (dashed: observer)

time [h]

a.)

b

.

)

Figure 9: Output response of the system and the observer

a.)

Percentage of fine particle

b.) Material hardness

0

0.5

1

1.5

2

2.5

3

3.5

4

4.5

5

0

0.01

0.02

0.03

0.04

Tracking error for the rate of change

x1-x1es

time [h]

[t/h]

0

0.5

1

1.5

2

2.5

3

3.5

4

4.5

5

0

0.02

0.04

0.06

0.08

0.1

0.12

Tracking error for grindability x2-x2es

time [h]

[t/Kwh]

Figure 10: Grindability and rate of change tracking error

6 ROBUSTNESS AGAINST

STRUCTURAL UNCERTAINTY

In practice, from the test results, correlation for

samples could not be obtained because of different

geological origins. However, experimental

relationships between dynamic elastic parameters

and Bond grindability, (Van Heerden, 1987), were

used to valid the observer simulation results. In the

dynamical analysis the dynamics of the error system,

obtained by combining the experimental process

results with the observer designed is analysed.

Fig.12 shows that the observer is stable for unknown

input.

0

0.5

1

1.5

2

2.5

3

3.5

4

4.5

5

-1.5

-1.0

-0.5

0

0.5

1.0

1.5

x 10

-

4

Tracking error for fine particle x3-x3es

time [h]

[%]

0

0.5

1

1.5

2

2.5

3

3.5

4

4.5

5

-3

-2

-1

0

1

2

x 10

-

4

Tracking error for material hardness x4-x4es

time [h]

Figure 11: Fine particle percentage and material hardness

tracking error

ICINCO 2004 - SIGNAL PROCESSING, SYSTEMS MODELING AND CONTROL

316

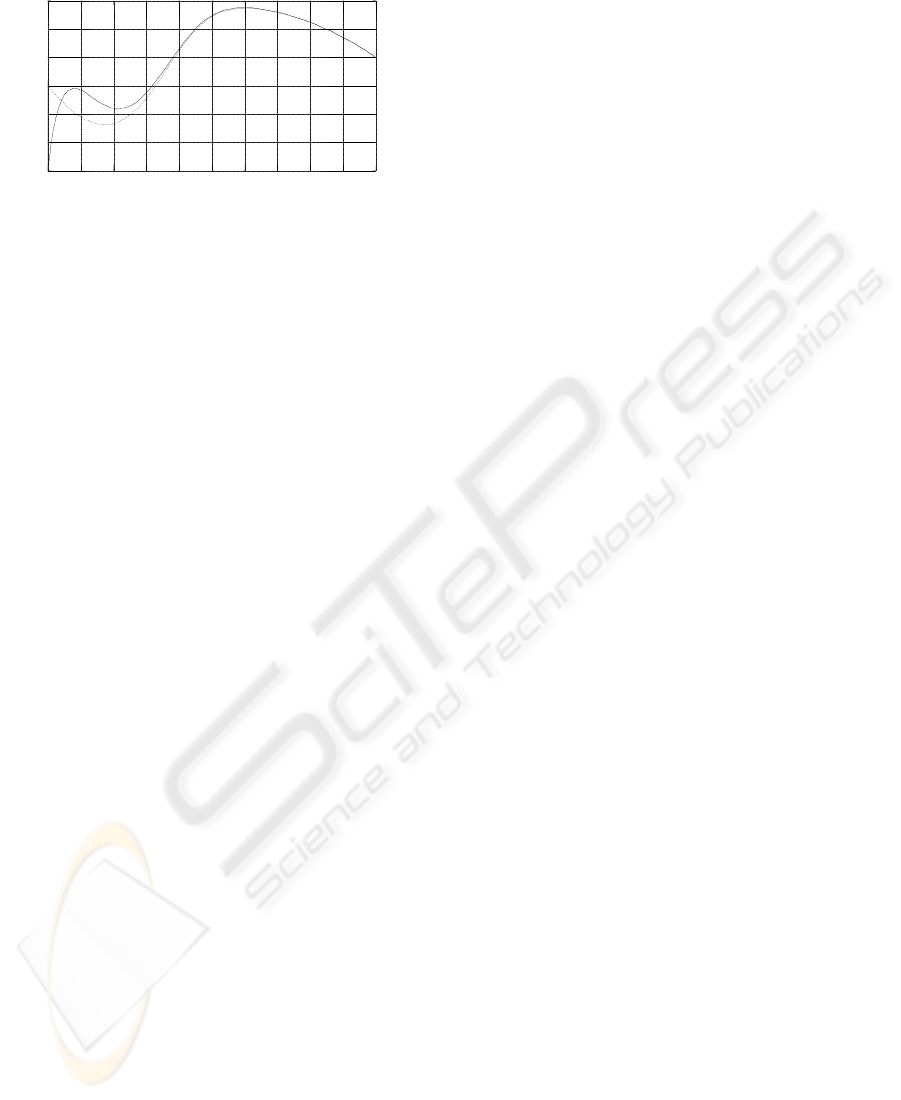
Grinding rate [t/h]

0

0.5

1

1.5

2

2.5

3

3.5

4

4.5

5

0

1

2

3

4

5

6

Rate of change of mass fraction x1, (solid: system);

(dashed: observer)

Figure 12: Observer convergence for unknown input

7 DISCUSSION

As a case study of mineral processing, the wet

grinding ball mill operated in continuous or fed-

batched mode has been studied. Simulation results

showed that significant part of the steady state error

is due to the model part and thus independent of the

observer design methodology. The robustness

against parametric and structural uncertainty can be

increased, although this will increase the noise

sensitivity. Since we herein want to track only the

truly time-varying features of the process dynamics,

the state observer designed strategy is satisfactory.

The load within the mill should be controlled at a

well chosen level because too high levels of the load

in the mill create process disturbances. The output

product fineness depends on the solids rate flow. In

view of the approximations involved in this

treatment, the agreement between the observer and

the model is remarkable. The estimation of the

observer converges to zero exponentially.

8 CONCLUSION

We have described symbolic computations for

reducing a nonlinear system to observable forms.

These tools can be applied to systems that are

linearly observable, locally observable with zero

input or merely locally observable.

The key impact of this development lies in the

system ability, to reduce material residence time, to

flow information and material in a much-improved

manner with the appropriate control strategy.

Additional elements to be considered in the

evaluation of the performance of the observer are

distributed parameters effects due to the large

sampling intervals often encountered in mineral

applications.

REFERENCES

Abou S.C., 1998. Contribution to ball mill modelling ‘’.

Doctorate thesis, Laval University, Ca,

Abou S.C., 1997. Grinding process modelling for

automatic control: application to mineral and cement

industries‘’. Engineering Foundation Conferences,

Delft, the Netherlands

Austin L. G., 1990. A mill power equation for SAG mills.

Minerals and Metallurgical Processing, pp.57-62.

Busawon K. ,Ratza M.& Hammouri H, 1998. Observer

design for a class of nonlinear systems. Int. J. Control

71, pp.405-418.

Davis, E.W., 1919. Fine crushing in ball mills. AIME

Transaction, vol. 61, pp.250-296.

Iwasaki M., Shibata, & Matsui., 1999. Disturbance-

observer-based nonlinear friction compensation in

table drive system. IEEE/ASME Trans. Mechatronic,

vol.4. pp.3-8.

Misawa E.A. & Hedrick J.K., 1989. Nonlinear observer. A

state of the art survey,. Trans. of the ASME, 111,

pp.344-352.

Morell S., 1993. The prediction of powder draw in wet

tumbling mills. Doctorate thesis, University of

Queensland.

Nijmeijer, H., & Van Der Schaft A.J., 1990. Nonlinear

dynamical control systems”. Springer Berlin.

Plummer A.R. & Vaughan N.D., 1996. Robust adaptive

control for Hydraulic Servo-systems, ASME J. of

dynamic systems, Measurement, and control. No 11.,

pp.237-244

Van Heerden, W.L., 1987. General relations between static

and dynamic moduli of rocks, (pp. 381-385). Int. J.

Rock Mech. Min. Sci. Geomech. no. 24.

Weller K. R, 1980. Automation in mining, mineral and

metal processing, Proc. 3rd IFAC symposium

Pergamon Press

. pp. 295-302

STATE OBSERVER FOR NONLINEAR SYSTEMS: APPLICATION TO GRINDING PROCESS CONTROL

317