MORPHOLOGICAL CHOICE OF PLANAR MECHANISMS IN

ROBOTICS

Peter Mitrouchev

Integrated Design Centre, "Sols, Solids, Structures - 3S" Laboratory, UMR 5521, Domaine Universitaire, B.P. n° 53,

38041 GRENOBLE Cedex 9, France

Keywords: Robot design, architecture, plan mechanisms, morphology

Abstract: In this paper a morphological confined choice for kinematic mechanisms in robotics is presented. It is based

on symmetries of structures. Pairs of groups of mutually symmetrical mechanisms are detected. Thus, the

number of possible configurations is confined by eliminating the symmetrical ones. Different cases of

symmetries have been studied. Expressions for the calculation of the number of frames and end-effectors

are presented. It enables the reduction of the number of structures by avoiding those that are isomorphic.

Following this, examples for applications for various kinematic structures are presented, enabling the field

of research to be restricted to the possible solutions.

1 INTRODUCTION

The choice of a kinematic mechanism applied in

robotics is conditioned by the number of degrees of

freedom of the task to be carried out by the robot.

The task itself imposes a kinematic chain compatible

with its number of degrees of freedom. The

kinematic chain thus imposed will be compatible

with the task if it possesses a number and type of

links and joints as those defined by the mobility and

connection laws of Mechanism and Machine Theory

(MMT). MMT supplies lists of possible

mechanisms. As there may be a large number of

these mechanisms, it is usually difficult to make a

choice amongst the available structures in the initial

design phase of the robot chain. In fact taking into

account the symmetries it can be noticed that there

are a significant number of isomorphic structures as

far as the position of the frame and of the end-

effector of the robot

. MMT contributed greatly to

planar and spatial mechanism synthesis with

different degrees of freedom ( Hervè, 1982).

The morphological (topological) synthesis of

kinematic chains has, for a long time, been the

subject of many papers. There are different methods

for the kinematic synthesis of planar chains with

simple revolute joints, with different degrees of

mobility and different numbers of links and joints.

These methods which enabled the lists of chains,

called A

lists, to be obtained are: intuition and

inspection (Crossley, 1964), graph theory

(Dobrjanskyi 1967, Woo 1967). Others consist of

transformation of binary chains (Mruthyunjaya

1984a, Mruthyunjaya 1984b) the concept of Assur

groups (Manulescu, 1987), or Franke's notation

(Davies 1966, Crossley 1966). New methods based

on genetic algorithms or neuronal networks are also

used (Chedmail 1995, Yannou 1997). These A

lists

are subdivided into many sub-lists, called B

, taking

into account the position of the frame and of the

end-effector of the robot.

The problem is how to choose amongst the

possible structures provided by MMT as far as the

position of the frame and the end-effector. The

objective is to find planar mechanisms with revolute

joints that provide guidance of a moving frame e.g.

the end-effector of an industrial robot, relative to a

base frame with a given degree of freedom. The aim

of this paper is to present a new method enabling the

reduction of the number of kinematic structures

provided by the MMT which are suitable for

robotics applications. It is based on the exploitation

of symmetries of the mechanisms. The sub-lists B

are then studied in order to extract the minimum

number of possible structures for the initial design of

kinematic chains of industrial robots, the two criteria

being the position of the frame and of the end-

effector of the robot.

193

Mitrouchev P. (2004).

MORPHOLOGICAL CHOICE OF PLANAR MECHANISMS IN ROBOTICS.

In Proceedings of the First International Conference on Informatics in Control, Automation and Robotics, pages 193-200

DOI: 10.5220/0001139801930200

 SciTePress

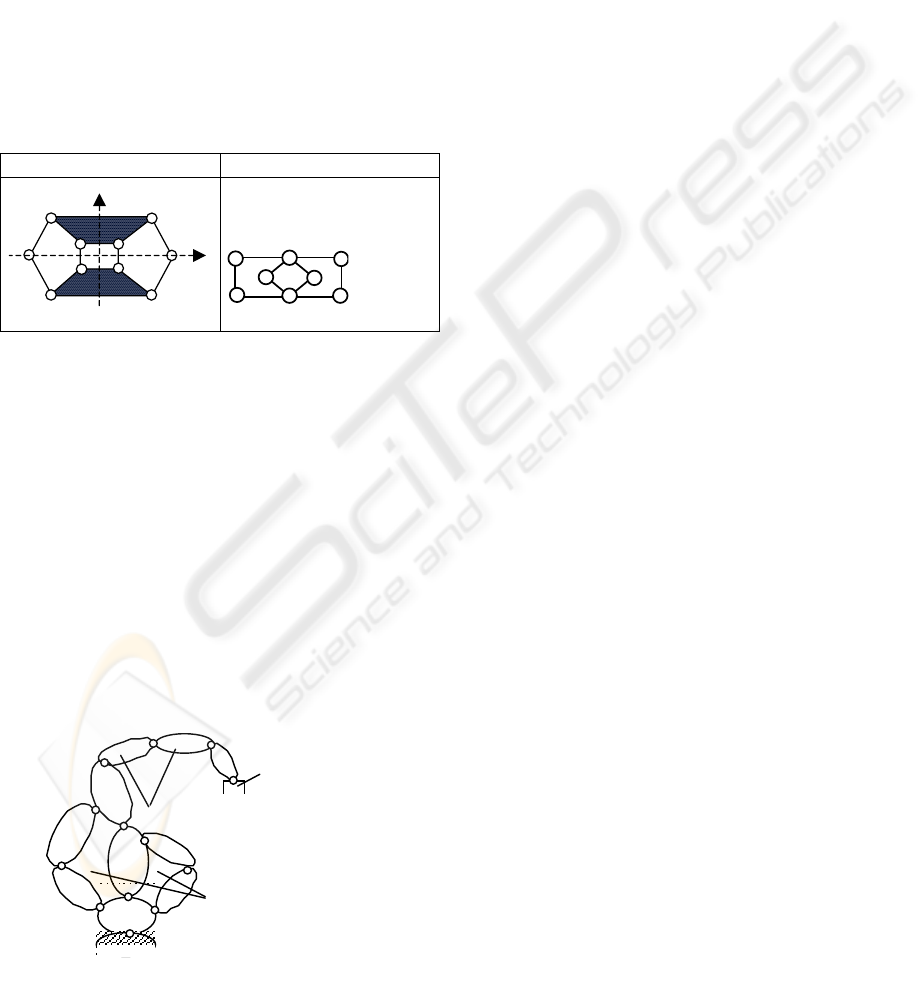
2 NOTIONS AND RESTRICTIONS

MMT proposes various ways of representing

kinematic structures. The most common, the

kinematic graph, consists in conserving a shape for

the links in order to better appraise the topology of

the structure. Nevertheless this presentation is

difficult to manage. Any kinematic structure may by

transformed into Crossley's inverse graph (Crossley,

1964) replacing every link (binary, ternary…) by a

point. The joints themselves are represented by a

line linking the points concerned. We note that the

kinematic graph expresses geometrical dimensions.

Obviously the inverse graph does not. But this letter

expresses better the symmetries of the structures if

there are any.

kinematic graph inverse graph

Figure 1: Representation of a structure by kinematic and

Crossley's inverse graph.

A robot being a complex mechanical system (MS)

characterised by a very important interaction

between its links, we define its architecture by

(Mitrouchev 1999): the main structure, which

generates the main motion of the robot and upon

which, stands the rest of the MS, the regional

structure, consisting of the arm and the forearm of

the robot (mechanical arm) and the local structure

usually consisting of three axes concurrent at one

point, and representing the wrist of the robot.

It is noted at this stage that the structure presented

by one or other ways (kinematic or inverse graph)

only presents the main structure of the robot (cf. fig.

2).

Let us consider a mechanism with M degree of

mobility, N links of any type, joined to each other by

simple revolute joints. In this paper only

mechanisms having main planar structures with

simple revolute joints usually applied in robotic

design will be studied. We note that the objective is

not to find among the mechanisms available a

particular one that fits well to a given task, but to

reduce the number of possible structures, the two

criteria being the position of the frame and the end-

effector of the robot.

3 MECHANISM DESIGN

MMT, being a part of the technological sciences, is

at the base of mechanism design in robotics. The

question is: amongst the available kinematic

structures supplied by MMT, how many of these are

suitable for application in kinematic chain design in

robotics? In order to reply to the above question it is

interesting to answer the following questions: why

and how?

Why ? Obviously it is not possible to dimension a

mechanism without being familiar with its topology.

The topological choice is normally made before the

dimensioning phase. It is this stage that presents the

most difficult problem in mechanism synthesis. It is

currently impossible to place the dimensioning

equations on the same level as the choice of

topology, because this choice is not governed by

equations i.e. assigning design variables for this or

that topology, except for: degree of mobility M,

number of links N and number of joints C (e.g. M=2,

N=7 and C=8).

How? Let us consider a list of mechanisms

provided by MMT and defined by the three

following parameters : number of links, number of

joints and number of degree of freedom. As we said,

we call this list A

list. We define also the B

list,

issued from an A

list taking into account the

position of the frame and of the end-effector. The

problem is to decide which structures may be

removed from the A

list, without restricting the

choice of available structures taking into account the

position of the frame and of the end-effector.

Let us consider an A

list of P topologies Topo i

extracted from a complete list of mechanisms with

respect to some parameters (e.g. mechanisms with

two degrees of mobility M=2, seven links N=7 and

eight joints C=8). Firstly from this list, B

sub-lists

are extracted with respect to criterion 1 "fixing a

frame" or "frame choice". Then each topology Topo

i gives several possibilities for attaching an end-

effector (pincers, paint gun, welding electrode). This

main structure

regional structure

local structure

frame

ure 2: To

olo

ical structure of a robot.

ICINCO 2004 - ROBOTICS AND AUTOMATION

194

is the second criterion "fixing an end-effector" or

"end-effector choice" with regard to the choice of

the frame used in the elaboration of the confined B

lists. For example, the B

list of fig. 3 below,

contains N.(N-1)=7.6 = 42 possible solutions but it

is noted that some of them appear twice: solutions 1

and 6, 2 and 5, 4 and 3 for the first possibility to fix

a frame. For the other possibilities it is reasonable to

suppose that there will be other dual (isomorphic)

solutions.

key: black element – frame;

- end -effector

Figure 3: Elaboration of the B

list.

4 PROPOSED METHOD

We indicate by:

- N

number of the links of a sub-chain,

- n

the number of links cut by an axis of

symmetry coincident with their axis of symmetry,

- n

the number of links not cut by an axis of

symmetry or cut, but not along their axis of

symmetry,

- n

the number of links cut solely by the axis of

symmetry a (cf. fig. 4 and figures below), or the

number of links containing the centre of symmetry

in the case of a central symmetry,

- n

the number of links cut solely by the axis of

symmetry b,

-n

the number of links cut both by the axes of

symmetry a and b,

- s the number of symmetries .

In order to present the method, various cases will

be demonstrated with respect to their type and to

their number of symmetries.

4.1 Different cases of symmetries

4.1.1 No symmetry

The simplest case is the one without any symmetry

(fig. 4a.). In this case there are N possibilities to fix

the frame. Thus Fc

= N. Concerning the position of

the end-effector there are (N-1) possibilities to attach

it for each position of the frame. Thus to each frame

choice belong (N-1) end-effector choices.

Figure 4: Structures without and with symmetries.

The structure of fig. 4a. above has the peculiarity of

having no symmetry, then:

NFc

(1)

and

)1(

−

NNEc (2)

4.1.2 Only one symmetry

We define the axis of geometrical symmetry in the

kinematic graph like the axis of topological

symmetry in the inverse graph. If a link is cut by an

axis of symmetry in the kinematic graph, the same

link is cut by the same axis (called axis of

topological symmetry) in the inverse graph.

Many mechanisms, like the structure in fig. 4b.,

have one axis of symmetry noted “a”. There are two

sub-chains 1276, 2348 and each of them has a

symmetry of links 1 and 7 for the furst sub-chain

1276 and 3 and 8 for the second one (2348). As

regards the position (choice) of the frame Fc,

symmetry dictates that the case where the frame is

on link 1 is the same as the cases when it is on 3, 7

or 8. It is the same for the links 6 and 4. Therefore

an inventory of two possibilities (solutions) are

taken to which must be added the two possibilities:

links 2 and 5. The latter two have the particularity of

being cut by the axis of symmetry. The first two

possibilities correspond to ((N-n

)/2)-1 solutions,

consequently for Fc we have:

Ai List

Bi List (42 solutions)

…

etc………………etc……………… etc…………

Topo i (M=2, N=7, C=8)

axis of symm.

MORPHOLOGICAL CHOICE OF PLANAR MECHANISMS IN ROBOTICS

195

(3)

Concerning the number of possibilities for the

attachment of an end-effector Ec, as a function of

the frame position:

- in the case where the frame is not situated

symmetrically, the number of possibilities for the

attachment of an end-effector is (N-1),

- in the case where the frame is placed

symmetrically, the same problem as the one treated

previously is found; that is to say the positions 1,3,7

and 8 are identical, as are 6 and 4. Consequently

there are (N-n

)/2+(n

-1) possibilities for Ec.

It should be noted that the number of cases when

the frame is placed symmetrically corresponds to the

number of the links cut by the axis of symmetry.

Thus for Ec we have:

()

⎟

⎠

⎞

⎜

⎝

⎛

−+

+−

−

(4)

4.1.3 Two symmetries

Two cases are to be considered for the two

symmetries.

4.1.3.1 None of the links are cut by an axis of

symmetry

The structure in fig. 5a below has two axes of

symmetry but they do not cut each other in a link.

As regards the choice of frame Fc:

- in the case of a link that is not cut, each link is

found four times by the system of symmetries, for

example links 2-1-4-5,

Figure 5: Structures with two symmetry.

- in the case of a link, which is cut, each link is

found twice, for example 7-8, 3-6, thus:

4224

=++=

(5)

Then, as regards choice Ec, working in

discriminately with symmetry a or b, requires two

identical methods (reflections):

- in the case where the frame is not situated

symmetrically, the number of possibilities to attach

the end-effector is (N-1), so there are n

/4 cases

thus described,

- in the case where the frame is placed

symmetrically with respect to symmetry a, the

number of possible solutions for the attachment of

an end-effector is (N-n

)/2+n

-1, so there are n

cases thus described.

Finally, after simplification, for Ec we have:

⎟

⎠

⎞

⎜

⎝

⎛

⎟

⎠

⎞

⎜

⎝

⎛

−+

+−

−

)1(

(6)

4.1.3.2 Links are cut by two axes of

symmetry

The structure of fig. 5.b has two axes of symmetry

that cross on a link. To fix the frame the same

reasoning as in the previous case can be adapted,

taking care to add the links cut by a and b

symmetries which are counted neither in n

nor in n

consequently:

(7)

It is the same process concerning the position of the

end-effector:

+−+

+−

−

⎟

⎠

⎞

⎜

⎝

⎛

)1(

⎟

⎠

⎞

⎜

⎝

⎛

⎟

⎠

⎞

⎜

⎝

⎛

−+++

+−+

(8)

4.1.4 Central symmetry

This is the case for the structure below. To fix the

frame, the difference between two cases must be

made:

ICINCO 2004 - ROBOTICS AND AUTOMATION

196

Figure 6: Structure with central symmetry.

- the centre of symmetry belongs to n

links, so there

are (N-n

)/2+n

possibilities,

- the centre of symmetry does not belong to a link,

so there are N/2 possibilities.

Finally for Fc we have:

= (9)

As regards the Ec choice we have:

⎟

⎠

⎞

⎜

⎝

⎛

−+

+−

−

)1(

Ec (10)

We can note that the equations (9) and (10) are the

same as the equations (3) and (4).

4.1.5 Three or more symmetries

Only one case of three symmetries is present in the

mechanisms studied (cf. fig 7.). This is the number

60 mechanism of the A

list of mechanisms, with

M=3, N=10 and C=12.

Figure 7: Structures with three symmetries.

In this particular case there are three symmetrical

sub-chains (1,2,3,4,5,6) with s=3. As regards the

position of the frame Fc (frame choice), we can note

that each sub-chain contains two symmetrical triples

of links (1,3,5 and 2,4,6) which correspond to (N

/s)

solutions. Consequently for Fc we have: Fc=

/s)=2.

In order to fix the end-effector, for a given

position of the frame, there are (N

-1)-n

solutions.

Consequently for the Fc positions of the frame we

have : E

[(N-1)- n

]=12 positions of the end-

effector.

5 GENERAL CASE

It is possible to bring together most of the equations

above in one equation as regards Fc and Ec. It is

only necessary to add the variable s indicating the

number of symmetries. As a result it is relatively

easy for the choice of the frame to present only one

formula for the cases studied above.

−

, s = 1,2 (11)

For the choice Ec:

()

++−++−

−

Ec 2

)1(

()

⎟

⎠

⎞

⎜

⎝

⎛

−++

++−++

s = 1, 2 (12)

Only one case is different, this is the case without

symmetry (s = 0) because the equations (11) and

(12) have no mathematical significance. Thus it is

found that the two equations as far as the cases

without symmetries are mainly the equations (1)

and (2).

6 EXAMPLES FOR

APPLICATIONS

In order to illustrate the expressions thus presented,

they are applied for some structures with different

degrees of mobility. As has already been said, the

proposed help does not allow the optimum solution

to be found, but reduces the field of research for this

solution. This is what will be shown by the

following examples applied to descriptions of the

main structures of industrial robots.

MORPHOLOGICAL CHOICE OF PLANAR MECHANISMS IN ROBOTICS

197

6.1 Structures with two degrees of

mobility, seven links and eight

joints

Let us consider the structure below found on an A

list provided by MMT with M=2, N=7 and C=8. The

list contains N(N-1) = 7.6 = 42 solutions (cf. fig.

3). Firstly the description file N=7, s=1, n

=1, n

=0,

=0, n

=1 is established. The created program

computes the the Fc and Ec variables. When there is

a central symmetry and not an axial one, this is

noted as a note in the respective line of the tables.

The confined B

list contains Ec=21 solutions, where

the four possibilities for Fc are distinguished (cf.

Table 1)

Amongst the twenty one possibilities, solution

number B23 from the table below was chosen by a

robot manufacturer in order to design the main

structure of the AKR-3000 robot presented in fig. 8

(Ferreti, 1981). In this case the frame was

transformed in to a quaternary link and the binary

link, where the end-effector was attached, in to a

ternary one.

Table 1: Confined Bi list of structures with two degrees

of mobility, seven links and eight joints

List B1 List B2 List B3 List B4

B11

B21

B31

B41

B12

B22

B32

B42

B13

B23

B33

B43

B24

B34

B44

B25

B35

B45

B26

B36

B46

topological structure AKR-3000 robot

Figure 8: AKR-3000 robot.

6.2 Structures with two degrees of

mobility, eleven links and

fourteen joints

The kinematic graph for a structure from an A

list

created by MMT (M=2, N=11, C=14) is presented

below (description file N=11, s=0, n

=0, n

=0,

=0). The Excel table gives the following results:

Fc=11 and Ec=110.

Amongst the one hundred and ten available

structures, a robot manufacturer has applied one

solution in order to design the main structure of the

Andromat robot presented below.

topological structure Andromat robot

Figure 9: Topological structure of Andromat robot.

ICINCO 2004 - ROBOTICS AND AUTOMATION

198

6.3 Structures with one degree of

mobility, eight links and ten joints

The table below groups the A

list of the sixteen

kinematic structures with one degree of mobility

represented by their kinematic and inverse graphs

and their description files.

Table 2: A

list of structures with one degree of mobility,

eight links and ten joints.

notat. inverse

graph

kinematic graph descrip.

file

G1-8

N=8,

s=2,

=0,

G1-8

N=8,

s=0,

=0,

G1-8

N=8,

s=0,

=0,

G1-8

N=8,

s=1,

=2,

=0,

G1-8

N=8,

s=1,

=2,

=0,

G1-8

N=8,

s=1,

=0,

G1-8

N=8,

s=1,

=4,

=0,

G1-8

N=8,

s=0,

=0,

G1-8

N=8,

s=2,

=2,

G1-8

N=8,

s=0,

=0,

G1-8

N=8,

s=0,

=0,

G1-8

N=8,

s=1,

=2,

=0,

G1-8

N=8,

s=1,

=2,

=0,

G1-8

N=8,

s=1,

=2,

=0,

G1-8

N=8,

s=2,

=4,

=0,

G1-8

N=8,

s=1,

=2,

=0,

The possible choices for the frame and the end-

effector obtained by the proposed method are

presented in table 3.

6.4 Other examples

An application under Silicon Graphics /UNIX has

been created based on the method presented.

Enabling the restriction of the number of structures

it is applied to other structures as examples with:

- one degree of mobility, ten links and thirteen

joints,

MORPHOLOGICAL CHOICE OF PLANAR MECHANISMS IN ROBOTICS

199

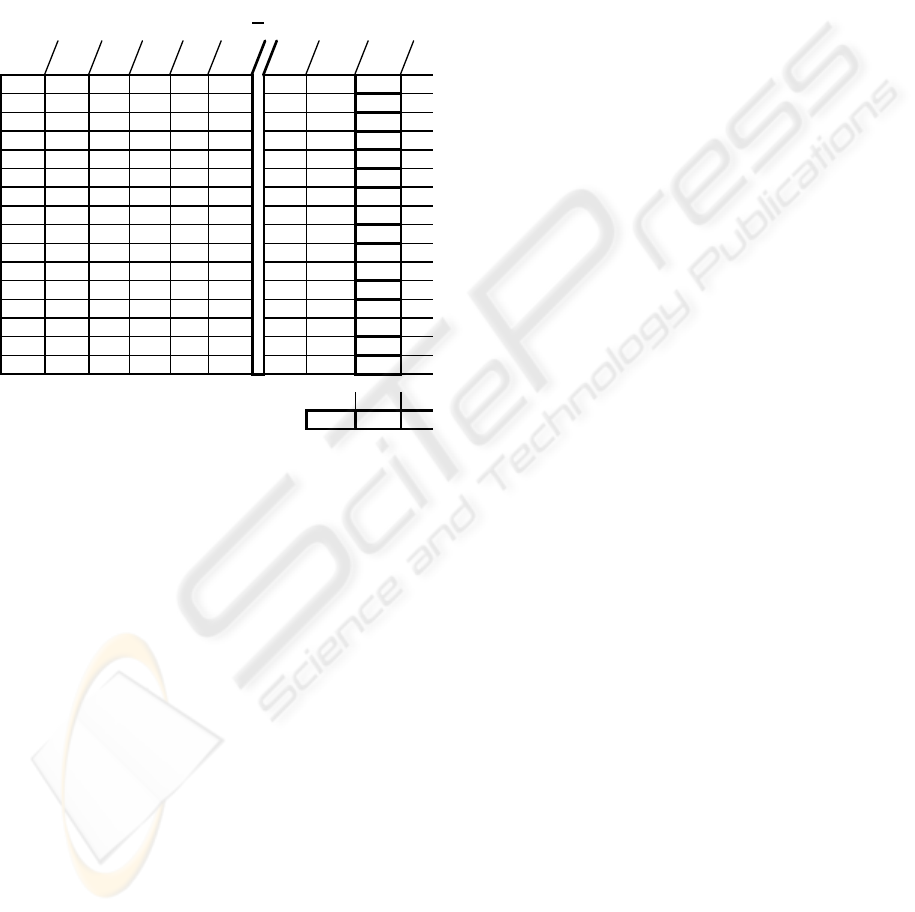
- two degrees of mobility, nine links and eleven

joints ,

- three degrees of mobility, ten links and twelve

joints.

In the majority of cases the kinematic structure

has axial symmetry. This symmetry is not mentioned

in the tables presented. However, the central

symmetry (not as much presented in the structures)

is mentioned, as previously stated in paragraph 6.1.

Table 3: Frame and the end-effector choices.

Structures with one degree of mobility, eight links and ten joints

n°

182242 0 214

280000 0 856

380000 0 856

481240 2 529

581240 2 529

681002 0 321

781250 4 210

880000 0 856

982250 4 315

10 8 0 0 0 0 0 8 56

11 8 0 0 0 0 0 8 56

12 8 1 0 0 0 2 5 29

13 8 1 2 5 0 2 6 34

14 8 1 2 4 2 2 4 16

15 8 2 2 4 2 4 3 17

16 8 1 2 5 0 2 5 29

tota l tota l

83 518

7 SUMMARY

The method presented in this paper enables the

morphological restriction for planar kinematic

mechanisms in robotics by avoiding those that are

isomorphic. It is based on the exploitation of

symmetries. The different cases of symmetry studied

provide expressions allowing the number of possible

structures to be calculated as regards the position of

the frame and of the end-effector. The proposed

expressions are then applied to different examples.

The results of this study may be useful to robot-

designers enabling them to limit the field of research

to the possible solutions.

REFERENCES

Chedmail, P., Ramstein E., 1995. Synthèse des

mécanismes et algorithmes génétiques, Journée

PRIMECA, Toulouse, pp.7-14.

Crossley, F. R. E., 1964. A cotribution to Grubler's theory

in the number synthesis of plane mechanisms, ASME,

Journal of Engineering for Industry, pp.1-8.

Crossley, F. E., 1966. On an unpublished work of alt, Jour.

Mechanisms,

1, pp.165-170.

Davies, T., Crossley F.E., 1966. Structural analysis of plan

linkages by Franke’s condensed notation, Jour.

Mechanisms,

1, pp.171-183, Pergamon Press.

Dobrjanskyi, L., Freudenstein, F., 1967. Some application

of graph theory to the structural analysis of

mechanisms, Journal of Engineering for Industry,

pp.153-158.

Ferreti, M., 1981. Panorama de 150 manipulateurs et

robots industriels, Le Nouvel Automatisme, n°

26,

Novembre-Décembre 1981, pp.56-77.

Hervè, L.M., 1982. Intrinsic formulation of problems of

geometry and kinematics of mechanisms, Mech. and

Mach. Theory,

17, N° 3 , pp.179-184.

Hwang, W-M., Hwang, Y-W., 1992. Computer-aided

structural synthesis of plan kinematic chains with

simple joints, Mech. Mach. Theory,

27, n°2, pp.189-

199.

Manolescu, N. et al., 1987. Structural and Kinematic

Synthesis of Planar Kinematic Chain (PKC) and

Mechanisms (PM) with Variable Structure During the

Work, Proc. of the 7-th World Congress, The Theory

of Mechines and Mechanisms,

1, 17-22 September

1987, Sevilla, Spain, pp. 45-48.

Mitrouchev P., André P. , 1999. Méthode de génération et

description de mécanismes cinématiques plans en

robotique. Journal Européen des Systèmes

Automatisés, ISSN : 0296-1598, (vol. 33, n°3) - avril

1999, pp. 285-304.

Mruthyunjaya, T.S., 1984a. A computerized methodology

for structural synthesis of kinematic chains : Part 1-

Formulation, Mech. Mach. Theory,

19, No.6, pp. 487-

495.

Mruthyunjaya, T.S., 1984b. A computerized methodology

for structural synthesis of kinematic chains : Part 2-

Application to several fully or partially known cases,

Mech. Mach. Theory,

19, No.6, pp. 497-505.

Woo, L. S., 1967. Type synthesis of plan linkages, Journal

of Engineering for Industry, February 1967, pp.159-

172.

Yannou B.,Vasiliu, A., 1997. Synhèse dimensionnelle de

mécanismes générateurs de trajectoires par réseaux de

neurones, 5

-ème

Colloque PRIMECA, La Plagne,

1997, pp.315-323.

ICINCO 2004 - ROBOTICS AND AUTOMATION

200