GUARANTEERRING SECURITY OF FINANCIAL TRANSACTION

BY USING QUANTUM CRYPTOGRAPHY IN BANKING

ENVIRONMENT

Solange Ghernaouti-H

elie and Mohamed Ali Sfaxi

HEC - University of Lausanne

1015 Switzerland

Keywords:

Security guarantee, Quantum cryptography, Key management, secure ﬁnancial transactions, IPSEC, perfor-

mances.

Abstract:

Protocols and applications could proﬁt of quantum cryptography to secure communications. The applications

of quantum cryptography are linked to telecommunication services that require very high level of security

such as bank transactions.

The aim of this paper is to present the possibility to use quantum cryptography in critical ﬁnancial transactions,

to analyse the use of quantum key distribution within IPSEC to secure these transactions and to present the

estimated performances of this solution.

After having introduced basic concepts in quantum cryptography, we describe a scenario of using quantum

key distribution in bank transactions in Switzerland. Then, we propose a solution that integrate quantum

key distribution into IPSEC. A performance analysis is done to demonstrate the operational feasibility of this

solution.

1 INTRODUCTION

Banks and ﬁnancial establishments need to secure

transaction and communication between them and

their clients. In fact, everyday thousand of million

dollars transactions are performed between banks.

This transmission must be secure and need to satisfy

security requirement such as authentication, conﬁden-

tiality and integrity. Quantum cryptography could be

used, in this context, to offer unconditional secure

communication.

The next section presents a scenario for quantum

cryptography application to secure bank transaction

over Internet and Intranet architectures. Then, we

prove the feasibility of the use of quantum cryptog-

raphy within the framework of IPsec.

Quantum cryptography could be applied to IP Se-

curity protocol (IPsec) [RFC 2401]. This protocol is

related to a collection of standards that was designed

speciﬁcally to create secure end-to-end secure con-

nections. The standard was developed by the Internet

Engineering Task Force (IETF) to secure communi-

cations over both public and private networks.

2 QUANTUM CRYPTOGRAPHY

FOR BANKS AND FINANCIAL

ESTABLISHMENTS

Nowadays, Banks and ﬁnancial institutions use either

symmetric cryptography or asymmetric cryptography.

Both techniques, as proved above, are not uncondi-

tionally secure. So transactions could be corrupted

and altered without the awareness of the bank. This

constitutes a serious danger because criminals and

malicious organizations could proﬁt of the breach to

steal and highjack. Securing critical ﬁnancial transac-

tion is mandatory and will be more and more neces-

sary to master economical crime.

2.1 Quantum Cryptography solution

To ensure maximum security, we need to maximize

the security in each ﬁeld such as storage, generation,

processing and transmission of data. In this para-

graph, we will focus on securing transmissions. The

transmissions are either from bank building to another

bank building of the same company, from cash dis-

penser to bank and from a bank to another. The dif-

ference resides in the distance, the degree of security

268

Ghernaouti-Hélie S. and Ali Sfaxi M. (2005).

GUARANTEERRING SECURITY OF FINANCIAL TRANSACTION BY USING QUANTUM CRYPTOGRAPHY IN BANKING ENVIRONMENT.

In Proceedings of the Second International Conference on e-Business and Telecommunication Networks, pages 268-274

DOI: 10.5220/0001414102680274

 SciTePress

required and the duration and the quantity of infor-

mation to send. Quantum cryptography ensures the

unconditional security of transmission and the aware-

ness if an eavesdropper tries to intercept or modify

the content of the transmission. Quantum cryptog-

raphy aims exploiting the laws of quantum physics

in order to carry out a cryptographic task. The un-

certainty relations of Heisenberg can in particular be

exploited to implement communication channels that

cannot be passively - i.e. without disturbance of the

transmission - eavesdropped. Its legitimate users can

detect eavesdropping, no matter what technology is

available to the spy (Bennet1984; Gisin2002).

The power of quantum cryptography lies primarily

in the fact that the keys distributed on the quantum

channel are invulnerable to eavesdropping and can

be guaranteed without assumptions on the comput-

ing power of an eavesdropper (Mayers1998; Lo1999).

Banks can actually use quantum cryptography at least

in two of the three types of transaction: bank build-

ing to another bank building of the same bank com-

pany or/and cash dispenser to the bank. In fact, we as-

sume that the distance connecting two bank buildings

in less than 100 km. So, the use of quantum cryptog-

raphy based on optical ﬁber is possible (IdQuan2004).

In this case, either a big amount of data could be ex-

change or a tiny amount of data that could be transmit-

ted frequently between bank buildings. Transmission

from cash dispenser to banks (if the cash dispenser is

not in the bank) can also be done using quantum cryp-

tography based on optical ﬁber. In fact, the danger is

that a malicious person could intercept the communi-

cations between the bank and the cash dispenser and

modify them like for instance credit some bank ac-

count or change the identiﬁcation of debited account.

Transaction, using quantum cryptography, would be

at that moment unconditionally secure and no one can

intercept them. Here we ensure the integrity and the

conﬁdentiality of the transmitted data.

Figure 1: Swiss bank - application of quantum cryptogra-

phy

2.2 Example of a bank scenario

In this paragraph, we present a scenario of quantum

cryptography implementation in Switzerland (small

country size). The bank company is called Swiss

Bank (SB). We assume that each bank have a head

quarter in every Canton. A main data base is located

in Zurich and every head quarter bank has to commu-

nicate all transaction to the data base in Zurich. SB

installs optic ﬁber between some head quarters in or-

der to create a ”private” quantum network (Figure 1).

At least, each head quarter has a quantum cryptogra-

phy receiver/sender. In order to reduce the volume of

exchanged data every 6 hours all head quarters send

data about transaction to Zurich (6h30, 12h30, 18h30

and 0h30).

The steps are the following:

All head quarters gather all transactions realized in

the Canton. Bank head quarter located in the far-

thest Cantons from Zurich sends their adjacent can-

ton. For instance, Lugano’s SB head quarter sends

data to Bellinzona, Poschiavo sends to Brigue... us-

ing quantum cryptography. These ”nodes” has to wait

until all adjacent cantons send have ﬁnished sending

data (or time-out) then decrypt data and send them

to the following BS head quarter according to a list.

Finally, the nearest and direct linked to Zurich head

quarters (Winterthur, Zoug, St-Gall, Coire...) ex-

change keys and communicate in a secure way using

quantum cryptography.

Every head quarter (say H) has two different lists.

Reception list: it is a list of all the head quarters that

send data to H. Send List: usually it contains only one

head quarter (the nearest to Zurich) but for availability

purpose it contains 2 BS head quarters.

Example of such lists (for Bellinzona):

Reception list Send list

Lugano Coire

St-Moriz

Ascona

The possible cost of such scenario is:

The optical ﬁber total length: about 2000 Km

Number of quantum cryptography station: (twice the

number of links) about 80

The cost of optical ﬁber per meter = 6 CHF

The cost of 2 quantum cryptography station = 150,000

CHF

The total cost of the scenario is 12000000 + 6000000

= 18 Million CHF 12 Million Euros.

So the price to ensure an unconditional secure trans-

mission is about 12 Million Euros.

This cost is huge but if we estimate the prestige gain

(in the image, the reputation and in term of conﬁ-

dence) of the bank this expense is justiﬁable. This

long term investment will be beneﬁcial to the bank.

GUARANTEERRING SECURITY OF FINANCIAL TRANSACTION BY USING QUNATUM CRYPTOGRAPHY IN

BANKING ENVIRONMENT

269

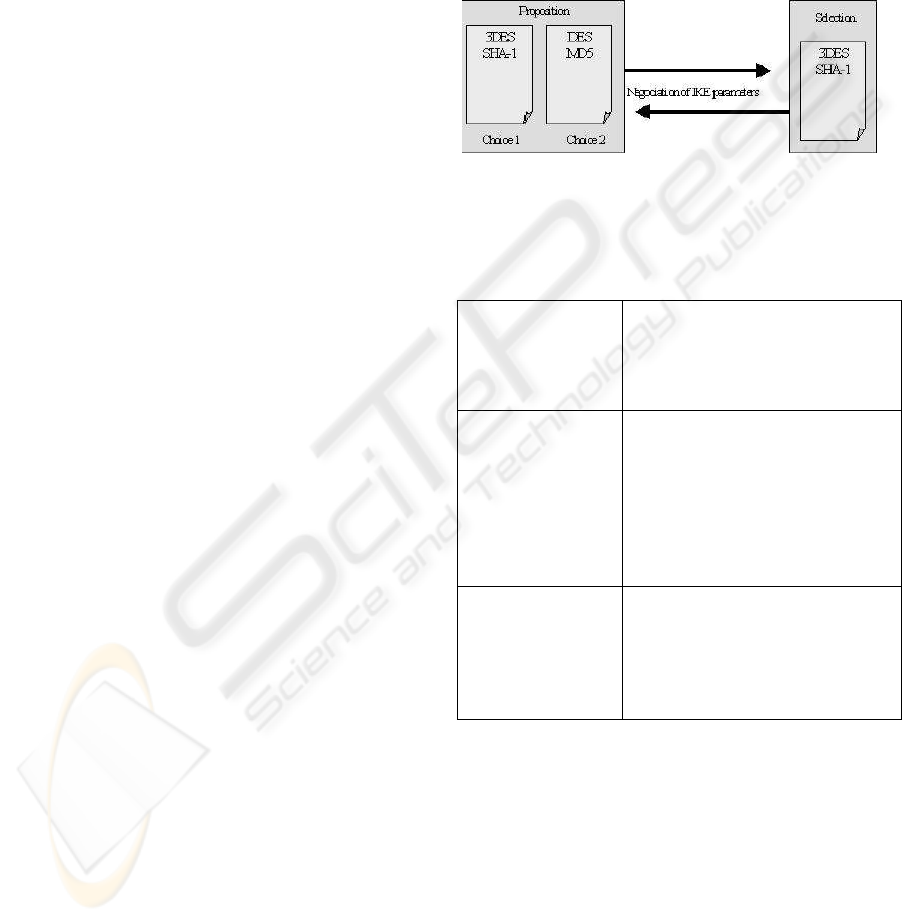
To apply this solution, we need to use algorithms

and protocols. IPsec could support the use of quan-

tum cryptography. We present the feasibility and the

theoretical performances of such application.

3 SEQKEIP OPERATING MODE

As IPsec uses classical cryptography to secure com-

munication, in this paragraph, we propose to use

quantum cryptography to replace the classical cryp-

tographic protocols used for symmetric distribution.

Using QKD in IPsec has already been proposed

and implemented by Elliot of BBN technologies (El-

liott2002). It proposes the idea of using QKD in IPsec

as Key generator for AES. In 2003, BBN technologies

describes the possibility of integrating QKD within

the standard IKE (Elliott2003) and announces some

concerns linked to the compatibility of QKD with

IKE. In our paper, we propose a QKD solution for

IPsec called SEQKEIP that is not based on IKE but

on ISAKMP. Using this method, we avoid the prob-

lem of compatibility between IKE and QKD.

The idea is to stick to the traditional IPsec and the

Internet Security Association and Key management

Protocol (ISAKMP). In fact, ISAKMP does not im-

pose any condition to is the negotiation mechanisms

or to the SAs parameters. To use quantum cryptog-

raphy with IPsec we have simply to deﬁne the two

phases described above. We create a Secure Quan-

tum Key Exchange Internet Protocol (SeQKEIP). The

SeQKEIP like IKE uses ISAKMP mechanisms and

takes advantage of quantum cryptography in order to

build a practical protocol.

SeQKEIP runs nearly like the IKE. It includes

3 phases: the phase 1 for the negotiation of the

ISAKMP SA, phase 2 for the negotiation of SA and

we add a phase called ”phase 0” in which Alice

and Bob will share the ﬁrst secret key. There are

only three modes in SeQKEIP: Quantum Mode, Main

Mode and Quick mode. Quantum mode is the quan-

tum cryptography key exchange in the phase 0. Main

Mode is used during the phase 1 and Quick Mode is

an exchange in phase 2. Both the Main Mode and the

Quick Mode are nearly the same of those in IKE.

Phase 0: Key exchange - Quantum Mode

This phase is the beginning of the secure exchange

using quantum cryptography. After, these exchange

both the sender and the receiver share a secret key.

This key constitutes the pre-shared secret in IKE

mechanism.

Phase 1: Negotiation of ISAKMP SA - Main Mode

During this phase, the cryptographic algorithm and

the hash function are negotiated. Only the two pa-

rameters discussed in the phase 1 constitute the Se-

QKEIP attribute. The method to authenticate is

the pre-shared secret (the secret key exchanged with

Quantum Key Exchange method). Contrarily to IKE,

SeQKEIP do not deﬁne DH groups and do not need

to use digital signature nor digital certiﬁcates (Figure

2). No cryptographic key are generated in this phase.

The ﬁrst exchanged key is used to encipher packets

and to authenticate users.

Figure 2: Message exchanged during the ﬁrst phase

After the phase 0 and the phase 1, both sender and

receiver will have the following information:

Shared secret key This key is generated during the

phase 0 with Quantum Key ex-

change mechanism. The secret

key is used to authenticate users

and to encrypt packet.

Encryption algo-

rithm

The encryption algorithm is ap-

plied to the phase 2 (negotia-

tion of SA parameters). The al-

gorithm could be 3DES, DES,

AES. But, if we want to have

the maximum security, we have

to use One-Time-Pad function

(OTP).

Hash function The hash function will give the

opportunity to the sender and

the receiver to check the in-

tegrity of the message and the

authentication of the correspon-

dents.

Note that the phase 0 and the phase 1 are totally

independent and could be done at the same time. We

need the secret key only from the phase 2.

Phase 2: Negotiation of SA - Quick Mode

As in IKE, the exchanged messages in phase 2 are

protected in authentication and conﬁdentiality by the

negotiated parameters of the phase 1 and phase 0. The

authentication is guaranteed by the addition of the

HASH block after the ISAKMP header and the con-

ﬁdentiality is ensured by the encryption of the whole

message blocks. The aim of this phase is to nego-

tiate the SA. i.e. to negotiate the ”IPsec” parame-

ICETE 2005 - SECURITY AND RELIABILITY IN INFORMATION SYSTEMS AND NETWORKS

270

ters. The SA parameters are (Mason2002): Desti-

nation address, Security Parameter Index (SPI), the

security mechanism (AH or ESP) and encryption &

Hash function, the session key and additional attribute

like the lifetime of SA.

For SeQKEIP, to extend security, we can use One-

Time-Pad encryption function. The ﬁrst exchanged

key, in this case, will have the length of the message.

We do not need thus any encryption algorithm for SA.

We still need a Hash function to verify the integrity of

the data. The run of IPsec could be modiﬁed in order

to use one-time-pad function.

In the beginning (Figure 3), the phase 0 and the

phase 1 start (1&2). After these two phases the para-

meters of the protocol are ﬁxed. In (3), we will use

key exchanged thanks to quantum cryptography. This

key will be used either as a session key (4) or in the

one-time-pad function (4’).

Figure 3: Functioning of IPsec with Quantum Cryptography

In (4), we use traditional symmetric cryptography

algorithms to exchange data. The IPsec packets are

the same as without the use of quantum cryptography.

The session key, therefore, is exchanged using quan-

tum key exchange. The lifetime duration of the ses-

sion key is very short and it is equal to the time needed

to exchange the secret key using quantum cryptogra-

phy. This solution is a transition solution to the (4’)

In (4’), we use quantum cryptography concepts to-

tally. The idea is to shift completely to the uncon-

ditional secure functions .i.e. quantum key exchange

and one-time-pad function. After ﬁxing the SA para-

meters, the ”session” keys length will be of the size

the data in the IPsec packet. Then, it is possible to use

one-time-pad function (simply perform an XOR of

the message and the key and then send the result). We

need to exchange key for every packet. The weakness

of this solution resides in the time needed to exchange

the key. The total bit rate is highly affected due to this

problem but as the quantum cryptography technology

is progressing, this issue will soon be solved.

There are two possibilities. The ﬁrst case is to

exchange the key and distillation using the quantum

channel (Time division multiplexing). The other is to

exchange only the key over the quantum channel and

all the other data over the public channel (Figure 4).

Figure 4: the two cases of using totally quantum cryptogra-

phy in IPsec

K: the duration to exchange the quantum key

D: the duration of key distillation

T: the duration of transmitting the message

1-ﬁrst solution:

In this case, we propose to use the quantum channel

to exchange the key and for distillation. There are two

possibilities: K+D is greater than T (K+D>T) and

K+D is less or equal to T (K+D≤T).

The effectiveness (θ) of this solution is given by ( θ

represents the difference between the use of quantum

cryptography and the use of unenciphered transmis-

sion):

a- K+D > T

If K+D>T then

θ =

T × N

(K + D) + T × N + (N − 1) × ((K + D) − T )

(1)

GUARANTEERRING SECURITY OF FINANCIAL TRANSACTION BY USING QUNATUM CRYPTOGRAPHY IN

BANKING ENVIRONMENT

271

Where N is the number of packet.

θ =

T × N

(K + D) + T × N − (N − 1) × T + (K + D) × (N − 1)

(2)

Finally, after simpliﬁcation:

θ =

T × N

T + (K + D) × N

(3)

If N is very large (inﬁnite), θ is equal to:

lim

N →∞

θ =

K + D

(4)

Example 1 Traditionally, the size of MTU (Maximum

Transmission Unit) is 1500 bytes (i.e. 12 Kbits); we

suppose that the unprotected header size is 250 bytes,

so we have to secure 1250 bytes i.e. 10 Kbits. There-

fore, the key length will be 10Kbits if we want to use

One-Time-Pad function. The ﬂow rate to exchange

the key is 1 MBit/s and about 100 MBit/s to exchange

normal data on optical ﬁber. We suppose that we

have an Internet connection of 1 Mbit/s. As the error

rate for exchanging quantum key is normally 50%, we

need to exchange 20 Kbits in order to get 10Kbits of

key length. We estimate the distillation data to be 40

Kbits. The time to XOR data with the key is neglected.

Having the previous assumption:

K = 20/1000 = 0.02 s

D = 40/100000 = 0.0004 s

And T = 12/1000 = 0.012 s

In this case, K+D (20.4 ms) is greater than T (12

ms). The effectiveness θ when the number of packet

N is inﬁnite (4) is equal to 120/204 ˜60 % of the total

performance.

NB: if we have a faster Internet connection, say

10Mbit/s, the effectiveness θ given by (4) will be

equal to 6 % of the total performance. In this case,

the use of SeQKEIP is useless if we see only the per-

formance. But, as the rate of quantum key exchange

is progressing the effectiveness will increase.

b- K+D≤T

If K+D≤T then

θ =

T × N

(K + D) + T × N

(5)

if N is very large (inﬁnite), θ is equal to:

lim

N →∞

θ =

= 1 (6)

So, in this case, there is no difference in the per-

formance between using SeQKEIP and IP. The addi-

tional time cost induced by the use of quantum cryp-

tography is negligible.

2-second solution

The quantum channel is used only to exchange the

key. The distillation is done over the public channel.

There are also two possibilities depending on the time

needed to exchange the key and, on the other hand, the

time to validate and send the message.

We take the same notation as previous:

K: the duration to exchange the quantum key

D: the duration of the key distillation

T: the duration of transmitting the message

So, we distinguish two scenarios: when K > D+T

and K ≤T+D.

a- K > D+T

If K>T+D then

θ =

T × N

K + (T + D) × N + (N − 1) × (K − (D + T ))

(7)

And, after simpliﬁcation:

θ =

T × N

(T + D) + K × N

(8)

if N is very large (inﬁnite), θ is equal to:

lim

N →∞

θ =

(9)

Example 2 We take the same parameters as in the

”NB” the previous example (10 Mbit/s for the Internet

connection, 1Mbit/s to exchange the quantum key).

Having the previous assumption:

K = 20/1000 = 0.02 s

D = 40/10000 = 0.004 s

And T = 12/10000 = 0.0012 s

In this case, K (20 ms) is greater than T +V (42 ms).

The effectiveness θ if the number of packet N is inﬁnite

(9) is equal to 12/200 = 6 % of the total performance.

The ﬂow rate conﬁguration is the both solutions

gives the same performance rate (6 %) of the whole

performance. To upgrade this rate, the only solution

is to have the K ≤T+D in this case and K+D ≤T in

the previous solution.

b- If K≤T+D

If K≤T+D then

θ =

T × N

K + (T + D) × N

(10)

if N is very large (inﬁnite), θ is equal to:

ICETE 2005 - SECURITY AND RELIABILITY IN INFORMATION SYSTEMS AND NETWORKS

272

lim

N →∞

θ =

T + D

(11)

If we take the following conﬁguration: the rate of

quantum key exchange is 1Mbit/s and the Internet

connection is 1Mbit/s, then T= 0.012 s and D = 0.04s.

T+V is greater than K (0.02 s). So, the effectiveness

θ if the number of packet N is inﬁnite (11) is equal to

12/52 = 23 % of the total performance.

4 CONCLUSION

Classical cryptography algorithms are based on math-

ematical functions. The robustness of a given cryp-

tosystem is based essentially on the secrecy of its (pri-

vate) key and the difﬁculty with which the inverse of

its one-way function(s) can be calculated. Unfortu-

nately, there is no mathematical proof that will es-

tablish whether it is not possible to ﬁnd the inverse

of a given one-way function. On the contrary, quan-

tum cryptography is a method for sharing secret keys,

whose security can be formally demonstrated.

As we have seen, using quantum cryptography in

conjonction with IPsec to offer a better level of se-

curity for organisations is possible. If, we apply the

quantum key exchange and one-time-pad function,

we reach the unconditional security in communica-

tion. The distillation of the quantum key could be

done in two different ways: over the optical chan-

nel or over the public channel. The cost of installing

this solution stills expensive nowadays. The perfor-

mance obtained when distilling the key over the op-

tical channel is higher than when using public chan-

nel (up to 100% when using optical channel versus

23% when using public channel). Actually, we can

reach 100Kbit/s when exchanging the quantum key

and hope to reach 1Mbit/s next few years. The possi-

ble ﬂow rate over an optical ﬁber is 100Mb/s. If, we

use an Internet connection of 1Mbit/s, we get 60% of

the total performance (solution1, a) i.e. a ﬂow rate

of 600Kbit/s if the distillation of the key is done over

the optical channel and we get only 23% of the to-

tal performance if we validate the key over the public

channel (solution 2, b) i.e. a ﬂow rate of 230Kbit/s. If

we could reach the rate of 10Mbit/s in quantum key

exchange and we use the ﬁrst solution, we will get a

performance of 100% in the ﬂow rate i.e. 1Mbit/s.

ACKNOWLEDGEMENT

This work has been done within the framework

of the European research project : SECOQC -

www.secoqc.net. We would like to thank IDQuan-

tique S.A (www.idquantique.com) and specially Gre-

goire Ribordy and Olivier Gay for their help and use-

ful information.

REFERENCES

Ghernaouti-H

elie, S; Sfaxi, M.A; Hauser, A; Riguidel,

M;All

eaume, R (2004). “Business model: advan-

tages, scenarios, patents and laws related to quantum

cryptography”. Secoqc project deliverable.

All

eaume R (2004). “R

ealisation exp

erimentale de sources

de photons uniques, caract

erisation et application

a la

cryptographie quantique” (Secoqc partner)

Bennet, C; Brassard, G (1983). IEEE International Confer-

ence on Computers, Systems, and Signal Processing.

IEEE Press, LOS ALAMITOS

Bennet, (1992). C Quantum Cryptography: Uncertainty in

the Service of Privacy. Science 257.

Donald S.Bethune and William P.Risk (2002).

”AutoCompensating quantum cryptography”.

New journal of physics 4 (2002)42.1-42.15

URL: http://www.iop.org/EJ/article/1367-

2630/4/1/342/nj2142.html

Clark, C. W; Bienfang, J. C; Gross, A. J; Mink, A; Her-

shman, B. J; Nakassis, A; Tang, X; Lu, R; Su, D. H;

Williams, C. J; Hagley E. W; Wen, J (2000). ”Quan-

tum key distribution with 1.25 Gbps clock synchro-

nization”, Optics Express.

Artur Ekert (1991). “Quantum Cryptography based on

Bell’s Theorem”. Physical Review Letters. URL:

http://prola.aps.org/abstract/PRL/v67/i6/p661

Elliott, C (2002). ”Building the quantum network”. New

Journal of Physics 4 (46.1-46.12)

Elliott, C; Pearson, D; Troxel, G (2003). ”Quantum Cryp-

tography in Practice”.

Freebsd people. ”IPsec outline”. URL:

http://people.freebsd.org/˜julian/

IPsec

4 Dummies.html

freesoft (2004). ”IPsec Overview”. URL:

http://www.freesoft.org/CIE/Topics/141.htm

Gisin, N; Ribordy, G; Tittel, W; Zbinden, H. (2002).

”Quantum Cryptography”. Reviews of Modern

Physics 74 (2002): http://arxiv.org/PS

cache/quant-

ph/pdf/0101/0101098.pdf

Grosshans,Van Assche, Wenger,Brouri,Cerf,Grangier

(2003). ”Quantum key distribution using gaussian-

modulated coherent states” Letter to nature. URL:

http://www.mpq.mpg.de/Theorygroup/CIRAC-

/people/grosshans/papers/Nat421

238.pdf

R.Hughes,J.Nordholt,D.Derkacs,C.Peterson, (2002).

”Practical free-space quantum key distribu-

tion over 10km in daylight and at night”. New

journal of physics 4 (2002)43.1-43.14.URL:

http://www.iop.org/EJ/abstract/1367-2630/4/1/343/

Labouret, G (2000). ”IPsec: pr

esentation technique”. Herv

Schauer Consultants (HSC). URL : www.hsc.fr

GUARANTEERRING SECURITY OF FINANCIAL TRANSACTION BY USING QUNATUM CRYPTOGRAPHY IN

BANKING ENVIRONMENT

273

Lo, H.K; Chau, H.F. (1999). ”Unconditional se-

curity of quantum key distribution over

arbitrarily long distances”. Science 283:

http://arxiv.org/PS

cache/quant-ph/9803/9803006.pdf

Mason A, (2002). ”IPsec Overview Part Five:

Security Associations”. Cisco Press. URL:

http://www.ciscopress.com/articles/

printerfriendly.asp?p=25443

Mayers, D (1998). “Unconditionnal Security in Quantum

Cryptography”. J. Assoc. Comput. Math. 48, 351

Paterson, K.G; Piper, f; Schack, R (2004). ”Why Quantum

Cryptography?”. http://eprint.iacr.org/2004/156.pdf

Riguidel, M; Dang-Minh, D; Le-Quoc, C; Nguyen-Toan,

L; Nguyen-Thanh, M (2004). ”Quantum crypt- Work

Package I”. ENST/EEC/QC.04.06.WP1B. (Secoqc

partner)

Rivest, R.L; Shamir, A; Adleman, L.M (1978). ”A Method

of Obtaining Digital Signature and Public-Key Cryp-

tosystems”. Communication of the ACM 21 no. 2

1978.

Wootters, W.K; Zurek, W.H (1982). ”A single quantum can-

not be cloned”. Nature, 299, 802

IdQuantique (2004) ”A Quantum Leap for Cryptography”.

http://www.idquantique.com/ﬁles/introduction.pdf

ICETE 2005 - SECURITY AND RELIABILITY IN INFORMATION SYSTEMS AND NETWORKS

274