
LOCALITY AND GLOBALITY: ESTIMATIONS OF
THE ENCRYPTION COLLECTIVITIES
Cristian Lupu
Romanian Academy
Ceter of New Electronic Architectures
Tudor Niculiu
”Politehnica” University of Bucharest
Dept. Eletronics, Telecommunications and IT
Eduard Frant¸i
Microtechnology Institute of Bucharest
Bucharest, Romania
Keywords:
Self-organization, collectivity, structure, architecture, interconnection, locality, globality, symmetry.
Abstract:
In this paper we try to define a collectivity, to model and to measure it. Because N. Bourbaki names ”collec-
tivizing relation” the relation defining a set, we name collectivities only the sets selected or built by the help
of the relations. The orthogonal interconnections model very well the collectivities. The behavior (structural
self-organization) around the origin is different for homogenous and non-homogenous interconnections. How
can we measure this behavior? A way is by locality and globality. The locality measures analytically by neigh-
borhoods, neighborhood reserves, Moore reserves and synthetically by diameters, degrees, average distances.
The globality is the behavior of an interconnection around a property. The globality vs. symmetry measures
by the compactity, efficiency and interconnecting filling. The locality and the globality are among primary
manifestations of the self-organization. In this way, collectivities modeled by self-organizing interconnections
can contribute to changing our fundamental view of computers by trying to bring them nearer to the nature.
1 INTRODUCTION STRUCTURE
AND ARCHITECTURE
A complexity system modelling means firstly the per-
ception of a self-organization of the system and then
the proper modelling. To perceive a complex, said
Wittgenstein, means to perceive the relations of its
constituent parts in a determined way. On the other
hand, one of the characteristics of the nature is the
collectivity. Through the computing terrain, Professor
Moshe Sipper said in the foreword to a recent book,
during the past few years a new wind has been sweep,
slowly changing our fundamental view of computers.
We want them, of course, to be faster, better, more
efficient - and proficient - at their tasks. But, more
interestingly, we are trying to imbue them with abili-
ties hitherto found only in nature, such as evolution,
learning, development, growth, and collectivity (Cas-
tro and Zuben, 2005). We can observe collectivities in
the not living world (universe galaxies, solar systems,
crystalline units) as in the living world (ant hills, bee
swarms, nations).
What properties are behind the relations who tie
the collectivities? Maybe is the gravity, the symmetry
or the survival instinct? In a word, structural self-
organization. The self-organization can be structural
and functional. Our paper refers to the structural self-
organization applied to the collectivities.
First let us define the collectivity. For this we must
answer to another question: what is a set? A set ”can
be selected by a membership or by a relation which
substantiate the membership or by bringing in the set
field elements which fulfill the relation” (Dr
˘
ag
˘
anescu,
1985). Because N. Bourbaki names ”collectivizing
relation” the relation defining a set, we name collec-
tivities only the sets selected or built by the help of
the relations. Therefore, we exclude the sets selected
by the membership, the most general. A collectivity
not means a set made, for example, of a star, a planet,
a crystal, an ant, a bee and a man.
The relation which substantiates the membership
of a collectivity is connected with its functionality:
a collectivity is made of the least functional entities.
For example, an interconnecting is made of nodes and
486
Lupu C., Niculiu T. and Fran¸ti E. (2006).
LOCALITY AND GLOBALITY: ESTIMATIONS OF THE ENCRYPTION COLLECTIVITIES.
In Proceedings of the Third International Conference on Informatics in Control, Automation and Robotics, pages 486-493
DOI: 10.5220/0001205404860493
Copyright
c
SciTePress

links which is equivalent with the graph definition (a
set X of nodes and an application Γ of X in X which
gives the set of connections). The encryption collec-
tivity means a set S of signs and an application (key)
K of S in S which gives encryptions.
In this paper we try to begin to study the collec-
tivities structural and by the help of the architec-
ture concept, a connection concept toward the rela-
tion/function. We start with the definition of the con-
cept of structure (Nemoianu, 1967). The word struc-
ture comes from the Latin where there are the noun
structura, with the meaning of building, and the verb
struere (to build) with the past participle structus. In
English and French the word has the same meaning:
edifice, way to build. The abstraction of the word
makes slowly: only in the XVII-XVIII
th
centuries ap-
pears in the sense of reciprocal relation of the parts
or the constitutive elements of a whole, determining
its nature, its organization. The initial meaning of
building maintains till now but abstracter sense will
be dominated more and more. During the XIX
th
cen-
tury, structure is generally opposite to function, like
static to dynamic.
The end of the XIX
th
century brings a new mean-
ing of the structure concept. It will begin to represent
not a simple configuration, a ”static” organization, but
a whole made by solidary elements, in which every-
one depends on all other ones and can not be what it
is than in and through them. Evidently, it is a step for-
ward. The connection between parts (the first mean-
ing) is something less necessary, less outlined, more
approximately, more vaguely and more generally than
the total interdependence system of each part with all
other parts (the second meaning). If the first meaning
is a sum, the second is a whole. This turning point
coincides with the penetration of the structure con-
cept in the humanities. The term has been changed
by a synonym, Gestalt, understood as form, pattern,
structure, the making of parts which are determined
by whole, system of its behavior can not equal with
the sum of the parts. Gestalt is not related to organi-
zation or to plan, but with an organism, a whole, an
entelechy. The entelechy is a term introduced by the
Austrian psychologist Ehrenfels appointing the fea-
tures (of geometric figures or melodies) by which they
exceed the sum characteristics. A geometric figure re-
mains itself even represented in other coordinate sys-
tem, decreased, enlarged, color modified. This invari-
ance of the transposing calls also isomorphism.
The linguistic researchers contribute resolutely to
the understanding and to the using of the structure
concept unifying both meanings: the coherent, co-
agulated globality and the relations system between
local parts or, in few words, the globality and the lo-
cality. This step in the evolution of the structure term
opens a path to the identification between structure
and essence of an object or a phenomenon. Wittgen-
stein writes in Tractatus that the manner in which the
objects depend some on the others in the state of af-
fairs constitutes the structure of the state of affairs.
Having in view the above, the structure of a col-
lectivity can be self-organized locally and globally.
For example, an interconnecting structure estimates
locally by neighborhoods. Thus, the locality is the be-
havior (structural self-organization) of a collectivity
around an origin. The origin can be temporal or spa-
cial. The locality definition refers to the first mean-
ing of the structure concept (the connection between
parts). The globality is the behavior (structural self-
organization) of a collectivity around a property. For
example, the interconnections can be estimated and
designed by the help of the symmetry properties. The
globality definition concerns to the second meaning
of the structure concept at which referred Wittgen-
stein (total interdependence system of each part with
all other parts).
On the other hand, the collectivity architecture,
a connection concept between the structure and the
function, gives a global meaning to the collectivity
with the aim to better understand the connection be-
tween the structure and the function of this collectiv-
ity. Thus, we can speak of the universe architecture,
a crystallographic system architecture, a house archi-
tecture, a town architecture, a computer architecture,
an interconnecting architecture, a communication ar-
chitecture. The architecture measures by the degree
of membership to global properties. The symmetry is
a global property.
Helping the interconnection as a collectivity model
we try to prove that the dichotomy locality-globality
covers mathematically one of the structural meanings
of the collectivity: the localization and the globaliza-
tion, i.e. a structural potential of a collectivity dy-
namics, a structural self-organization of a collectiv-
ity. The dynamics of an encryption collectivity can
help us to the decryption process.
2 INTERCONNECTION AS A
COLLECTIVITY MODEL
The interconnections made of N nodes and L links
model very well the collectivities. The nodes are the
members of the collectivity which are tied by links.
If there are the encryption collectivities the nodes are
signs and the links are the set of encryption keys (a
key is included in the set L). We shall limit, with-
out losing too much of generality, to the orthogonal
interconnections (Duato et al., 1997). The algebraic
representation of an orthogonal interconnection can
be made in a mixed radix number system, MRNS.
Any number N can be represented in MRNS as a
product of whole numbers, N = m
r
m
r−1
... m
1
.
LOCALITY AND GLOBALITY: ESTIMATIONS OF THE ENCRYPTION COLLECTIVITIES
487

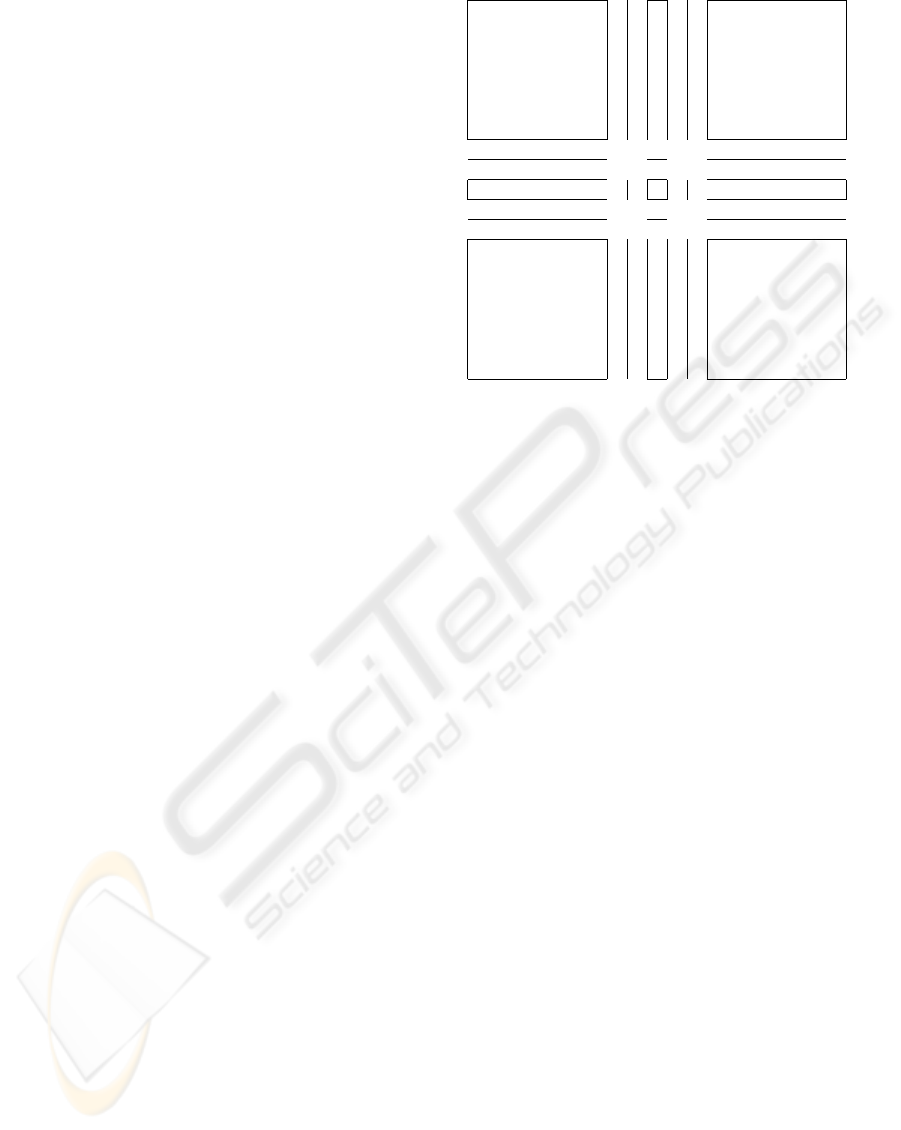
u u u u
u u u u
u u u u
u u u u
u u u u00 03
40 43
Figure 1: A GHT with N = m
2
· m
1
= 5 · 4.
On the basis of this representation, to each node of
an interconnection we can associate an address X,
0 ≤ X ≤ N − 1, made of r digits. Afterwards,
we present some orthogonal interconnections as col-
lectivities, i.e. sets selected or built by relations.
A generalized hypercube, GHC, is a collectivity
with N = m
r
m
r−1
... m
1
nodes interconnected in
r dimensions. In every dimension i, i = 1, 2, ..., r,
the m
i
nodes are interconnected all by all, i.e. ev-
ery node X = (x
r
x
r−1
... x
i+1
x
i
x
i−1
... x
1
)
is connected with the nodes addressed by X
′
=
(x
r
x
r−1
... x
i+1
x
′
i
x
i−1
... x
1
), where 1 ≤ i ≤ r,
0 ≤ x
′
i
≤ m
i−1
and x
′
i
6= x
i
. From GHC de-
rives the hypercube, HC, with N = m
r
, the bi-
nary hypercube, BHC, with N = 2
r
nodes, and the
completely connected structure, CCS, with N = m
nodes. A generalized hypertorus, GHT, have N =
m
r
m
r−1
... m
1
nodes in r dimensions, in every di-
mension i, i = 1, 2, ..., r, the m
i
nodes being inter-
connected in a torus, i.e. every node X is connected
with the nearest neighbor nodes addressed by X
′
=
(x
r
x
r−1
... x
i+1
x
′
i
x
i−1
... x
1
), where 1 ≤ i ≤ r,
x
′
i
= |x
i
± 1|
modulo m
i
. From GHT derives the hy-
pertorus, HT, with N = m
r
, the binary hypercubes
also, and the torus, T, with N = m. A generalized
hypergrid have N = m
r
m
r−1
... m
1
nodes in r di-
mensions, in every dimension i, i = 1, 2, ..., r, the m
i
nodes being interconnected in a chain, i.e. every node
X is connected in a grid with the nodes addressed
by X
′
= (x
r
x
r−1
... x
i+1
x
′
i
x
i−1
... x
1
), where
1 ≤ i ≤ r; x
′
i
= x
i
± 1|x
i
6= 0 and x
i
6= m
i
− 1;
x
′
i
= x
i
+ 1|x
i
= 0; x
′
i
= x
i
− 1|x
i
= m
i
− 1. From
GHG derives the hypergrid, HG, with N = m
r
, the
chain, C, with N = m nodes and BHC again.
These are homogenous (at links) interconnections.
As example of non homogenous interconnections
we gave a variation of non-homogenous orthogo-
nal interconnections, the generalized hyper struc-
tures, GHS (Lupu, 2002). A GHS is an inter-
connection in which every node X is connected in
the dimension i, 1 ≤ i ≤ r, to the nodes ad-
dressed by an interconnecting vector
∪
k
i
j=1
X
ij
=
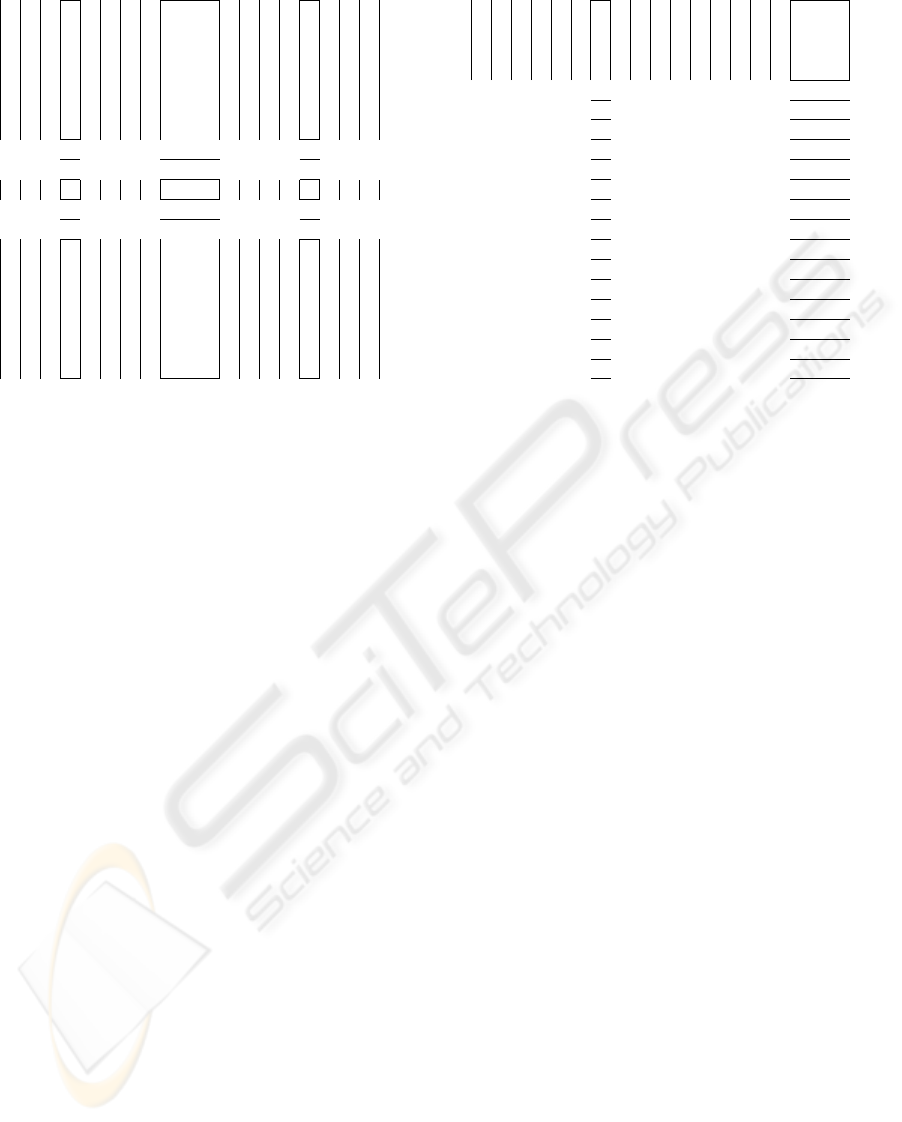
u u u u
u u u u
u u u u
u u u u
u u u u
00 03
40 43
Figure 2: A GHS with N = m
2
· m
1
= 5 · 4. The intercon-
necting vector is
X
21
, X
11
and GHS is coded (CCS, T ).
(x
r
x
r−1
... x
i+1
x
′
i
x
i−1
... x
1
).
∪
k
i
j=1
X
ij
spec-
ifies that a node of GHS is connected by a vector of
unions of elementary interconnection structures, in-
stead of a single elementary interconnection struc-
ture in the homogeneous interconnections. This in-
terconnecting vector has r elements, 1 ≤ i ≤ r. So,
this interconnecting vector is defined, on one hand,
by the number of dimensions, r, and, on the other
hand, by k
i
elementary interconnection structures,
i = 1, 2, ..., r, for which the unions
∪
k
i
j=1
X
ij
are
specified, j = 1, 2, ..., k
i
. X
ij
are homogeneous in-
terconnections, like tori, T, grids, G, and completely
connected structures, CCS, and must not be disjoint
for a dimension.
In the figures 1 and 2 we give two examples of
simple homogenous and non-homogenous intercon-
nections. At homogenous regular interconnections,
as the GHC or HT, the origin position does not mat-
ter. The interconnections are spherical, the diameter
is the same. At irregular networks, as the general-
ized hypergrids and other non-homogenous intercon-
nections, it matters where the position of the origin is.
The ”structural” behavior around the origin is differ-
ent for homogenous and non-homogenous intercon-
nections. How can we measure this behavior? One
way is by locality and globality.
3 LOCALITY: A FIRST SENSE OF
COLLECTIVITY STRUCTURE
The collectivities having as a model the interconnec-
tions made of nodes and links can be estimated by
locality and globality. The locality is the spatial be-
havior of interconnection around an origin. As in
physics, where the gravity characterizes attraction of
the objects, the locality defines the interconnection:
nearer objects communicate better or nearer nodes in-
terconnect easier. As we told above, the locality defi-
nition refers to the first meaning of the structure con-
ICINCO 2006 - ROBOTICS AND AUTOMATION
488

cept, the connection between parts (links of nodes).
The locality measures analytically by neighborhoods,
neighborhood reserves, Moore reserves and syntheti-
cally by diameters, degrees, average distances (Lupu,
2004a). We consider the locality to be classified
firstly as structural (topological), and, secondly, as
functional. Therefore, the locality of an interconnec-
tion will be defined by two localities: a structural lo-
cality and a functional locality.
The structural localities can be appreciated by
neighborhoods. The neighborhoods can be classified
as surface (radial) neighborhoods and volume (spher-
ical) neighborhoods. The surface neighborhood of
an interconnection is the number of nodes at a dis-
tance d, SN
d
(O) = N
d
(O), where O is the ori-
gin chosen arbitrarily. The volume neighborhood is
V N
d
(O) =
P
d
i=1
N
d
(O). By neighborhoods, the
structural locality can be evaluated analytically. An-
other measure, more synthetically, of the structural lo-
cality is the diameter: at the same number of nodes,
the smaller diameter is the bigger locality is.
A problem, as we told above, is that the neighbor-
hoods and the diameters depend on the origin posi-
tions. At homogenous regular interconnections, as
the generalized hypercubes or hypertori, the origin
position does not matter. At irregular interconnec-
tions, as the generalized hypergrids and other non-
homogenous structures, it matters where the position
of the origin is. The topographic model presented in
(Lupu, 2004b) helped us to study the description and
the behavior of the direct interconnections, homoge-
nous and, especially, non-homogenous. The proper-
ties of interconnecting locality can be better ”read” by
the diameter contour patterns in the structural relief
of the interconnection.
We introduced a measure that helps us to reveal
the interconnection relief, the state of agglomeration.
The structural localities are more or less agglomer-
ated, as in reality. The depth of the valley (minimum
diameter) informs us about maximum agglomerated
locality, and the height of the peak (maximum diame-
ter) about the minimum agglomerated locality. Thus,
structural state of agglomeration of an interconnec-
tion node is given by the interconnection diameter
computed with the origin in the corresponding node.
The contour patterns of structural states of agglom-
eration (of the diameters computed with the origin in
every node) constitute a map with the structural relief
of the interconnection.
The structural locality is an invariable information
depending on the topology. A functional point of view
on the interconnection locality can take into consider-
ation the message routing distributions, Φ
O
(d), where
O is the origin and d is the distance.
As the structural locality, the functional locality
measures also by neighborhoods: a functional sur-
face neighborhood, F SN
d
(O) = Φ
O
(d) × N
d
(O),
and a functional volume neighborhood, F V N
d
(O) =
P
d
i=1
Φ
O
(i) × N
i
(O). For the functional locality,
there is also a synthetic measure like diameter, the
functional average distance. The functional average
distance helps the next definition: the functional state
of agglomeration of an interconnection node is given
by the functional average distance of the intercon-
nection computed with the origin in the correspond-
ing node. Shorter the functional average distance is,
greater the state of functional agglomeration is! Us-
ing the contour patterns of the functional states of ag-
glomeration we can draw a map depicting the func-
tional relief of the interconnection (see next section).
The surface and volume neighborhoods, on the
one hand, and the diameter or degree, on the other
hand, are analytical and synthetic evaluation means
of the intercommunication capability of interconnec-
tions, measuring the structural locality. By functional
neighborhoods and, indirectly, by functional average
distance, it expresses which part of the structural lo-
cality is used by communication process implemented
on the network. In other words, the functional neigh-
borhoods and the functional average distances express
the functional locality of the interconnections.
Obviously, for a given interconnection, SN
d
≥
F SN
d
and V N
d
≥ F V N
d
. The difference between
the two types of neighborhoods represents what we
named the neighborhood reserve. The neighborhood
reserve is of surface, SNR
d
= SN
d
− F SN
d
, or of
volume, V NR
d
= V N
d
− F V N
d
. Using the neigh-
borhood reserve, we introduced a design/evaluation
criterion of a topology by enunciating the following
conjecture: the intercommunication structural poten-
tial of an interconnection is optimally used in a com-
munication process characterized by a routing distri-
bution Φ if the neighborhood reserve is minimal.
To evaluate the structural locality of an intercon-
nection, besides the neighborhoods and neighborhood
reserves, we proposed a simple measure: the Moore
reserve based on the Moore bound. As it is known,
the Moore bound is given as the maximum number
of nodes which can be present in a graph of given
degree l and diameter D: N
Moore
= 1 + l(((l −
1)
D
−1)/(l−2)). This bound is deduced from a com-
plete l-tree with diameter D and is an absolute limit
for a diametrical volume neighborhood, V N
d
(O) =
P
d
i=1
N
d
(O), in any graph (interconnection) of l de-
gree and D diameter. Except for the complete l-ary
trees, this bound is rarely reached. Petersen graph,
completely connected structures and rings with odd
number of nodes are interconnections that reach the
Moore bound. Therefore, it makes sense to com-
pute for an interconnection how far is this bound: the
farther away the Moore bound, the structural local-
ity properties are worse. This is implemented by the
Moore reserves.
LOCALITY AND GLOBALITY: ESTIMATIONS OF THE ENCRYPTION COLLECTIVITIES
489
The surface Moore reserve is defined by the differ-
ence between the number of nodes in a correspond-
ing Moore tree at the distance d, with the degree in
considered interconnection, and the surface neigh-
borhood in considered interconnection: SM R
d
=
l(l − 1)
d−1
− N
d
. The Moore reserve is defined
by the difference between the Moore bound at the
distance d and the volume neighborhood: MR
d
=
N
Moore
(d) − V N
d
.
4 HOMOGENEITY AND
SYMMETRY
Based on the topographic model we estimate three
bidimensional interconnections more and more non-
homogenous and asymmetrical. Let us draw, in the
first example, the functional relief for uniform dis-
tribution of bidimensional interconnection having 20
nodes on a dimension.
The unidimensional elementary interconnection
structure, non-homogenous, EIS1, is the same in both
dimensions being composed of a completely con-
nected structure (nodes 0 ÷ 8), a grid (nodes 8 ÷
11) and, again, of a completely connected structure
(nodes 11÷19). EIS1 has, in this way, 20 nodes ”sym-
metrically arranged”.
In the figure 3 we give the contour patterns for the
uniform distribution. First, we notice the perfect sym-
metry in both dimensions thanks to the symmetry of
the EIS, the same in both dimensions. According to
this symmetry, we observe that the biggest part of the
functional relief is formed of four tablelands having
the same height, 5.5 nodes, orientated to the four car-
dinal points. In the middle of the interconnection, like
a cross 4 nodes wide, four canyons deepen, with the
average distance of 4.5 nodes. Right in the intercon-
nection center there is a valley, the most agglomer-
ated part of the structure, with a depth of 3.5 nodes.
The biggest slope of the average distance
¯
d
U
(O), to
the interconnection middle, is 2 nodes, and the slopes
crossing the canyons are 1 node.
The functional reliefs for the other distributions
(structural and exponential) look likewise. The
heights or the slopes are the difference.
Let us draw, in the second example, the functional
relief of a bidimensional non-homogenous structure
which has in the first dimension an elementary in-
terconnection structure EIS2 being composed of a
completely connected structure (nodes 0 ÷ 8), of a
grid (nodes 8 ÷ 11) and, again, of a completely con-
nected structure (nodes 11 ÷ 19) and in the second
dimension, the elementary structure EIS3 being com-
posed of a torus (nodes 0 ÷ 8), of a completely con-
nected structure (nodes 8 ÷ 11) and, again, of a torus
(nodes 11 ÷ 19). In the figure 4 we give the con-
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
5.5
5.5
5.0
4.5
4.5
5.0
5.5
5.5
5.5
5.5
5.0
4.5
4.5
5.0
5.5
5.5
5.5
5.5
5.0
4.5
4.5
5.0
5.5
5.5
5.5
5.5
5.0
4.5
4.5
5.0
5.5
5.5
5.0 4.5 4.5 5.0
5.0 4.5 4.5 5.0
4.0 3.5
@
@@
@
@
@
@
@
Figure 3: The functional relief for the bidimensional inter-
connection with the non-homogenous EIS1 for the uniform
distribution. The contour patterns of the functional average
distance
¯
d
U
(O) are drawn.
tour patterns of this interconnection for uniform dis-
tribution. The bidimensional interconnection is sym-
metrical too, though it has in the making of the el-
ementary interconnection structures, EIS2 and EIS3,
different homogenous sub-interconnections. The re-
lief of this interconnection is more varied: four peaks,
rather small tablelands, 7.5 nodes height, and a larger
valley, of four nodes, separating the network in two
along x
2
dimension and in the middle of x
1
dimen-
sion, 5.5 nodes depth. Still there are two saddles 6.5
nodes height between the peaks and, in the middle of
the network, as in the previous example, the deepest
valley (the most agglomerated part), 4.5 nodes depth.
The symmetry is not the same on the two intercon-
nection axes, like in the first example. The symmetry,
in present example, differs from an axis to the other
and, therefore, is weaker.
In the last example is given a non-homogenous in-
terconnection with a marked characteristic of asym-
metry. Let us draw the functional relief of a non-
homogenous bidimensional interconnection with 20
nodes per dimension. On the first dimension there
is an elementary interconnecting structure EIS4 be-
ing composed of a completely connected structure
(nodes 0 ÷ 5), a grid (nodes 5 ÷ 12) and a torus
(nodes 12÷19). On the second dimension the elemen-
tary interconnecting structure EIS5 is composed of a
torus (nodes 0÷10), a completely connected structure
(nodes 10÷15) and, again, a torus (nodes 15÷19). In
the figure 5 we give the contour patterns of this asym-
metrical on both axes interconnection. The structure
presents only partial symmetries on certain areas.
We presented three bidimensional interconnections
with the same number of nodes per dimension and
ICINCO 2006 - ROBOTICS AND AUTOMATION
490
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
6 6.5 7 7.5 7.5 7 6.5 6 5.5 5.5 5.5 5.5 6 6.5 7 7.5 7.5 7 6.5 6
6 6.5 7 7.5 7.5 7 6.5 6 5.5 5.5 5.5 5.5 6 6.5 7 7.5 7.5 7 6.5 6
5.5 6 6.5 7 7 6.5 6 5.5 5 5 5 5 5.5 6 6.5 7 7 6.5 6 5.5
5 5.5 6 6.5 6.5 6 5.5 5 4.5 4.5 4.5 4.5 5 5.5 6 6.5 6.5 6 5.5 5
5 5.5 6 6.5 6.5 6 5.5 5 4.5 4.5 4.5 4.5 5 5.5 6 6.5 6.5 6 5.5 5
5.5 6 6.5 7 7 6.5 6 5.5 5 5 5 5 5.5 6 6.5 7 7 6.5 6 5.5
6 6.5 7 7.5 7.5 7 6.5 6 5.5 5.5 5.5 5.5 6 6.5 7 7.5 7.5 7 6.5 6
6 6.5 7 7.5 7.5 7 6.5 6 5.5 5.5 5.5 5.5 6 6.5 7 7.5 7.5 7 6.5 6
@@
@
@
@
@
@@
@@
@
@
@@
@
@
@
@
@
@
@
@
@
@
@
@
@
@
Figure 4: The functional relief for the bidimensional non-
homogenous interconnection with the elementary structures
EIS2 and EIS3. The contour patterns of the functional aver-
age distance
¯
d
U
(O) for the uniform distribution are drawn.
with elementary interconnection structures more and
more non-homogenous. The functional reliefs proved
these three interconnections have a more and more
marked asymmetry, the structures having a more and
more emphasized ”structural dynamism”, structural
self-organization. This structural dynamism leads to a
more and more powerful structural self-organization
property. Therefore, the non-homogeneity leads, on
the one hand, to the asymmetry, and, on the other
hand, to the more intense structural self-organization.
5 GLOBALITY: A WAY FROM
THE STRUCTURE TO THE
ARCHITECTURE
One of the most important properties of any physical
space structure is the symmetry. The transformation
that keeps the structure of the space is named auto-
morphism. Giving a space configuration, a structure,
a form, an interconnection, we can emphasize a set
of space automorphisms, which leave unchangeable
this interconnection. Thus, the emphasizing automor-
phisms form a group which describes precisely the
symmetry of the giving configuration.
The amorphous space has a total symmetry corre-
sponding to the group of all automorphisms. The
symmetry of an interconnection will be described, as
we have told, by a subgroup of all automorphisms.
The total symmetry of the space defined by n points
(nodes, permutations) will be described by S
n!
, while
a partial symmetry is expressed by a subgroup (of per-
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
q q q q q q q q q q q q q q q q q q q q
13.012.512.011.511.010.510.010.010.511.011.512.012.513.012.512.012.512.512.512.5
13.012.512.011.511.010.510.010.010.511.011.512.012.513.012.512.012.512.512.512.5
12.512.011.511.010.510.09.5 9.5 10.010.511.011.512.012.512.011.512.012.012.012.0
12.011.511.010.510.09.5 9.0 9.0 9.5 10.010.511.011.512.011.511.011.511.511.511.5
11.511.010.510.09.5 9.0 8.5 8.5 9.0 9.5 10.010.511.011.511.010.511.011.011.011.0
11.010.510.09.5 9.0 8.5 8.0 8.0 8.5 9.0 9.5 10.010.511.010.510.010.510.510.510.5
10.510.09.5 9.0 8.5 8.0 7.5 7.5 8.0 8.5 9.0 9.5 10.010.510.09.5 10.010.010.010.0
10.09.5 9.0 8.5 8.0 7.5 7.0 7.0 7.5 8.0 8.5 9.0 9.5 10.09.5 9.0 9.5 9.5 9.5 9.5
10.510.09.5 9.0 8.5 8.0 7.5 7.5 8.0 8.5 9.0 9.5 10.010.510.09.5 10.010.010.010.0
11.010.510.09.5 9.0 8.5 8.0 8.0 8.5 9.0 9.5 10.010.511.010.510.010.510.510.510.5
11.511.010.510.09.5 9.0 8.5 8.5 9.0 9.5 10.010.511.011.511.010.511.011.011.011.0
12.011.511.010.510.09.5 9.0 9.0 9.5 10.010.511.011.512.011.511.011.511.511.511.5
12.512.011.511.010.510.09.5 9.5 10.010.511.011.512.012.512.011.512.012.012.012.0
13.012.512.011.511.010.510.010.010.511.011.512.012.513.012.512.012.512.512.512.5
12.512.011.511.010.510.09.5 9.5 10.010.511.011.512.012.512.011.512.012.012.012.0
12.011.511.010.510.09.5 9.0 9.0 9.5 10.010.511.011.512.011.511.011.511.511.511.5
11.511.010.510.09.5 9.0 8.5 8.5 9.0 9.5 10.010.511.011.511.010.511.011.011.011.0
@@@@@@@
@@@@@@@
@@@@@@
@
@@@@@@
@
@@@@@@
@
@@@@@@
@
@@@@@@ @@
@@@@@@ @@
@@@@@@ @@
@@@@@@ @@
@@@@@@ @@
@@@@@@ @@
@@@@@@@
@@@@@@@
@@@@@@@
Figure 5: The functional relief of the bidimensional inter-
connection with elementary structures EIS4 and EIS5 for
uniform distribution. The contour patterns of the functional
average distance
¯
d
U
(O) are drawn.
mutations) included in S
n!
. Therefore, symmetrical
groups S
n!
model the symmetry of a space defined by
n nodes and inversely. The total symmetry of a space
is represented by a total interconnection, a completely
connected structure with n! nodes.
As an example, the plane figures have as constitu-
tive symmetries only the identity, rotation, translation,
reflection and reflection-translation. It is known that a
rectangle has the following four symmetries: the iden-
tity, I; the two reflections S
1
and S
2
vs. non-parallel
sides perpendicular bisectors, A
S
1
and A
S
2
; the ro-
tation with 180
◦
, R. The four automorphisms can
be represented by an interconnection, the vertexes of
which are noted 1, 2, 3 and 4. With this, we equate the
symmetries of the rectangle with following permuta-
tions (generators): I = (1 2 3 4), S
1
= (2 1 4 3),
S
2
= (4 3 2 1) and R = (3 4 1 2). The four sym-
metries form a commutative group to the composition
operation but, equating them with permutations, we
notice that these symmetries form only a subgroup of
the symmetric group of order 4, S
4!
. In this way, we
can examine the symmetry properties of plane figures,
which divide the symmetric groups S
n!
in different
subgroups. Let us note by G
S
the groups (subgroups)
of symmetries which divide the symmetric group S
n!
.
We defined at the beginning of the paper
that the globality is the behavior (structural self-
organization) of a collectivity around a property.
How does it define the globality of the plane figures
vs. symmetry property? A quantitative appreciation,
a measure of the globality vs. symmetry, which we
note Γ
n
, is given by the ratio of the order of group
of symmetries and the order of symmetric group:
Γ
n
= |G
S
|/|S
n!
|. The inverse of Γ
n
we denominated
LOCALITY AND GLOBALITY: ESTIMATIONS OF THE ENCRYPTION COLLECTIVITIES
491

group locality, L
n
, (Lupu and Niculiu, 2005).
The globalities must be compared at the same num-
ber of interconnecting nodes (same S
n!
). For ex-
ample, the globalities vs. symmetry of the tetragon
and rectangle are the same for they refer to the same
symmetric group, S
4!
, while we can not say any-
thing about globalities of the isosceles triangle and
the square for they refer to the different symmetric
groups, S
3!
and S
4!
. The maximum globality will be
obtained when G
S
= S
n!
= 1. Let us give three plane
figures, an isosceles triangle, a trigon and an equi-
lateral triangle, all having 3 interconnecting nodes,
so referring to S
3!
. The isosceles triangle has two
symmetries, I and S, its globality being the least,
G
S
/S
3!
= 1/3. The trigon has three symmetries, I,
R
1
and R
2
. Its globality is equal to 1/2. The equilat-
eral triangle has 6 symmetries, I, R
1
, R
2
, S
1
, S
2
and
S
3
. Its globality is the biggest, 1.
Instead of relying on the logic distances between
the nodes (locality), we want to evaluate/design a in-
terconnection (collectivity) based on properties. The
globality put the properties, a constructive, synthetic
principle, an architectural principle, before the dis-
tances, an analytic principle, especially tied to the
locality. The logic distances ”disappear” into a
globality, which displays the properties. The local-
ity principle helped us to design/evaluate new non-
homogenous interconnection networks, as general-
ized hyper structures, and the globality principle
helped us to imagine a new interconnection paradigm
based on symmetrical morphemes and ensembles and
that we will shortly introduce in next paragraphs.
The morphological interconnection, that we pro-
pose as a new model for a collectivity, have to en-
semble in S
n!
elementary entities. We shall name
these entities, morphemes, and the tying interconnec-
tion, morphological interconnection. If we use the
architectural principle of globality vs. symmetry we
shall name symmetrical morphemes, symmetrical en-
sembles and symmetrical interconnection.
The symmetrical morphemes, helping us to build
symmetrical ensembles, are bidimensional or tridi-
mensional forms emphasizing in a symmetric group
S
n!
by the Cayley graphs (Akers and Krishnamurthy,
1989) of (sub)groups of symmetry, G
S
. These groups
of symmetry represent the symmetries of plane or
tridimensional figures. For example, the symmetries
of the right line segment are the identity I = (1 2)
and the reflection S = (2 1). G
S
has a Cayley graph
with a transposition. The symmetries of the isosceles
triangle are the same, the identity I = (1 2 3) and the
reflection S = (1 3 2). The Cayley graph associated
to the symmetries of the isosceles triangle is also with
2 nodes and a transposition, the only difference being
the defining automorphisms symmetric groups, S
2!
for segment and S
3!
for isosceles triangle. The sym-
metries of the trigon are identity I = (1 2 3) and two
q q qq q q
0
q
qq
q
q
q
@
@
0.166
q qq
q
q
q
T
T
0.333
q qq q
q q
0.555
q q
q q
q q
1
K
EL
q q q q
q q
A
A
A
A
0
q q
qq
q q
H
H
H
H
0.027
qq
qq
q q
H
H
H
H 0.111
p
p
p
p
p
p
A
A
A
A
1
K
EP
Figure 6: Compactity of the ensembles K
E
realized by sim-
ple symmetric morphemes in architectural space S
3!
.
rotations R
1
= (2 3 1) and R
2
= (3 1 2). The com-
plete (Lupu, 2004a) Cayley graph of the trigon sym-
metries subgroup is a directed graph. It is an overlap
of two hamiltonian circuits (cycles as permutations)
in the opposite direction, representing minimal Cay-
ley graphs of the trigon symmetries. The symmetries
of the equilateral triangle are the identity I = (1 2 3),
the rotation with 180
◦
R
1
= (2 3 1), the rotation with
240
◦
R
2
= (3 1 2) and the reflections S
1
= (1 3 2),
S
2
= (3 2 1) and S
3
= (2 1 3). The symmetric
morpheme of the equilateral triangle has the globality
Γ = G
S
/S
3!
= 1. The morpheme of the right line
segment is a linear morpheme, of the triangle and the
square are plane morphemes and the morphemes of
the pyramid and the prism are spatial morphemes.
A first symmetric ensemble characteristic appreci-
ates its compactity. The maximal compactity of an
ensemble will be obtained when all morphemes will
have all nodes, links, surfaces and volumes intercon-
nected. There are four basic rules of morphemes in-
terconnecting: common nodes (CN), common links
(CL), common surfaces (CS) and common volumes
(CV). In this way, the compactity is a measure of mor-
phemes interconnecting in an ensemble. The com-
pactity is minimal for CN interconnecting and maxi-
mal for CV interconnecting. Let us note the ensem-
bles compactity with K
E
and it will express different
for the three types of morphemes: K
EL
= Γ
2
m·n
N
M
,
K
EP
= Γ
3
s·m·n
L
M
·N
M
and K
ES
= Γ
4
v·s·m·n
NS
M
·L
M
·N
M
,
where Γ is the globality; n is the number of nodes
interconnected, n = 0...
N
M
Γ
; m is the number of link
interconnected, m = 1...
L
M
Γ
(m = 1 for no link in-
terconnected); s is the number of surfaces intercon-
nected, s = 1...
NS
M
Γ
(s = 1 for no surface intercon-
nected); v is the number of volumes interconnected,
v = 1...
1
Γ
(v = 1 for no volume interconnected);
N
M
is the nodes number of the morpheme; L
M
is
the edges number of the morpheme; NS
M
is the sur-
faces number of the morpheme. In the figure 6 we
give some examples of symmetric ensembles struc-
tured in the architectural space S
3!
with linear and
plane morphemes. It also mentions the compactity
ICINCO 2006 - ROBOTICS AND AUTOMATION
492
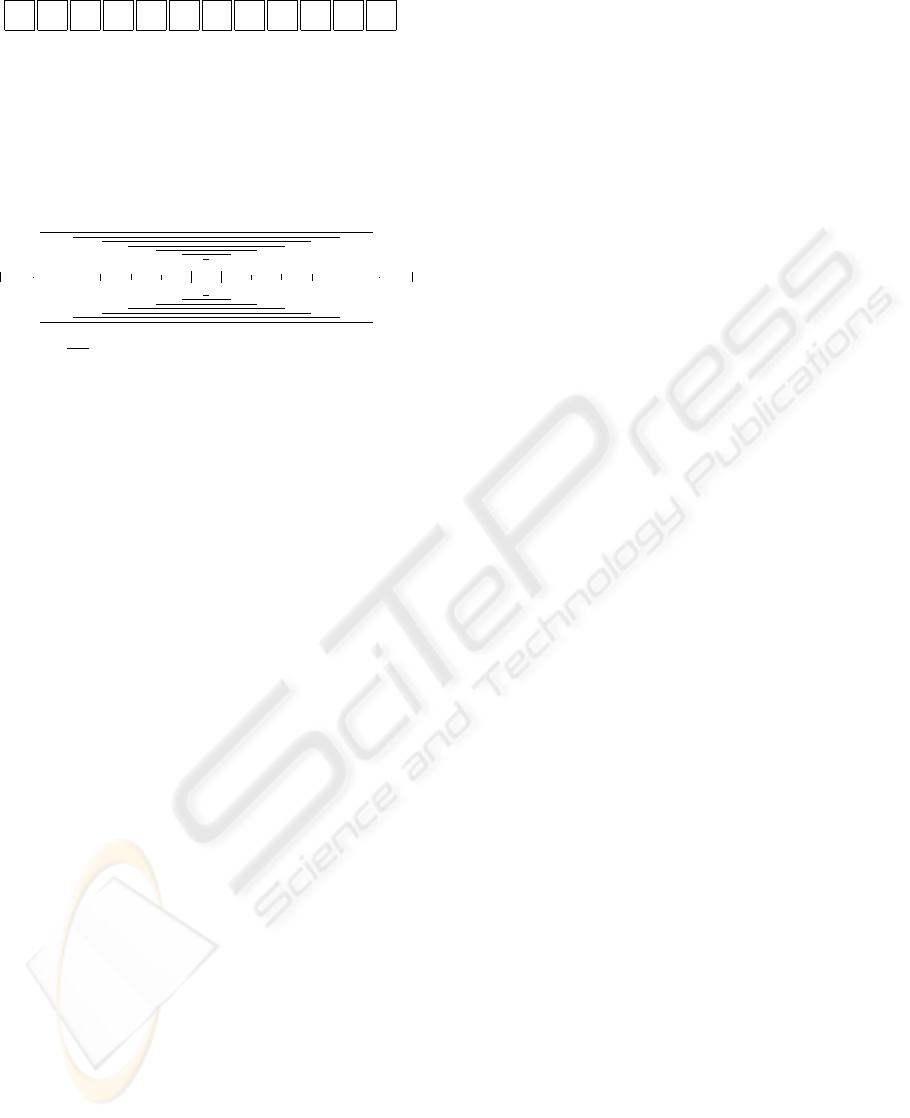
q q q q q q q q q q q q
q q q q q q q q q q q q
q q q q q q q q q q q q
q q q q q q q q q q q q
00 01 02 03 04 05 06 07 08 09 010 011 012
10 11 12 13 14 15 16 17 18 19 110 111 112
Figure 7: A GHG build by the rule CL in architectural space
S
4!
from the 12 symmetrical morphemes of the tetragon.
K
EL
for linear ensembles and K
EP
for plane ensem-
bles. About the other ensembles characteristics, the
interconnecting efficiency in pure ensembles and the
capacity of filling, we shall write in another paper.
q q q q q q q q q q q q
q q q q q q q q q q q q
q q q q q q q q q q q q
q q q q q q q q q q q q
#
"
!
'
&
$
%
'
&
$
%
7 6.5 6 5.5 5 4.5 4 4.5 5 5.5 6 6.5 7
Figure 8:
d
U
functional relief of the ensemble of the fig. 7.
After a short evaluation of the symmetrical ensem-
bles by outside measurements involving the globality
and the geometry of the symmetric morphemes, let us
appreciate by inside measurements which will offer
a view on the on the communicability of them. The
symmetrical ensembles are build in S
n!
of symmetri-
cal morphemes which have a property or more, tied
by some general rules. For example, in the figure 7
we give a generalized hypergrid assembled in S
4!
of
12 symmetrical morphemes of the tetragon. A gen-
eralized hypergrid, GHG, is assembled in two dimen-
sions by rule CL and for the algebraic representation
we used MRNS. In the figure 8, using the topographic
model mentioned above we obtained a functional re-
lief with an uniform routing distribution.
6 CONCLUSION
In this paper we tried to approach in other way
the problem of encryption. Instead of occupying,
for example, with the algorithms (functional self-
organization) (Lupu et al., 2005), we questioned what
hides behind the algorithms. A possible answer is
the (encryption) collectivities modeled as intercon-
nections (structuralized self-organization). Our prin-
cipal aim was to define the collectivities, then to
model and to measure them. The collectivity is a priv-
ilege of structuralized nature (living and not living).
A collectivity is at least an interconnection. Locality
and globality are among the most general structural
measures, the primitives of an interconnection which
models a collectivity. The locality supposes an origin
and the globality, a property. The locality is the struc-
tural self-organization around an origin and the glob-
ality, around a property. The architecture, a connec-
tion concept between the structure and the function of
the collectivity, measures by the degree of member-
ship to global properties, like symmetry. Helping with
these concepts, self-organization, structure, architec-
ture, function, interconnection, locality and globality,
we tried to model and to measure a collectivity. Dis-
covering the rules that govern the future interconnec-
tion environment is a major challenge (Zhuge, 2005)
and, maybe, one of the future interconnection envi-
ronments is the collectivity model.
ACKNOWLEDGEMENTS
The work of this paper was done with financial sup-
port from SCRIPT Project 8/2005 from Romanian
Security Program. The authors would like to thank
one of the anonymous reviewers for its valuable com-
ments.
REFERENCES
Akers, S. B. and Krishnamurthy, B. (1989). A group - the-
oretic model for symmetric interconnection networks.
IEEE Trans. on Computers, 38(4):555–566.
Castro, L. D. and Zuben, F. V. (2005). Recent Developments
in Biologically Inspired Computing. Idea Group Pub-
lishing, Brazil.
Dr
˘
ag
˘
anescu, M. (1985). Ortophysics (in Romanian). Edi-
tura S¸tiint¸ific
˘
a s¸i Enciclopedic
˘
a, Bucures¸ti.
Duato, J., Yalamanchili, S., and Ni, L. (1997). Intercon-
nection Networks. An Engineering Approach. IEEE
Computer Society Press, Los Alamitos, California.
Lupu, C. (2002). Generalized hyper structures and inter-
connection locality. Romanian Journal of Information
Science and Technology, 5(4):365–382.
Lupu, C. (2004a). Interconnecting. Locality and Symmetry
in Computers Orthogonal Networks (in Romanian).
Editura Tehnic
˘
a, Bucures¸ti.
Lupu, C. (2004b). Locality measured by contour patterns, a
topographic model. In Hamza, M. H., editor, Proceed-
ings of the 15th IASTED International Conference on
Modelling and Simulation, pages 50–54, Marina Del
Rey, California, USA. ACTA Press.
Lupu, C., Firtat, B., and Enoiu, C. (2005). Cryptography
methods using the rsa algorithm. WSEAS Transactions
on Communications, 4(4):153–156.
Lupu, C. and Niculiu, T. (2005). Interconnection locality
and group locality. In Proc. of the EUROCON 2005,
pages 656–659, Belgrade, Serbia and Montenegro.
Nemoianu, V. (1967). The Structuralism (in Romanian).
Editura pentru Literatur
˘
a Universal
˘
a, Bucures¸ti.
Zhuge, H. (2005). The future interconnection environment.
IEEE Computer, 38(4):27–33.
LOCALITY AND GLOBALITY: ESTIMATIONS OF THE ENCRYPTION COLLECTIVITIES
493
