A NEW HYBRID SAMPLING STRATEGY FOR PRM PLANNERS

To Address Narrow Passages Problem

Sofiane Ahmed Ali, Eric Vasselin, Alain Faure

GREAH, Le Havre University, 25 Philippe Lebon street BP 540-F76058, Le Havre, France

Keywords: Probabilistic path planner, probabilistic roadmap, Robot Path Planning, Randomized Algorithms, Random

sampling.

Abstract: The probabilistic path planner (PPP) is a general planning scheme that yields fast robot path planners for a

wide variety of problems, involving high degree of freedom articulated robots, non holonomic robots, and

multiple robots. This paper presents a new probabilistic approach for finding paths through narrow

passages. Our probabilistic planner follows the general framework of probabilistic roadmap (PRM), but to

increase sample density in difficult areas like narrow passages, we define two sampling constraints in order

to get much more points than a classic PRM gets in such areas. We simulate our planner in 2D

environments and the simulations results shows good performance for our planner.

1 INTRODUCTION

Robot path planning, which asks for the computation

of collision free paths in environments containing

obstacles, has received a great deal of attention in

the last decades The basic problem is about

computing a collision free-path that brings the robot

from his current position to some desired goal

position. The space where the robot and the

obstacles are physically present is called the

workspace

, using the formalism introduces by

(Lozano, 1983) the planning is mostly performed in

another space, the configuration space

C . Each

placements of the robot in

W is mapped to a point

C . The portion of C corresponding to collision-

free placements of the robot is referred to as the

free-configuration space

free

CS . At the GREAH

laboratory, a dynamic model of car like robot was

done (Guerin 2005) and our work is to design an

efficient path planner for this mobile robot.

During the past decades probabilistic roadmap

(PRM) (Kavraki, 1994) (Horsch, 1994) (Svestka,

Overmars, 1995) (Svestka, Vleugels, 1995) has

emerged as a powerful framework for path planning

of robots with many degrees of freedom. The main

idea of (PRM) is to sample at random a robot’s

configuration space and connect the sampled points

to construct a roadmaps graph, which captures the

connectivity of the free space. Despite the success of

(PRM) planners, path planning with many degrees

of freedom is difficult and even uncertain.

The first difficulty is laid in many cases to the higher

computational cost paid by (PRM) to construct the

roadmap graph, indeed (PRM) spends a lot of time

in checking collision-free connections between all

the sample points.

The second difficulty remains a classic problem of

(PRM) planners. Sampling points in difficult areas

like narrow passages pose significant problem,

because narrow passages have small volumes and

the probability of sampling from small sets is low.

In this paper, we propose a new probabilistic

approach that reduces the computational cost of

classic (PRM) planners and increases sample density

of points in narrow passages.

The key idea to reduce the computational cost of

(PRM) planner is to construct a single road instead

of roadmaps, our planner connects the starting

configuration to the goal configuration by generating

random configuration from

free

CS , and constructs

one single local path that connect this current

configuration to the immediate configuration

generated before by our planner. Thus, collision-free

connection is made only between two

configurations, instead of the all existent

configurations. We exploit the notion of “visibility

set” (Barraquand, 1997) (Hsu, 1999) and also used

561

Ali S., Vasselin E. and Faure A. (2006).

A NEW HYBRID SAMPLING STRATEGY FOR PRM PLANNERS - To Address Narrow Passages Problem.

In Proceedings of the Third International Conference on Informatics in Control, Automation and Robotics, pages 561-564

DOI: 10.5220/0001220405610564

 SciTePress

in (Nissoux, 1999), to define a termination condition

for our planner. Two points of the configurations

space are considered visible to each other if they can

be connected by a collision-free straight line path.

To improve the sample density in narrow passages

we define a local planner that given two

configurations, sample the path connecting them,

and extract a new collision-free straight line path.

We add to these local planner two constraints. The

predefined distance that our local planner uses to

sample near the current configuration, and the

angular constraint that guarantee always for our

mobile robot moving towards the goal configuration.

Section (2) reviews related work on sampling

configurations in narrow passages, section (3) gives

an overview of our planner, section (4) analyses our

algorithm section (5) presents some resulting paths

from simulations and comments them, section (6)

make a general conclusions about our planner and

presents the future extension of this work.

2 RELATED WORK

The difficulty posed by narrow passages and its

importance, were noted in early worm in PRM

planners. One possibility is to sample more densely

near the obstacles boundaries (Boor, 1999) (Collins,

2003). The Gaussian sampler uses this idea (Boor,

1999). However, in some cases many points near

obstacles boundaries lie far from narrow passages

and do not improves the connectivity of roadmaps.

Other approaches to narrow passages sampling

includes dilating free spaces (Hsu, 1998), and

retracting to the medial axis of free spaces

(Wilmarth, 1999). Both approaches require a high

geometric computation time in high dimensional

configuration spaces

3 OVERWIEW OF THE

PLANNER

In this paper we follow the general framework of

(PRM), but our planer computes one single road

which connects the starting and the goal

configuration with always sampling random

configuration from

free

CS . The local planner then,

connects only the current randomly selected

configuration with the previous configuration added

before. We name our planner the probabilistic single

road planner (PSRP). The idea is to try to get a

simple algorithm that reduces the computational cost

of (PRM). Intuitively by checking collision free

connection only between two milestones our (PSRP)

will save a lot of computation time rather than

spending at checking collision free connection

between all milestones generated by (PRM). The

design of our algorithm is based on tree components

defined below.

3.1 Visibility Path

Consider a robot

ℜ

moving in a workspace

ℑ

let

CS be the configuration space of the robot. Let

free

CS be the collision free configuration space

ℑ

. Let L be any local planner that computes a

path

),(

qqL

(e.g. straight line segment) between

two given configurations

q and

q . We define the

visibility domain of a configuration

q for L by:

}

{

)1(,),(,)(

FqqLFqqVisi

∈∈=

We use this relation to define a new domain of

visibility. Given

goal

, two configurations

and

q the path computed by the local planner, is

sampled at

n points

x and the new visibility

domain is defined as following:

{

}

)2(,),(,)( FqxLFqxVisi

goaligoaliL

∈

Where

free

CSF

3.2 Distance Sampling

Our local planner uses a threshold distance to

connect only milestones within this predefined

distance.

3.3 Angular Sampling

The second constraints is about an angular sampling

to guide the search of a solution path toward the goal

configuration see (Figure 1) In figure (1) we

consider that our algorithm samples milestone1 and

is able to sample two possible milestones (milestone

2 and )2

. For each possible milestone our planer

put a predefined angular constraint

intconstra

on the

difference of the size of the angle between milestone

1 and one of the possible current milestones

2 ,

ICINCO 2006 - ROBOTICS AND AUTOMATION

562

and, the angle between milestone 1 and the goal

milestones.

Goal configuration

int

constra

int

constra

Figure 1: Angular sampling.

The algorithm of angular sampling is given as

follows:

Algorithm1: Randomized angular sampling

1. Repeat

2. Pick M1, M2 from

F /*M1, M2: milestones

←

Angle (

goal

←

Angle (

MM ,

)

4 if (

int21 constra

≤− ) then add M1, M2 to G

5. Else goes to 1.

4 ANLYSES OF (PSRP)

Our algorithm is designed to reduce computational

cost resulting from (PRM) and this, by using the two

sampling constraints we defined above, the notion of

visibility (3.1) and. local geometry tests. We define

the function

f as follows:

1=f If

freegoali

CSqxL ∈),( (3)

The key idea of this strategy is about the local path

computed between two configurations

and

q (i.e.

two milestones) randomly picked. Clearly our

algorithm samples this path at points

x , and a

collision free test is made on each point, a path is

computed between

q and the last

x returning a

positive response for the collision free test.

We also use the idea of hybrid sampling, by

combining our local planner, with the two

constraints presented in (3.2) and (3.3) to increase

sampling in narrow passages. A simplify version of

our algorithm is given:

start

0←f

3. M1

start

q←

4 while (

0=f )

5. Select a random free configuration M2

6. Compute

d,,

/* d : the distance between

M1and M2

7. If (

))()^(

maxint21

constra

≤≤−

θθθ

then

8 sample line segment M1M2 at

x points

9. Check collision at each

x for M1M2 and

goal

10 if (

then

11. Create edge (

)

1 i

xM ∪ edge ( )

goali

12 else create edge

()

1 i

13 add

x to

←

Assume that (PSRP) sample a milestone near

obstacle, indeed our local planner compute local

paths between one milestone and the last

x point

returning a negative response for the collision free

test, thus we have a big probability to get one

milestone near an obstacle boundary. By choosing a

small predefined distance value, for example the

width of the narrow passages, and the angular

constraint to be less or equal to

2/pi , we increase

the sampling points at the narrow passages figure(2).

init

goal

Figure 2: Computed path in narrow passages.

5 SIMULATIONS

We simulate our algorithm on Matlab/simulink

software. To examine our planner performance we

simulate it on several difficult environments with

variation for each, the predefined distance and the

angular opening constraints. We present some

interesting resulting paths in the figures below:

Figure 3: Computed path1 by the first variant of PSRP.

A NEW HYBRID SAMPLING STRATEGY FOR PRM PLANNERS - To Address Narrow Passages Problem

563

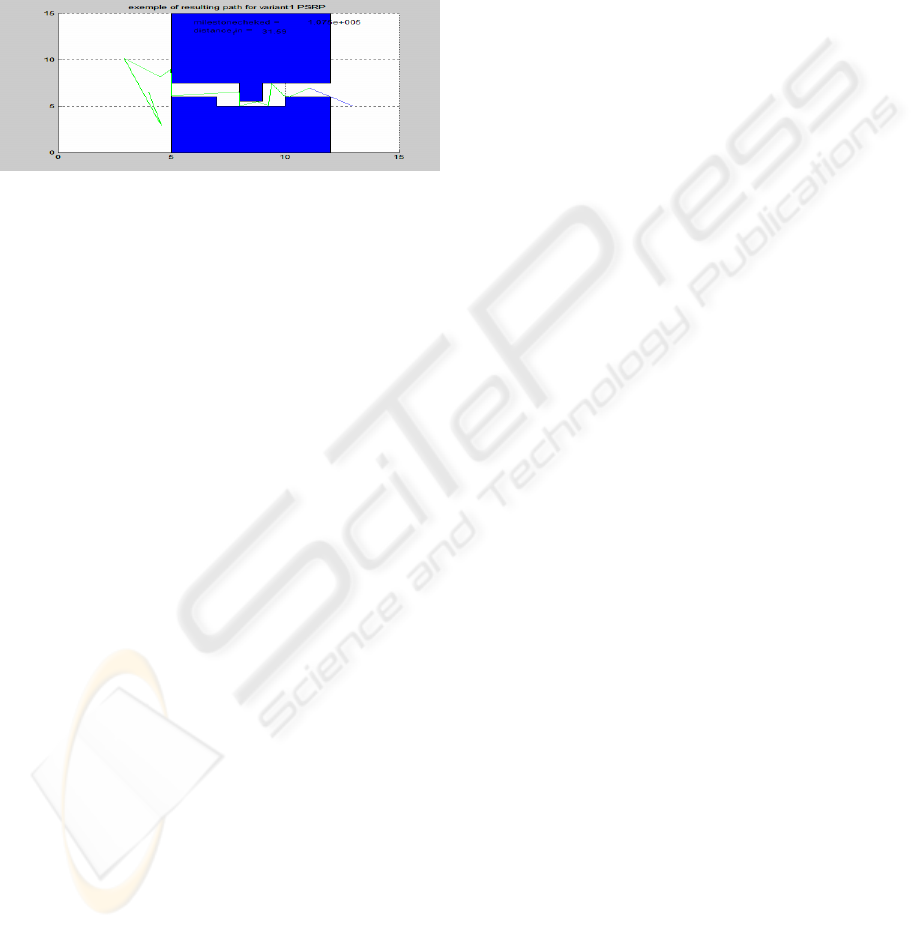
Fig.3: This environment contains two obstacles,

separated by a small narrow passage area. We set the

predefined distance constraint manually to be in [0,

8], the angular constraint was taken in [0, ]2/

The path computed in green colour presents the first

variant algorithm contribution, the second path in

blue colour shows the contribution of the visibility

path component.

Figure 4: computed path2 by the first variant of PSRP.

Fig.4: The environment in Fig.9 presents many

narrow passages. Our PSRP check a large number of

milestones thus, increase

mil

T , but sample only well-

placed milestones

6 CONCLUSIONS AND FUTURE

WORK

We have presented a new probabilistic approach to

address the narrow passages problem. The

simulating results show that (PSRP) gives an

efficient response for this problem. The main issues

that we are interested in exploring further in the

future, is about the predefined distance and the

angular constraints that are chosen manually, a

promising approach is to adjust these two constraints

through on- line learning by taking into accounts the

obstacles positions.

REFERENCES

T.Lozano-Perez., “Spatial planning: A Configuration

space approach”, IEEE Trans. On computers,

Vol.32(2), pp.108-112, 1983

F.Guérin, E.Leclerq, A.Faure, M.Gorka. commande d’un

robot par vision artificielle. JESA 2005.

L.Kavraki and J.-C. Latombe. Randomized preprocessing

of configuration space for fast path planning. In Proc

IEEE Internat. Conf. on Robotics and Automation,

pages 2138-2145, San Diego, USA, 1994.

Th.Horsch, F.Schwartz, and H.Tolle. Motion planning for

many degrees of freedom-random reflections at C-

space obstacles. In proc. IEEE Internat. Conf. on

Robotics and Automation, pages 3318-3323, San

Diego, USA, 1994.

P.Svestka and M.H. Overmars. Motion planning for car-

like robots using a probabilistic larning approach.

Inter. Journal of Rob. Research, 1995.

P.Svestka and J.Vleugels. Exact motion plannig for

tractor-trailer robots. In Proc IEEE Internat. Conf. on

Robotics and Automation, pages 2445-2450, Nagoya,

Japan, 1995.

J.Barraquand, L.E.Kavraki, J.-C.Latombe, T. Li, R.

Motwani, and P.Raghavan, “A random sampling

scheme for path planning” International Journal of

Robotics Research, vol.16, no.6, pp.759-774, 1997.

D.Hsu, J.-C. Latombe and R.Motwani, “path planning in

expansive configuration spaces”, International Journal

of Computational Geometry & Applications, vol.9, no.

4&5, pp.495-512, 1999.

C. Nissoux, T.Simeon and J.-P. Laumond “ Visibility

roadmaps” in Proceeding of the 1999 IEEE/RSJ

International Conference on Intelligent Robot and

Systems, 1999, pp.1316-1321.

V.Boor, M.H.Overmars, and A.F. van der stappen “The

Gaussian sampling strategy for probabilistic roadmap

planners” in Proceedings of the 1999 IEEE

International Conference on Robotics and Automation,

1999, pp. 1018-1023.

A.D.Collins, P.K. Agarwal, and J.L.Harer, “HPRM: A

hierarchical PRM,” in Proceeding of the 2003 IEEE

International Conference on Robotics and Automation,

2003, pp.4433-4438.

D.Hsu, L.E. Kavraki, J., C. Latombe, R. Motwari, and S.

Sorkin, “On finding narrow passages with

probabilistic roadmap planners” in Proceedings of the

Workshop on Algorithmic Foundations of Robotics

1998, pp. 141-153.

S.A.Wilmarth, N.M. Amato, and P.F. Stiller, “Motion

planning for a rigid body using random networks on

the medial axis of the free spaces” in Proceedings of

the 15

Annual ACM Symposium on Computational

Geometry, 1999, pp.173-180.

ICINCO 2006 - ROBOTICS AND AUTOMATION

564