
STABILIZATION OF UNCERTAIN NONLINEAR SYSTEMS VIA
PASSIVITY FEEDBACK EQUIVALENCE AND SLIDING MODE
Rafael Castro-Linares
CINVESTAV-IPN, Department of Electrical Engineering, Av. IPN 2508, Col. San Pedro Zacatenco, 07360 Mexico, D.F., Mexico
Alain Glumineau
IRCCyN, UMR 6597 CNRS, Ecole Centrale de Nantes, 1 rue de la Noe, 44321 Nantes Cedex 03, France
Keywords:
Passivity feedback equivalence, sliding mode technique, stabilization, uncertain nonlinear systems.
Abstract:
In this paper, a sliding mode controller based on passivity feedback equivalence is developed in order to
stabilize an uncertain nonlinear system. It is shown that if the nominal passive system obtained by feedback
equivalence is asymptotically stabilized by output feedback, then the uncertain system remains stable provided
the upper bounds of the uncertain terms are known. The results obtained are applied to the model of a magnetic
levitation system to show the controller methodology design.
1 INTRODUCTION
In the last decade the concept of passivity has been
mainly used in the stability analysis of continous-time
state-space nonlinear systems (Cai and Han, 2005;
Mahmoud and Zribi, 2002) and to analyze the sta-
bility properties of nonlinear interconnected systems
and special cascaded structures (Byrnes et al., 1991;
Ortega, 1991). Besides, an important question arises
when the model of the system contains uncertain el-
ements such as constant or varying parameters that
are not known or imperfectly known. Under such
imperfect knowledge of the model, the feedback that
makes the uncertain system passive is no longer ro-
bust. Some works using nonlinear adaptive control
have been recently devoted to this issue (Su and Xie,
1998; Duarte-Mermoud et al., 2002). On the other
hand, the control of nonlinear systems with uncertain-
ties via the sliding mode technique has been widely
studied in the literature to attain robust control struc-
tures; see, for example the results presented in (Tunay
and Kaynak, 1995).
The goal of the present paper is to develop a con-
troller via passivity feedback equivalence and sliding
modes that permits to stabilize an uncertain nonlin-
ear system. Stabilization is obtained whenever the
passive system associated to the nominal system is
asymptotically stabilized by output feedback; a sim-
ilar approach was presented in (Loria et al., 2001)
where a different sliding surface is proposed. The
study is completed by means of an example of height
distance regulation in the model of a magnetic levita-
tion system.
2 PASSIVITY EQUIVALENCE
AND STABILIZATION USING
SLIDING MODES
One considers uncertain MIMO nonlinear systems
described by
Σ
U
:
˙x = f(x) +∆f(x) + (g(x) + ∆g(x))u,
y = h(x)
(1)
where x ∈ ℜ
n
is the state vector, u ∈ ℜ
p
is the in-
put vector, y ∈ ℜ
p
is the output vector. f and the p
columns of the matrix g are C
∞
vector fields, and the
p components of the vector h are C
∞
functions. ∆f
and the p columns of the matrix ∆g are smooth vector
fields defined on ℜ
n
which represent the model un-
certainties. In addition, we suppose, without loss of
generality and after a possible coordinates shift, that
f(0) = 0 and h(0) = 0. The MIMO nonlinear system
(1) without uncertainties, also referred as the nominal
system, is described by
Σ :
˙x = f(x) + g(x)u,
y = h(x).
(2)
339
Castro-Linares R. and Glumineau A. (2007).
STABILIZATION OF UNCERTAIN NONLINEAR SYSTEMS VIA PASSIVITY FEEDBACK EQUIVALENCE AND SLIDING MODE.
In Proceedings of the Fourth International Conference on Informatics in Control, Automation and Robotics, pages 339-342
DOI: 10.5220/0001614603390342
Copyright
c
SciTePress

This is, Σ is given by Σ
U
with ∆f(x) = 0 and ∆g(x) =
0 for all x.
Let us now assume that the nominal system Σ
has relative degrees r
1
= 1,...,r
p
= 1, that the ma-
trix L
g
h(0) is nonsingular and that it is weakly min-
imal phase; this is, system (2) is locally equiva-
lent to a passive system (Byrnes et al., 1991). Let
S(y, v) = col{S
1
(y,v),.. .,S
p
(y,v)} be an p dimen-
sional smooth function that we refer as the switching
function where v is a new input signal. In this work,
we set S(y, v) as
S(y, v) = y−
t
0
v(τ)dτ. (3)
In the sliding mode, S =
˙
S = 0, and the state trajectory
of the nominal system is constrained to evolve on the
sliding surface M
S
by the so-called equivalent control
u = u
eq
. If an initial point does not belong to M
S
, the
attractivity condition (
˙
S)
T
S ≤ −λ with λ > 0 must be
satisfied in a neighbourhood of M
S
, so that this sur-
face becomes attractive (Utkin, 1992). The control
law which permits to reach the sliding surface can be
obtained from the expression
˙
S = −F(S) where F(S)
is, in general, a discontinuous vector function of its
arguments.
Writting the uncertain system (1) in the new co-
ordinates (y, z), with z being a set of complimentary
coordinates, and substituting the feedback
u = u
slid
= b(y, z)
−1
[−F(S) − a(y, z) + v]. (4)
where b(y, z) is nonsingular for all (y,z) near
(0,0) and setting F(S) = Γsign(S) where
sign(S) := col{sign(S
1
),. . .,sign(S
p
)} and
Γ > 0, one has
˜
Σ
U
:
˙y = v− Γsign(S) + ∆a(y,z)
+∆b(y, z)b
−1
(y, z)(−a(y, z) − Γsign(S) + v)
˙z = f
∗
(z) + p(y,z)y+ {
∑
m
i=1
q
i
(y, z)y
i
}v
+∆p(y,z)y+ {
∑
m
i=1
∆q
i
(y, z)y
i
}v
+{
∑
m
i=1
∆r
i
(y, z)y
i
}Γsign(S)
(5)
where p(y,z) and the q
i
(y,z)’s are suitable matrices
of appropriate dimensions and ˙z = f
∗
(z) are the so
called zero dynamics of the nominal system. ∆p(y,z),
the ∆q
i
(y,z)’s, and the ∆r
i
(y,z)’s are matrices which
represent the terms associated to the uncertainties in
the z variables. ∆a(y,z) and ∆b(y, z) represent the un-
certainties associated to the y variable.
Since it is assumed that the nominal system is
weakly minimal phase, its zero dynamics are Lya-
punov stable with a time-independent and C
2
Lya-
punov function W
∗
(z), and one chooses the signal v
as (Byrnes et al., 1991)
v = [I + M(y,z)]
−1
[−(L
p(y,z)
W
∗
(z))
T
+ w] (6)
where M(y,z) = [(L
q
1
W
∗
)
T
·· · (L
q
p
W
∗
)
T
]
T
. This
choice makes the closed-loop nominal system
[ ˙y
T
˙z
T
] =
f(y,z) + g(y,z)w passive from the input w
to the output y. Assuming that this passive system
is also locally zero state detectable
1
, its equilibrium
(y,z) = (0,0) can be can be made asymptotically sta-
ble by the simple output feedback w = −φ(y) with
φ(0) = 0 and y
T
φ(y) > 0 for each y 6= 0. Let us define
define ξ = (y,z) and substitute the assignment (6) to-
gether with w = −φ(y) into the uncertain system (5).
The resulting closed-loop system can then be written
as
˙
ξ =
¯
F(ξ) +
¯
G(ξ) (7)
where
¯
F(y,z) =
¯
f(y,z)− ¯g(y,z)φ(y),
¯
G(ξ) =
¯
G
1
(ξ)+
¯
G
2
(ξ)
and
¯
G
1
(y,z) =
¯
G
11
(y,z)
0
,
¯
G
2
(y,z) =
0
¯
G
22
(y,z)
(8)
with
¯
G
11
(y, z) = −Γsign(S) + ∆a(y,z)
+∆b(y, z)b
−1
(y, z)(−a(y, z) − Γsign(S)
+[I + M(y, z)]
−1
[−(L
p(y,z)
W
∗
(z))
T
− φ(y)]),
¯
G
22
(y, z) = ∆p(y, z)y+ {
∑
m
i=1
∆r
i
(y, z)y
i
}Γsign(S)
+{
∑
m
i=1
∆q
i
(y, z)y
i
}[I
+M(y, z)]
−1
[−(L
p(y,z)
W
∗
(z))
T
− φ(y)].
We now assume that the uncertain terms satisfy the
uniform bounds
k
¯
G
1
(ξ) k≤ δ
1
, k
¯
G
2
(ξ) k≤ δ
2
(9)
for all ξ ∈ D where D = {ξ ∈ ℜ
n
:|| ξ ||< r} with r > 0
or, equivalently,
k
¯
G(ξ) k≤ δ
1
+ δ
2
= δ (10)
for all D. Notice that ξ = 0 is a locally asymptoti-
cally equilibrium point of the system
˙
ξ =
¯
F(ξ) and
one can then assure, by using the Lyapunov approach,
that for all bounded initial conditions ξ(0), the solu-
tion ξ(t) of the uncertain system (7) is locally ulti-
mately bounded for t ≥ 0. Moreover, one can show
that the sliding surface M
S
becomes attractive for any
initial point ξ(0) ∈ D if
Γ ≥ [1− k ∆bb
−1
sign(S) k]
−1
[k ∆a k
+ k ∆bb
−1
([I + M]
−1
[−(L
p
W
∗
)
T
− φ] − a) k +λ]
(11)
whenever k ∆bb
−1
sign(S) k6= 1, with λ being a
nonzero positive constant (see, in particular, (Khalil,
1996), Lemma 5.3, Chapter 5, p. 216).
1
A system (2) is locally zero-state detectable if there
exists a neighbourhood U of 0 such that, for all x ∈ U,
y(t) = h(x(t)) ≡ 0 implies that x(t) → 0 as t → ∞. It is said
to be locally zero-state observable if there exists a neigh-
bourhood U of 0 such that, for all x ∈ U, y(t) = h(x(t)) ≡ 0
implies that x(t) = 0.
ICINCO 2007 - International Conference on Informatics in Control, Automation and Robotics
340
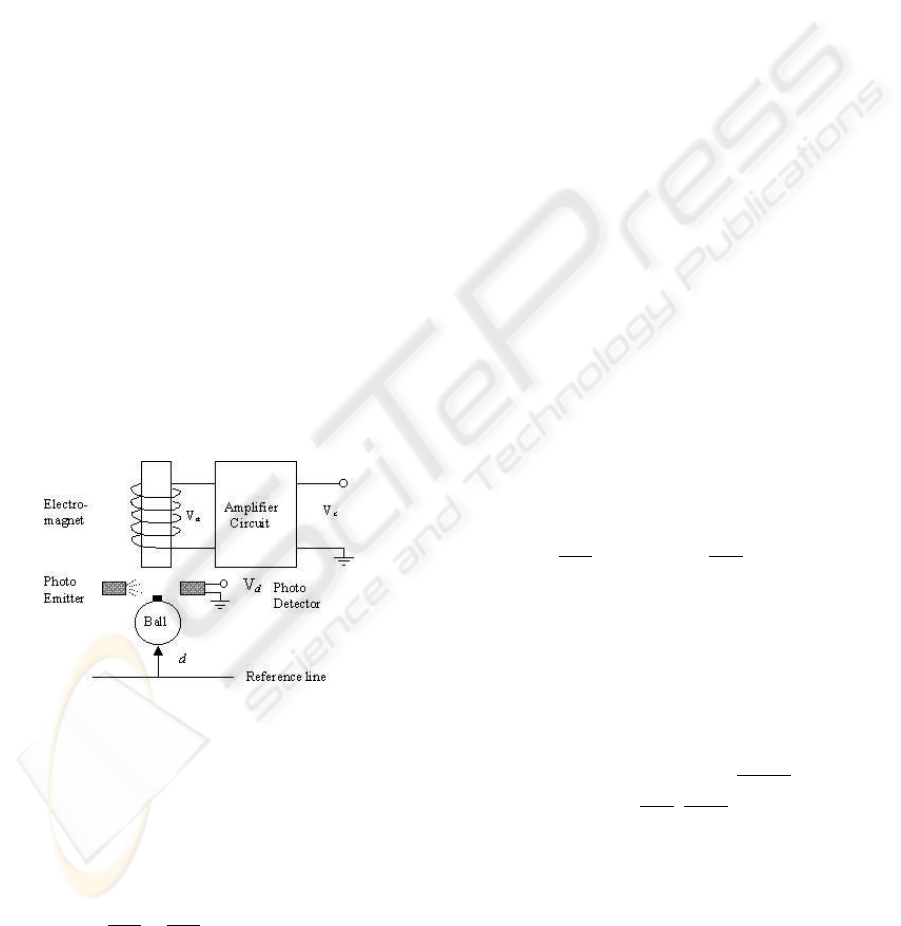
3 APPLICATION TO THE
MODEL OF A MAGNETIC
LEVITATION SYSTEM
In this work we consider the single-axis levitation
system described in (Cho et al., 1993) (see Fig. 1).
A force balance analysis leads to a state space rep-
resentation of the system with state x = (x
1
,x
2
) =
(d − d
0
,
˙
d −
˙
d
0
) and control input u = V
c
−V
c0
where
d is the the distance of the ball from the reference line
and V
c
is the control voltage applied to the amplifier;
d
0
and
˙
d
0
are equilibrium points for a given nominal
control voltage V
c0
. The state space representation is
given by
˙x = f(x) + g(x)u, y = h(x) = x
2
(12)
with
f(x) =
x
2
ˆ
b(x
1
)V
c0
/m− g
, g(x) =
0
ˆ
b(x
1
)/m
(13)
where m is the mass of the ball, g is the gravity
and
ˆ
b(x
1
) = 1/[a
1
(x
1
− d
0
)
2
+ a
2
(x
1
− d
0
) + a
3
], with
a
1
, a
2
and a
3
being real constant parameters. Since
L
g
h(x) =
ˆ
b(x
1
)/m 6= 0, the system has a relative de-
gree r = 1 . Thus, in the coordinates ξ = (y,z) =
(x
2
,x
1
), the levitation system (12),(13) takes the form
˙y = [
ˆ
b(z)V
c0
/m− g] + [
ˆ
b(z)/m]u,
˙z = y.
(14)
Figure 1: Schematic diagram of the magnetic levitation sys-
tem.
The system’s zero dynamics are then described
by the first order differential equation ˙z = f
∗
(z) =
0 for which the quadratic positive definite function
W
∗
(z) = (1/2)z
2
satisfies L
f
∗
W
∗
(z) = 0, and the sys-
tem is weakly minimum phase. One then has that the
feedback
u =
m
ˆ
b(z)
[−
ˆ
b(z)
m
V
c0
+ g− z+ w] (15)
makes the system (14) feedback equivalent to a C
2
passive system from w to y with a C
2
storage func-
tion V = W
∗
(z) + (1/2)y
2
. Even more, the resultant
closed-loop system is a loosless one because of the
fact that
˙
V = yw. One can also verify that this closed-
loop system is zero-state observable, thus the addi-
tional feedback
w = −ky, (16)
with k > 0, can make the origin (y, z) = (0,0) of the
system
˙
ξ =
˙y
˙z
=
¯
F(ξ) =
−k −1
1 0
ξ =
¯
Aξ. (17)
asymptotically stable.
In (Cho et al., 1993) it is noticed that the solenoid
characteristics change with temperature, and a change
of ±20% can appear in
ˆ
b(x
1
) when the levitation sys-
tem has been operated for a short period of time.
Thus, the actual force-distance relationship, denoted
by b(d), may be expressed as
b(d) =
ˆ
b(d) + ∆
ˆ
b(d) (18)
where ∆
ˆ
b(d) is an unknown modeling error which can
be as high as 20% of
ˆ
b(d). The uncertain model asso-
ciated to the nominal model (14) can then be written,
also in the coordinates ξ = (y,z), as
˙y = [
ˆ
b(z)V
c0
/m− g] + [∆
ˆ
b(z)V
c0
/m]
+([
ˆ
b(z)/m] + [∆
ˆ
b(z)/m])u,
˙z = y.
(19)
This is, the uncertainties are given by ∆a(y,z) =
∆
ˆ
b(z)V
c0
/m and ∆b(y,z) = ∆
ˆ
b(z)/m.
The switching function S(y,v) is given by (3) with
v = −z+ w. Such a choice leads to the control law
u = u
slid
=
m
ˆ
b(z)
[−Γsign(S) −
ˆ
b(z)
m
V
c0
+ g− z+ w],
(20)
with Γ > 0, which allows to reach the sliding surface
in a finite time. By selecting the additional output
feedback (16), we obtain the closed-loop system
˙
ξ =
¯
Aξ+
¯
G(ξ) (21)
where
¯
G(ξ) =
¯
G
1
(ξ) =
[−Γsign(S) +
∆
ˆ
b(z)V
c0
m
+
∆
ˆ
b(z)
ˆ
b(z)
[
ˆ
b(z)V
c0
m
+ g− Γsign(S)
−z− ky]]
0
(22)
From the size of the modelling error ∆
ˆ
b(z) one can
verify, after some computations, that the uncertainty
term
¯
G
1
(ξ) satisfies the uniform bound ||
¯
G
1
(ξ) ||≤ δ
for a constant δ. It then follows that the solution ξ(t)
of the uncertain system (21) is ultimately bounded for
t ≥ 0.
STABILIZATION OF UNCERTAIN NONLINEAR SYSTEMS VIA PASSIVITY FEEDBACK EQUIVALENCE AND
SLIDING MODE
341
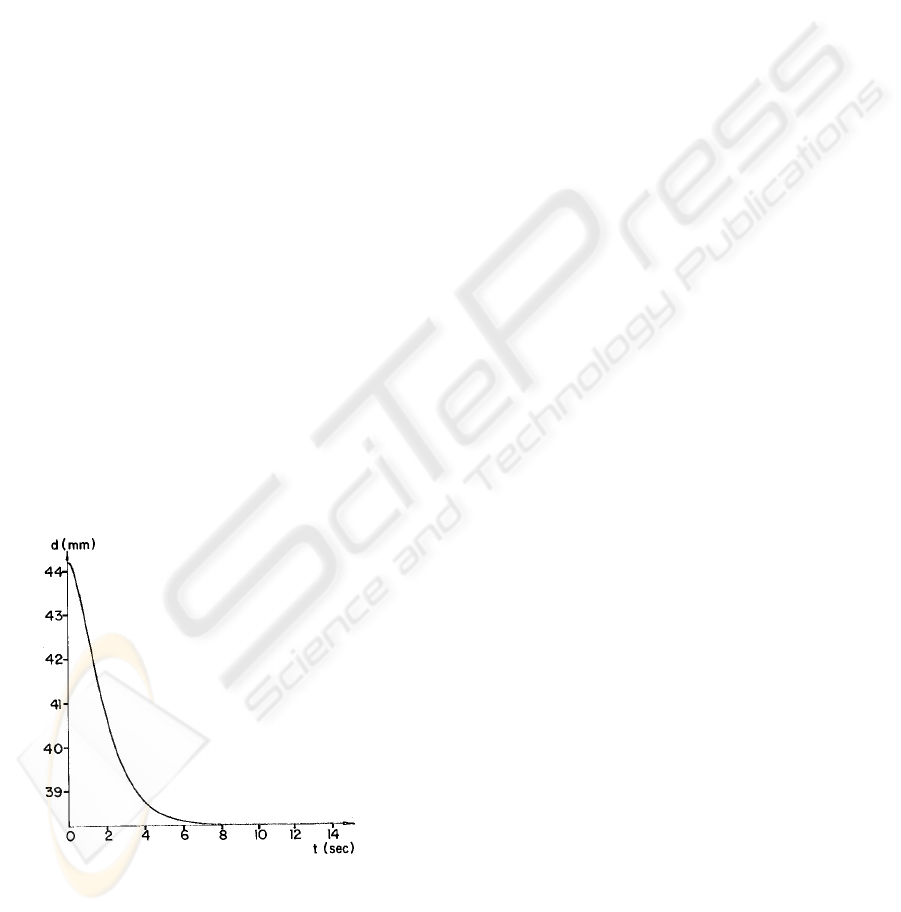
The magnetic levitation system described by
equations (12),(13) was simulated together with the
passivity based sliding mode controller (3),(20). The
nominal value of the ball’s mass m and the con-
stant coefficients used in the force-distance relation-
ship
ˆ
b(z) were selected as in (Cho et al., 1993), this
is m = 2.206 gr, a
1
= 0.0231/mg, a
2
= −2.4455/mg,
a
3
= 64.58/mg. In fact, as it is noted in (Cho et al.,
1993), the validity of the
ˆ
b(x
1
) is constrained to the
range of 35 mm and 48 mm. By choosing the nominal
value of the control applied to the amplifier circuit to
beV
c0
= 4.87 volts, we obtained the equilibrium point
(d
0
,
˙
d
0
) = (38.2 mm,0 mm/sec). The initial condi-
tions of the magnetic levitation system were fixed to
x
1
(0) = 44.2 mm and x
2
(0) = 0 mm/sec, while the
controller parameters were selected as Γ = 10 and
k = 2. In order to diminish the effect of chattering
due to the discontinuity of the sign function, a satura-
tion function given by
sat(S) =
1, if S > ε
S/ε, if −ε ≤ S ≤ ε
−1, if S < −ε
with ε > 0, was used instead of the sign function.
In order to evaluate the performance of the control
scheme, a variation of 20% in the value of the func-
tion
ˆ
b(z) was introduced at t = 7 sec in all the simula-
tions. The time closed-loop plot corresponding to the
distance d is shown in Figures 2 for ε = 0.001. From
this plot, we can notice that the distance of the ball to
the reference line is always regulated to the equilib-
rium point d
0
= 38.2 mm with no overshoot.
Figure 2: Closed-loop response of the distance, d; ε =
0.001.
4 CONCLUSIONS
In this paper, a passivity-based sliding mode con-
troller design that allows to stabilize an uncertain non-
linear system has been presented. The proposed con-
troller has also been applied to the model of a mag-
netic levitation system in order to regulate the height
of a levitated ball around at one of its equilibria.
REFERENCES
Byrnes, C. I., Isidori, A., and Williams, J. C. (1991). Passiv-
ity, feedback equivalence, and the global stabilization
of minimum phase nonlinear systems. In IEEE Trans-
actions on Automatic Control, vol.36, pp. 1228-1240.
IEEE.
Cai, X. S. and Han, Z. Z. (2005). Inverse optimal con-
trol of nonlinear systems with structural uncertainty.
In IEE Proceedings Control Theory and Applications,
vol. 152, pp. 79-83. IEE.
Cho, D., Kato, Y., and Spilman, D. (1993). Sliding mode
and classical control of magnetic levitation systems.
In IEEE Control Systems, pp. 42-48. IEEE.
Duarte-Mermoud, M. A., Castro-Linares, R., and Castillo-
Facuse, A. (2002). Direct passivity of a class of mimo
non-linear systems using adaptive feedback. In Inter-
national Journal of Control, vol. 75, pp. 23-33. Taylor
and Francis.
Khalil, H. (1996). Nonlinear Systems. MacMillan Publish-
ing Company, New York, 2nd edition.
Loria, A., Panteley, E., and Nijmeier, H. (2001). A remark
on passivity-based and discontinuous control of un-
certain nonlinear systems. In Automatica, vol.37, pp.
1481-1487. Elsevier.
Mahmoud, M. S. and Zribi, M. (2002). Passive control
synthesis for uncertain systems with multiple-state
delays. In Computers and Electrical Enginnering,
vol.28, pp. 195-216. Pergamon.
Ortega, R. (1991). Passivity properties for stabilizing of
cascaded nonlinear systems. In Automatica, vol. 27,
pp. 423-424. Elsevier.
Su, W. and Xie, L. (1998). Robust control of nonlinear feed-
back passive systems. In Systems Control Letters, vol.
28, pp. 85-93. Elsevier.
Tunay, I. and Kaynak, O. (1995). A new variable struc-
ture controller for affine nonlinear systems with non-
matching uncertainties. In International Journal of
Control, vol. 62, pp. 917-939. Taylor and Francis.
Utkin, V. I. (1992). Sliding Modes in Control and Optimiza-
tion. Springer, New York, 1st edition.
ICINCO 2007 - International Conference on Informatics in Control, Automation and Robotics
342
