
IRREVERSIBILITY MODELING APPLIED TO THE CONTROL
OF COMPLEX ROBOTIC DRIVE CHAINS
Omar Al Assad, Emmanuel Godoy
Ecole Supérieure d’Electricité (Supélec) Gif-sur-Yvette F-91192 Cedex, France
Vincent Croulard
General Electric Healthcare, 283 rue de la Minière, Buc F-78530 Cedex, France
Keywords: Robotic, Modeling, Drive chain, Mechanical irreversibility, Efficiency, Gearbox.
Abstract: The phenomena of static and dry friction may lead to difficult problems during low speed motion (e.g. stick
slip phenomenon). However, they can be used to obtain irreversible mechanical transmissions. The latter
tend to be very hard to model theoretically. In this paper, we propose a pragmatic approach to model
irreversibility in robotic drive chains. The proposed methodology consists of using a state machine to
describe the functional state of the transmission. After that, for each state we define the efficiency
coefficient of the drive chain. This technique gives conclusive results during experimental validation and
allows reproducing a reliable robot simulator. This simulator is set up for the purpose of position control of
a medical positioning robot.
1 INTRODUCTION
Modern control theories in robotics are more and
more turned towards model-based controllers such as
computed torque controllers, adaptive controllers or
feedforward dynamics compensators. Therefore,
dynamic modeling has become an inevitable step
during controllers design. Besides, accurate dynamic
modeling is a key point during simulations and the
mechanism design process.
In the literature, the problem of robot dynamic
m
odeling is treated in two steps. The first one
concerns the mechanical behavior of the robot
external structure considered often as a rigid
structure. Many researchers have treated this
problem and different techniques have been
introduced to solve this issue. The two best-known
methods in this matter are the Newton-Euler
formulation and the Lagrange formulation (Khalil,
1999). The second step concerns the drive chain
modeling which includes motors, gears and power
loss modeling. Despite the advances made in the
field of mechanical modeling, some issues are still
without a convenient solution. We can mention, for
instance, the phenomenon of irreversibility that
characterizes certain types of mechanical
transmissions such as worm gears (Henriot, 1991).
This characteristic is often required for security
reasons like locking the joint in case of motor failure
or unexpected current cut-off. The purpose of this
paper is to present a new modeling approach based
on a state machine in order to simulate irreversible
transmissions.
This paper is organized as follows. In section 2,
we
give a brief overview of the LCA vascular robot,
which is used as an application for this study.
Section 3 presents details about the modeling
approach used for the robot structure and drive chain.
Section 4 presents the irreversibility modeling issue
and the proposed solution. Section 5 illustrates the
experimental validation results. Finally, section 7
presents some concluding remarks.
2 LCA ROBOT PRESENTATION
The LCA vascular robot (figure 1) is used for
medical X-ray imaging. It is a four-degrees-of-
freedom open-chain robot composed of the following
links: the L-arm (rotational joint), the Pivot
(rotational joint), the C-arc (it has a translation
movement in a circular trajectory template. Hence, it
217
Al Assad O., Godoy E. and Croulard V. (2007).
IRREVERSIBILITY MODELING APPLIED TO THE CONTROL OF COMPLEX ROBOTIC DRIVE CHAINS.
In Proceedings of the Fourth International Conference on Informatics in Control, Automation and Robotics, pages 217-222
DOI: 10.5220/0001629802170222
Copyright
c
SciTePress
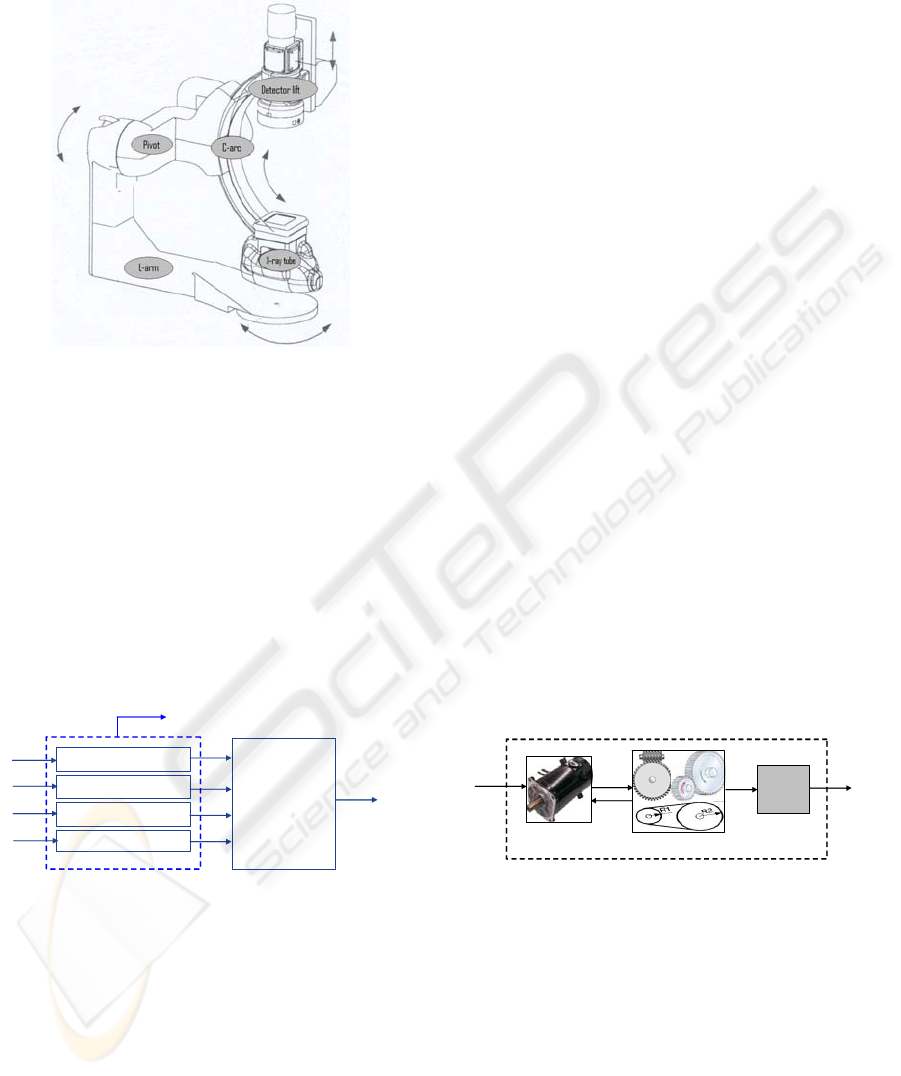
can be considered as a rotational joint around the
virtual axis crossing the C-arc center) and the Lift
(prismatic joint)
Figure 1: LCA robot.
3 MODELING APPROACH
The modeling of the LCA robot requires a clear
distinction between the dynamic model of the
mechanical structure and the drive chain model
(figure 2). In fact, the dynamic model describes
merely the relation between the applied torques and
the ideal mechanical reaction of the gantry given by
the joints acceleration.
The drive chain model takes into account the hard
nonlinearities of the system such as the joint friction
and the gear irreversibility.
L-arm Motorisation
Pivot Motorisation
C-arc Motorisation
Lift Motorisation
Robot
Dynamic
Model
Drive chain
Position
Velocity
Accelerati
o
Γ
1
Γ
2
Γ
3
V
4
V
1
V
2
V
3
Γ
4
Current
i
V
are the motors command voltage.
i
Γ
are the axes driving torques.
Figure 2: The robot model structure.
3.1 Dynamic Modeling
Two main methods can be used to calculate the
dynamic model of the robot mechanical structure.
We can mention the Newton-Euler formulation and
the Lagrange formulation (Khalil, 1999).
Most authors use the Lagrange formulation that
gives the mathematical expression of the model as:
)(),()( qQqqqCqqA +
+
=
Γ
(1)
Where are respectively the vectors of joints
position, velocity, and acceleration.
qqq
,,
)(qA
: the 4x4 robot inertia matrix.
qqqC
⋅
),(
: the 4x1 Coriolis and centrifugal torque/
forces vector.
)(qQ
: the 4x1 gravitational torques/ forces vector.
Γ
: the 4x1 input torques/ forces vector.
To simulate the robot movement, we should use the
inverse of the dynamic model as follow:
),,( qqfq
Γ
=
This model can be obtained directly using the
recursive Newton-Euler equations, or it can be
inferred from equation (1):
(2)
))(),(()(
1
qQqqqCqAq −−Γ=
−
The “A” matrix is inverted symbolically; this will
result in a heavy mathematical expression, costly in
term of computation time. Alternatively, this inverse
can be calculated after numerical calculation
of which leads to faster simulations.
)(qA
3.2 Drive Chain Modeling
The next step consists of modeling the drive chain,
which includes the electrical motor (DC motor for
this application), the mechanical transmission (gears)
and the elements of power dissipation (friction)
(figure 3).
We will describe briefly the first and the second
elements and emphasize the third element, which is
the purpose of this paper.
Γ
i
V
i
gears
Γ
m
Motor
Friction model
Γ
g
Ω
m
Figure 3: Drive chain model.
Actually, the phenomenon of irreversibility,
obtained using specific transmissions and particular
geometric dimensioning, is a complex problem and
leads instinctively to non linear models. It can be
treated using several approaches. In a microscopic
point of view, the contacts among driving and driven
elements are modeled as well as the applied forces.
However, this rigorous approach leads to very
complex analytical models, with serious difficulties
in the implementation and simulations phases,
particularly in the case of closed loop structures
ICINCO 2007 - International Conference on Informatics in Control, Automation and Robotics
218
including controllers (Henriot, 1991). Besides, the
identification of this type of models is very
complicated due to the significant number of
parameters. In a macroscopic point of view, the
power transfer between the motor and the load is
modeled with an efficiency coefficient taking into
account the power transfer direction (load
driving/driven) (Abba, 1999), (Abba, 2003).
However, a proportional coefficient is insufficient to
represent the irreversibility behavior. In our
approach, we suggest the use of a state machine to
define the current functional state of the transmission
in order to reproduce the irreversibility.
3.2.1 DC Motor Modeling
The DC motor is a well-known electromechanical
device. Its model has two inputs, the armature
voltage and the shaft velocity. The output is the
mechanical torque applied on the shaft. The DC
motor behavior is modeled using two equations
(Pinard, 2004) the electrical equation of the armature
current (3) and the mechanical equation of the motor
torque (4):
dt
dI
LIREV ⋅+⋅=−
(3)
where
V
is the motor voltage.
I
is the armature
current. is the electromotive force. is
the motor velocity; and the motor parameters
are: (the back electromotive constant), (the
motor resistance) and (the motor inductance).
memf
qKE
⋅=
m
q
emf
K
R
L
IK
tm
⋅
=Γ
(4)
where is the motor torque and is the motor
torque constant ( )
m
Γ
t
K
emft
KK =
3.2.2 Gears Modeling
In this paper, we consider rigid gears’ models. In this
case, the model’s mathematical expression depends
only on the gear ratio N. Therefore, the output torque
is obtained using the following relation:
mg
N
Γ
⋅
=
Γ
and the speed of the motor shaft is obtained using:
.
qNq
m
⋅=
The gear’s ratio is given by simple mathematical
expressions (Henriot, 1991) or via the gears
datasheet.
3.2.3 The Power Dissipation in Drive Chain
This section is the most essential in drive chain
modeling. In fact, good power dissipation modeling
helps to reproduce complex gear behaviors such as
irreversibility. The power dissipation will be
illustrated through the friction phenomenon.
In robotics, friction is often modeled as a function
of joint velocity. It is based on static, dry and viscous
friction (Khalil, 1999), (Abba, 2003). These models
produce accurate simulation results with simple drive
chain structures. However, in the presence of more
complex mechanisms such as worm gears these
models lack reliability.
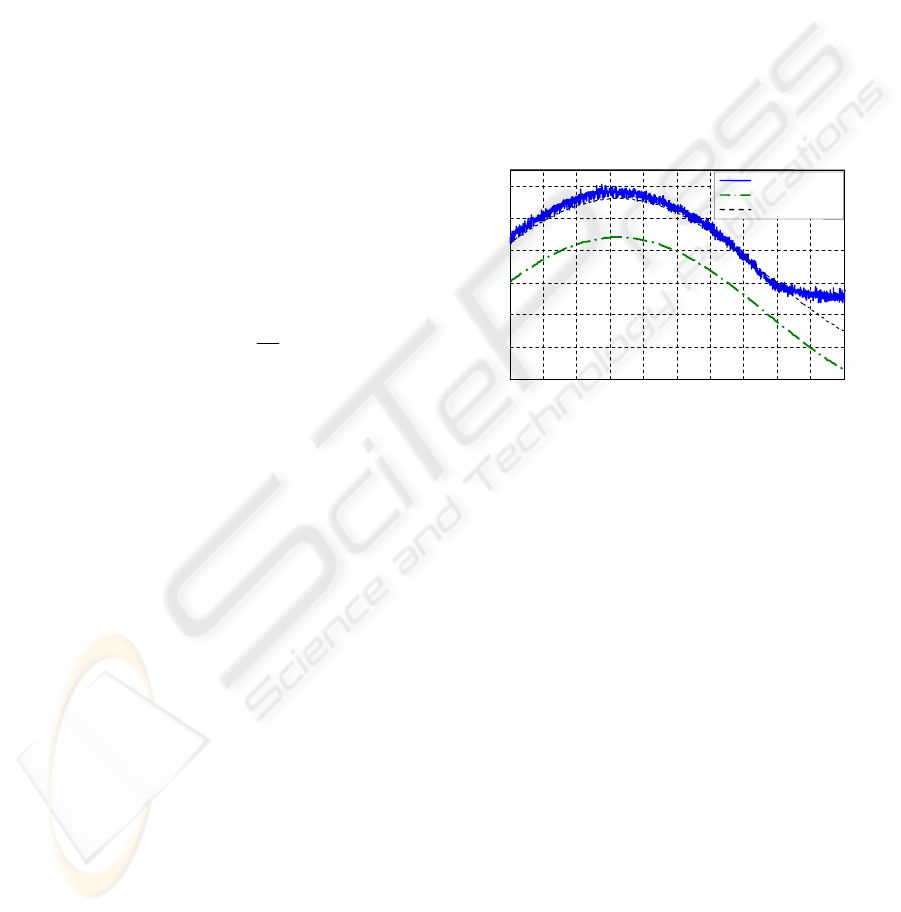
To illustrate this phenomenon, we can compare
the theoretical motor torque required to drive the
LCA pivot axis in the case of a reversible
transmission and the real measured motor torque.
Figure 4 and 5 show the applied torques on the pivot
axis during a 7°/sec and -7°/sec constant velocity
movement.
-100 -80 -60 -40 -20 0 20 40 60 80 100
-2
-1
0
1
2
3
4
Axis position (deg)
Torque (Nm)
Measured Tm
Load Torque
Reversible gear Tm
Figure 4: Motor and load torque variation during constant
velocity rotation (7 °/s).
During this movement, the robot dynamic is
represented by the following dynamic equation:
flm
Γ+Γ
=
Γ
(5)
where
l
Γ
is the load torque and is the friction
torque. Consequently, we expect that the motor
torque will have the same behavior as the load torque
because the friction torque is constant. However,
these results reveal an important difference between
the measured motor torque and the expected motor
torque with a drive chain using only velocity friction
model.
f
Γ
IRREVERSIBILITY MODELING APPLIED TO THE CONTROL OF COMPLEX ROBOTIC DRIVE CHAINS
219

-100 -80 -60 -40 -20 0 20 40 60 80 100
-3
-2
-1
0
1
2
3
Axis position (deg)
Torque (Nm)
Reversible gear Tm
Measured Tm
Load Torque
Figure 5: Motor and load torque variation during constant
velocity rotation (-7 °/s).
We can see that the irreversibility seriously
influences the motor torque. Actually, the
irreversibility compensates the gravity torque when
the load torque becomes driving. Therefore, it is
essential to expand the friction model to take into
consideration more variables such as motor torque
and load torque in order to reproduce irreversibility
in a simulation environment. Thus, the friction model
applied on motor shaft will have the following
structure:
f
Γ
),,()(),(
mlmfTmfvmmfsf
qqq
ΓΓΓ+Γ+ΓΓ=Γ
(6)
where :
),(
mmfs
q
ΓΓ
: 4x1 vector of the static friction model
)(
mfv
q
Γ
: 4x1 vector of the velocity friction model
),,(
mlmfT
q
ΓΓΓ
: 4x1 vector of the torque friction
model.
fs
Γ
and are the classical friction terms used
usually in drive chain modeling (Dupont, 1990),
(Armstrong, 1998). While, presents the term that
takes account of the irreversibility behavior.
fv
Γ
fT
Γ
limilimili
mimilimimimilimifTi
q
qq
Γ⋅ΓΓ+
Γ⋅Γ
Γ
=ΓΓΓ
),,(
),,(),,(
μ
μ
(7)
where
),,(
milimimi
q
ΓΓ
μ
and
),,(
milimili
q
ΓΓ
μ
are
the motor and load friction dynamic coefficients.
Let’s consider now the complete robot dynamic
model:
flmmm
qqANqJ Γ+Γ++⋅=Γ
−
)(
1
(8)
where and is the 4x4
motors and gears inertia matrix. By replacing (6) in
(8) we obtain:
))(),((
1
qQqqqCN
l
+=Γ
−
m
J
llmmmfv
mmfslmmm
q
qqqANJ
Γ⋅+Γ⋅+Γ+
ΓΓ+Γ+⋅+=Γ
−
μμ
)(
),())((
2
(9)
where
m
μ
and
l
μ
are respectively 4x4 diagonal
matrixes:
{
}
{}
4,,1;)],,([diag
4,,1;)],,([diag
…
…
=ΓΓ=
=ΓΓ=
iq
iq
milimilil
milimimim
μμ
μμ
By regrouping the terms of equation 11 we obtain:
(10)
)(),(
))((
2
mfvmmfs
llmmmm
qq
qqANJ
Γ+ΓΓ+
Γ⋅+⋅+=Γ⋅
−
ηη
where
)(
44 mm
I
μ
η
−
=
×
and
)(
44 ll
I
μ
η
+=
×
.
The new terms
m
η
and
l
η
, which depend on
m
Γ
,
l
Γ
and , introduce the efficiency concept in the
robot dynamic model. The next section will focus on
the proposed approach used to calculate the drive
chain efficiency coefficients.
m
q
4 EFFICIENCY COEFFICIENTS
ESTIMATION
One of the complex issues in drive chain modeling is
the estimation of the transmission efficiency
coefficient. One technique consists of theoretically
calculating the efficiency of each element of the
drive chain using the efficiency definition (Henriot,
1991):
in
out
P
P
==
Power Emitted
Power Received
η
(11)
The calculation of this coefficient requires the
determination of the driving element whether it is the
motor or the load. We talk then about the motor
torque efficiency (
m
η
) or the load torque efficiency
(
l
η
). Therefore, the received power “ ” could be
either from the motor or the load.
in
P
Actually, this method can be applied with simple
gear mechanisms such as spur gears, whereas for
complex gears, such as worm gears, the calculation
of the efficiency coefficient using analytical
formulas tends to be hard and inaccurate due to the
lack of information concerning friction modeling as
well as the complexity of the contact surface
between gears’ components (Henriot, 1991). The
alternative that we propose is to experimentally
identify the efficiency coefficient according to a
functional state of the drive chain, for instance, when
the load is driving the movement or when the motor
is driving the movement. This leads us to create a
state machine with the following inputs and outputs:
Table 1: State machine inputs and outputs.
ICINCO 2007 - International Conference on Informatics in Control, Automation and Robotics
220
Inputs Outputs
m
Γ
: motor torque (Tm)
m
η
: motor efficiency
l
Γ
: load torque (Tl)
l
η
: load efficiency
m
q
: motor velocity
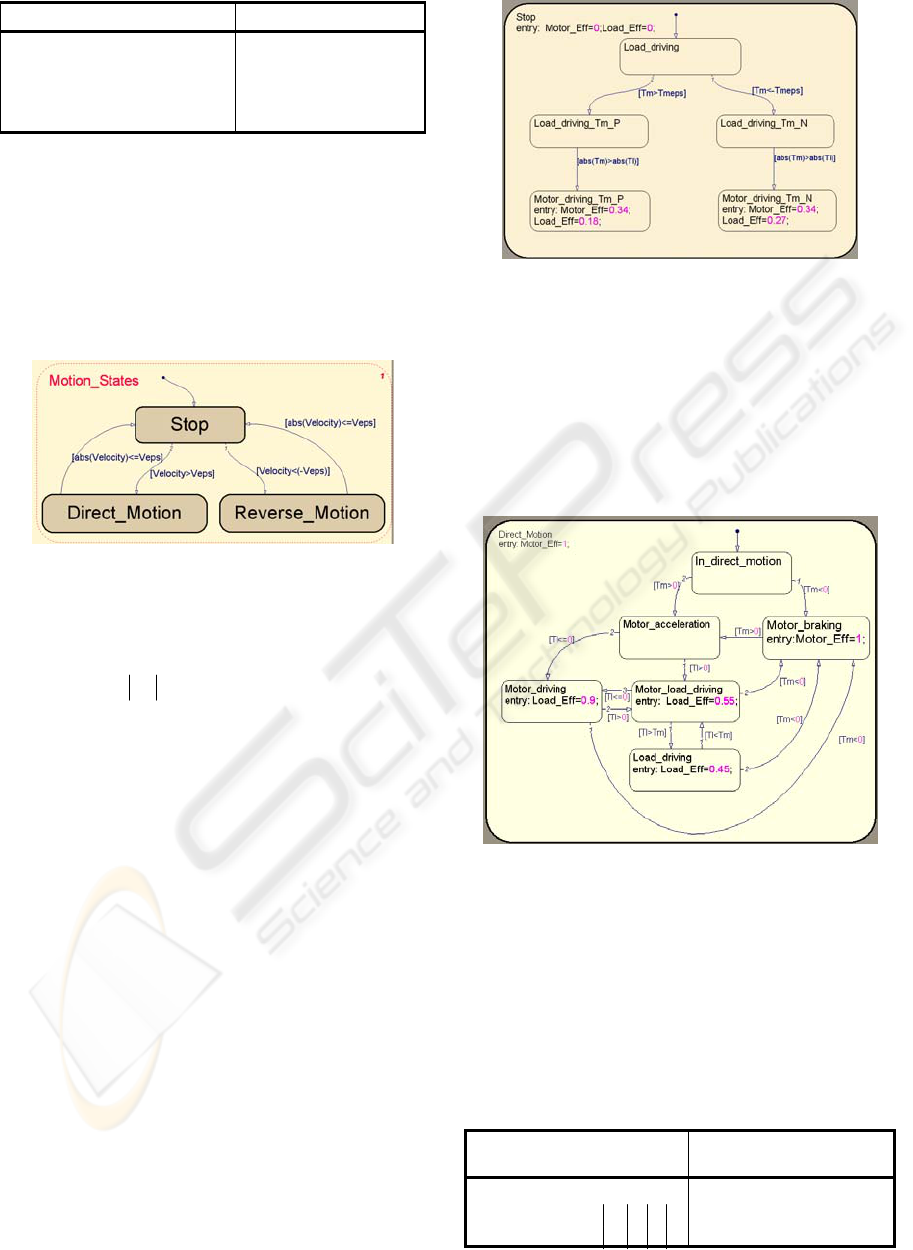
Now, we will present the states and the criteria of
states transitions that we have used for LCA robot
drive chain modeling. The state machine includes
two levels: the upper level that describes the motion
(figure 6) and the lower level that describes the
switch between motor driving and load driving states
(figures 7, 8), and associates an efficiency coefficient
for each state. In this level, the transition condition is
the sign of the velocity.
Figure 6: Motion state machine.
In the upper level, the transition condition is the
sign of the velocity. In fact, for simulation
convergence issue the drive chain is considered
stopped when
epsm
Vq <
, where is the stop
velocity threshold.
eps
V
In the lower level, a sub-state has been combined
to each motion state:
• The stop states (figure 7)
During the stop phase, the drive chain is
irreversible (the load torque cannot drive the
movement). Motion is observed when the motor
torque becomes superior to the load torque.
In the lower level, the state transition is based on
the motor and load torque values. As for (figure
6), represents the motor torque threshold, it is
used for simulation convergence issue
( ).
eps
V
eps
Tm
Nm 10
5−
=
eps
Tm
Figure 7: Stop state machine.
• The direct motion states
For the direct motion state (if V>0), we have four
main states (figure 8), the states transitions are given
by the following conditions: and : the
motor is driving;
0>Γ
m
0<Γ
l
0>
Γ
m
and : we distinguish
two states whether
0>Γ
l
ml
Γ
>
Γ
or not; and : the
motor is braking (load driving)
0<Γ
m
Figure 8: direct motion state machine.
• The reverse motion states
The reverse motion (V<0) state machine has the
same structure as the direct motion one. We need to
replace
m
Γ
and
l
Γ
by
m
Γ
−
and . The table 1
summaries the drive chain efficiency coefficients for
each state: (Motor driving / Motor and load driving /
Load driving).
l
Γ−
Table 2: Drive chain efficiency coefficients.
States
Direct
motion
l
η
Reverse
motion
l
η
1-
0
<
Γ
⋅
Γ
lm
0.9 0.9
2-
0>
Γ
⋅
Γ
lm
&
lm
Γ<Γ
0.55 0.16
3-
0>
Γ
⋅
Γ
lm
&
lm
Γ>Γ
0.45 0.06
IRREVERSIBILITY MODELING APPLIED TO THE CONTROL OF COMPLEX ROBOTIC DRIVE CHAINS
221
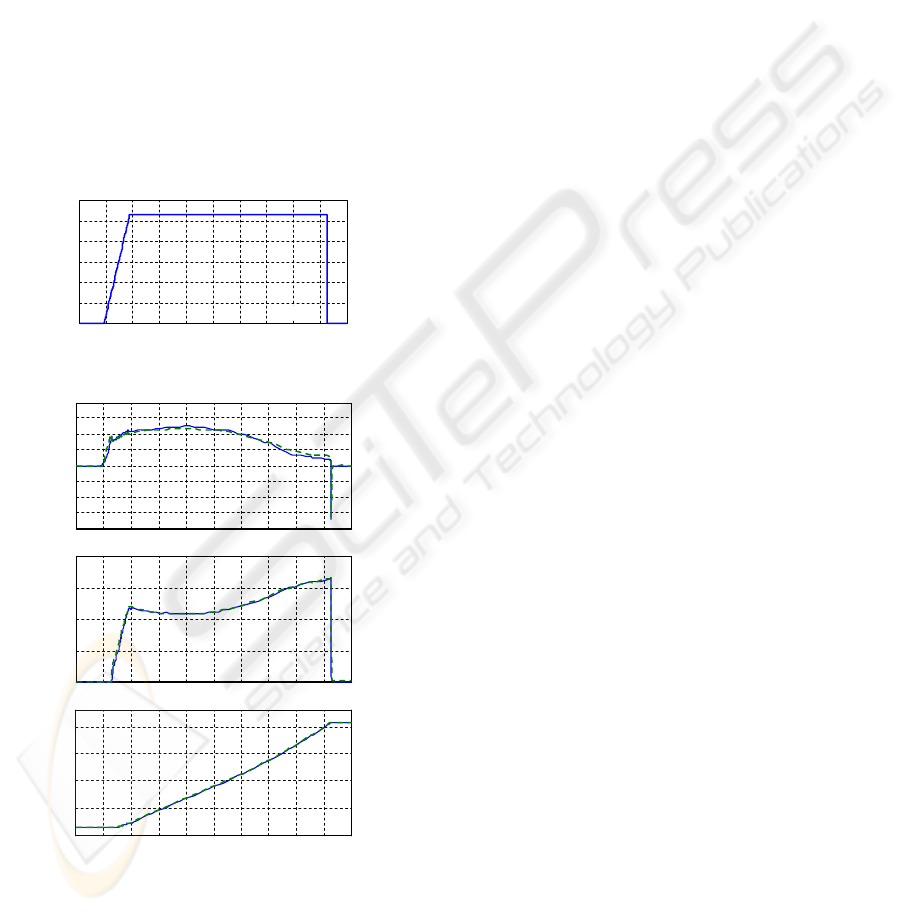
5 EXPERIMENTAL VALIDATION
The validation of the drive chain model has been
done on the pivot axis. The efficiency coefficients
have been identified using experimental measures.
We compare the open loop response of the pivot
joint and the simulation results to a voltage input for
both direct and reverse motion. Figure 9 shows the
applied voltage on the motor pivot axis for direct
motion. Figure 10 shows the experimental results
(dashed curve) of current, velocity and position and
those obtained in simulation (solid curve). We notice
in that the simulation response represents the same
behavior as the real mechanism. In this figure we
distinguish four main phases: the starting phase 24s
to 25s, the motor driving phase 25s to 37.8s, the load
driving phase 37.8s to 4.2s and the braking phase
4.2s to 4.3s.
22 24 26 28 30 32 34 36 38 40 42
0
10
20
30
40
50
60
Time (s)
Motor voltage (V)
Figure 9: Open loop motor command voltage.
22 24 26 28 30 32 34 36 38 40 42
-20
-15
-10
-5
0
5
10
15
20
Current (A)
22 24 26 28 30 32 34 36 38 40 42
0
5
10
15
20
Velocity (deg/sec)
22 24 26 28 30 32 34 36 38 40 42
-100
-50
50
100
130
0
Time (s)
Position (deg)
Figure 10: Direct motion outputs.
By comparing the obtained results, we notice that
the differences are low for direct motion as well as
for reverse motion. Therefore, these results prove
that the used model is able to represent accurately the
irreversibility property of the pivot drive chain.
6 CONCLUSIONS
In this paper, we presented a methodology in order to
model the irreversibility characteristic in
electromechanical drive chains. The proposed
approach uses a macroscopic modeling of the gears,
which are usually the origin of irreversibility in a
drive chains. It consists of creating a state machine
representing different functional states of the gears
and attributing an efficiency coefficient to each
specific state.
The validation of the proposed modeling was
carried out on the Pivot axis of the LCA robot. The
methodology has been tested in particular when the
position trajectory leads to some transitions “motor
driving to load driving” and the obtained results
confirm the correctness of the used model.
The perspectives of this work concern two
research orientations. The first one is the definition
and the study of an automatic procedure to identify
the efficiency coefficient for each state. The second
one is the investigation of the trajectory planning and
the control of robots with irreversible transmissions
when considering state machines for gear’s
modeling.
REFERENCES
Abba G., Chaillet N. (1999) “Robot dynamic modeling
using using a power flow approach with application to
biped locomotion”, Autonomous Robots 6, 1999, pp.
39–52.
Abba G., Sardain P.(2003), “Modélisation des frottements
dans les éléments de transmission d'un axe de robot en
vue de son identification: Friction modelling of a robot
transmission chain with identification in mind”,
Mécanique & Industries, Volume 4, Issue 4, July-
August, pp 391-396
Armstrong B. (1998), “Friction: Experimental
determination, modelling and compensation”, IEEE
International Conference on Robotics and Automation,
Philadelphia, PA, USA, April, vol. 3, pp. 1422–1427.
Dupont P.E.(1990) “Friction modeling in dynamic robot
simulation”, Robotics and Automation, 1990.
Proceedings., IEEE International Conference, pp.
1370-1376 vol.2
Henriot G. (1991) “Traité théorique et pratique des
engrenages”, 5
th
ed. Dunod ed. vol. 1.
Khalil W., Dombre E. (1999) “Modélisation identification
et commande des robots”, 2
nd
ed. Hermes ed.
Pinard M. (2004), “Commande électronique des moteurs
électriques”, Paris Dunod ed. pp 157-163
ICINCO 2007 - International Conference on Informatics in Control, Automation and Robotics
222
