
FEASIBILITY OF SUBSPACE IDENTIFICATION FOR BIPEDS
An Innovative Approach for Kino-Dynamic Systems
Muhammad Saad Saleem and Ibrahim A. Sultan
School of Science and Engineering, University of Ballarat, Mount Helen, Victoria, Australia
Keywords:
Biped, subspace identification, kino-dynamic, operational space control, biped stability, crisp control.
Abstract:
Different approaches have been briefly overviewed which have been used in stability of biped robots. Current
implementations either mimic human behavior or use heuristic control. This paper suggests the use of model-
free crisp control in operational space configuration for better control and understanding of kino-dynamic
systems and biped robots.
1 INTRODUCTION
Designing a control strategy for a biped robot can
be quite tedious as dynamics involved are non-linear,
multi-variable, naturally unstable and foot-ground in-
teraction is limited (Wolkotte (2003); Kim et al.
(2004); Caballero et al. (2004)). These all problems
suggest that controller should be sophisticated enough
to cater for all these factors. This is why most imple-
mentations don’t use classical control techniques but
rely on techniques which mimic human behavior or
are based on heuristic control (Pratt (2000)).
In order to examine crisp control and a mathemat-
ical solution for biped stability using other than above
mentioned techniques, subspace identification is pro-
posed, which then can be coupled with post-modern
control techniques such as H
∞
to design a model-free
control system. In theory such controllers are devel-
oped but has never been used for kino-dynamic sys-
tems (Favoreel et al. (1998); Woodley et al. (2001a)).
In the paper, previous implementations of biped
robots are mentioned in section 2. Model-free and
model based implementations are briefly discussed in
section 3. Subspace identification and its model-free
implementations are discussed in section 4. Proposed
implemetation is mentioned in section 5. Section 6
discusses the results of using subspace identification
technique in biped robot leg.
2 PREVIOUS
IMPLEMENTATIONS
An overview of literature suggests that history of
biped robots has only handful of milestones. Quasi-
dynamic walking gait on bipeds was achieved in 1980
by Kato et al. using artificial muscles (Kato et al.
(1983)). In 1983, Raibert demonstrated a planar one-
legged hopping robot that could hop at desired veloc-
ity and jump over small obstacles (Raibert (1986)).
In 1990, McGeer demonstrated first passive walking
for robots that could walk down a slop without any
active elements (McGeer (1990)). In 1997, Honda in-
troduced its biped robot P2 which set a new trend in
bipeds. Latest from Honda, ASIMO, has state-of-the-
art technology in this field (Sakagami et al. (2002);
Hirai et al. (1998); Lim and Takanishi (2005)). Con-
trol systems employed in the development of bipeds
can be divided into different categories.
Most of the robots fall into the category which
employs simple models, which can be calculated by
Newtonian mechanics, others are based on walking
and running dynamics (Kajita et al. (1992); Schwind
(1998)). These models are because of the inspira-
tion from biometrics (McMahon (1984); Alexander
(1996)). This technique is best used when trajectory
is given. It can be subdivided into further two types.
First one are the bipeds which are clone of ASIMO
and others are based on intuitive control. The best ef-
133
Saad Saleem M. and A. Sultan I. (2007).
FEASIBILITY OF SUBSPACE IDENTIFICATION FOR BIPEDS - An Innovative Approach for Kino-Dynamic Systems.
In Proceedings of the Fourth International Conference on Informatics in Control, Automation and Robotics, pages 133-140
DOI: 10.5220/0001646801330140
Copyright
c
SciTePress

fort in this technique has come from Pratt and Pratt,
and most impressive implementations in this type of
control framework also came from the same group
(Pratt and Pratt (1998, 1999); Pratt (2000)).
Other type of controllers are based on “neural” os-
cillators or pattern generators (Taga (1995)). There
are studies which suggest that vertebrates have some
kind of pattern generation mechanism which enables
them to walk dynamically. Generators can be hand-
tuned to construct a detailed feedback response for
dynamic walking. Last type of controllers are the
ones which are based on machine learning.
3 METHODS TO USE
EXPERIMENTAL DATA
To design a control system, equations for a biped
robot can be calculated from Newtonian mechanics.
It is shown in (Pratt (2000)) that equation of motion
of a massless leg with a torso having mass m, can be
written as:
ml
2
¨
θ
1
= mgl sinθ
1
− 2ml
˙
l
˙
θ
1
− J
¨
θ
b
(1)
here θ
1
is the angle between the normal axis to
ground and axis going through the CoP (center of
pressure) in foot and center of mass of the torso, J
is the rotational moment of inertia, and θ
b
is the angle
between torso axis and the leg. Equation 1 suggests
that there are three ways to change rotational dynam-
ics about center of pressure. First method is to change
the position of the body, which will change θ
1
and lo-
cation for center of pressure. This method is the most
effective one. Second method is to change the inertial
momentum J and third method is to change the length
l. Effect because of the last two quantities is not much
when compared with effect due to change in location
of center of pressure.
As we are more interested in exploring a more ro-
bust and generic solution for kino-dynamic systems,
techniques to use experimental data to determine sys-
tem equations will be discussed. There are four meth-
ods to use experimental data as shown in table 1
(Woodley (2001)). Mainly, choice depends on ap-
plication. For real-time systems which are easy to
model, indirect control is a better choice. The sys-
tem then adapts itself and updates its model param-
eters according to the conditions. Normally on-line
model based design is referred as indirect control. If
a system is hard to model from first principles (as
Newton’s laws of motion) or there are time varying
nonlinearities then direct adaptive control would suite
the application. Examples of plants which are diffi-
cult to model are arc furnaces (Wilson (1997); Staib
and Staib (1992)) and helicopter rotors (Lohar (2000);
Tischler et al. (1994)). Biped robots on the other hand
can be modeled but they exhibit time varying non-
linearities (Wolkotte (2003); Kim et al. (2004); Ca-
ballero et al. (2004)).
4 SYSTEM IDENTIFICATION
There are many system identification techniques. The
list starts with classical prediction error (PE) and
its variants; auto regression with exogenous input
(ARX), output error (OE), auto regression moving
average with exogenous input (ARMAX), and Box
Jenkins (BJ) (Norton (1986); Ljung (1999)).
4.1 Subspace Identification
Aside from classic system identification methods,
there are subspace identification methods, which
gained a lot of popularity in recent years (Morari and
Lee (1999)).
If plant’s input and output values at discrete times
are given by (Overschee and Moor (1996)):
u
0
u
1
.
.
.
u
n−1
,
y
0
y
1
.
.
.
y
n−1
Hankel matrices for past and future inputs are
written as
U
p
,
u
0
u
1
··· u
j−1
u
1
u
2
·· · u
j
.
.
.
.
.
. ·· ·
.
.
.
u
i−1
u
i
·· · u
i+ j−2
∈ R
im× j
U
f
,
u
i
u
i+1
·· · u
i+ j−1
u
i+1
u
i+2
·· · u
i+ j
.
.
.
.
.
. ·· ·
.
.
.
u
2i−1
u
2i
·· · u
2i+ j−2
∈ R
im× j
Similarly Hankel matrices for past and future out-
puts can be written as Y
p
∈ R
il× j
and Y
f
∈ R
il× j
re-
spectively. Let us define W
p
as
W
p
,
U
p
Y
p
Linear least squares predictor of Y
f
with given W
p
and U
f
can be written as Frobenius norm minimiza-
tion
min
L
w
,L
u
Y
f
−
L
w
L
u
W
p
U
f
2
F
ICINCO 2007 - International Conference on Informatics in Control, Automation and Robotics
134

Table 1: Four different techniques of control design from experimental data.
With Plant Model Without Plant Model
Online Indirect Adaptive Direct Adaptive
Offline Model Based Design Direct Control Design
From subspace orthogonal project, L
w
and L
u
is cal-
culated as
L
w
L
u
= Y
f
W
p
U
f
T
W
p
U
f
W
p
U
f
T
†
(2)
where † denotes pseudo-inverse. Future outputs can
be predicted from past inputs, outputs, and future in-
puts.
ˆy
k
.
.
.
ˆy
k+i−1
= L
w
u
k−i
.
.
.
u
k−1
y
k−i
.
.
.
y
k−1
+ L
u
u
k
.
.
.
u
k+i−1
(3)
Pseudo-inverse is normally calculated through
Singular Value Decomposition (SVD) but Woodley
et al. presented another way by using Cholesky fac-
torization, which is computationally faster and con-
sumes less memory (Woodley et al. (2001b)). It has
already been used for guidance and control of un-
manned vehicle (Kelbley (2006)).
4.2 Advantages of Subspace
Identification Methods
Subspace Identification Methods (SIM) have many
advantages over classical system identification tech-
niques (Overschee and Moor (1996)). Notables are:
• From plant’s input and output data, a predictor is
found same as Kalman filter states, which makes
it a simple least square problem. The whole archi-
tecture is streamlined and user-friendly.
• When implemented in direct adaptive control,
plant model is not needed to be simplified, which
can omit useful information from plant, as in SIM,
all the plant information is stored in a compact
form of subspace predictor.
• Output of subspace identification methods is in
state space form which makes it easy to imple-
ment in computer but it’s architecture has been
exploited in different model free implementations
as well (Woodley et al. (2001b); Favoreel et al.
(1999b,a)).
Wernholt used SIM to solved system identification
problem for ABB IRB 6600 robot (Wernholt (2004)).
Hsu et al. used N4SID in style translation for human
motion. These are some of the examples that how
SIMs are being used.
4.3 Reported Problems in Subspace
Identification Methods
There are a few problems in subspace identification
methods. Many of these problems have been dis-
cussed in recent literature and partial remedies have
been suggested (Chou and Verhaegen (1997); Lin
et al. (2004); Wang and Qin (2004); Chiuso and Picci
(2005)). Some of these problems are:
• Biased estimate for closed loop data.
• Errors-in-variables situation due to a projection
performed in the algorithm.
• Assumption of noise-free input.
It is expected that in direct adaptive system, which
calculates plant’s model and designs controller in re-
altime, this problem will not faced but final answer to
this can only be given after its implementation.
4.4 Types of Subspace Identification
Methods
There are many implementations of subspace identi-
fication methods. Notables are:
• Canonical variate analysis (CVA) (Larimore
(1990)).
• Multivariable output-error state space (MOESP)
(Verhaegen and Dewilde (1992)).
• Numerical algorithms for subspace state space
system identification (N4SID) (Overschee and
Moor (1994)).
• Eigensystem realization analysis (ERA) (Juang
(1994)).
• Subspace fitting (Jansson and Wahlberg (1996)).
• Stochastic subspace identification method using
principal component analysis (SIMPCA) (Wang
and Qin (2004)).
FEASIBILITY OF SUBSPACE IDENTIFICATION FOR BIPEDS - An Innovative Approach for Kino-Dynamic Systems
135

Inverse
kinematics
Controller Actuator and Drivers
Manipulator
Transducer
-
Figure 1: Joint space control.
Controller Actuator and Drivers
Manipulator
Transducer
-
Figure 2: Operational space control.
5 PROPOSED
IMPLEMENTATION
Joint space control is consisted of two subproblems.
First, manipulator inverse kinematics is performed
and then joint space control scheme is devised which
allows the end effector to follow the reference input.
The main computational burden in this scheme is be-
cause of inverse kinematics, which is normally per-
formed by using different optimization techniques,
as in a redundant system, there can be infinite solu-
tions for a given task (Lope et al. (2003); Gupta et al.
(1993); Kim et al. (2003)). Many implementations of
joint space control can be found in the literature (Laib
(2000); Kelly (1997); Arimoto (1995); Kelly (1993);
Wen et al. (1992); Tomei (1991); Takegaki and Ari-
moto (1981); Zhang et al. (2000)).
In many applications, desired path of end effector
is specified in operational space. Operational space
control, on the other hand, is used for constrained
manipulator motions (Sciavicco and Siciliano (2000);
Sapio and Khatib (2005)). These constraints can be
because of gravity or kinematically imposed. It can be
seen in figure 2 that inverse kinematics is embedded
in the closed-loop control law but not explicitly per-
formed as shown in figure 1 (Sciavicco and Siciliano
(2000)). Operational space control and task space
control sometimes allude to the same concept (Khalil
and Dombre (2004); Xie (2003); Sciavicco and Sicil-
iano (2000)). Sapio and Khatib has simulated oper-
ational control schemes in physiological model of a
human body under constrained conditions (Sapio and
Khatib (2005)).
6 EXPERIMENT
MATLAB
R
and Simulink
R
by MathWorks Inc. have
been employed to simulate a bipedal leg with torso.
Under the action of normal gravity and exogenous
Foot
Ankle
A
B
C
Figure 3: Foot ground interaction. On the left is the side
view and on the right is the top view of foot model where
points A, B, and C are connected to three dampers and
springs. Dampers and springs connected on sides are re-
sponsible for friction with the ground.
force signals at each joint, the leg falls down and tra-
jectory of torso is recorded. Using Subspace Identi-
fication, a predictor is found. This predictor is then
applied on input joint signals. First, predicted trajec-
tories are presented and then trajectories are predicted
by updating previous trajectory from actual outputs
after every prediction.
Following algorithm gives error between actual
and predicted trajectories:
1. Prediction horizon, i is chosen and experiment is
performed with given input and resultant torso tra-
jectory is noted
2. From noted trajectory, a predictor is calculated us-
ing subspace projection algorithm
3. Outputs are calculated using subspace predictor
and given inputs at joints
4. Difference between calculated values and actual
values are plotted for each axis
5. Prediction horizon is changed and the whole pro-
cess is repeated
One of the challenges in simulations was to sim-
ulate foot-ground interaction. Many implementations
can be found in the literature (Hsu et al. (2005); Ogi-
hara and Yamazaki (2001); Wang (2005); Wolkotte
(2003)). Model with three contact points was devised
after inspiration from human foot. This is shown in
figure 3.
6.1 Assumptions
It is assumed that there are only three points where
foot can touch the ground as shown in figure 3 and
there is no air friction.
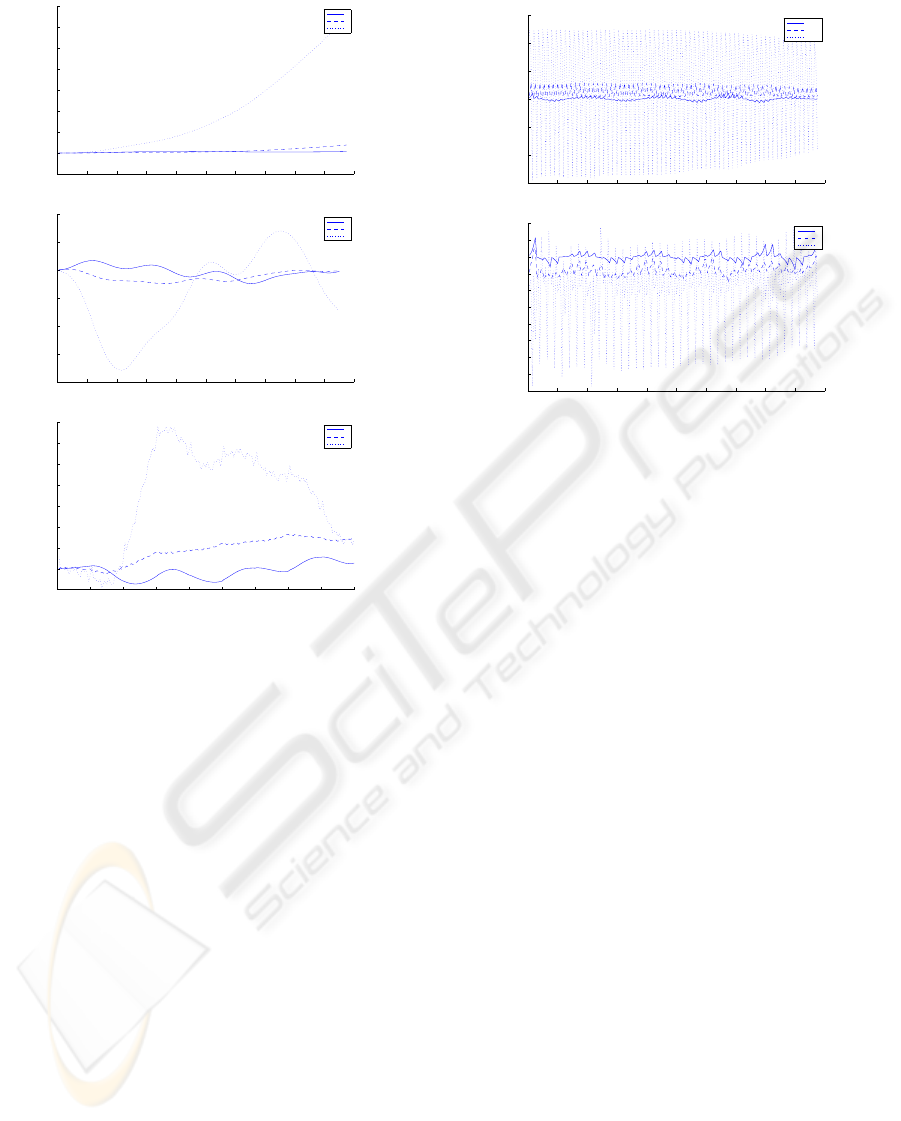
6.2 Results
For prediction horizon i less than a certain value,
the system simply fails to predict the future outputs.
Some suggest that the value of i should be 2 to 3 times
ICINCO 2007 - International Conference on Informatics in Control, Automation and Robotics
136

Table 2: Supposed values of different parameters for simulation.
Length or radius [m] Width [m] Height [m] Mass [kg]
Torso 0.1 0.4 0.5 20
Thigh 0.05 0.4 10
Calf 0.05 0.4 5
Foot 0.3 0.07 0.3 2
Shape I
1
[kg m
2
] I
2
[kg m
2
] I
3
[kg m
2
]
Torso Parallelepiped 0.688 0.4333 0.2833
Thigh Cylinder 0.2333 0.2333 0.05
Calf Cylinder 0.0698 0.0698 0.0063
Foot Parallelepiped 9.6667e-4 0.0151 0.0158
the expected order of the system for stable and accu-
rate results (Woodley (2001)), however, there is no
hard and fast rule. In our experiments, the predic-
tion horizon more than 10 did not improve the ac-
curacy of the prediction. Increasing the value of i
can also be computationally expensive as even with
Cholesky/SVD factorization technique, the complex-
ity of finding a subspace predictor is O(ij+i
3
), where
j is number of prediction problems (Golub and Loan
(1996)). It can be seen in the simulation and graphs
that for movements of more than 1 meter, the error is
in the order of micrometers. These results are very
encouraging especially when there are multiple rigid
bodies which are coupled together with rotatory joints
and ground-foot interaction is present with given fric-
tion.
7 FUTURE WORK
To find subspace predictor, Hanekel matrix structure
can be exploited for a better real-time operation. This
work can be extended to a complete implementation
of a model-free control system such as the one sug-
gested by Woodley et al.. One of the challenges in
the actual implementation is determination of uncer-
tainty block ∆ for the given system using techniques
such as model unfalsification but without excessive
overload of high computations (Woodley et al. (1998),
Paul B. Brugarolas (2004)).
REFERENCES
Alexander, R. M. (1996). Optima for Animals. Princeton
University Press, revised edition.
Arimoto, S. (1995). Fundamental problems of robot con-
trol: Parti, innovations in the realm of robot servo-loops.
Robotica, 13:19–27.
Caballero, R., Armada, M. A., and Akinfiev, T. (2004).
Robust cascade controller for nonlinearly actuated biped
robots: experimental evaluation. International Journal
of Robotics Research, 23(10/11):1075–1095.
Chiuso, A. and Picci, G. (2005). Consistency analysis of
some closed-loop subspace identification methods. Au-
tomatica, 41(3):377–391.
Chou, C. T. and Verhaegen, M. (1997). Subspace algo-
rithms for the identification of multivariable dynamic
errors-in-variables models. Automatica, 33:1857–1869.
Favoreel, W., Moor, B. D., Gevers, M., and Overschee, P. V.
(1998). Model-free subspace-based LQG-design. Tech-
nical report, Katholieke Universiteit Leuven.
Favoreel, W., Moor, B. D., Gevers, M., and Overschee, P. V.
(1999a). Closed loop model-free subspace-based LQG-
design. In Proceedings of the IEEE Mediterranean Con-
ference on Control and Automation, Haifa, Israel.
Favoreel, W., Moor, B. D., and Overschee, P. V. (1999b).
Model-free subspace-based LQG-design. In Proceedings
of the American Control Conference, pages 3372–3376.
Golub, G. H. and Loan, C. F. V. (1996). Matrix Computa-
tions. The Johns Hopkins University Press.
Gupta, M. M., Rao, D. H., and Nikiforuk, P. N. (1993). Dy-
namic neural network based inverse kinematics transfor-
mation of two- and three-linked robots. In 12th World
Congress, International Federation of Automatic Con-
trol, Sydney, Australia, pages 289–296.
Hirai, K., Hirose, M., Haikawa, Y., and Takenaka, T. (1998).
The development of honda humanoid robot. In Proceed-
ings of the IEEE International Conference on Robotics
and Automation (ICRA), pages 1321–1326.
Hsu, E., Pulli, K., and Popovi
´
c, J. (2005). Style transla-
tion for human motion. ACM Transactions on Graphics
(TOG), 24(3):1082–1089.
Jansson, M. and Wahlberg, B. (1996). A linear regression
approach to state-space subspace system identification.
Signal Processing, 52:103–129.
Juang, J. N. (1994). Applied System Identification. PTR
Prentice-Hall.
Kajita, S., Yamaura, T., and Kobayashi, A. (1992). Dynamic
walking control of a biped robot along a potential energy
conserving orbit. IEEE Transactions on Robotics and
Automation, 6(1):431–438.
FEASIBILITY OF SUBSPACE IDENTIFICATION FOR BIPEDS - An Innovative Approach for Kino-Dynamic Systems
137

Kato, T., Takanishi, A., Jishikawa, H., and Kato, I. (1983).
The realization of the quasi-dynamic walking by the
biped walking machine. In Morecki, A., Bianchi, G.,
and Kedzior, K., editors, Fourth Symposium on Theory
and Practice of Walking Robots, pages 341–351. Polish
Scientific Publishers.
Kelbley, R. J. (2006). Guidance and control of an unmanned
surface vehicle. Master’s thesis, Computer Engineering,
University of California, Santa Cruz.
Kelly, R. (1993). Comments on adaptive pd controller
for robot manipulators. IEEE Trans. Robot. Automat.,
9:117–119.
Kelly, R. (1997). Pd control with desired gravity compen-
sation of robotic manipulators: A review. Int. J. Robot.
Res., 16(5):660–672.
Khalil, W. and Dombre, E. (2004). Modeling, Identification
and Control of Robots. Kogan Page Science.
Kim, D., Kim, N.-H., Seo, S.-J., and Park, G.-T. (2004).
Fuzzy Modeling of Zero Moment Point Trajectory for a
Biped Walking Robot. Lecture Notes in Computer Sci-
ence. Springer-Verlag GmbH, 3214 edition.
Kim, J. O., Lee, B. R., Chung, C. H., Hwang, J., and Lee,
W. (2003). The Inductive Inverse Kinematics Algorithm
to Manipulate the Posture of an Articulated Body. Lec-
ture Notes in Computer Science. Springer-Verlag GmbH,
2657 edition.
Laib, A. (2000). Adaptive output regulation of robot manip-
ulators under actuator constraints. IEEE Trans. Robot.
Automat., 16:29–35.
Larimore, W. E. (1990). Canonical variate analysis in iden-
tification, filtering and adaptive control. In IEEE Confer-
ence on Decision and Control, pages 596–604.
Lim, H. and Takanishi, A. (2005). Compensatory motion
control for a biped walking robot. Robotica, 23(01):1–
11.
Lin, W., Qin, S. J., and Ljung, L. (2004). A frame-
work for closed-loop subspace identification with inno-
vation estimation. Technical Report 2004-07, Depart-
ment of Chemical Engineering, The University of Texas
at Austin, Austin, TX 78712, USA and Link
¨
oping Uni-
versity, SE-581 83 Link
¨
oping, Sweden.
Ljung, L. (1999). System identification: theory for the user.
Prentice-Hall, Upper Saddle River, NJ, USA.
Lohar, F. A. (2000). H
∞
and µ-synthesis for full control of
helicopter in hover. In 38th Aerospace Sciences Meeting
and Exhibit, Reno, NV. American Institute of Aeronau-
tics and Astronautics.
Lope, J. d., Zarraonandia, T., Gonz
´
alez-Careaga, R., and
Maravall, D. (2003). Solving the inverse kinematics in
humanoid robots: A neural approach. Lecture Notes in
Computer Science, 2687 / 2003:177–184.
McGeer, T. (1990). Passive dynamic walking. The Inter-
natinal Journal of Robotics Research, 9(2):62–82.
McMahon, T. (1984). Mechanics of locomotion. The Inter-
national Journal of Robotics Research, 3(2):4–28.
Morari, M. and Lee, J. H. (1999). Model predictive con-
trol: Past, present and future. Computers and Chemical
Engineering, 23:667–682.
Norton, J. P. (1986). Introduction to Identification. Aca-
demic Press.
Ogihara, N. and Yamazaki, N. (2001). Generation of human
bipedal locomotion by a bio-mimetic neuro-musculo-
skeletal model. Biological Cybernetics, 84:1.
Overschee, P. V. and Moor, B. D. (1994). N4SID:
Subspace algorithms for the identification of combined
deterministic-stochastic systems. Automatica, 30(1):75–
93.
Overschee, P. V. and Moor, B. D. (1996). Subspace Identi-
ficiation for Linear Systems. Kluwer Academic Publish-
ers.
Paul B. Brugarolas, M. G. S. (2004). Learning about dy-
namical systems via unfalsification of hypotheses. In-
ternational Journal of Robust and Nonlinear Control,
14(11):933–943.
Pratt, J. and Pratt, G. (1998). Intuitive control of a pla-
nar bipedal walking robot. In Proceedings of the IEEE
International Conference on Robotics and Automation
(ICRA).
Pratt, J. and Pratt, G. (1999). Exploiting natural dynamics
in the control of a 3D bipedal walking simulation. In
Proceedings of the International Conference on Climb-
ing and Walking Robots (CLAWAR).
Pratt, J. E. (2000). Exploiting Inherent Robustness and Nat-
ural Dynamics in the Control of Bipedal Walking Robots.
PhD thesis, Department of Electrical Engineering and
Computer Science, Massachusetts Institute of Technol-
ogy.
Raibert, M. (1986). Legged Robots That Balance. The MIT
Press.
Sakagami, Y., Watanabe, R., Aoyama, C., Matsunaga, S.,
Higaki, N., and Fujimura, K. (2002). The intelligent
ASIMO: system overview and integration. In IEEE/RSJ
International Conference on Intelligent Robots and Sys-
tem, volume 3, pages 2478–2483.
Sapio, V. D. and Khatib, O. (2005). Operational space con-
trol of multibody systems with explicit holonomic con-
straints. In Proceedings of the 2005 IEEE International
Conference on Robotics and Automation.
Schwind, W. J. (1998). Spring Loaded Inverted Pendu-
lum Running: A Plant Model. PhD thesis, University
of Michigan.
Sciavicco, L. and Siciliano, B. (2000). Modelling and Con-
trol of Robot Manipulators. Springer, 2nd edition.
Staib, W. E. and Staib, R. R. (1992). A neural network
electrode positioning optimization system for the electric
arc furnace. In International Joint Conference on Neural
Networks, volume 111, pages 1–9.
Taga, G. (1995). A model of the neuro-musculo-skeletal
system for human locomotion. I. Emergence of basic
gait. Biological Cybernetics, 73(2):97–111.
Takegaki, M. and Arimoto, S. (1981). A new feedback
method for dynamic control of manipulators. ASME J.
Dyn. Syst., Meas., Control, 102:119–125.
Tischler, M. B., Driscoll, J. T., Cauffman, M. G., and Freed-
man, C. J. (1994). Study of bearingless main rotor dy-
namics from frequency-response wind tunnel test data.
ICINCO 2007 - International Conference on Informatics in Control, Automation and Robotics
138

In American Helicopter Society Aeromechanics Special-
ists Conference.
Tomei, P. (1991). Adaptive pd controller for robot manipu-
lators. IEEE Trans. Robot. Automat., 7:565–570.
Verhaegen, M. and Dewilde, P. (1992). Subspace model
identification. part i: the output-error state-space model
identification class of algorithms. International Journal
of Control, 56:1187–1210.
Wang, J. and Qin, S. J. (2004). A new deterministic-
stochastic subspace identification method using princi-
pal component analysis. Technical report, Department
of Chemical Engineering, The University of Texas at
Austin.
Wang, J. M.-C. (2005). Gaussian process dynamical models
for human motion. Master’s thesis, Graduate Department
of Computer Science, University of Toronto.
Wen, J., Kreutz-Delgado, K., and Bayard, D. (1992). Lya-
punov function-based control laws for revolute robot
arms. IEEE Trans. Automat. Contr., 37:231–237.
Wernholt, E. (2004). On Multivariable and Nonlinear Iden-
tification of Industrial Robots. PhD thesis, Department of
Electrical Engineering, Link
¨
oping University, SE-581 83
Link
¨
oping, Sweden.
Wilson, E. (1997). Adaptive profile optimization for the
electric arc furnace. In Steel Technology International,
pages 140–145.
Wolkotte, P. T. (2003). Modelling human locomotion. Tech-
nical report, Institute of Electronic Systems, Aalborg
University.
Woodley, B., How, J., and Kosut, R. (2001a). Model free
subspace based H
∞
control. In Proceedings of the 2001
American Control Conference, volume 4, pages 2712–
2717.
Woodley, B., Kosut, R., and How, J. (1998). Uncertainty
model unfalsification with simulation. In Proceedings of
the 1998 American Control Conference, volume 5, pages
2754–2755.
Woodley, B. R. (2001). Model free subspace based H
∞
con-
trol. PhD thesis, Department of Electrical Engineering,
Stanford University.
Woodley, B. R., How, J. P., and Kosut, R. L. (2001b).
Subspace based direct adaptive H
∞
control. Interna-
tional Journal of Adaptive Control and Signal Process-
ing, 15(5):535–561.
Xie, M. (2003). Fundamentals of Robotics, volume 54 of
Machine perception and artificial intelligence. World
Scientific.
Zhang, Y., Tian, H., Wang, Q., and Qiang, W. (2000). Servo
control in joint space of biped robot using nonlinear H
∞
strategy. In Jiang, D. and Wang, A., editors, Proceedings
of SPIE, International Conference on Sensors and Con-
trol Techniques (ICSC 2000), volume 4077, pages 386–
391.
Figure 4: Free fall of a biped leg with exogenous force sig-
nals at its joints. Top six and bottoms ones are shots taken
from the same simulation but from different angles after ev-
ery 0.1 seconds.
FEASIBILITY OF SUBSPACE IDENTIFICATION FOR BIPEDS - An Innovative Approach for Kino-Dynamic Systems
139
0 20 40 60 80 100 120 140 160 180 200
−0.005
0
0.005
0.01
0.015
0.02
0.025
0.03
0.035
sampling time
differences between actual and calculated values (meters)
x
y
z
0 20 40 60 80 100 120 140 160 180 200
−4
−3
−2
−1
0
1
2
x 10
−4
sampling time
differences between actual and calculated values (meters)
x
y
z
0 20 40 60 80 100 120 140 160 180
−2
0
2
4
6
8
10
12
14
x 10
−4
sampling time
differences between actual and calculated values (meters)
x
y
z
Figure 5: Error in the calculation of torso position. Above
graphs are with i = 5, i = 10, and i = 20 respectively. Note
that the largest movement of torso is in the z-direction, the
error is also the most in this direction.
0 20 40 60 80 100 120 140 160 180 200
−6
−4
−2
0
2
4
6
x 10
−5
sampling time
differences of angles between actual and calculated in radians
hip
knee
ankle
0 20 40 60 80 100 120 140 160 180 200
−16
−14
−12
−10
−8
−6
−4
−2
0
2
4
x 10
−6
sampling time
differences between actual and calculated values (meters)
xyz
Figure 6: Error in the calculation of torso position when
data is updated from actual torso position after every predic-
tion. Above graphs are with i = 5 and i = 20 respectively.
Note that even for very small prediction horizon i.e. i = 5,
the error is very small.
ICINCO 2007 - International Conference on Informatics in Control, Automation and Robotics
140
