
MODELING NON-GAUSSIAN NOISE FOR ROBUST IMAGE
ANALYSIS
Sio-Song Ieng
ERA 17 LCPC, Laboratoire des Ponts et Chauss
´
ees, 23 avenue de l’amiral Chauvin, B.P. 69, 49136 Les Ponts de C
´
e, France
Jean-Philippe Tarel
ESE, Laboratoire Central des Ponts et Chauss
´
ees, 58 Bd Lefebvre, 75015 Paris, France
Pierre Charbonnier
ERA 27 LCPC, Laboratoire des Ponts et Chauss
´
ees, 11 rue Jean Mentelin, B.P. 9, 67035 Strasbourg, France
Keywords:
Image Analysis, Statistical Approach, Noise Modeling, Robust Fitting, Image Grouping and Segmentation,
Image Enhancement.
Abstract:
Accurate noise models are important to perform reliable robust image analysis. Indeed, many vision prob-
lems can be seen as parameter estimation problems. In this paper, two noise models are presented and we
show that these models are convenient to approximate observation noise in different contexts related to image
analysis. In spite of the numerous results on M-estimators, their robustness is not always clearly addressed
in the image analysis field. Based on Mizera and M
¨
uller’s recent fundamental work, we study the robustness
of M-estimators for the two presented noise models, in the fixed design setting. To illustrate the interest of
these noise models, we present two image vision applications that can be solved within this framework: curves
fitting and edge-preserving image smoothing.
1 INTRODUCTION
In computer vision, it is common knowledge that
data are corrupted by non-Gaussian noise, outliers
and may contain multiple statistical populations. It
is a difficult task to model observed perturbations.
Several parametric models were proposed in (Huang
and Mumford, 1999; Srivastava et al., 2003), and
sometimes based on mixtures (Hasler et al., 2003).
Non-Gaussian noise models imply using robust al-
gorithms to reject outliers. The most popular tech-
niques are Least Median Squares (LMedS), RANSAC
and Iterative Reweighted Least Squares (IRLS). The
first two algorithms are close in their principle and
achieve the highest breakdown point, i.e. the admis-
sible fraction of outliers in the data set, of approx-
imately 50%. However, their computational burden
quickly increases with the number of parameters. In
this paper, we focus on the IRLS algorithm, which is
an extension of least-squares allowing non-Gaussian
noise models, see (Huber, 1981). IRLS means Iter-
ative Reweighted Least-Squares, where the weight λ
is a particular function of the noise model at the value
of the residual. One may argue that IRLS algorithm is
a deterministic algorithm that only converges towards
a local minimum close to its starting point. This dif-
ficulty can be circumvented by the so-called Gradu-
ated Non Convexity (GNC) strategy. The IRLS algo-
rithm, even with the GNC strategy, is usually very fast
compared to LMedS and RANSAC and it also able to
achieve the highest breakdown point.
Indeed, let us consider the linear problem:
Y = XA+ B (1)
where Y = (y
1
,···,y
n
) ∈ R
n
is a vector of observa-
tions, X = (x
1
,···,x
n
) ∈ R
n×d
the design matrix, A ∈
R
d
the vector of unknown parameters that will be esti-
mated by the IRLS algorithm, and B = (b
1
,···,b
n
) ∈
R
n
the noise. The noise is assumed independent and
identically distributed but not necessarily Gaussian.
We consider the fixed design setting, i.e. in (1), X is
assumed non-random. In that case, as demonstrated
in (Mizera and M
¨
uller, 1999), certain M-estimators
attain the maximum possible breakdown point. How-
ever, if X cannot be assumed non-random, the break-
down point of M-estimators drops towards zero (Meer
et al., 2000). This underlines how important the way
computer vision problems are formulated is. To our
183
Ieng S., Tarel J. and Charbonnier P. (2007).
MODELING NON-GAUSSIAN NOISE FOR ROBUST IMAGE ANALYSIS.
In Proceedings of the Second International Conference on Computer Vision Theory and Applications - IFP/IA, pages 183-190
Copyright
c
SciTePress
opinion, many problems can be expressed in the fixed
design setting, allowing to apply the fast and efficient
IRLS algorithm.
The paper is organized as follows. In Sec. 2,
we present the non-Gaussian noise models we found
of interest in diverse contexts, which is shown in
Sec. 3. Then in Sec. 4, we prove that M-estimators
that achieve the maximum breakdown point of ap-
proximately 50% can be built based on these proba-
bility distribution families. Finally in Sec. 5, we illus-
trate the interest of these theoretical results, by pre-
senting two applications, casted in the fixed design
setting: curves fitting for lane-marking shape estima-
tion and edge-preserving image smoothing.
2 NON-GAUSSIAN NOISE
MODELS
We are interested in parametric functions families that
allow a continuous transition between different kinds
of probability distributions. We here focus on two
simple parametric probability density functions (pdf)
of the form pd f (b) ∝ e
−ρ(b)
suitable for the IRLS al-
gorithm, where ∝ denotes the equality up to a factor.
A first interesting family of pdfs is the exponential
family (also called generalized Laplacian, or gener-
alized Gaussian, or stretched exponential (Srivastava
et al., 2003)):
E
α,s
(b) =
α
sΓ(
1
2α
)
e
−((
b
s
)
2
)
α
(2)
The two parameters of this family are the scale s and
the power α, which specifies the shape of the noise
model. Moreover, α allows a continuous transition
between two well-known statistics: Gaussian (α = 1)
and Laplacian (α =
1
2
). The associated ρ function is
ρ
E
α
(b) = ((
b
s
)
2
)
α
.
As detailed in (Tarel et al., 2002), to guarantee
the convergence of IRLS,
ρ
′
(b)
b
has to be defined on
[0,+∞[, which is not the case for α ≤ 0 in the expo-
nential family. Therefore, the so-called smooth ex-
ponential family (SEF) S
α,s
was introduced in (Ieng
et al., 2004):
S
α,s
(b) ∝
1
s
e
−
1
2
ρ
α
(
b
s
)
(3)
where ρ
α
(u) =
1
α
((1+ u
2
)
α
−1).
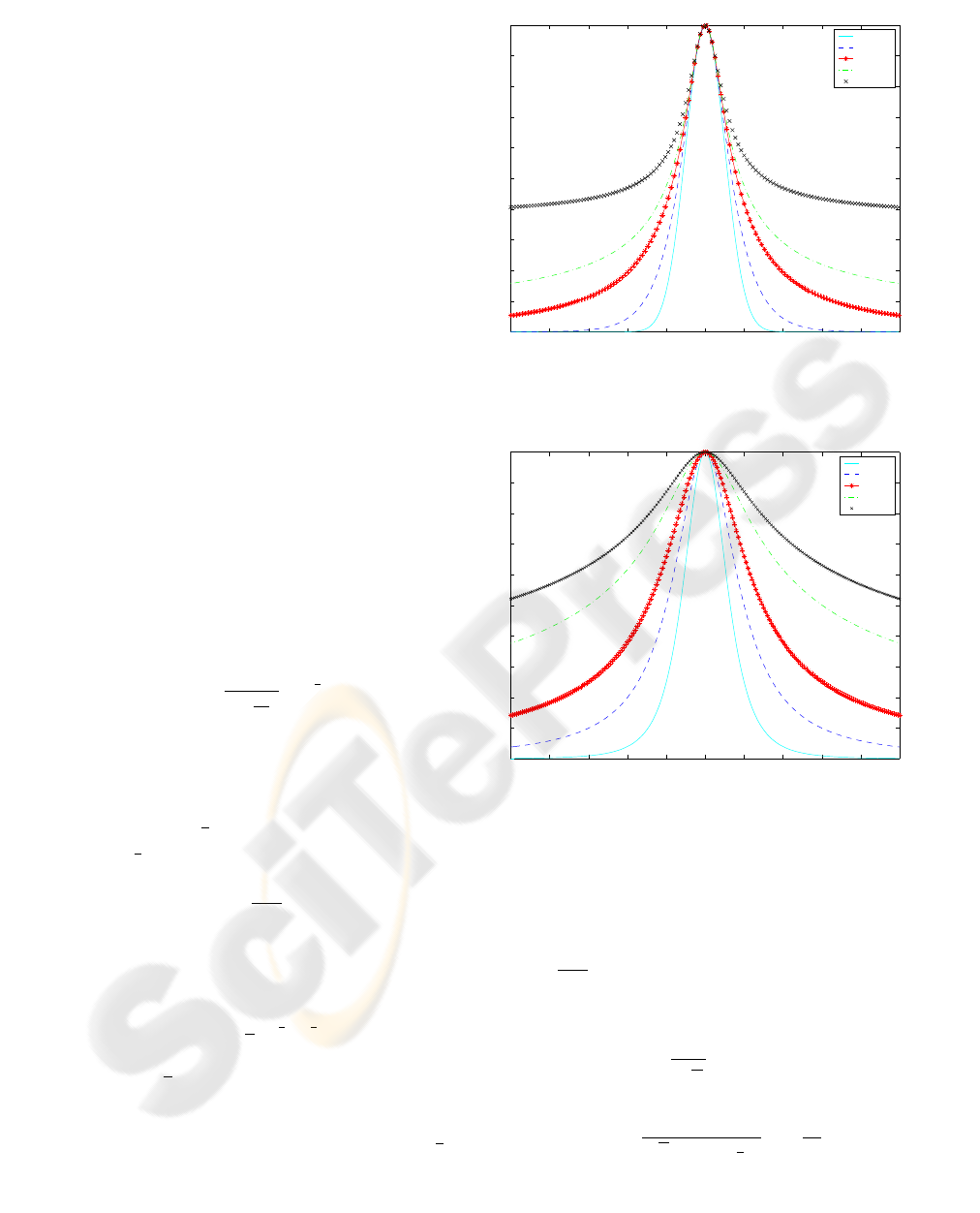
Similarly to the exponential family, α allows a
continuous transition between well-known statistical
laws such as Gauss (α = 1), smooth Laplace (α =
1
2
)
and Geman & McClure (α = −1). These laws are
shown in Figure 1. For α ≤0, S
α,s
can be always nor-
malized on a bounded support, so it can still be seen
−10 −8 −6 −4 −2 0 2 4 6 8 10
0
0.1
0.2
0.3
0.4
0.5
0.6
0.7
0.8
0.9
1
S
α,s
distribution
α = 1
α = 0.5
α = 0.1
α = −0.1
α = −0.5
Figure 1: SEF noise models, S
α,s
. Notice how tails are
getting heavier as α decreases.
−10 −8 −6 −4 −2 0 2 4 6 8 10
0
0.1
0.2
0.3
0.4
0.5
0.6
0.7
0.8
0.9
1
β = 2
β = 1
β = 0.6
β = 0.3
β = 0.2
Generalized T−Student Distributions
Figure 2: GTF noise models, T
β,s
. Notice how tails are
getting heavier when β is increasing towards 0.
as a pdf. In the smooth exponential family, when α
is decreasing, the probability to have large, not to say
very large errors (outliers) increases.
In the IRLS algorithm, the residual b is weighted
by λ =
ρ
′
(b)
b
. Notice that while the pdf is not defined
when α = 0, its weight does and it corresponds in fact
to the T-Student law. Moreover, it is easy to show
that the so-called generalized T-Student pdfs have the
same weight function
1
1+
b2
s2
up to a factor. We define
the Generalized T-Student Family (GTF) by:
T
β,s
(b) =
Γ(−β)
√
πΓ(−β−
1
2
)s
(1+
b
2
s
2
)
β
(4)
where β < 0. This family of pdfs also satisfies the re-
quired properties for robust fitting. It is named gener-

alized T-Student pdf (Huang and Mumford, 1999) in
the sense that an additional scale parameter is intro-
duced compared to the standard T-Student pdf. No-
tice, that the case β = −1 corresponds to the Cauchy
pdf. These laws are shown in Figure 2. The GTF can
be rewritten as:
T
β,s
(b) ∝
1
s
e
−
1
2
ρ
β
(b)
(5)
where ρ
β
(b) = −2βlog(1+
b
2
s
2
).
The parameters of the GTF are s and β (β < 0).
They play exactly the same role as s and α in the SEF.
For −
1
2
≤β < 0, as previously the pdf is defined only
for a bounded support.
3 IMAGE NOISE MODELING
We have used with success SEF and GTF for noise
modeling in different image analysis applications. We
now illustrate this on two particular examples, where
geometric and photometric perturbations are consid-
ered in turn, and modeled using the smooth exponen-
tial family (SEF).
3.1 Geometry
In this experiment, we have taken a set of 150 im-
age of the same marking with different perturbations
such as stones, shadows and so on, see Figure 3. The
ground-truth position of the lane marking center was
obtained by hand. It is shown in black in Figure 3.
Then, for each of these images, local marking cen-
ters are extracted, see (Ieng et al., 2004) for details,
and the horizontal distances to each feature center
with respect to the reference are collected. The MLE
approach is then applied to estimate α and s at the
same time by a non-linear minimization with a gra-
dient descent. The best parameters within SEF are
α = 0.05 and s = 1.1. As shown in Figure 4, these
parameters seems to lead to a nice noise model.
3.2 Photometry
We performed a similar experiment for photometric
information. We have taken a set of 19 images of the
same scene with different light conditions as shown
on Fig 5. The ground truth is not easy to build con-
trary to the previous section. As a consequence, we
compute, for each pair of images, the histogram of
the differences in intensity between the two images,
for each pixel. Rather than to sample the pdf of the
perturbations, we sample the autocorrelation function
of the pdf.
Figure 3: Three of the 150 images of the same marking
with different perturbations. The black straight line shows
the reference marking position.
After fitting of the SEF model, we obtained a
quite accurate model of the autocorrelation function
as shown in Fig 6, with α = 0.02 and s = 9.
4 ROBUSTNESS STUDY
Following (Mizera and M
¨
uller, 1999), in the fixed de-
sign case, robustness of an M-estimator is character-
ized by its breakdown point which is defined as the
maximum percentage of outliers the estimator is able
to cope with:
ε
∗
(
ˆ
A,Y,X) =
1
n
min{m : sup
˜
Y∈B(Y,m)
k
ˆ
A(
˜
Y,X)k= ∞}
(6)
where
˜
Y is a corrupted data set obtained by arbitrary
changing at most m samples, B is the set of all
˜
Y:

−200 −150 −100 −50 0 50 100 150 200
−10
−9
−8
−7
−6
−5
−4
−3
−2
Log histogram fit
residual
log(probability)
Figure 4: Log histogram of the residual errors in feature
centers collected from 150 images after local marking ex-
traction. The obtained distribution is well approximated by
the best fit SEF (3) with parameters α = 0.05 and s = 1.1.
B(Y,m) = {
˜
Y : card{k : ˜y
k
6= y
k
} ≤ m} and
ˆ
A(
˜
Y,X)
is an estimate of A from
˜
Y. It is important to notice
that the previous definition is different from the one
proposed in (Hampel et al., 1986) which is not suited
to the fixed design setting.
Mizera and M
¨
uller (Mizera and M
¨
uller, 1999) also
emphasize the notion of regularly varying functions,
and described the link between this kind of regular-
ity and robustness property. By definition, f varies
regularly if there exists a r such that:
lim
t→∞
f(tb)
f(t)
= b
r
(7)
When the exponent r equals zero, the function is said
to vary slowly, i.e. the function is heavily tailed.
We now assume that the ρ function of the M-
estimator follows the four following conditions:
1. ρ is even, non decreasing on R
+
and nonnegative,
2. ρ is unbounded,
3. ρ varies regularly with an exponent r ≥0,
4. ρ is sub-additive: ∃L > 0, ∀t,s ≥ 0, ρ(s + t) ≤
ρ(s) + ρ(t) + L.
The main result proved in (Mizera and M
¨
uller,
1999) is that the percentage ε
∗
is bounded by a func-
tion of r:
Theorem. Under the four previous conditions on ρ,
and if r ∈ [0, 1], then ∀Y and X,
M(X,r)
n
≤ ε
∗
(
ˆ
A,Y,X) ≤
M(X,r) + 1
n
(8)
where, with the convention 0
0
= 0, M(X,r) is defined
as:
M(X,r) = min
A6=0
{card(K) :
∑
k∈K
|X
t
k
A|
r
≥
∑
k/∈K
|X
t
k
A|
r
}
(9)
Figure 5: Three of the 19 images of the same scene with
different lighting conditions.
where K runs over the subsets of {1,2,···,n}.
When the exponent r is zero, the exact value of the
percentage ε
∗
is known. The following theorem states
its value.
Theorem. Under the four previous conditions on ρ
and if r = 0, then ∀ Y and X,
ε
∗
(
ˆ
A,Y,X) =
M(X,0)
n
=
1
n
⌊
n−
N (X) + 1
2
⌋ (10)
where ⌊x⌋ represents the integer part of x, and
N (X) = max
A6=0
{card{X
k
: X
t
k
A = 0}, k = 1,···,n}.
This value is also the maximum achievable value
which is approximatively 50%. As a consequence, M-
estimators with zero r exponent are of highest break-
down point.
Finally in (Mizera and M
¨
uller, 1999), it is shown
that the bounds on the percentage ε
∗
are related to r as

−300 −200 −100 0 100 200 300
0
0.005
0.01
0.015
0.02
0.025
0.03
0.035
0.04
Intensity
pdf
data
fit
Figure 6: Histogram of the intensity differences collected
from 19 images. The obtained distribution is well approxi-
mated by the best fit SEF (3) with parameters α = 0.02 and
s = 9.
a decreasing function. This is stated by the following
lemma:
Lemma. If q ≥ r ≥ 0 then
M(X,q) ≤ M(X,r) ≤ M(X,0) = ⌊
n−
N (X )+ 1
2
⌋.
With these three results, the relationship between
the exponent r of the function ρ and the robustness
of the associated M-estimator was clearly established.
As a consequence of these important theoretical re-
sults, the robustness of a large class of M-estimators
can be compared just by looking at the exponent r of
the cologarithm of their associated noise pdf. To illus-
trate this, we now apply the above results to the SEF
and GTF pdfs described in Sec. 2.
4.1 The SEF Case
Let us prove that the robustness of SEF based M-
estimators is decreasing with α ∈]0,0.5]. To this end,
we first check the four above conditions on the ρ
α
function. Function ρ
α
is clearly even, non decreasing
on R
+
and nonnegative. The first condition is thus
satisfied. The second one is fulfilled only when α > 0,
due to the fact that ρ
α
is bounded for α ≤0. Looking
at the ratio:
ρ
α
(tb)
ρ
α
(t)
=
(1+
t
2
b
2
s
2
)
α
−1
(1+
t
2
s
2
)
α
−1
=
(
1
t
2
+
b
2
s
2
)
α
−
1
t
2α
(
1
t
2
+
1
s
2
)
α
−
1
t
2α
we see that when α > 0, lim
t→∞
ρ
α
(tb)
ρ
α
(t)
= b
2α
. As
a consequence, the ρ
α
function varies regularly and
the third condition is also satisfied. For the fourth
condition, we can use Huber’s Lemma 4.2 (Huber,
1984), to prove the sub-additivity when α ∈]0,0.5[.
For α = 0.5, it can also be proved that ρ
α
is sub-
additive. All conditions on ρ being fulfilled, the first
Theorem applies, and using the Lemma, we prove that
the robustness of SEF M-estimators is increasing to-
wards the maximum of approximatively 50%, with re-
spect to a decreasing α parameter within ]0,0.5].
4.2 The GTF Case
Let us prove that the robustness of GTF M-estimators
is maximum, whatever the value of β. We shall first
check the 4 conditions on the associated ρ function.
The first two assumptions are easy to check for ρ
β
.
Looking at the ratio:
ρ
β
(tb)
ρ
β
(t)
=
ln(t
2
) + ln(
1
t
2
+
b
2
s
2
))
ln(t
2
) + ln(
1
t
2
+
1
s
2
))
we deduce: lim
t→∞
ρ
β
(tb)
ρ
β
(t)
= 1 = b
0
. As a conse-
quence, the ρ
β
function varies slowly, and the third
condition is fulfilled. The fourth condition is proved
by using Huber’s Lemma 4.2 (Huber, 1984). All
conditions on ρ being satisfied, the second Theorem
applies, showing that GTF M-estimators achieve the
highest breakdown point of approximately 50%.
5 APPLICATIONS
We now describe two applications showing how in-
teresting the use of SEF or GTF is in applying robust
algorithms to problems related to geometry and pho-
tometry.
5.1 Curve Fitting for Lane-marking
Tracking
This application is detailed in (Tarel et al., 2002; Ieng
et al., 2004). The problem of tracking a lane mark-
ing can be handled using Kalman filtering, if the lane
marking is robustly fitted in each image, which can
be cast in the linear framework (1). In that case,
A = (a
1
,··· ,a
d
) are the parameters of a curve within
a linearly parameterized family: y =
∑
d
j=1
a
j
f
j
(x).
The road shape features (x
i
,y
i
) are given by the lane-
marking centers extracted using a local feature ex-
tractor (Ieng et al., 2004). In (1), the noisy obser-
vations are the y
i
. The vector x
i
= ( f
j
(x
i
))
j=1,···,d
is assumed non random. The problem is thus set
in the fixed design and maximum robustness can be
achieved by modeling the geometric noise using the
GTF family. Within the GTF family, the IRLS algo-
rithm is used several time to refine the curve fitting

Figure 7: Three examples of detected lane-marking of de-
gree 3 (in green). The uncertainty about the lane-marking
location are also shown (in red).
result with decreasing scale s, until the scale of the
noise is reached. The initial solution is obtained with
a scale large enough to imply a convex minimization
problem. The SEF family can also be used if it bet-
ter corresponds to the observed noise. In such a case
rather than to decrease the scale, we refine the curve
fitting by decreasing α step-by-step until the α of the
observed noise is achieved. The initial solution is ob-
tained with α = 0.5 where the minimization problem
is convex. These two strategies are examples of the
GNC strategy.
Finally, the IRLS, with GNC strategy, allows
curve tracking in real time, contrary to other robust
methods such as LMedS and RANSAC. Three exam-
ples of main curve detection and tracking are shown
in Fig. 7.
5.2 Edge-Preserving Image Smoothing
(a)
(b)
Figure 8: The original image (a), perturbated with 20% salt
and pepper noise (b) (PSNR=11.5dB).
Image smoothing is an important topic in image anal-
ysis. Figure 8 shows an original image and the same
image perturbated with photometric salt and pepper
noise. As is well-known, using classical Gaussian fil-
tering degrades edges, as shown in Figure 9(a). This
motivated many works on nonlinear filtering (Astola
and Kuosmanen, 1997) and edge-preserving image
smoothing, see e.g. (Kervrann and Boulanger, 2006)
for more references. In particular, bilateral filter-
ing (Tomasi and Manduchi, 1998) is very intuitive be-
cause it is only a generalization of Gaussian smooth-
ing that takes into account both spatial and intensity
variations in the vicinity of each pixel. In (Elad,
2002; Kervrann, 2004), the bilateral filtering theoret-
ical background is explained which opens the possi-
bility of using high breakdown point M-estimators de-
rived from SEF and GTF noise models. Indeed, bilat-
eral filtering can be seen as a linear estimation prob-

lem Y = A + B where Y is the observed image, A is
the source image and B is the noise. Thus, bilateral
filtering can be set as in (1) within the fixed design.
Let us consider a particular pixel (i, j). Its ob-
served intensity is y
i, j
, and a
i, j
is the noiseless inten-
sity that is to be estimated over a square neighbor-
hood [i −m,i + m] ×[ j −m, j + m], assuming a non-
Gaussian noise. To this end, the following error crite-
rion is minimized:
e(a
i, j
) =
m
∑
k=−m
m
∑
l=−m
ρ(a
i, j
−y
i+k, j+l
)k(k,l) (11)
where ρ characterizes the noise model along inten-
sities and k is a decreasing function w.r.t. the dis-
tance to the origin. This k allows to take into ac-
count the spatial distribution of the pixels and most
of the time, a Gaussian kernel is used (Tomasi and
Manduchi, 1998). One iteration of bilateral filtering
directly consists of applying the IRLS algorithm de-
rived from (11), for each image pixel.
In (Tomasi and Manduchi, 1998; Elad, 2002;
Kervrann, 2004), it is suggested to use functions from
the M-estimator theory, for ρ. ρ
α
is an interesting
candidate because it allows continuous transition be-
tween different kinds of pdfs, with increasing robust-
ness when α decreases. Figure 9 displays the re-
sults obtained on the noisy image shown in Figure 8
for bilateral filtering with SEF and different values
of α. When α = 1, the obtained filter is equivalent
to a weighted mean with Gaussian weights. When
α = 0.5, ρ(x) approximates |x| and hence, the filter
behaves as a spatially-weighted median filter. As can
be seen on Figure 9, the lower the value of α, the bet-
ter the restauration quality. However, when α < 0.5,
ρ
α
becomes non-convex and the estimator can get
stuck in a local minimum, resulting in poor results as
show on Figure10(a).
Similarly to (Kervrann, 2004) where the scale pa-
rameter is decreased regularly, we propose to decrease
α step-by-step in a continuation strategy. This is an-
other illustration of the effectiveness of the GNC strat-
egy, see Figure 10(b).
6 CONCLUSION
In this paper, we applied Mizera and M
¨
uller’s funda-
mental work on M-estimators breakdown point cal-
culation in the field of image analysis. In the fixed
design setting, they shown that certain M-estimators
can achieve maximum robustness. Using their re-
sults, we discussed the robustness of M-estimators
based on two non-Gaussian pdfs families, that we in-
troduced under the names of SEF and GTF. In par-
ticular, we shown that the GTF noise model leads to
(a)
(b)
(c)
Figure 9: On the noisy image in Figure 8(b), a bilat-
eral filtering is applied with different values of α within
SEF: Gaussian-weighted mean filtering in (a) with α = 1
(PSNR=20.3dB), α = 0.75 in (b) (PSNR=25.2dB), and
equivalent to weighted median filtering in (c) with α = 0.5
(PSNR=28.1dB). Notice how results are improved when α
decreases.

(a)
(b)
Figure 10: The result after bilateral filtering with SEF and
α = 0.25 is in (a) (PSNR=19.6dB). Outliers sill remains due
to the fact that the corresponding ρ function is non convex.
However, a better result is obtained in (b) using GNC strat-
egy (PSNR=28.1dB).
M-estimators that achieve the maximum breakdown
point of approximately 50%, and that the robustness
associated with SEF increases towards the maximum
as α decreases towards 0.
In the second part of this paper, we illustrated how
useful these results are in the context of image analy-
sis: SEF and GTF approximate models seems to cor-
rectly fit observed noise pdfs in diverse applications
and contexts. Moreover, many image analysis prob-
lems can be seen as parametric estimation or cluster-
ing. In the applications we shown (curve fitting and
edge-preserving image smoothing), we observed the
advantage of varying the noise model, progressively
introducing robustness, with the so-called GNC strat-
egy. We therefore believe that the SEF and GTF fam-
ilies can also be used with advantages in many other
image analysis algorithms.
REFERENCES
Astola, J. T. and Kuosmanen, P. (1997). Fundamentals of
nonlinear digital filtering. CRC Press, Boca Raton,
New York, USA, ISBN 0-8493-2570-6.
Elad, M. (2002). The origin of the bilateral filter and ways to
improve it. IEEE Transactions on Image Processing,
11(10):1141–1151.
Hampel, F., Rousseeuw, P., Ronchetti, E., and Stahel, W.
(1986). Robust Statistics. John Wiley and Sons, New
York, New York.
Hasler, D., Sbaiz, L., Ssstrunk, S., and Vetterli, M. (2003).
Outlier modeling in image matching. IEEE Transac-
tions on Pattern Analysis and Machine Intelligence,
25(3):301–315.
Huang, J. and Mumford, D. (1999). Statistics of natural
images and models. In Proceedings of IEEE con-
ference on Computer Vision and Pattern Recognition
(CVPR’99), pages 1541–1547, Ft. Collins, CO, USA.
Huber, P. J. (1981). Robust Statistics. John Wiley and Sons,
New York, New York.
Huber, P. J. (1984). Finite sample breakdown of m- and p-
estimators. Annal of Statistics, 12:119–126.
Ieng, S.-S., Tarel, J.-P., and Charbonnier, P. (2004). Eval-
uation of robust fitting based detection. In Proceed-
ings of European Conference on Computer Vision
(ECCV’04), pages 341–352, Prague, Czech Republic.
Kervrann, C. (2004). An adaptative window approach for
image smoothing and structures preserving. In Pro-
ceedings of European Conference on Computer Vi-
sion, pages 132–144, Prague, Czech Republic.
Kervrann, C. and Boulanger, J. (2006). Unsupervised
patch-based image regularization and representation.
In Proc. European Conf. Comp. Vision (ECCV’06),
Graz, Austria.
Meer, P., Stewart, C. V., and Tyler, D. (2000). Ro-
bust computer vision: An interdisciplinary challenge.
Computer Vision and Image Understanding: CVIU,
78(1):1–7.
Mizera, I. and M
¨
uller, C. (1999). Breakdown points and
variation exponents of robust m-estimators in linear
models. The Annals of Statistics, 27(4):1164–1177.
Srivastava, A., Lee, A., Simoncelli, E., and Zhu, S. (2003).
On advances in statistical modeling of natural im-
ages. Journal of Mathematical Imaging and Vision,
18(1):17–33.
Tarel, J.-P., Ieng, S.-S., and Charbonnier, P. (2002). Us-
ing robust estimation algorithms for tracking explicit
curves. In European Conference on Computer Vision
(ECCV’02), volume 1, pages 492–507, Copenhagen,
Danmark.
Tomasi, C. and Manduchi, R. (1998). Bilateral filtering for
gray and color images. In Proceedings of 6th Inter-
national Conference on Computer Vision, pages 839–
846, New Delhi, India.
