THE DESIGN OF BIAXIAL JOINT FOR MOBILE

ELECTRONICS WITH THE ANALYSIS ON ARTHROSIS

Zhao Danpu, Yi Qiang, Nie Chenghui, Chen Ken, Liu Li

Dept. of Precision Instruments and Mechanology, Tsinghua University, Beijing 100084, China

Xu Leon, Salo Antti

Nokia Research Center, Beijing 100013, China

Keywords: Bionic, joint, arthrosis.

Abstract: To bring forward the new form factors is one of the key drivers for future mobile electronic devices. On the

other hand, some form factors in nature with evolution process have been the excellent and adaptive. In this

paper, we pay attention to the characteristics of arthrosis, researched on the difference between the arthrosis

and machine joint. Then the essentials and parameters of the biaxial joint design were introduced. After that

a biaxial joint concept for portable electronics based on the bionic principle was proposed. Finally, we

provided the statics analysis of the biaxial joint.

1 INTRODUCTION

In recent years, more and more mobile electronic

devices have become an improtant part of people’s

life. One of the key drivers for future electronic

mobile devices is to enable drastic change of the

physical appearance of mobile terminals with totally

new product category possibilities.

The clamshell type electronic mobile device is

the most popular type. The joint divided into two

parts and the opening angle is general at 160 degree.

Obviously, the structure of the joint limits the

opening angle. Another problem of this type of

communication between two parts is secular fold. So

many researches pay attention to the design of rotary

joint.

Most of rotary joints only have one axial and two

parts of joint turned encircling the axial. Because of

the motion intervention, the friction cannot be

avoided.

In our research, the design of a biaxial joint

based on the bionic principle is introduced. The

biaxial joint has two perpendicular axes. Two pairs

of apposing movements take place along these axes

respectively and circumduction is permitted.

2 THE CHARACTERISTICS OF

ARTHROSIS

Locomotor system includes bones, joints and

muscles. Each bone is linked with joints. Every

arthrosis has some common structures. The typical

one is as Figure 1. Compare with the machine joint,

the arthrosis have some characteristics:

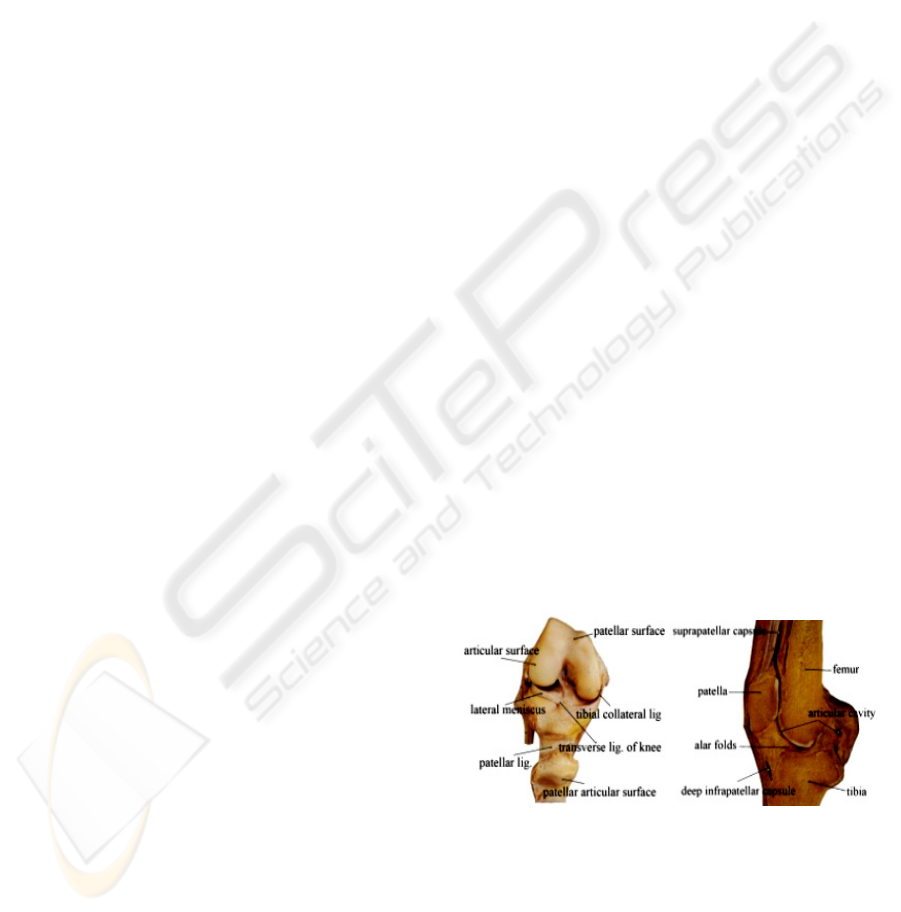
Figure 1: The structures of a typical synovial joint.

The articular surfaces are the smooth surfaces

composed by non-standard curve or surfaces.

The articular surfaces indirectly connect with

cartilages. The articular discs make joint surfaces fit

further with one another, increase stability of joints

and absorb large forces of compression and shear.

138

Danpu Z., Qiang Y., Chenghui N., Ken C., Li L., Leon X. and Antti S. (2008).

THE DESIGN OF BIAXIAL JOINT FOR MOBILE ELECTRONICS WITH THE ANALYSIS ON ARTHROSIS.

In Proceedings of the First International Conference on Biomedical Electronics and Devices, pages 138-142

DOI: 10.5220/0001048101380142

 SciTePress

The ligaments are the driver of body structures.

Usually, one arthrosis connects with one more

ligaments. When the arthrosis move, the ligaments

around it will cooperated drive the arthrosis to move

with a non-repeated track.

3 DESIGN OF THE BIAXIAL

JOINT

3.1 The Essentials of the Joint Design

In this project, a bionic joint would be designed for

the joint of electronic devices. Therefore some

essentials of the main joint design as follow:

While design joints the rolling friction should be

selected for decreasing the affect of friction.

Usually the wire is easily failed when folded

repeatedly. So the wire should cling on the joint

surface to acquire the support. Also the curvature

radius of joint should be enlarged at full steam.

As articular discs, some filling would be

accepted and it can make joint surfaces fit further

with each other, increase stability of joints and

absorb the large forces of compression and shear.

Imitate the body drivers, some special drivers

should be selected with the small structure and for

parallel movement.

3.2 The Surfaces Design of Joint

In body, the hinge joint has two parts, concave and

convex, and movement takes place on sagittal plane,

e.g., the elbow and ankle. With the analysis on the

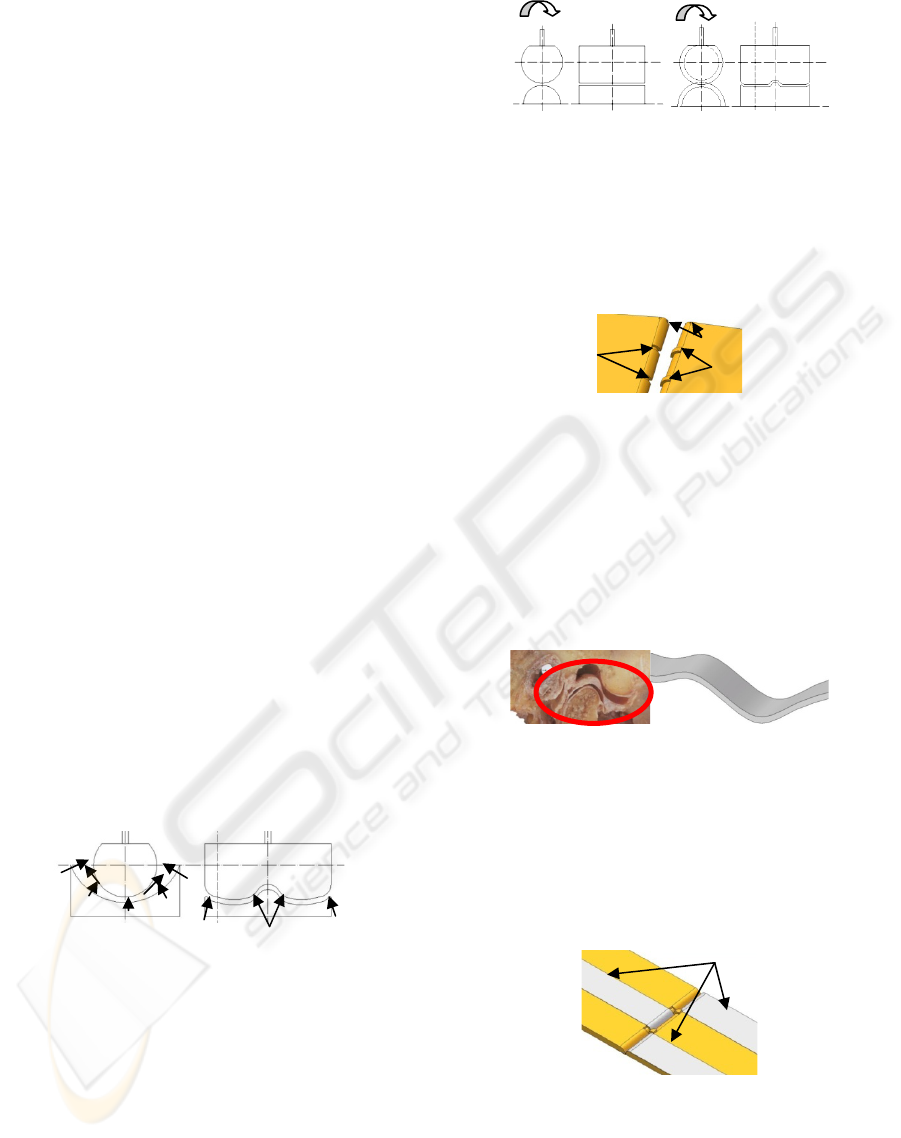
structures of hinge joint, it is described as Figure 2.

Figure 2: The sketch of hinge joint. The N means the

support forces, f is the frictions, F is the transverse forces.

For avoiding the friction, the concave part will be

replaced by convex, and the two parts are tangent.

See Figure 3 (a). Transverse displacement should

also be considered. Simulate the cooperation of the

neck and convexity, some keys and slots should be

designed. So the joint is showed as Figure 3 (b).

(a) (b)

Figure 3: The sketch of joint.(a) joint with double convex,

(b) joint with key and slot.

As the analysis before, the curvature radius should

be enlarged. So the section of the joint part can be

designed as demisemi circularity. So the biaxial joint

structure is described as Figure 4.

Figure 4: The sketch of final biaxial joint structure.

3.3 The Design of Medium in Joint

For the fit and stable of the structure of synovial

joints, there are other structures inside the joint, such

as articular cartilages.

See the sagittal section of temporomandibular

joint in Figure 5 (a), the articular disc suit on the

surfaces of two joint parts.

(a) (b)

Figure 5: (a) The sagittal section of temporomandibular

joint. (b) The sketch of connective band.

Simulate the structure and function of the articular

disc, a connective band is design as Figure 5 (b). It is

suited on the surfaces of two joint parts.

For keeping balance, three bands from different

directions interlude the joint cavity. See Figure 6.

Figure 6: The biaxial joint with connective band.

3.4 The Design of the Joint Driver

In the movement of articular, muscles drive the

joint. They compress and elongate to change the

direction between two attachments on the different

F F F

Connective band

Slot

Key

Dot for muscle

THE DESIGN OF BIAXIAL JOINT FOR MOBILE ELECTRONICS WITH THE ANALYSIS ON ARTHROSIS

139

bones. The greatest excellence of using muscle

drivers in joint is the minimal volume. In this

research, artificial muscles would be selected as

joint drivers.

In the biaxial joint, one part would turn around

the other back and forth. Therefore two couples of

drivers should be emplaced on the double side of the

joint.

For balance, they should be distributed

symmetrically. In this design, the turn range of the

joint can be from 0 to 360 degree. See Figure 7.

Figure 7: The biaxial joint with artificial muscles.

4 STATICS ANALYSIS OF THE

BIO-JOINT

In this paper, because of the tangent motion between

two joint surfaces, the slip resistance can be ignored.

Predigesting the joint structure, the joint surfaces

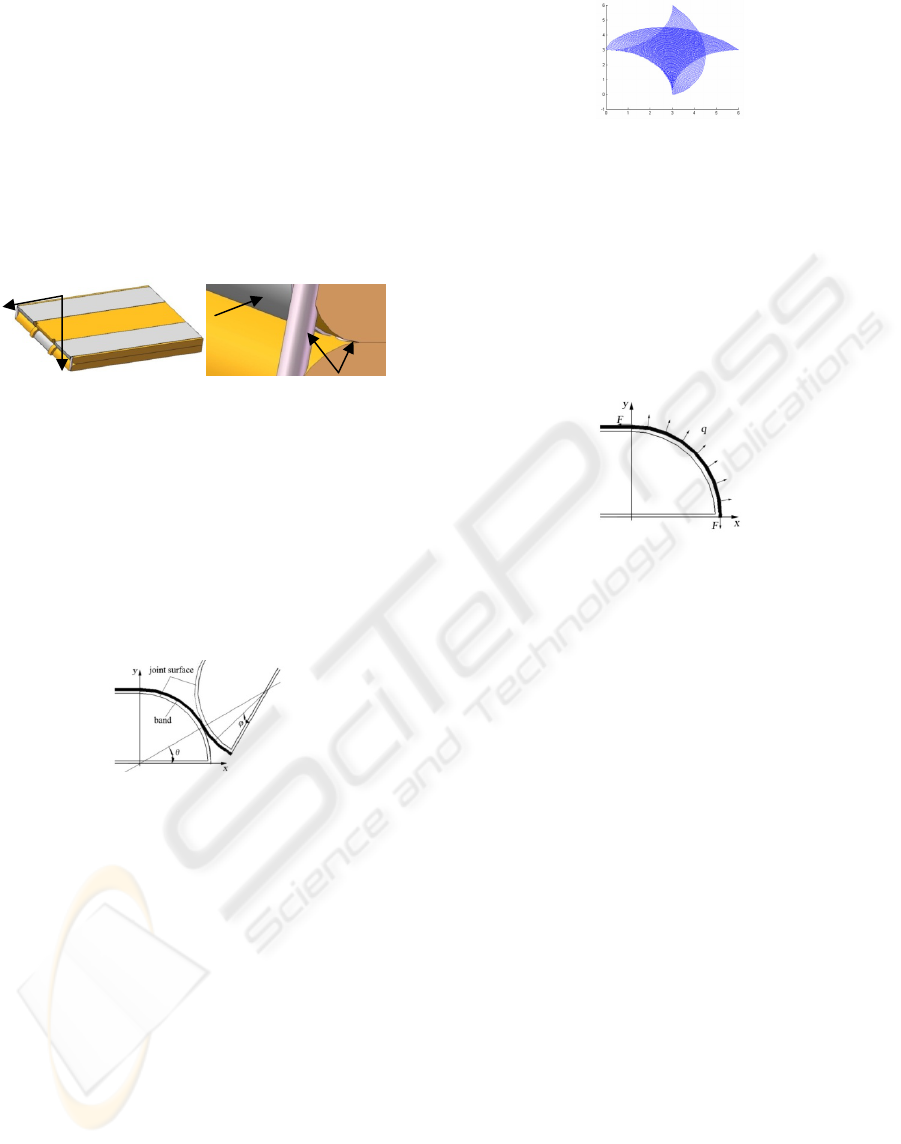
with connective band can be modelled as Figure 8.

Figure 8: The model of the system.

4.1 The Motion Track

If we establish the coordinate at one of the circles,

the other one rotated on its surfaces. So the motion

track equation of discretionary point on the moving

circle can be founded as

X=2Rcosθ-Rsin(π/2-2θ+φ) (1)

Y2Rsinθ-Rcos(π/2-2θ+φ)



(2)

Here φ is the angle between the point on the joint

surface and underside of the joint.

Figure 9: The motion track of the joint.

When the φ changed from 0 to π/2, we draw one

track with every 0.25π degree. And the tracks are

described as Figure 9 in area XY.

4.2 The Forces of the System

For ensuring the reliability of connection of two

joint surfaces, beforehand force F would be added

on the bands. The forces are showed in Figure 10.

Figure 10: The sketch of the forces on system.

Here, F is the beforehand force, q is the forces

density. So the force on arc with the length as Rdθ is

f=qRdθ.

The component of forces on the axial X and Y is

=qRdθ

sinθ, f

=qRdθ

cosθ

(3)

According to the principle of forces balance, it

can be described as

θθ

dqRF

∫

sin

(4)

Then

q=F/R (5)

4.3 The Stress

4.3.1 Stress on the Band

As it described before, in this design the fix up of

joint is realized by some connective bands. So the

bands are the primary force suffering object. With

the repeatedly folding, it should be laid-back and

wearing. Thereby the integrality and reliability of

structure would be destroyed. So in this part, the

stress of connective band would be analyzed.

Firstly, the normal stress for the beforehand

forces can be

=F/A

(6)

Artificial muscle

Connective band

Artificial muscles

BIODEVICES 2008 - International Conference on Biomedical Electronics and Devices

140

Here, A

is the section area of connective band.

Then, the normal stress for bending moment is

=My/I

(7)

Here, y is the direction between the point and central

on section. And M=F(R-Rcosθ) is the bending

moment on the section,

∫

bbz

dAyI

is the

moment of inertia for axial

So the whole normal stress on connective band

can be described as

=σ

+σ

= F/A

+ My/I

(8)

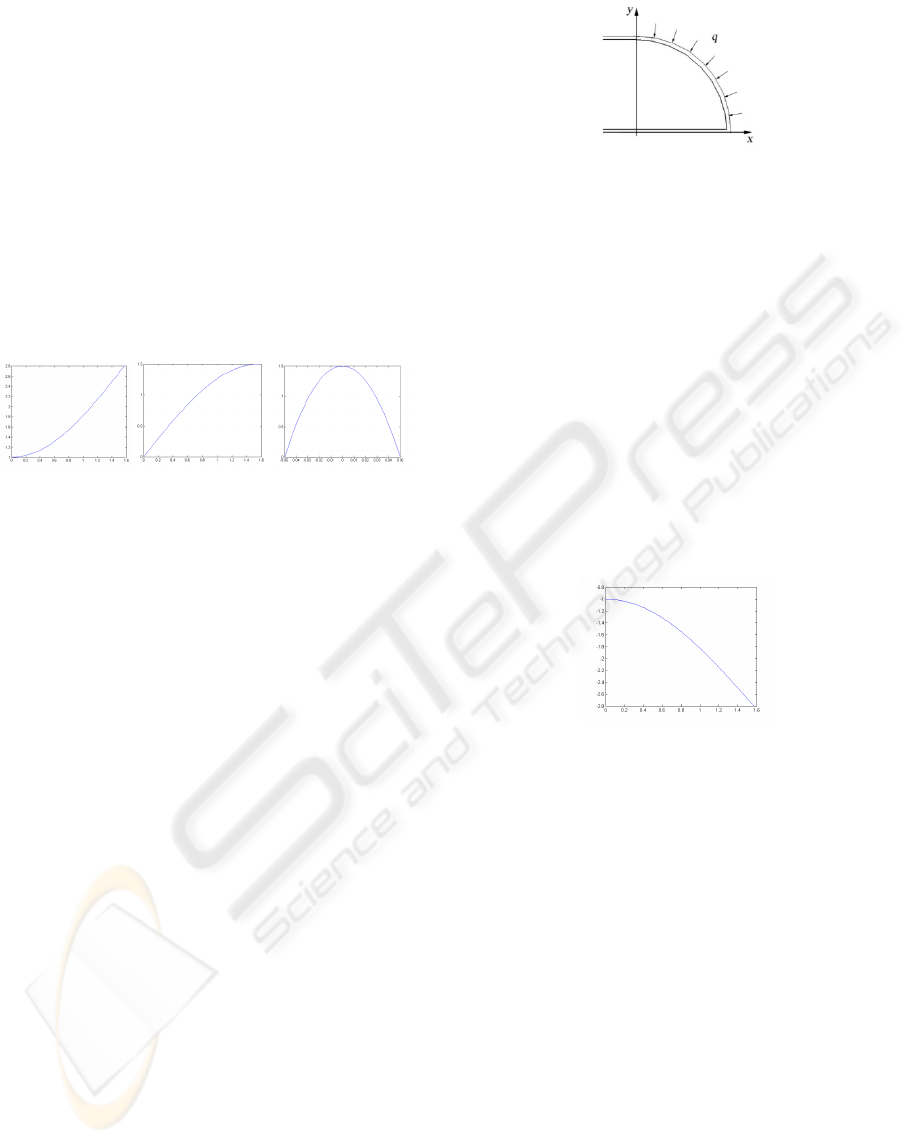
The maximal normal stress on connective band is

at the point when

y=y

max

. It changed with θ can be

described as Figure 11 (a).

(a) (b) (c)

Figure 11: The maximal stress on connective band. (a)

Normal stress changed with θ, (b) Shear stress changed

with θ, (c) The τ

change with y.

At the same time, the shear stress at y on section

can be showed as

=3F

(1-4y

)/2bh

(9)

Here,

is the thickness of band, b is the breadth

of it, and F

=Fsinθ is the shearing force on the

section.

In Figure 11 (b) the curve of maximal shear

stress changed with

θ is described.

A maximum of F

when θ=π/2 can be get. So

the

with such F

can be described as Figure 11 (c).

The maximum of

can be found when y=0.

4.3.2 Stress on the Joint Surface

Because of the no direct touch between two joint

surfaces, the joint surface gets the press form

connective bands only when it turned. See Figure

12. Due to the radial press with the forces density

the axial loads is

F, so the normal stress from the

press on the joint surface is

=qR/ A

=F/A

(10)

Here, A

is the section area of joint surface.

Figure 12: The sketch of the forces on joint surface.

Then, the normal stress for bending moment on

the joint surface is

=My/I

(11)

Here,

y is the direction between the point and central

on section. And

()

∫

−=

ϕϕθ

dqRM sin

is the

bending moment on the section,

∫

jzj

dAyI

the moment of inertia for axial

So the whole normal stress on joint surface is

=-σ

+σ

= -F/A

+ My/I

(12)

The maximal normal stress on joint surface is at

the point when

y=y

max

. It changed with θ can be

described as Figure 13.

Figure 13: The maximal normal stress changed with θ.

Also, the shear stress is existed on the joint

surface. The shear stress at

y on section can be

showed as

=3F

(1-4y

)/2bh

(13)

Here,

is the thickness of joint, and

()

∫

ϕϕθ

d-qRF cos is the shearing force.

So we can get a similar curve as Figure 12, the

maximal shear stress changed with

y is described.

4.4 The results

In this design, when one surface round on another,

although the point on one changed his track

direction after it pass the tangency part, but due to

the tangent point track is arc, the move is smooth.

Based on the forces analysis, there’s no slip

resistance between two surfaces. It’s the virtue of

such design, because it defence the energy wasting

and surfaces wearing; and at the same time, it is a

THE DESIGN OF BIAXIAL JOINT FOR MOBILE ELECTRONICS WITH THE ANALYSIS ON ARTHROSIS

141

shortcoming, because it can not stop at any part with

friction. But here we bring out the driver of artificial

muscle, the orientation is ensured.

When selecting the material of connective band,

only if the allowed stress is larger than maximal one

gained before, reliable structure can be accepted.

5 CONCLUSIONS

As one of the most important part of electronic

mobile devices, the design of joint catches the great

attention of researchs. One of the key drivers for the

devices is to enable drastic change of the physical

appearance of mobile terminals with totally new

product category possibilities. In this paper, some

characteristics of the arthrosis are analyzed. And we

bring out a biaxial joint for electronic mobile

devices based on the bionic principle. At last, the

statics of such biaxial joint is analyzed.

REFERENCES

Stephanie, R., Michelle, M., Stephen, E., 2004. Anatomy

for Diagnostic Imaging, SAUNDERS. Spain, 2

edition.

Liu, Z. Y., 2007. Chinese-English Textbook of Systemic

Anatomy, Science Publishing Company, China, 1

edition.

William, C. M., 2005. Smart Technology for Aging,

Disability, and Independence: the state of the science,

WILEY-INTERSCIENCE. New Jersey, 1

edition.

Ben P., 1975. Dynamic Anatomy and Physiology,

Macmillan publishing Co. American, 1

edition.

Zhou X., Zhao Zh., 2000. Establishment of TMJ model

including mandible by mean s of three-dimensional

finite element method. J Pract Stomatol, Vol. 16 (1),

P17-19.

LI X., ZHANG R., 2004. Artificial Muscles Based on

Conducting Polymers. Journal of Materials Science &

Engineering, Vol. 22 (1), P128-131.

CAO X., WANG B., 2004. The Decision of Fatigue Stress

Table of Belt. Journal Of Wuhan University Of

Technology, Vol. 26 (9), P67-70.

BIODEVICES 2008 - International Conference on Biomedical Electronics and Devices

142