A WIRELESS ACQUISITION SYSTEM FOR MONITORING THE

INFLUENCE OF LOADS ON VERTEBRAL COLUMN

BEHAVIOUR

João Eduardo Castro Ribeiro

and João Paulo Pereira do Carmo

ξχ

Instituto Politécnico de Bragança, Bragança, Portugal

Departamento de Tecnologia Mecânica

Departamento de Electrotecnia

Keywords: Biotelemetry, biomechanical and Wireless electromyography (EMG).

Abstract: This paper presents a wireless acquisition module (WAM). This allows the monitoring of heavy loads

influence on vertebral column’s behaviour. Each module makes the electromyography (EMG), to measure

the electric potentials on the iliocostalis and longissimus thoracis muscles, and use a dual-axis accelerometer

to get the movements of the body, in order to obtain the complete behaviour of the vertebral column. The

solution chosen to transmit the body’s measured signals for further processing, is a wireless link working in

the 433 MHz ISM band. The acquired information is transmitted with a maximum rate of 40 kbps, a

resolution of 9.8 μV, and accommodates two analog channels. An analog channel with differential input

connected to the electrodes, is used to measure the EMG signal, while the remained channel is used in the

patient’s movements measurements. The dimensions of the proposed acquisition system are

about 7×5×2 cm, and will help to understand the influence of heavy loads as a risk factors in the vertebral

column, such as the scoliosis and lordosis.

1 INTRODUCTION

The human posture has been an object of studies in

biomechanics, once some deviations of structural

and functional positions induce an unbalanced body.

These deviations usually, affects the vertebral

column and are caused by physical efforts, bad

postures in work, deficiency in sustentation muscles,

infections and congenital causes. The main

pathologies of vertebral column caused by the

referred deviations, are the scoliosis and lordosis.

Sometimes these pathologies appears in children

when they carry the heavy backpacks on the backs,

in this case, it’s very important monitoring the

influence of loads (backpack weight) in vertebral

column behaviour.

The vertebral column has very important functional

requirements, which the most significant are: it

carries and supports the thoracic cage, maintaining

the balance between it and abdominal cavity; it gives

attachment to many muscles of the pectoral and

pelvic girdles; it provides anchorage for many

powerful muscles, which move the vertebral

column, these same muscles maintaining the balance

and erectness of the human trunk; it acts as shock

absorber, by virtue of its curvatures and the

intervertebral discs, receiving and distributing the

impacts associated with the dynamic functioning of

the body; it is able, by virtue of its flexibility, to

produce and accumulated moments of force as well

as to concentrate and transmit forces receiving from

other parts of the body (Palastanga et al, 2002).

When someone carries a heavy object like a

backpack on the back, the center of gravity of body

changes and it will be necessary some adaptations to

maintain the balance, these adaptations are possible

because the action of powerful muscles like

iliocostalis or longissimus thoracis, and the relative

movement among the intervertebral discs. In the

children who carries heavy backpacks repeatedly

and for long time they could suffer injuries in the

vertebral column that can develop pathologies like

scoliosis. Figure 1 show vertebral column with a

scoliosis.

The study of influence of backpack weighs on the

vertebral column of children is an important issue,

that has been worked by many researchers for years

(Nissinen at al, 1994; Widhe, 2001; Skaggs et al,

2006).

In this work it is used a different approach, applying

indirect information, using the electrical potential

233

Eduardo Castro Ribeiro J. and Paulo Pereira do Carmo J. (2008).

A WIRELESS ACQUISITION SYSTEM FOR MONITORING THE INFLUENCE OF LOADS ON VERTEBRAL COLUMN BEHAVIOUR.

In Proceedings of the First International Conference on Biomedical Electronics and Devices, pages 233-237

DOI: 10.5220/0001049302330237

 SciTePress

generated by the muscles, when they contract and

when they are rest. The technique which could

measure this electrical potential is the

electromyography (EMG). Based on the results of

EMG, combined with the movements of the body,

measured by the accelerometers, it is possible to

know by numerical simulation, the displacement

occurred on the insertions points between the

muscles and the vertebral column

(Conceição, F. et al, 2007; Pato, M. et al, 2007).

Using these values of displacements in a finite

element code, like ANSYS®, we can compute the

value of stress field in the vertebral column,

especially it is possible to observe where are the

points more affected and the respective stress value.

Figure 1: Radiograph showing a vertebral column with a

scoliosis.

2 KINEMATICS

The skeletal system of the trunk comprises the

pelvis, vertebral column and rib cage. The vertebral

column is divided into four regions: cervical;

thoracic; lumbar and sacrum.

The vertebral column is a flexible rod with seven

mobile segments in the cervical region, twelve

segments in the thoracic region and five segments in

the lumbar region. The fourth region of the vertebral

column, the sacral-coccyx region, includes nine

fused vertebrae that together with the right and left

ilia form the pelvis.

Two adjacent vertebrae and their interposed

intervertebral disk form a motion segment of the

vertebral column. Each segment has six degrees of

freedom (DOF). In the vertebral column, there are

two types of joints: the intervertebral joints between

the vertebrae and adjacent disks and the facet joints

between the facets (articular processes) of the

neighbouring vertebrae. Movement at the

intervertebral and facet joints of the same motion

segment is coupled.

Figure 2: The regions of the vertebral column (Bridwell,

2007).

The intervertebral disks are flexible spacers between

adjacent vertebrae. The disk consists of a central

nucleus pulposus, which is a ball of hydrophilic

jelly, and the outer annulus fibrosus, a series of

laminae formed by collagen fibers. The disc height

(thickness) increases from the cervical lumbar

region from about 3 mm to 9 mm.

Any change in vertebral column posture involves the

joined movement of several motion segments.

People cannot move the individual motion segments

independently.

Kinematics of the vertebral column deals either with

the specific motion segments or with the entire

region of the vertebral column or

both (Zatsiorsky, 1998).

Each of the motion segments has six DOF: because

the intervertebral disks can deform, the vertebrae, in

addition to being able to rotate, and translate. The

vertebral column as whole can produce only three

movements: flexion-extension, lateral flexion and

axial rotation. The vertebral column movement

results from concurrent rotation and translation of

the vertebrae. The amount of motion available at

various motion segments depends mainly on the size

of the disks, while the orientation of the facet joint

surfaces, which changes from region to region,

defines the direction of the allowable movement.

The relative movement of the motion segments of

the vertebral column is allowed by an elastic

connection provide by the intervertebral disks.

Vertebral flexion and extension causes compression

in one part of the disk and traction in another part.

When a relative motion between two consecutive

vertebrae is analyzed, the lower vertebra is usually

considered a fixed body and the upper vertebra is

treated as moving body. The coordinate axes are

taken along the inferior and posterior margins of the

stationary, lower vertebra.

BIODEVICES 2008 - International Conference on Biomedical Electronics and Devices

234

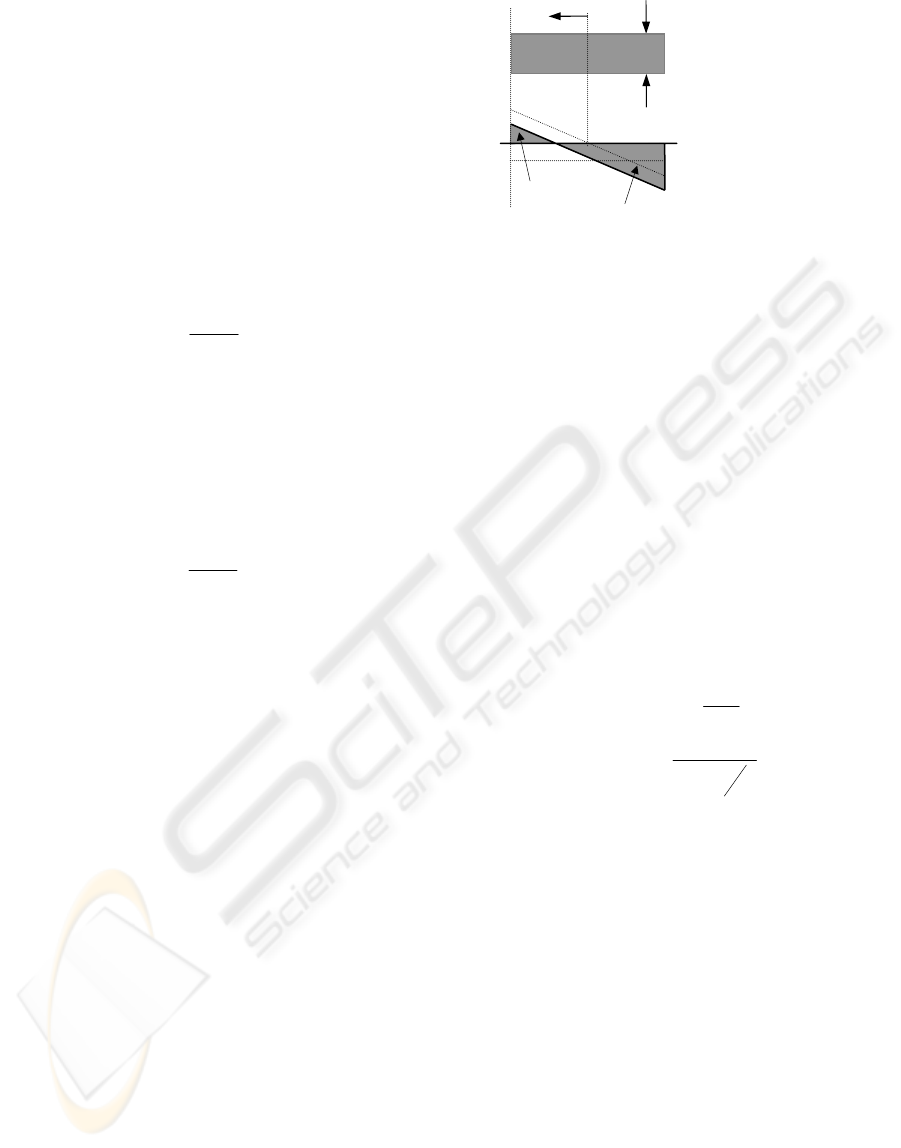
Because the disks can deform, the same resultant

force and torque being applied to various vertebrae

produces different movements depending on the disk

stiffness and its dimensions, height and diameter.

The rotation and translation of the vertebrae results

in a torsion and bending of intervertebral disks. In

case of torsion, the disk is subjected to equal and

opposite twisting couples at the two adjacent

vertebrae. The axial rotation, α

, is the angle by

which the top vertebra turns with respect to the

bottom vertebra. For a disk of a height H, radius r,

and cross-sectional area A, on which a torque M

acting, the angle of twist is:

(1)

where G is the shear modulus and I

is the polar

moment of inertia. The bending occurs when the line

of force does not coincide with the symmetry axis of

de column, and appears a bending stress

(Adams and Dolan, 1991). The equation relating

angular displacement of the disk, α

, with the

applied bending moment, M

, is

(2)

where E is the modulus of elasticity and I

is a

diametral moment of inertia, I

= 0.5I

Considering that the column is loaded with two

forces, F and –F, acting in opposite directions along

the same line of action.

The line of action does not pass through the center

of gravity (G). The center of gravity is the location

of the resultant force acting in compression and

proportional to the elements of area. The column is

under joint action of compression and bending and is

stressed correspondingly (Adams and Dolan, 1988).

The compressive stress is F/A, where A is the area

of the horizontal section. The bending stress is

M.y/I, where M is the bending moment; y is the

distance from the neutral line of the column (this is

the line that experiences neither compression nor

tension during bending); and I is the area moment of

inertia. The bending moment equals the product F×e,

where e is the eccentricity (distance from the line of

force to the center of the column). This distance is

crucial in provoking bending stress.

The relative movement of a vertebra with regard to

the vertebra immediately below it can be viewed as a

combination of rotation and translation. An infinite

number of combinations of rotation and translation

can describe the same movement. To simplify the

kinematics analysis is assuming that a vertebra

rotates around a pole whish (1) is located on the

inferior end plate of the moving vertebra and (2)

moves parallel to the inferior margin of the reference

vertebra, axis X of the coordinate system.

In framework of this model, the vertebral motion is

viewed as a translation along the axis X and the

rotation around the pole, the center of rotation

(Cossette, J. et al, 1971). Because a rotation about

the instantaneous center of rotation (ICR) is equal to

the rotation about any parallel axis, the angle

subtended at the ICR by the arc of motion of C is

equal to the angle of rotation (θ) undergone by the

whole vertebra. Because the triangle CC’R is an

isosceles triangle, HR = CC’/2tan(θ/2), where HR

and CC’ are the height and base of the triangle. If

the location of the ICR is experimentally

determined, location of C can be found as

CR IRC

XX=−

(3)

()

2tan

CR IRC

=−

(4)

The position of the ICR is determined by the

location of the center of the rotation, the translation

of the vertebra in parallel with the axis X and the

rotation of the vertebra. In some patients, as

compared with healthy people, the ICR is displaced.

The displacement can be explained in terms of the

three mentioned factors. For example, elevation of

the ICR can occur only when the translation is

decreased, the rotation is increased, or both. The

three mentioned mechanisms can be explained by a

combination of biomechanical changes, such as

increased muscle pull.

Disc

Stress diagra

Zero stress

Extension

Compression

-F

Figure 3: A model of intervertebral disk bending: the

stress distribution is in vertical cross section of a column

loaded by off-center forces, F and –F.

A WIRELESS ACQUISITION SYSTEM FOR MONITORING THE INFLUENCE OF LOADS ON VERTEBRAL

COLUMN BEHAVIOUR

235

Figure 4: An illustration of a vertebra that has rotate and

translated from an initial position to a final position about

an ICR located at R. The center of rotation C translates to

C’. H is the midpoint of interval CC’. θ is the angle of

rotation.

3 WIRELESS ACQUISITION

MODULE

The application described in this paper, needs

wireless acquisition modules (WAMs), to

simultaneously acquire the information from the

muscles and the movements of the patient. In the

first case, the use of standard electrodes makes

possible to measure the electrical potential, above

the patient’s tissue. In the second case, a dual-axis

accelerometer will measure the motion and the

positioning of the patient.

Normally, the barrier layer forms the typical 50 kΩ

impedance for 1 cm

of skin. There is also a skin

potential between the inside and outside of the

barrier layer of typically 30 mV. When the skin

stretches, the skin potential decreases to about

25 mV and this 5 mV change is what we observe as

motion artifact (Webster et al, 1984). These are the

signals that are being acquired by the electrodes, and

further amplified by the instrumentation and further

converted to the digital domain.

The Figure 5 shows the architecture of a WAM,

which is composed by an amplifier, followed by an

analog-to-digital converter (ADC) to convert the

amplified EMG signals to the digital domain, and a

dual-axis accelerometer.

The amplifier is a chain composed by the MAX4460

instrumentation amplifier, and by the MAX4249

single-ended low-noise amplifier. The MAX4460

was chosed due to its characteristics, e.g., the

low-power consumption, the ultra low-power input

current and the increased common-mode rejection

performance. This chip amplify the differential

signals obtained from the two potentials collected

with the EMG electrodes, placed in the patient’s

skin. The high input impedance of this amplifier

matches with the small-signal differential voltages

collected in the electrodes. The microcontroller

automatically selects the most appropriated gain of

this amplifier, which can be: one, ten and one

hundred. The MAX4249 makes the differential to

single ended conversion of the acquired EMG

signal, while makes a further amplification. This

amplifier was choosed due its low-noise

characteristics, to not contaminate the EMG signal.

2-axis

accelerometer

16F628

uController

ADC

transceiver

GND

Electrodes

To the

antenna

Figure 5: The block diagram of the wireless acquisition

module.

The analysis of the EMG signal is made in the

amplitude domain, thus, before proceeding to the

ADC conversion, it is required a peak detection of

the amplified EMG signal, followed by an

integration (Robertson et al, 2004). This is

mandatory process eliminates the fluctuations that

characterise the EMG signal. As shown in Figure 6,

the discharge of the capacitor, is made with a bipolar

junction transistor (BJT). The pulse transformer is

made in the WAM’s PCB, in order to not

compromise the compactness of the WAM. The

circuit size WAM achieved, using surface mount

(SMDs) devices, for all the components, including

the micropower, rail-to-rail output, dual op-amp

MAX4471, used in the circuit of the integrator.

The measurements of the motion and the positioning

of the patient’s body is made with the use of the

ADIS16003, dual-axis accelerometer of MEMS

type. This chip connects to the microcontroller, by

way of an integrated Serial Port Interface (SPI). This

accelerometer was choosed, due its build-in

temperature sensor. This sensor is very useful to

make the compensation of the temperature gradients,

specially when the devices are placed in contact with

the human body.

C’

, Y

)

ICR

, Y

ICR

)

BIODEVICES 2008 - International Conference on Biomedical Electronics and Devices

236

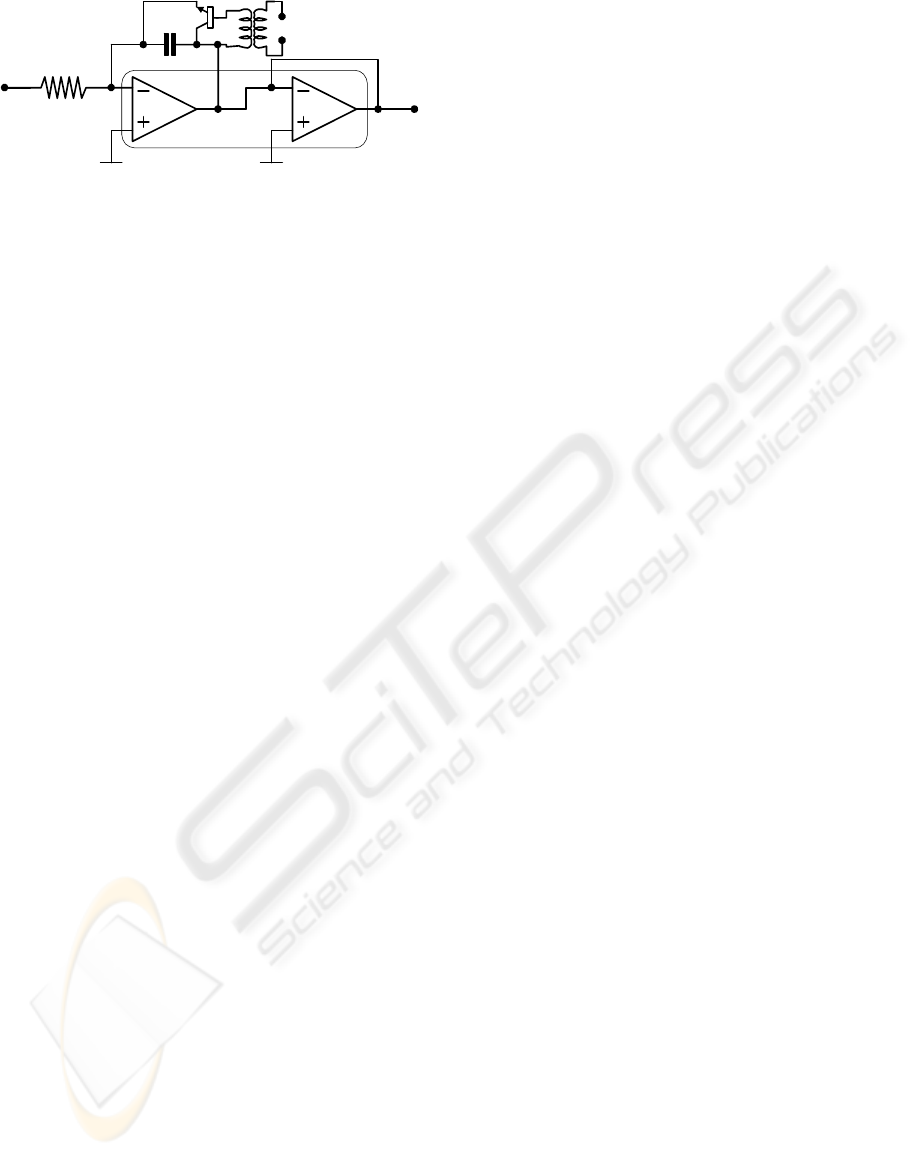
Figure 6: Integration circuit.

The microcontroller PIC16F628 from the Microchip

manufacturer, controls all the electronics, as well as

the communication between WAMs, and from the

WAM and the external base-station, where all the

processing of the acquired data is made by a health

professional, or a medical doctor. It was selected the

BiM433 radio-frequency (RF) transceiver, from the

Radiometrix manufacturer to wirelessly send the

data. A set of routines and services were developed

to make the RF communication the most reliable as

possible. The most important of these routines are

the Manchester line coding, used to solve de DC

balancing problem in the communication, when long

sequences of zeros or ones must be transmitted. It

was included a mechanism of error control in all

data frames (information, control and acknowledge

frames), e.g., the CRC field. This field makes the

detection of transmission errors in the received

bitstream (Schmidt, 2000). To make the

multi-element communication possible and reliable,

the WAMs use the Carrier Sense Multiple Access

with Collision Avoidance protocol (CSMA-CA) to

start the transmissions (Chandra et al, 2000). This

simplifies the management procedures of the

communication among modules, and between the

base-station. This makes easy to place (or remove)

new WAMs in the clothes of patients, in a

plug-and-play fashion.

4 CONCLUSIONS

It was described in this paper, a wireless acquisition

module, which will be used on the diagnosis of

vertebral column risk factors, such as the scoliosis

and lordosis. These factors normally associated to

appears in children when they carry the heavy

backpacks on the backs. Thus, it’s of extremely

importance to characterise the influence of heavy

loads (backpack weight) in the vertebral column

behaviour. This solution fits the medical doctors

requirements for an easy placement and removal of

the WAMs. This is true for the target application,

because it is needed only with a low number of

WAMs (no more than five), making possible to

mount a wireless network with these plug-and-play

modules. The main advantage of this solution, is the

maintenance of the mobility and lifestyle of patients

during the diagnosis.

A set of two alkaline 1.5-V class AA bateries

supplies the wireless acquisition module. When the

RF subsystem is on, these modules have a power

consumption of 46 mW. With the RF subsystem off,

these modules have a consumption of only 10 mW.

REFERENCES

Adams, M., Dolan, P., 1991, A technique for quantifying

bending moment acting on lumbar spine in-vivo,

Journal of Biomechanics, Vol. 24, pp. 117-126.

Adams, M., Dolan, P., Hutton, W., 1988, The lumbar

spine in backward bending, Spine, Vol. 13,

pp. 1019-1026.

Choi, P., et al, 2003, An experimental coin-sized radio for

extremely low-power WPAN (IEEE 802.15.4)

application at 2.4 GHz, IEEE Journal of Solid State

Circuits, Vol. 38, No. 12, pp. 2258-2268.

Conceição, F. et al, 2007, Modelação do actuador

músculo-tendão: os elementos elásticos em série como

slemento central de potenciação de força, Proceedings

of CMNE/CILAMCE, In Portuguese.

Cossette, J. et al, 1971, The instantaneous center of

rotation of the third lumbar intervertebral joint, Journal

of Biomechanics, Vol. 4, pp. 149-153.

Bridwell, K., 2007, http://www.spineuniverse.com/

displayarticle.php/article1286.html.

Kaggs D., et al, 2006, Back pain and backpacks in school

children, Journal of Pediatric Orthopedy, Vol. 26,

Nr. 3, pp. 358-363.

Nissinen M, et al, 194, Anthropometric measurements and

the incidence of low back pain in a cohort of pubertal

children, Spine, Vol. 19, Nr. 12, pp. 1367-1370.

Pato, M., Martins, J., Pires, E., 2007, Um modelo de

elementos finitos para músculos esqueléticos,

proceedings of CMNE/CILAMCE, In Portuguese.

Robertson, D., et al, 2004, Research methods in

biomechanics, Human Kinetics.

Schmidt, T., 2000, Microchip Technology Inc., CRC

generation and checking, Application note AN730.

Webster, J. et al, 1984, Reducing motion artifacts and

interference in biopotential recording, IEEE

Transactions on Biomedical Engineering, Vol. 31,

No. 12, pp. 823-826..

Widhe T., 2001, Spine: posture, mobility and

pain - A longitudinal study from childhood to

adolescence, European Journal of Spine,

Vol. 10, Nr. 2, pp. 118-123.

Palastanga, N., et al, 2002, Anatomy and human

movement, Fourth Edition, Edited by Butterworth

Heinemann, pp. 445-537.

Zatsiorsky, V., 1998, Kinematics of human motion, Edited

by Human Kinetics, pp. 311-326.

discharge

MAX4471

A WIRELESS ACQUISITION SYSTEM FOR MONITORING THE INFLUENCE OF LOADS ON VERTEBRAL

COLUMN BEHAVIOUR

237