MAGNETIC COUPLING ANALYSIS OF A TET POWER

DELIVERY SYSTEM

Thushari Dissanayake, David Budgett

Bioengineering Institute,University of Auckland, 70 Symond Street, Auckland, New Zealand

Aiguo Patrick Hu

Department of Electrical and Computer Engineering,University of Auckland, 3 Grafton Road, Auckland, New Zealand

Keywords: TET, inductive power transfer, power efficiency, coupling coefficient, mutual inductance.

Abstract: This paper presents a comparative study of methods to determine the coupling coefficient between primary

and secondary coils used in a transcutaneous energy transfer system designed for powering implantable

biomedical devices. A coupling analysis covering typical misalignments between coils is presented using an

analytical model, a simulated model and practical experimental measurements. The simulated model shows

good agreement with the experimental measurements. The performance of the system is characterised by

carrying out a loss analysis to compute the power efficiency of the system for different misalignment

situations. It was established that variable coupling affects the maximum power transfer capacity but has a

low impact on the power efficiency for coil separations of less than 30mm.

1 INTRODUCTION

Many implantable biomedical devices require

electrical energy for operation. Two common

methods of supplying power are using an

implantable battery or by having a percutaneous lead

from the implant to an external power supply (N. de

N. Donaldson 1983). The downsides of these

methods are the limited life span of the battery and

the potential risk of infection associated with wires

through the skin. Inductively coupled power transfer

(ICPT) technology enables transfer of power across

the skin without direct electrical connectivity. This

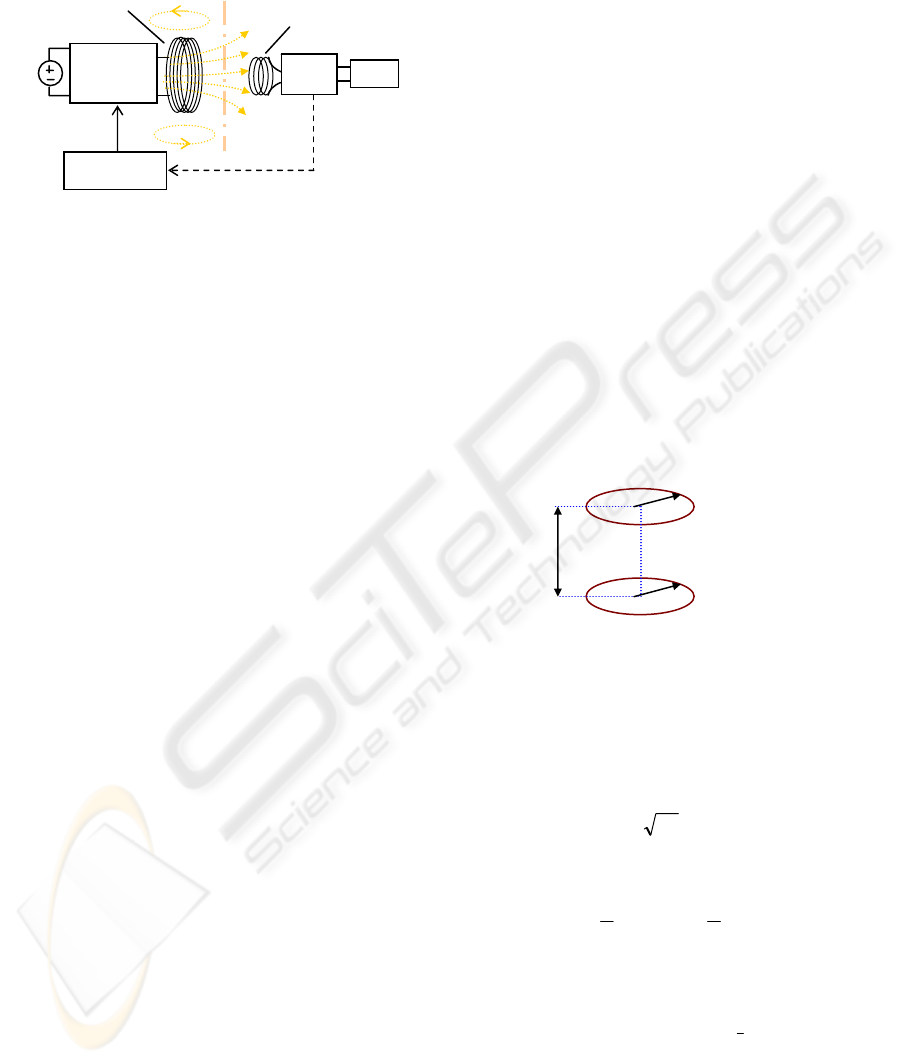
form of transcutaneous energy transfer (TET) is

illustrated in figure 1. The primary coil is located

outside the body and generates an electromagnetic

field. This time varying field penetrates the skin and

induces currents and voltages in the implanted

secondary coil which can be used to derive power

for the biomedical device.

In high power applications such as left

ventricular assist devices (LVADs), the TET coils

are located in areas of soft tissue where the coupling

conditions are highly variable. Normal patient

posture changes or differences in fitting the external

parts relative to the internal parts are likely to result

in changes to the alignment of the primary and the

secondary coils and their relative coupling (John C.

Schuder 1971). The coupling between two coils can

be represented by their mutual inductance. The

definition of mutual inductance is given by

Neumann’s double integral formula

∫∫

⋅

jio

dsds

(1)

Where µ

is the permeability of free space, ds

and ds

are elements of two coils and R

is the

magnitude of the distance from ds

to ds

. This

demonstrates that the mutual inductance is a

function of the coil geometries and the distance

between them (F.C Flack 1971). A more intuitive

representation of coil coupling is given by the

coupling coefficient, k, defined by:

21LL

k =

(2)

Where L

and L

are the self inductances of the

primary and the secondary coils respectively (C. M.

Zierhofer 1996). The coupling coefficient will equal

170

Dissanayake T., Budgett D. and Patrick Hu A. (2008).

MAGNETIC COUPLING ANALYSIS OF A TET POWER DELIVERY SYSTEM.

In Proceedings of the First International Conference on Biomedical Electronics and Devices, pages 170-175

DOI: 10.5220/0001051101700175

 SciTePress

1 for perfect coupling between two coils and zero for

no interaction.

Figure 1: Block diagram of a TET system.

Knowing the coupling coefficient between two

coils under a range of orientations is valuable for

accessing their ability to transfer sufficient power to

the load. This information can be used to guide the

design process for primary and secondary coils. The

coupling between primary and secondary coils has

been categorised into three components:

a) Separation: coils are axially aligned with a

separation gap (usually occupied by skin and

fat). For an abdominal TET site, this gap is

estimated to be within the range of 10 to 30mm.

b) Displacement: where the separation gap between

the coils is constant, but their centres are

displaced by a distance x. Typical range of

displacement is estimated to be +/-20mm.

c) Rotation: the primary coil is tilted off-axis with

respect to the secondary coil by an angle α.

It is vital to meet the power requirements of the

implantable load at various coil orientations, and to

not cause excess heating inside the implantable

device. Power efficiency is also a valuable element

in TET systems as the freedom of the patient is

restricted by the weight and duration of the external

battery pack they must carry (Hochmair 1984). This

paper provides a comparative study of the coupling

coefficient and power efficiency of a TET system

for various coil coupling conditions.

2 COUPLING ANALYSIS

Three methods of determining the coupling

coefficient between primary and secondary coils are

presented. The first is an analytical method based on

models established by Soma et. al. (Mani Soma

1985). This approach enables calculation of coupling

coefficients for a variety of geometrical offsets

between two single turn coils. The second method is

a finite element approach using JMAG Studio 8.0 to

model the coil geometries and numerically solve for

the magnetic flux density and coupling coefficient.

Finally, physical coils are constructed and the

coupling coefficient measured experimentally.

The coil geometry used in the analytical model is

considerably simplified in order to provide a

tractable closed form solution. This model assumes

that the coils are a single turn so that other

dimensional data (i.e. internal and external radius) of

the coils are ignored. The average radius between

the internal and external radius is used in these

calculations. The self inductance of L

and L

in this

instance is taken to be the inductance of a single turn

coil. For the simulated model, the coil geometry

used is the same as the experimental physical coils.

2.1 Analytical Model

To derive the analytical model, the orientation

between the primary and secondary coils needs to be

defined. Adopting the same misalignments as used

by Soma (Mani Soma 1985), the following three

misalignment conditions are considered:

2.1.1 Axially Aligned Separation

Figure 2: Axially aligned separation.

Figure 2 illustrates the orientation of the coils when

they are axially aligned. The distance d represents

the minimum separation between the two coils. The

characterisation of mutual inductance in this

orientation is given by equation 3.

)(0 sGabMF

(3)

Where

)(

)( sE

sKs

sG −

⎟

⎠

⎞

⎜

⎝

⎛

−≡

(4)

K(s) refers to the complete elliptic integral of the

first kind,

dtsttsK

∫

−

−−=

)]1)(1[()(

(5)

And E(s) is the complete elliptic integral of the

second kind,

Primary

Secondary

Primary Coil

Secondary

Coil

Load

Skin

Power

Converter

Supply

Controller

Pickup

Magnetic

coupling

Power

feedback

MAGNETIC COUPLING ANALYSIS OF A TET POWER DELIVERY SYSTEM

171

dtsttsE

∫

−

−−=

)]1()1[()(

(6)

The variable s, defines the mathematical

relationship between the primary and the secondary

coils. The formula for s is given by

()

dba

≡

(7)

2.1.2 Lateral Displacement

Figure 3: Lateral displacement.

Lateral displacement with parameter x is

illustrated in figure 3. Given that x<b in practical

situations, the mutual inductance for lateral

displacement can be defined by equation 8. G(s) in

this equation is also determined by equation (4).

)(

xba

(8)

Where s becomes

()

)(4

dxba

xba

+−+

−

≡

(9)

2.1.3 Angular Rotation

Figure 4: Angular rotation.

Figure 4 demonstrates angular rotation about the z

axis. In this analysis, misalignments up to 25

are

considered. The value of s used to calculate M

taken to be the average value of s

min

and s

max

. The

formulas used for evaluation of M

are as follows

)(

cos

avg

(10)

Where s

avg

maxmin

avg

(11)

min

and s

max

are given by

aabaaddba

aab

cos2sin2

cos4

222

min

++++

≡

(12)

aabaaddba

aab

cos2sin2

cos4

222

max

+−++

≡

(13)

2.2 Simulated Model

The use of numerical simulation alleviates many of

the geometrical simplifications that were used in the

previous analytical model. JMAG was used to

simulate the coupling relationship between the

primary and the secondary coils. Figure 5 illustrates

the mesh created for two coils for an axially aligned

orientation with 15mm separation. The mesh

contained 1435 nodes and 6982 elements.

Calculating the magnetic field generated took 6

seconds running on a 3.3GHz PC.

Figure 5: Mesh of primary and secondary coils generated

in JMAG – axially aligned.

The coils were defined by the number of turns,

physical dimensions, and ESR (Equivalent Series

Resistance). Within JMAG, an external circuit was

produced to represent the two coils and a sinusoidal

current was injected into one of the inflow faces of

the primary coil. The secondary coil was shorted to

obtain the short circuit current and equation 14 was

used to calculate the mutual inductance of the coil

for various orientations.

(14)

Primar

Secondary

Primary

Secondary

Secondar

Primar

Uni

: m

BIODEVICES 2008 - International Conference on Biomedical Electronics and Devices

172

Where I

is the short circuit rms (Root Mean

Square) current in the secondary winding, L

is the

secondary inductance, and I

is the primary rms

current. Figure 6 shows the magnetic field density

contours for a 20mm laterally displaced secondary

coil with a separation of 10mm. The magnetic field

in the secondary coil is at its highest on the edge

facing the primary and gradually drops off to zero on

the face furthest from the primary.

Figure 6: Magnetic field distribution when the secondary

coil is laterally displaced by 20mm for a separation of

10mm

2.3 Experimental Measurements

Figure 7: CAD model of physical test rig used to hold

primary and secondary coils in known physical

orientation.

A test rig was built (see figure 7) to accurately

locate the primary and secondary coils at known

orientations. The rig allows fixing of the secondary

coil in all three misalignments discussed in the

previous section. Equation 15 was used for

determining the coupling coefficient between the

coils.

openps

shortedps

1−=

(15)

Where L

ps_shorted

is the primary inductance when

the secondary is shorted, and L

ps_open

is the primary

inductance when the secondary is open, which is

equal to the primary coil inductance L1. At the same

time as acquiring coupling coefficient

measurements, the efficiency of power transfer was

also measured for the whole TET system. The

efficiency was computed as the ratio of the power

delivered to the medical device over the power

drawn from the external battery source supplying the

TET system.

3 COUPLING AND EFFICIENCY

RESULTS

The first evaluation considered a pair of coils with a

very simple geometry which could be accurately

represented using all methods. Both coils used a

single winding and figure 8 illustrates the effect on

the coupling coefficient when the lateral

displacement is increased from 0 to 20 mm at 10mm

separation. The lines ka, ks and kp corresponds to

the coefficient of coupling from the analytical

model, simulated model and experimental

measurements respectively. As expected the

coupling coefficient drops as the lateral

displacement increases, and all three methods

produced consistent results. Similar consistency is

seen for axial alignment and angular rotation cases.

Effect of displacement on coupling at 1cm

seperation

0.05

0.1

0.15

0.2

0.25

0 5 10 15 20

Lateral displacement (mm)

Couplin

Figure 8: The effect of lateral displacement on coupling at

10mm separation.

However, practical TET coils will not consist of

single turns, and they will have bulk which can be

characterised by internal and external radius and also

a physical height. Physical coils were constructed

with a self inductance of 30.4µH for the primary and

8.75µH for the secondary coil.

Effect of seperation on coupling and

efficiency

0.05

0.1

0.15

0.2

0.25

0.3

10 15 20 25 30

Distance (mm)

Couplin

40%

50%

60%

70%

80%

90%

Efficiency

Figure 9: Effects of separation between coils on coupling

and efficiency when the coils aligned axially.

2.4e-3

2.0e-3

1.6e-3

1.2e-3

8e-4

4e-4

0e-0

Primar

coil

Secondar

coil

Uni

: Tesla

Rotation Axis

Separation

Displacement

MAGNETIC COUPLING ANALYSIS OF A TET POWER DELIVERY SYSTEM

173

The three methods of evaluating the coupling

coefficient were implemented using genuine

physical parameters, and the results are shown in

figure 9. With the coils aligned axially, the coupling

coefficient decreases as the separation increases. The

simulated coupling coefficients have a mean

deviation of 16% from the experimental results.

However, the analytical results are far away. This is

illustrating the effects of the physical bulk of the

coils. The efficiency of the power transfer is also

shown in figure 9. The coupling coefficient drops at

a greater rate than the efficiency. This is illustrating

that, although the coupling coefficient may be low,

good power efficiency can still be achieved.

Figure 10 illustrates the effects of displacement

on coupling and efficiency when the coils are

separated by 10mm and 20mm. Again, the simulated

and practical coupling coefficients are fairly similar

(with a mean deviation of 9.5%), while the

analytical model significantly under estimates the

practical result at 10mm separation. However at

20mm separation, the analytical coupling coefficient

is in much better agreement with the simulated

results. This suggests that for lateral displacement at

larger separations, the magnetic coupling for the

physical coils is reasonably well represented by

idealised coils. The effect of lateral displacement on

efficiency was very small (efficiency dropped from

79% to 77% for 20mm lateral displacement) at

10mm separation. Efficiency drops more rapidly

down to 72% at 20mm separation.

Effect of lateral displacement on coupling

and efficiency

0.1

0.2

0.3

05101520

Displacement (mm)

Coefficienct of coupling

50%

60%

70%

80%

Efficiency

ka_10 mm

ks _10 mm

kp_10 mm

Efficiency_10 mm

Figure 10: Effects of displacement on coupling and

efficiency for coil separations of 10mm and 20mm.

The impact of rotational misalignment on

coupling was similar to the lateral displacement

case. The simulation result underestimated the

practical coupling for both 10mm and 20mm

separation however it was within a mean deviation

of 14.76% of the experimental measurements.

4 DISCUSSION

The maximum power transfer of a TET system

refers to the maximum power that can be transferred

from primary to secondary coil disregarding the

losses associated with the components in the circuit.

The maximum power that can be transferred by a

current-fed push-pull resonant converter TET system

has been derived by Si et. al. (Ping Si 2007) using

mutual inductance between the primary and

secondary coils. This relationship is shown in

equation 16 as a function of the coupling coefficient.

LLf

max

(16)

Where L

and L

are the primary and secondary

coil inductances, k is the coupling coefficient, P

max

the maximum power transfer, V

is the DC input

voltage, V

is the output voltage at the load and f is

the system resonant operating frequency.

Equation 16 is based on the assumption that high

order harmonic components are negligible and the

dc current is continuous at the pickup. These

assumptions are reasonable for practical circuits.

Equation 16 shows that for given primary and

secondary circuits, the maximum power transfer

capacity of a TET system is proportional to the

coupling coefficient. Good coupling can increase the

maximum possible power transfer from primary to

secondary coils. However, in terms of the power

efficiency, from the previous results shown in

section 3, it is clear that coupling does not have a

dramatic effect. This is a very important feature of

the system, meaning it is possible to achieve high

power efficiency for a loosely coupled TET system.

During the power transfer process, there is little

power loss in the air gap between the primary and

the second coils. Therefore the coupling coefficient

is not sensitive to the overall power efficiency of the

system. However with low coupling, high magnetic

strength is required to deliver the same amount of

power, so the required current and/or voltage use to

generate the field needs to be higher, resulting in a

higher loss in the drive circuits. To further

understand the power efficiency issue, the power

losses in each component of the TET system was

identified.

The loss components were measured from the

TET system and are presented in figure 11. The total

power loss between the input and output of the

system was also measured, and the difference

0.05

0.1

0.15

0.2

0 5 10 15 20

50%

60%

70%

80%

ka_20 mm

ks_20 mm

kp_20 mm

Efficiency_20 mm

BIODEVICES 2008 - International Conference on Biomedical Electronics and Devices

174

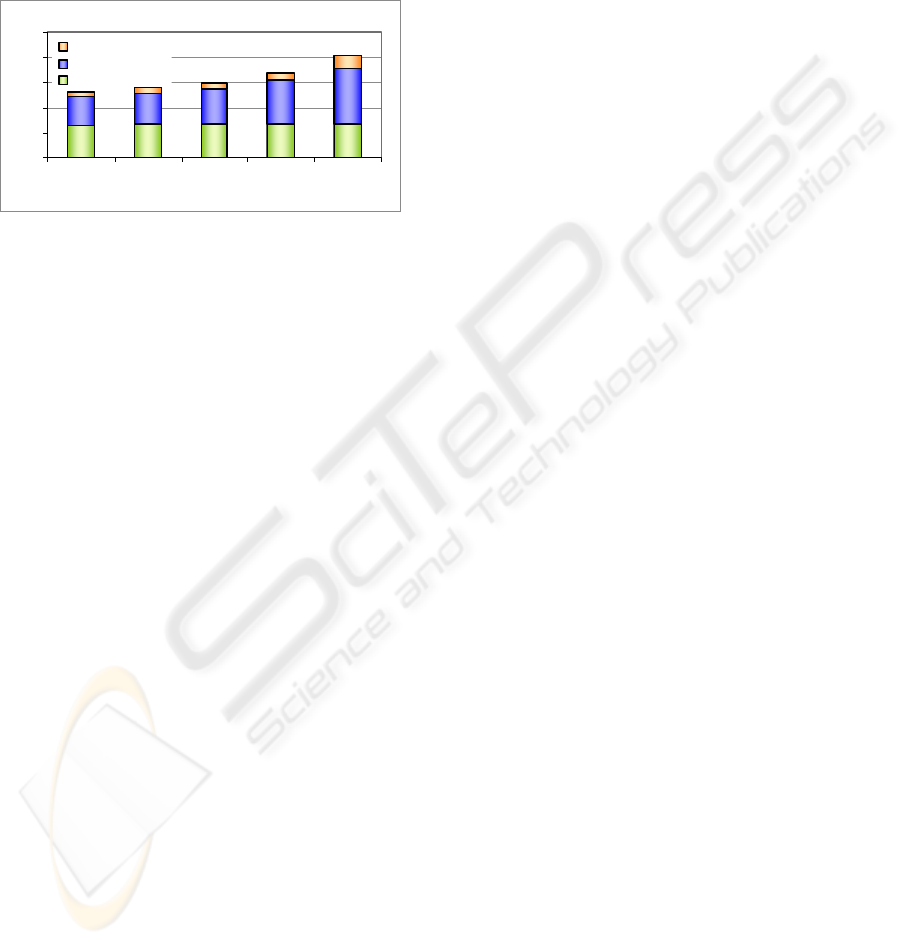
between the sum of the identified primary and

secondary losses, and the actual total losses

measured are presented as “Other” in figure 11. The

experiment was conducted under a constant load of

10W, and the input power to the TET system was

adjusted accordingly for each alignment

configuration to maintain the output power to be

constant.

Power loss contribution

10 15 20 25 30

Distance (mm)

Power loss (W)

Other

Primry loss

Secondary loss

Figure 11: Loss contributions for fully aligned case when

delivering 10W to a load.

Figure 11 shows that the total power loss

increases when the separation between the coils is

larger. However the power loss at the secondary

power circuit is more or less the same due to the

same load and circuit operating condition. This

means no additional losses are occurring at the

secondary even when the coupling is poorer. Thus

there is no complication of temperature rise and risk

of tissue damage.

The increase in total system loss is mainly from

the primary power circuit due to the need for higher

coil currents to compensate when the air gap

separation is larger. A higher strength magnetic field

is needed thus the current in the primary coil and its

tuning capacitor has to be higher, resulting in higher

losses. In this circuit losses in other parts of the

primary converter such as dc conduction losses and

switching losses also increase at the same time due

to the increased dc voltage at the input. However,

they are relatively small because the dc current of

the primary converter is relatively small compared to

the ac resonant current. Therefore (Equivalent Series

Resistance) ESR values for the primary coil and

tuning capacitor are important parameters to

consider in designing an efficient TET system.

5 CONCLUSIONS

Three methods of determining the coupling

coefficient for specific coil orientations covering the

typical range of TET coils suitable for supplying

10W of power are presented in this paper. The

analytical method was shown to be valid for

idealised coil configurations although it could not be

used to model the actual experimental setup

accurately. Numerical simulation gave a superior

match with experimental results, and is appropriate

for assessing different coil designs efficiently.

The coupling coefficient is a major factor

determining the maximum power transfer capacity

of a TET system. However, it does not determine the

system power efficiency. Power losses at the implant

were shown to be largely constant. Therefore there is

no additional heat and temperature rise when the

coupling becomes poorer. A small overall power

efficiency drop was caused mainly by the ESR

losses in the primary circuit. With a maximum coil

separation of 30mm, the variation in coupling

coefficient reduced the overall power efficiency

from approximately 80% to 70%.

REFERENCES

C. M. Zierhofer, E. S. H. (1996). "Geometric approach for

coupling enhancement of magnetically coupled coils."

IEEE transactions on biomedical engineering 43: 708-

714.

F.C Flack, E. D. J., D. M. Schlapp (1971). "Mutual

inductance of air-cored coils: Effects on design of

radio-frequency coupled implants." Medical and

Biological Engineering 9: 79-85.

Hochmair, E. S. (1984). "System optimization for

improved accuracy in transcutaneous signal and power

transmission." IEEE transactions on biomedical

engineering 31: 177-186.

John C. Schuder, J. H. G., Hugh E. Stephenson Jr (1971).

"An inductively coupled RF system for the

transmission of 1kW of power through the skin." IEEE

transactions on biomedical engineering 18: 265-273.

Mani Soma, D. C. G., Robert L. White (1985). "Radio-

frequency coils in implantable devices: Misalignment

analysis and design procedure." IEEE transactions on

biomedical engineering 34: 276-282.

N. de N. Donaldson, T. A. P. (1983). "Analysis of

resonant coupled coils in the design of radio frequency

transcutaneous links." Medical and Biological

Engineering and computing 21: 612-627.

Ping Si, P. A. H., J. W. Hsu, M. Chiang, Y. Wang, Simon

Malpas, David Budgett (2007). "Wireless power

supply for implantable biomedical device based on

primary input voltage reglation." 2nd IEEE conference

on Industrial Electronics and Applications.

MAGNETIC COUPLING ANALYSIS OF A TET POWER DELIVERY SYSTEM

175