ECG SIGNAL DENOISING

Using Wavelet in Besov Spaces

Shi Zhao, Yiding Wang

Graduate University of Chinese Academy of Sciences, Zhongguancun East Road, Beijing, China

Hong Yang

Graduate University of Chinese Academy of Sciences, Zhongguancun East Road, Beijing, China

Keywords: ECG, noise reduction, wavelet, Besov, nonlinear shrinkage function.

Abstract: This paper proposes a novel technique to eliminate the noise in practical electrocardiogram (ECG) signals.

Two state-of-the-art denoising techniques, which both based on wavelet bases, are combined together. The

first one is discussing wavelet bases in Besov spaces. Compared to traditional algorithms, which discuss

wavelets in

()

R spaces, the proposed technique projects ECG signals onto Besov spaces for the first time.

Besov space is a more sophisticated smoothness space. Determining the threshold of shrinkage function in

Besov space could eliminate Gibbs phenomenon. In addition, instead of using linear shrinkage function, the

proposed algorithm uses nonlinear hyper shrinkage function, which is proposed by Poornachandra. The

function tends to keep a few larger coefficients representing the function while the noise coefficients tend to

be reduced to zero. Combining the two techniques, we obtain a significant improvement over conventional

ECG denoising algorithm.

1 INTRODUCTION

Removing noise is an pertinent problem in ECG

signals processing. Usually, there are two kinds of

noises in ECG, power line frequency noise and

white noise. Power line frequency noise can be

regarded as the result of an electromagnetic

compatibility issues: background electromagnetic

field interference from surrounding equipments and

from buldings and power conductors. White noise is

usually considered from the measure equipment.

Previously, different filters based on Fourier

bases are used to eliminate the noises, such as notch

filter. The problem of these methods is that they

could not reduce the two kinds of noises at the same

time. In addition, because the notch has a relatively

large bandwidth, which means that the other

frequency components around the desired null are

severely attenuated, this method brings in signal

distortions. In 1995, Donoho (David L Donoho,

1995) proposed a novel denoising algorithm based

on wavelet shrinkage. It provides excellent

performance and since then, wavelets became a

state-of-the-art denoising method. Before long, P. M

Agante (P M Agante, 1995) applied soft-threshold

method in ECG and achieve good results. However,

traditional wavelet method has its drawbacks. They

are not shift invariant; therefore, for the signals not

smooth enough, it will appear Gibbs Oscillation

phenomenon at the location where the signal is sharp

changed. In ECG signals, there are R waves, which

change sharply. As a result, Traditional wavelet

denoising algorithm brings in Gibbs oscillation after

R waves.

In this paper, we apply two techniques to

eliminate the noise and restrain the Gibbs

phenomenon at the same time. First, we determine

the threshold of wavelet shrinkage function in Besov

spaces. Besov space

()

is a smoothness space

with

> ,

(,) [1, )pq

∈

+∞ , it is defined by

()

(){ ()| }

BL f LR f

∈<∞

(1)

Where the Besov seminorm

()



is linked to the

smoothness modulus of the considered function.

Besides that, in stead of linear shrinkage function,

we use nonlinear shrinkage model (S.

Poornachandra, 2007). Combining the two novel

250

Zhao S., Wang Y. and Yang H. (2008).

ECG SIGNAL DENOISING - Using Wavelet in Besov Spaces.

In Proceedings of the First International Conference on Bio-inspired Systems and Signal Processing, pages 250-254

DOI: 10.5220/0001058302500254

 SciTePress

techniques, we obtain a significant improvement

over conventional wavelet denoising algorithm. In

order to certify our idea, the noises in ECG signals

in our experiment are not added by hand. They are

from actual interfering. We collect the ECG signals

with noises by our own devices.

2 INTRODUCTION TO WAVELET

SHRINKAGE FUNCTION IN

BESOV SPACE

Wavelet is defined as orthonormal basis functions

for the expansion of functions belonging to various

function spaces. Usually, it is the space of squared

integrable real functions

()

R (functions with finite

energy). Recently, it has been shown that more

sophisticated smoothness spaces, such as Besov

spaces, provide a suitable and more refined

characterization of real-life signals (Kathrin

Berkner, 2000). The wavelet series representation of

a function

() ( )

tLR∈ could be express as

21 21

() () ()

jk k jk jk

kZ j j kZ

tct t

ϕψ

−∞−

∈=∈

∑∑∑

(2)

is called farther wavelet and

is called mother

wavelet.

()

and

()

are the dilation and

translation of the wavelet function.

() 2 (2 )

ϕϕ

=−

(3)

() 2 (2 )

ψψ

=−

(4)

, k are the scaling and translation parameters

respectively,

,jk Z∈

could maintain the unity

norm of the basis function at various scales. The

coefficients

kjk

= and ,

kjk

= . Often

we set

0j =

, and in that case there is only one

scaling coefficient. The wavelet series are usually

discussed in

()

R spaces, but in our research, we

use a more sophisticated set of functions, Besov

spaces

()

( 0

<<∞, 0 p<≤∞, 0 q<≤∞). In

Besov spaces, for a function

()

∈ , its norm

could be defined using its wavelet coefficients as (5)

(Kathrin Berkner , 2000)

(/21)

()

|| || | | 2 | |

pjppp

jk jk

kjjk

fc d

+−

⎛⎞

⎛⎞⎛ ⎞

⎜⎟

⎜⎟⎜ ⎟

⎜⎟

⎝⎠⎝ ⎠

⎝⎠

∑∑∑

(5)

The three Besov parameters have natural

interpretations: a

-norm of the wavelet

coefficients is taken within each scale

, a weighted

q -norm is taken across scale, and the smoothness

parameter

controls the rate of decay of the

d ,

increasing

corresponds to increasing smoothness.

Based on reference (Antonin Chambolle, 1998),

the denoising problem could be described as follow.

Given a positive parameter

and a signal

, find a

function

that minimize over all possible function

the functional

()

|| || || ||

fff

+−

(6)

Choose a proper

, the

could be the denoising

signal of

. For simpleness, we set Besov

parameters

1pq

= . Then the problem could be

expressed as follow:

()

(1/2) 0

,,,

min 2 | |

jk jk jk

jk jk

ddd

−

+−

∑∑

(7)

That means for each

, k , we estimate the d

)

use follow expression:

2(1/2)

(1/2)

()2 ||

()max(| |2 /)

arg min

sign d d

−

−+

=⋅ −

)

(8)

That means the ECG signal has small Besov

norm if the wavelet coefficient in each scale have

small

l norms and those

l norms decay rapidly

across scale.

Note that any wavelet basis having

vanishing moments can be used to measure a Besov

norm (Hyeokho Choi, 2004).

3 INTRODUCTION TO

NONLINEAR SHRINKAGE

MODEL

Donoho and Johnstone were first to formalize the

wavelet coefficient thresholding for removal of

additive noise from deterministic signals (David L

Donoho, 1995). Wavelet thresholding is based on

the property that typical real-world signals have

sparse representations in the wavelet domain. The

small coefficients are usually correlated to noise.

Therefore, by choosing an orthogonal basis, which

could efficiently approximates the signal with few

nonzero coefficients; we could choose a particular

threshold and set the coefficient bellow the threshold

to zero. Using these coefficients in an IDWT to

reconstruct the data, we could kill the noise.

ECG SIGNAL DENOISING - Using Wavelet in Besov Spaces

251

The shrinkage function proposed by Donoho and

Johnstone are the hard and the soft shrinkage

function. Hard thresholding simply sets the

coefficients below a threshold T to zero, as (9). Soft

thresholding first shrinks each coefficient by

T and

then hard thresholds, as (10).

()

≤

(9)

(

)

( ) sgn( ) | |

xxT

=−

(10)

Both hard and soft shrinkages have their

disadvantages. Due to the discontinuities of the

shrinkage function, hard shrinkage estimate tends to

have bigger variance and can be unstable, that is,

sensitive to small changes in the data. The soft

shrinkage estimate tends to have bigger bias, due to

the shrinkage of large coefficients (S.

Poornachandra, 2007).

To overcome the drawbacks of hard and soft

shrinkage, we decide to use nonlinear shrinkage

function. There are two kinds nonlinear shrinkage

estimate in our experiment. The first is called

nonnegative garrote shrinkage function (M. Vetterli,

1995), which was first introduced by Breiman

(1995) as follow:

() 1 ( / )

xx x

δλ

⎡⎤

=−

⎣⎦

(11)

The shrinkage function

()

is continuous and

it provides a good compromise between the hard and

the soft shrinkage functions. It is less sensitive than

hard shrinkage to small fluctuations and less biased

than soft shrinkage. The second shrinkage function

is called hyper shrinkage, which is proposed by S.

Poornachandra as follow:

(

)

( ) tanh( * ) | |

hyp

xxt

δρ

=−

(12)

The major advantage of hyper shrinkage is its

nonlinearity, that is, the function in wavelet domain

tends to keep a few larger coefficients representing

the function while the noise coefficient tend to be

reduced to zero.

4 NOISE REDUCTION BY OUR

METHOD

The objective of this paper is to eliminate the noise

buried in practical ECG signals. In our research, we

combine the two techniques we mention above.

First, we determined the threshold of shrinkage

function for each level in Besov spaces. It is

obviously that for each subband, the parameter

should be different. We set

for each level

experimentally. Then we use the two kinds of

nonlinear shrinkage functions to obtain the estimated

coefficients. Finally, using these coefficients the

original ECG signal is thus recovered. The general

process is showed bellow. The decomposition level

is 6.

Step 1. Choose db3 wavelets, and do DWT.

Step 2. Choose

at each level. For the fist level

0.9

, and

0.25* (log( 2))

sqrt j

for each

level.

Step 3. Determine the threshold based on the

Step 4. Apply hyper shrinkage function and the

estimated coefficients obtained.

Step 5. IDWT use the estimated coefficients.

5 SIMULATIONS AND RESULTS

In our research, the ECG signals are obtained by our

own devices. Each piece of signal is about 1 min

long. The sampling rate is 1200Hz.

In our research, we use five different denoising

methods. We show original signal and the processed

4 signals and their spectrums in Fig.1 to Fig.6. In

order to see clearly, we show their details of the

sample points around R waves. The method in Fig.2

determines the threshold in

()

R spaces and use

hard thresholding shrinkage function, while in Fig.3

the thresholds is determined in

()

R spaces and use

soft thresholding method. The other three discuss the

thresholds in Besov spaces. Whereas Fig.4 uses soft

shrinkage function, Fig.5 use nonnegative garrote

shrinkage function and the last one uses hyper

shrinkage function.

Figure 1: The original signal and its spectrum.

BIOSIGNALS 2008 - International Conference on Bio-inspired Systems and Signal Processing

252

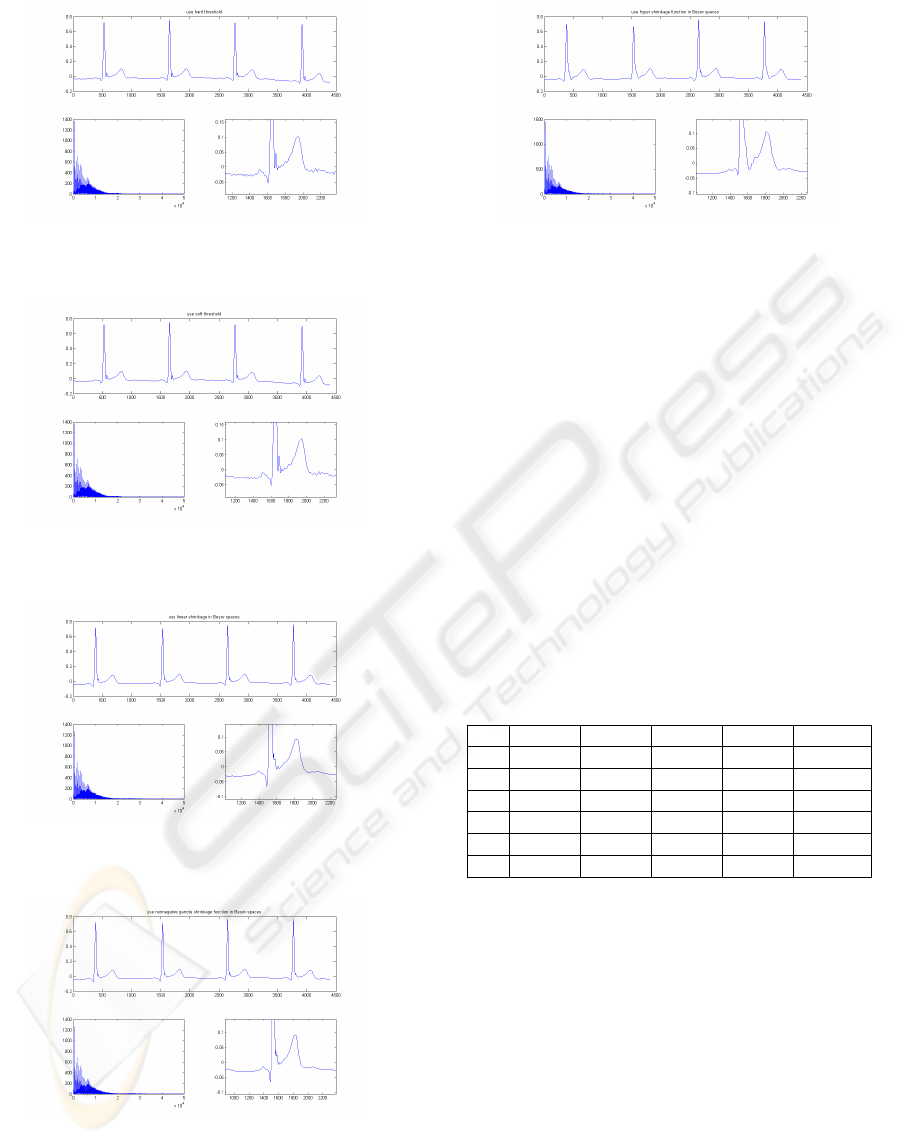
Figure 2: Determine the threshold in

()

R and use hard

thresholding.

Figure 3: Determine the threshold in

()

R and use soft

thresholding.

Figure 4: Determine the threshold in Besov spaces and use

soft thresholding.

Figure 5: Determine the threshold in Besov spaces and use

nonnegative garrote shrinkage function.

Figure 6: Determine the threshold in Besov spaces and use

hyper shrinkage function.

As we seen from the pictures above, combined

with threshold determined in Besov spaces and

hyper shrinkage function, the recovered signal is the

most visually pleasant. The proposed technique

almost eliminate Gibbs phenomenon. To describe

the oscillation of the recovered signal

quantificational, we calculate the total variation of

the six signals. Total variation for a uniform

sampling discrete signal

is defined as (S. Mallat,

1998).

|| || | [ ] [ 1]|

NV N N

ffnfn

−−

∑

(13)

Where

|| ||

f is the Total Variation. In order to

certify the effectiveness of the proposed method, we

give 4 pieces of signals’ Total Variation. They are

show in Table 1.

Table 1: Total Variation of the signals.

1 2 3 4 average

T1 0.3914 0.3747 0.3801 0.3875 0.3834

T2 0.1789 0.1654 0.1388 0.1252 0.1521

T3 0.1789 0.1654 0.1388 0.1252 0.1521

T4 0.1721 0.1517 0.1006 0.1177 0.1355

T5 0.1758 0.1504 0.1030 0.1206 0.1374

T6 0.1431 0.1371 0.0803 0.0919 0.1131

In the above table, T1 means the original signals’

Total Variation. T2 to T6 correspond Fig.2 to Fig.5.

In the table, we could notice easily that discussing

threshold in Besov space and using nonlinear

shrinkage function could obtain good results. And

among those, hyper shrinkage is the most effective,

it has the least oscillation.

6 CONCLUSIONS

This paper proposes a novel approach to eliminate

the noises in practical ECG Signals. First, we use the

characterization of Besov space, which is a

smoothness spaces, through wavelet

ECG SIGNAL DENOISING - Using Wavelet in Besov Spaces

253

decompositions. Then we apply nonlinear shrinkage

function instead of linear shrinkage function. The

experiment results show that the proposed algorithm

is visually pleasant compared to traditional methods.

It could eliminate the noise successfully, and at the

same time, it suppresses Gibbs oscillation. The

proposed technique has potential application in data

acquisition systems, which are generally

encountered by noise.

ACKNOWLEDGEMENTS

This research is supported by High Technology

Research and Development Program of China (863

Program): 2006AA01Z133. The ECG signals

collection device is designed by Shen Yadong, who

is a graduate student in Tsinghua University, China.

REFERENCES

S. Poornachandra, N. Kumaravel, 2007. A novel method

for the elimination of power line frequency in ECG

signal using hyper shrinkage function. Digital Signal

Process, doi:10.1016/j.dsp.2007.03.011.

S. Mallat, 1998. A Wavelet Tour of Signal Processing.

Academic Press. San Diego, 2

edition.

David L Donoho, 1995. De-noising by soft thresholding.

IEEE Transactions on Information Theory, 41(3): 613-627.

P M Agante, J P Marques de Sa, 1995. ECG noise filtering

using wavelets with soft-threshold method. IEEE

Computers in Cardiology, 26:535-538.

M. Vetterli, J. Kovacevic, 1995. Wavelet and Subband

Coding. Prentice Hall International, Englewood Cliffs,

NJ.

Kathrin Berkner, Michael J. Gormish, Edward L.

Schwartz, and Martin Boliek, 2000. A new wavelet-

based approach to sharpening and smoothing of

images in Besov spaces with applications to

deblurring. Proceedings. 2000 International

Conference on Image Processing, Vol 3: 10-13

Hyeokho Choi, Richard G. Baraniuk, 2004. Multiple

wavelet basis image denoising using Besov ball

projections. IEEE signal processing letters, Vol. 11.

NO.9.

D. Leporini, J. C. Pesquet, 2000. Bayesian wavelet

denoising: Besov priors and non-Gaussian noises.

Elsevier Science Signal Processing,81: 55-67.

Alexandre Almeida, 2004. Wavelet bases in generalized

Besov spaces. Elsevier mathematical analysis and

applications, Appl.304: 198-211.

Antonin Chambolle, Ronald A. DeVore, Nam-yong Lee,

and Bradley J. Lucier, 1998. Nonlinear wavelet image

processing: variational problems, compression, and

noise removal through wavelet shrinkage. IEEE

Transactions on image processing, Vol. 7, NO.3.

BIOSIGNALS 2008 - International Conference on Bio-inspired Systems and Signal Processing

254