MATHEMATICAL MODELLING OF THERMAL AREA

IN CUTTING TOOL

Daschievici Luiza, Ghelase Daniela and Goanta Adrian

Dunarea de Jos University Galati, Faculty of Engineering from Braila, Calea Calarasilor, nr. 29, Braila, Romania

Keywords: Cuttings, friction, heating, wearing.

Abstract: Since experimental researches regarding cutting process have stated a proportionality dependence of wear

medium intensity on cutting area temperature and because this fact was avoid or ignored by thorough

studies and researches, we considered to be helpful developing a physical-mathematical model able to

correlate the two phenomena: wear and temperature in the cutting area. The complete and correct research

on thermal phenomena in the cutting area is possible only by taking into consideration the feed-back

relation between the physical and phenomenological elements of the studied tribosystem and also, by taking

into account the splinter movement, resulting in a continuous supplying with cold layers of the splinter area

and in heat evacuating by warm splinter movement.

1 RESEARCH ON METAL

CUTTING, SPECIFIC

PROCESSES

AND PHENOMENA

Metal cutting, due to the action of tool blade pushed

on the processed material, leads to a complex status

of forces and deformations in the cutting area.

This simple diagram is the base for any detailed

study on cutting process. It is a basic physical

model, completing other specific models of elasticity

and plasticity, thermodynamics, tribology, thus

forming a complex model, more or less detailed

according to requirements or claims. For almost 100

years of researching on cutting process, many

theoretical and experimental data have been

gathered, but they are far beyond from being a

unitary whole.

The present paper develops on this quite

applicable, enough explored, but still insufficiently

understood field of application.

Based on the knowledge, the definition of the

splinter appearance process has been stated,

containing also the essence of interdisciplinary

aspects.

The essence of these aspects is already shown in

the specialty literature, in detail, and this paper deals

only on those aspects considered able to be

improved.

The analysis of some definite aspects solving

manner presented in the specialty literature led to the

conclusion that errors have been done, errors able to

stray from the real status.

The main phenomena and processes

accompanying the cuting process are friction,

tribosystem heating and tool wear. The tribosystem,

consisting of tool, processed part and splinter, can be

considered as a system with direct contact, where no

lubricating film is present. Based on his own

researches and taking into account some results of

his predecessors, Coulomb stated the three dry

friction rules, which are often partially confirmed. It

can be reminded that friction depends on the relative

speed, this fact being experimentally ascertained at

the beginning of the XIX century, as shown in figure

1 (Tomulescu, 2000).

The experimental researches dawn up so far led

to the conclusion that dry friction force depends on

many factors such as: the normal pressure force, the

relative sliding speed, the type of the contact and the

value of the contact area, the quality and the

roughness of the surface, the nature of materials in

contact, the character of the friction areas: rigid or

elastic, tenacious or fragile. Even human and

materials resources were highly implied in research,

the complex friction phenomenon did not allow a

universal valid theory elaboration, for at least from

the quality point of view.

275

Luiza D., Daniela G. and Adrian G. (2008).

MATHEMATICAL MODELLING OF THERMAL AREA IN CUTTING TOOL.

In Proceedings of the Fifth International Conference on Informatics in Control, Automation and Robotics - ICSO, pages 275-280

DOI: 10.5220/0001478902750280

 SciTePress

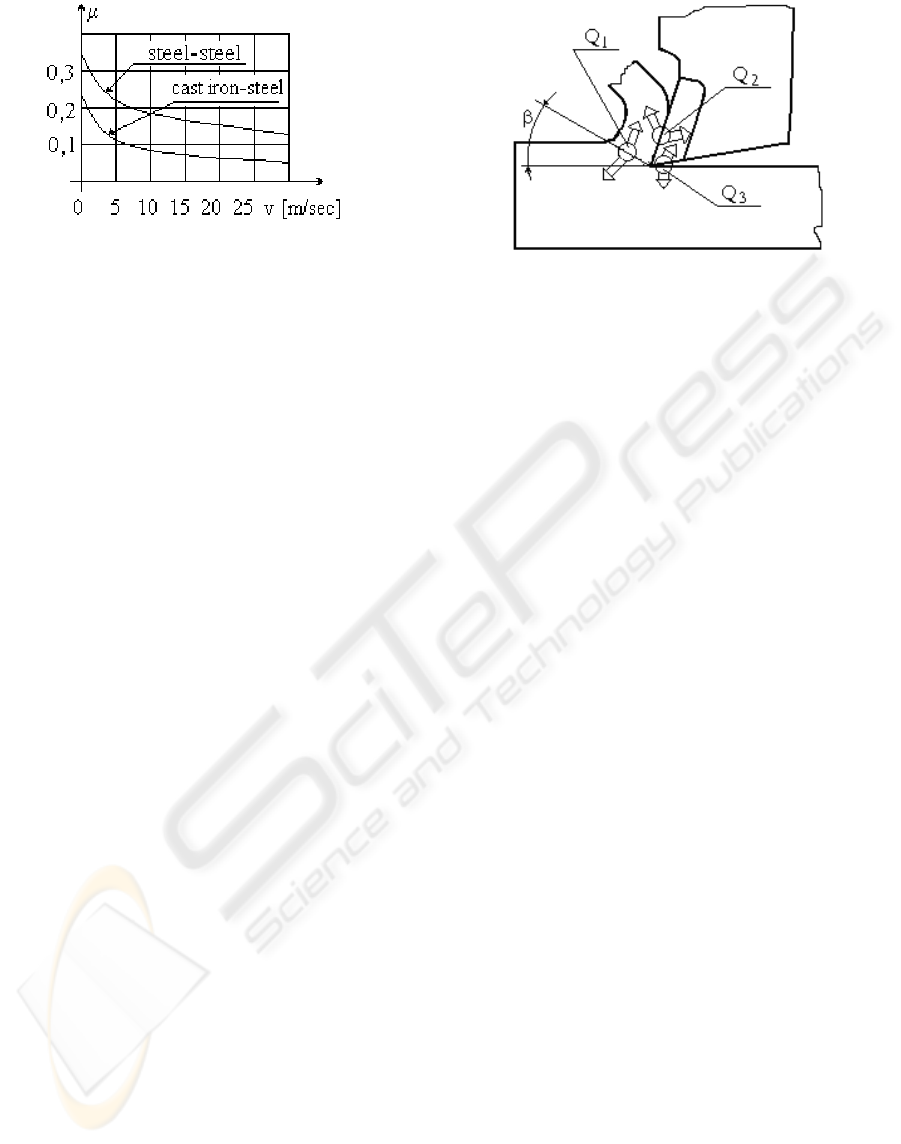
Figure 1: The variation of friction coefficient on speed.

Taking into account the prevalent role of friction on

tribosystem parts heating, thus influencing the

cutting tool wear, one considered necessary to do a

research on he friction coefficient for a specific

cutting case and highlight the fact that the friction

coefficient is a non-coulombian one (Tomulescu,

1999; Tomulescu, 2003).

As for the dry or lubricated cutting friction, its

framing in the dry friction category is based on the

finding that the cooling fluids do not enter into the

friction area.

The researches shown that the mechanical work

necessary for volume plastic deformation in the cut

metal layer is partially transformed into caloric

energy that heats the cutting area; this process

depends on the thermal conductivity of the cut metal

and on the working conditions of cutting process.

The mechanical work consumed in the cutting

process can be expressed by the next equation

(Dumitras, 1983):

L=L

+ L

(1)

where:

, L

and L

are parts of the mechanical work

transforming into heat during cutting and dispersed

in the environment through the splinter, the tool and

the processed part;

, L

and L

are parts of mechanical work,

consumed in the cutting process, with little values

comparative with L

, L

The mechanical work consumed in the cutting

process, entirely transformed in heat, L

, L

and L

components, generates the heating sources Q

, Q

and Q

, fig. 2, ordered by their intensity, as follows:

In fact, depending on the cutting process,

different heat exchanges may develop between the

processed part, the splinter and the tool, depending

on the cutting process nature, so that the

temperatures in the splinter, the tool and the

processed part being not abided by the distribution

of sources intensities.

Figure 2: Mechanical work heat dispersing.

The factors influencing the heat generated by the

cutting process are:

- the physical and mechanical characteristics of

cut metal

- the cutting tool material

- the geometrical parameters of the tool

- the parameters of the cutting conditions

Since the blade temperature is the main wear-

influencing factor, the researches went towards

finding the empiric relations between blade

temperature and the main cutting tool elements, less

interest being paid to the aspect of heat quantities

evaluation.

The blade wear behaviour depends in a large

measure on its temperature. The experimental

researches performed so far show that there is some

proportionality between wear intensity and blade

tool temperature, the variation rules demonstrating

obvious parallelisms. That is why, in order to

accurately evaluate wear intensity (and wear

evolution) depending on cutting parameters, it is

necessary to know the cutting temperature, including

the tool blade temperature, depending on the cutting

parameters.

Based on the researches performed, a series of

dependence relations between the tribosystem

elements temperatures have been defined.

The mathematic model shown by the specialty

literature suits to an uniform stationary thermal

status, respective to the same temperature in the

entire splinter and tool blade and time constant, quite

far from reality even for a qualitative and

phenomenological analysis (Dumitras, 1983).

The hypothesis taken into consideration,

according to which heating in the cutting area is

uniform and stationary can be appreciated as a

particular case that cannot be real.

That is why a specific model is required for the

heating sources, able to lead to a correct

ICINCO 2008 - International Conference on Informatics in Control, Automation and Robotics

276

determination of real thermal status, non-stationary

and non-uniformly distributed, that, in time, leads to

a stationary and non-uniformly distributed thermal

status, depending on the influencing factors, the

most important of them being as follows:

- the cutting process parameters;

- the physical and mechanical properties of the

tool blade and splinter;

- the environmental heat exchange;

- the feed-back interdependence between

different influencing factors;

- the cutting process dynamic phenomena.

Cutting wear comes up because of interfering factors

effects and it is important to be known, especially

regarding the cutting tool.

Tool wear is progressive and it manifests under

many aspects (temperature increasing, processed

area deterioration, cutting forces increasing), finally

leading to their stop functioning.

The researches highlighted more blade wear

types, as shown in figure 3 that represents these

wear influences and makes possible an appreciation

of their weight as part of total wear. The diagram

shows that the abrasive wear has the highest

influence; it is determined by the friction conditions

of areas in contact: tool - processed part-splinter.

Figure 3: The influences of partial wear on total wear.

The splinter temperature increases due to the energy

exclusively obtained from friction: the friction

between the splinter and the tool and the friction

between inter- and intracrystals that comes up during

splinter formation and separation. The higher the

temperature the more plastic is the splinter, some of

its areas pass to the liquid phase, the intensity of the

above mentioned frictions decreases, smaller

amounts of energy are freed, splinter temperature

decreases, the splinter is more solid, more intense

frictions develop tending to increase the

temperature, and so on. Therefore, a combination of

effects with opposite tendencies takes place leading

to a splinter temperature, which is not equal to the

melting temperature of the processed part, but an

equilibrium temperature beyond the melting one.

Speed increasing, especially for high cutting speed,

leads to a feedback chain, according to figure 4.

Figure 4: The feedback influence of temperature

increasing on the mechanical characteristics of the

processed material.

According to this chain, cutting speed increasing

leads to cutting area temperatures increasing, the

effect is a deformation resistance and mechanical

work decreasing, thus implying wear reducing and a

higher tool durability.

The analysis of cutting conditions influence on

the tool temperature and tool wear leads to the

conclusion that the variation of wear medium

intensity is very similar to the variation of the

temperature on the tool.

The main objective of the present paper is to

combine in a single model the dynamic, thermal and

tribologic phenomena, in order to evaluate in

advance the wear intensity and to find out the way to

influence it.

2 THE RESEARCH

METHODOLOGY AND THE

UTILIZED MANNERS

Among the considered objectives, there can be

mentioned:

- the research of dynamic phenomena;

- the research of thermal and wear phenomena.

It was necessary to develop a physical model of the

phenomenon in order to perform the researches; in

fact, the phenomenon is a conventional image of the

real status, representing the basics of mathematical

modelling. The model has mathematical equations,

functionally describing the physical model, and

through it, the real phenomenon. For mathematical

model solving, difficult to be analytically solved,

there have been used numerical methods to obtain

solutions for the differential equations (Tomulescu,

2000).

The stages covered for phenomenon modelling

are, as follows:

- cutting area forces modelling, based on

Merchant model for free orthogonal cutting, where

is taken into consideration the fact that the splinter is

MATHEMATICAL MODELLING OF THERMAL AREA IN CUTTING TOOL

277

balanced by two categories of external and internal

forces (Tomulescu, 2000);

- Heating Sources Modelling, figure 2;

It is thought that the heating source consists

both by the non-conservative mechanical work

wasted by plastic deformation in the cutting plane

area and by the non-conservative mechanical work

from the friction on the escaping and on the laying

tool area.

- Heat Dispersing Modelling. The heat disperses

into a non-homogeneous environment consisting of

splinter, blade and tool body, each having different

caloric coefficients, both as value and temperature

dependence.

Solving the problem of heat dispersing under

transitory conditions and in a heterogeneous

environment leads to temperature knowing for every

moment and in each point of the considered

environment.

The theoretical study program, including the

above-mentioned models, materialized in a very

complex physical and mathematical computer

model, enables the researching of the influence of

different factors, such as:

- the parameters of the cutting conditions (speed,

advance, depth);

- tool blade material;

- the cutting manner (continuous or interrupted).

As previously shown, the friction in the cutting area

takes place under very particular conditions, such as

high pressures, relative high-speed values, no

lubrication. The bibliographical research shows that

coulombian friction is an exaggerated approximation

of dry friction.

As long as a realistic mathematical model is

desired, the friction model for the cutting area

should have a friction coefficient depending on

speed for the couple splinter-blade.

By mathematical modelling, the differential

equation for heat conducting is:

⎟

⎠

⎞

⎜

⎝

⎛

∂

θ∂

∂

θ∂

∂

θ∂

⋅λ=

∂

∂θ

⋅⋅ρ

zyx

(2)

that for an anisotropic and non-homogeneous

material generally turns to:

()

⎟

⎠

⎞

⎜

⎝

⎛

∂

∂θ

⋅λ

∂

⎟

⎠

⎞

⎜

⎝

⎛

∂

∂θ

⋅λ

∂

⎟

⎠

⎞

⎜

⎝

⎛

∂

∂θ

⋅λ

∂

=θ⋅⋅ρ

∂

(3)

where:

- material density (kg/m

);

c - material specific heating (J/kgK);

- material thermal conductivity (W/mK).

The integration of the differential equation (3) is

analytically difficult to solve, and the specialty

literature does not offer exact solutions for each

practical case. To obtain an analytical result, the

following solution is used:

(

)

(

)( )

z,y,xFtTz,y,x,t

⋅

(4)

replacing it in heat equation (3) leads to:

()()

()

⎥

⎦

⎤

⎢

⎣

⎡

∂

⋅λ+

∂

⋅λ⋅+

⎥

⎦

⎤

⎢

⎣

⎡

∂

⋅λ⋅=⋅

∂

⋅⋅ρ

z,y,xF

tTz,y,xF

(5)

Heat exchanges inside and on the areas of the

tribosystem the mathematic model, knowing the

border conditions, extremely difficult to be

analytically described, materializes elements and

that is why numerical integration is preferred, the

most suited being the finite differences method.

The friction coefficient used by the computing

program was experimentally determined by using

energetic methods.

The results obtained for the friction coefficient

clearly led to the conclusion that, in this case, the

friction is non-coulombian (Tomulescu, 2003). Its

dependence on speed is shown in figure 5.

Figure 5: The variation of friction coefficient on relative

speed.

The present paper also uses the results obtained by a

classical research regarding tool wear, results taken

out from a research project performed for

manufacturing assimilation of metallic carbide

cutting plates. These results were synthesized in

wearing diagrams, VB = f(T), as shown in figure 6,

for some specific processing cases. These wear

curves, continuous in time, enabled the study of

ICINCO 2008 - International Conference on Informatics in Control, Automation and Robotics

278

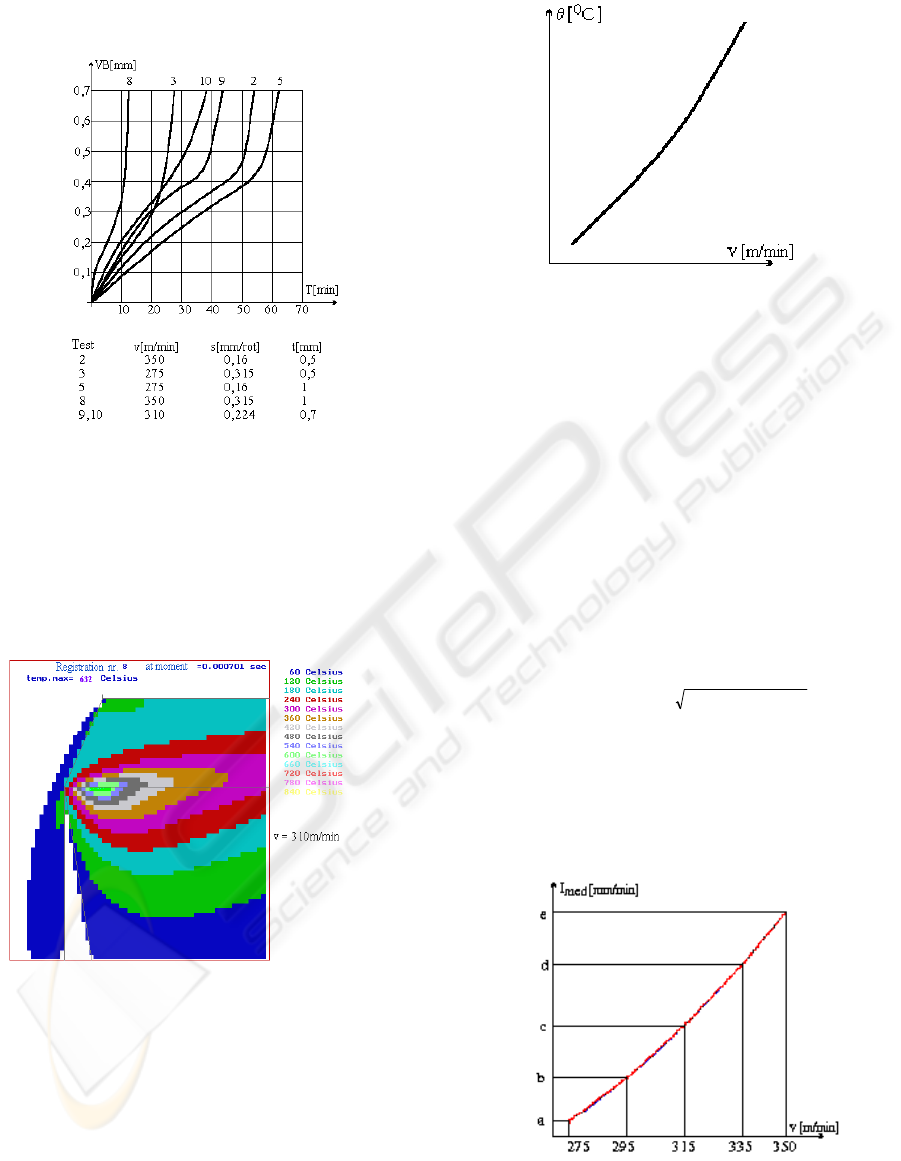
wear evolution correlated to the suggested

mathematical modelling.

Figure 6: VB wear of TNGG 22.04.12/P10 plates for steel

lathing 8550/97HB.

Taking into account the fact that the speed mostly

determines process thermal status, with implications

on cutting tool wear, it was considered necessary to

be analyzed for the heating process, especially on

the laying area, in order to diminish the implications

and wear reducing.

Figure 7: Thermal status in splinter-tool tribosystem.

Figure 8: The variation of temperature on cutting speed.

By solving the mathematical model using the

specialized developed program, thermal areas are

obtained, as shown in figure 7; when analyzing for

different cutting processes, with the required

parameters v, s and t, appreciations on wear and

durability of cutting tools can be stated. The

obtained data can be used to trace a curve for

temperature dependence on speed, as shown in

figure 8 and the mathematic relation is:

v,v,

max

⋅⋅+⋅−=θ

−3

107143821001

(6)

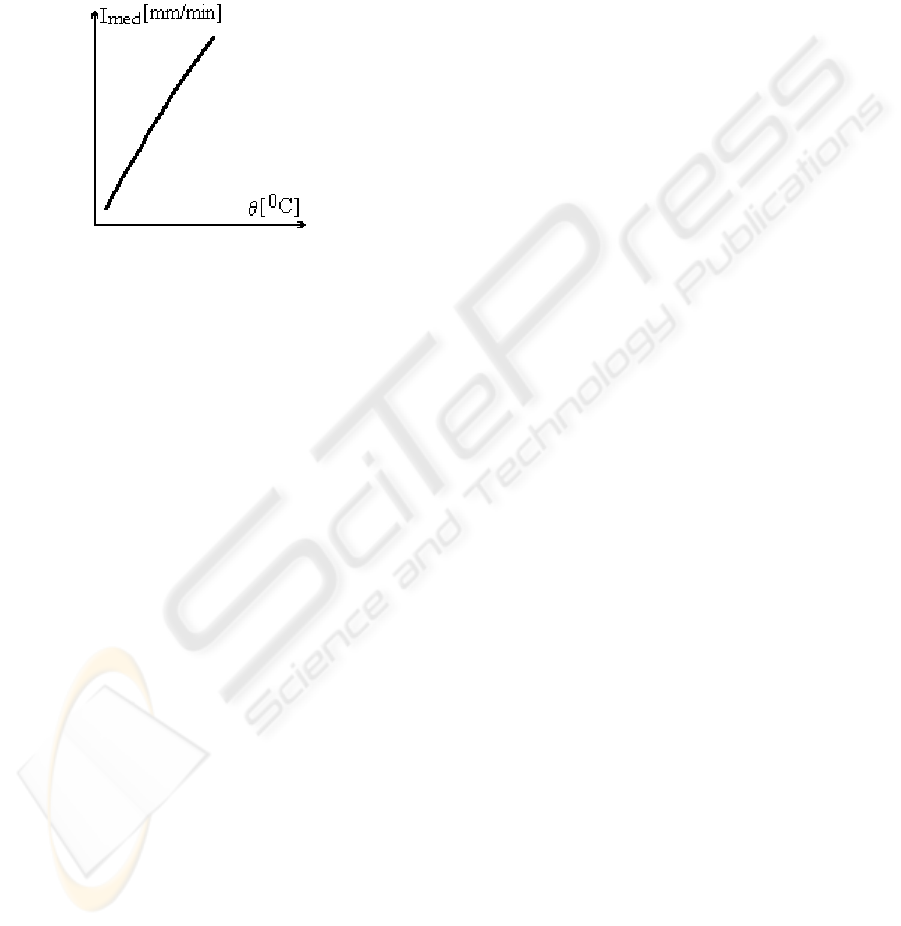
Notice the similitude between θ°[C] = f(v) curve and

med

=f(v) curve, figure 9; a direct relation

med

=f(θ°C) for case (P10) can be stated, as shown in

figure 10 and mathematic relation proposed is:

θ⋅⋅+

+−θ⋅⋅⋅+−=

−

1076639

614870531212102438706340

,,,,I

med

(7)

Diagram analysis concludes that there is a good

proportionality of wear medium intensity with the

maximum temperature of cutting process, an

experimentally stated fact, also presented by the

specialty literature.

Figure 9: The variation of medium intensity on maximum

temperature.

MATHEMATICAL MODELLING OF THERMAL AREA IN CUTTING TOOL

279

Experimental and theoretical researches

regarding tool blade temperature and wear medium

intensity settled up similitude between the

evolutions of the two phenomena, leading to the

conclusion that thermal phenomena evolution

modelling enables the evolution of wear medium

intensity, by applying a constant of proportionality,

experimentally known, which remains the same for a

couple splinter-blade.

Figure 10: The variation of medium intensity on maximum

temperature.

3 CONCLUSIONS

Based on the performed studies, a series of

conclusions have been stated, among which are, as

follows:

- the most important thermal sources, such as the

source created by plastic deformations in the cutting

plane and the source created by the friction between

the splinter and the tool blade escaping area, have

intensities and distributions depending on the values

of the cutting conditions parameters and on the

splinter-blade couple; they heat the cutting area to

temperatures non-homogeneous distributed, and the

temperatures influences those materials constants

related to heating sources intensity;

- thermal status in the cutting area is

characterized by a maximum in the splinter pressure

center on the escaping area, as long as, in the wear

area (the laying area) the temperature is much lower;

- it has been stated a dependence relation

between a parameter characteristic tool blade wear,

such as wear medium intensity, and cutting area

temperature; the relation is, with pretty small

deviations, a directly proportional dependence one;

thus, by measuring the tribosystem temperature, the

wear medium intensity can be evaluated, at least for

the cutting process.

REFERENCES

Dumitras, C., Militaru, L., 1983. Aschierea metalelor si

fiabilitatea sculelor aschietoare.

Ed. Tehnica,

Bucuresti.

L. Tomulescu, C. Falticeanu, Gh. Oproescu, 1999.

“Dynamic and thermal aspects of tribologic processes

between tool blade and splinter during splitting”

Balkantrib’99, The 3

International Conference of

Tribology, Sinaia,, ISSN 1221 - 9371, pg.233- 240.

Tomulescu, L., Ghelase, D., 2003. Experimental results

obtained by friction process researching in processed

part – tool tribosystem in cutting area. International

Science and Engineering Conference Machine -

Building and Technosphere of the XXI Century

Sevastopol, Ukraine, ISBN 966-7907-12-0, pg. 267 –

271

Tomulescu, L., 2000. Tribologia taisului de scula

aschietoare.

CEPROHART, Braila.

ICINCO 2008 - International Conference on Informatics in Control, Automation and Robotics

280