SYNTHESIS

METHOD OF A PN CONTROLLER USING

FORBIDDEN TRANSITIONS SEQUENCES

R. Bekrar, N. Messai, N. Essounbouli, A. Hamzaoui and B. Riera

CReSTIC, IUT de Troyes, 9 rue de Qubec BP 396, 10026 Troyes Cedex, France

Keywords:

Forbidden state problem, Forbidden transitions sequences, Supervisory control, Discrete event systems, Petri

Nets.

Abstract:

In this paper, we propose a control synthesis method for Discrete Event Systems (DES) modelled by a bounded

ordinary Petri Nets (PN) to treat a forbidden state problem. The considered PN is transitions controllable

and contains measurable and non measurable places. The PN controller is synthesised using the forbidden

transitions sequences. The latter, are deduced from the PN reachability graph and considered as a forbidden

language generated by the PN model. To show the efﬁciency of the proposed method an illustrative example

is presented.

1 INTRODUCTION

In Discrete Event Systems (DES), it is important

to prevent the system to reach undesirable states.

Hence, given a DES model and a speciﬁcation of

the desired behaviours, one must to synthesise an

efﬁcient controller in order to achieve this goal.

Among tools used to synthesis a DES controller, Petri

Nets (PN) have been successfully considered as an

efﬁcient formalism for DES control.

This paper is considered as the second phase of

our work (Bekrar et al., 2006a; Bekrar et al., 2006b)

on the identiﬁcation of a DES. It allows to complete

the identiﬁed model by adding control places. Indeed,

the identiﬁed PN model can generate all the DES

states. However, it can generates, sometimes, some

states which are not observed during the system

functioning. Thus, our objective is to synthesise a PN

controller to prevent the reachability of the forbidden

states and guarantees the desired ones.

The synthesis problem of PN controllers has been

widely treated in the literature. It was introduced

either as a forbidden states problem (FSP) or a

forbidden state transitions problem (FSTP). The

synthesis methods of a PN controller allow to add a

set of control places to the initial model in order to

generate the desired behaviours. Several approaches

are suggested in order to solve this problem. Among

them we can ﬁnd, the logical predicate based solu-

tions (Krogh and Holloway, 1991; Boel et al., 1995;

Holloway et al., 1996). The key idea is to perform an

off-line structural analysis for determining algebraic

expressions. The latter will be evaluated on-line for

the current marking in order to decide the ﬁring of the

controllable transitions. These approaches are very

effective for the on-line PN control. Nevertheless,

they are valid only with a speciﬁc classes of PN.

Other approaches, which give generally nonmaxi-

mally permissive solution, have been proposed in the

literature (Giua et al., 1992; Moody and Antsaklis,

2000; Basile et al., 2006). They take the form of PN

which simpliﬁes the analysis and the implementation

of the controller. Based on the theory of regions

many works have been developed (Ghaffari et al.,

2002; Ghaffari et al., 2003; Achour and Rezg, 2006;

Lee et al., 2006). The objective is to solve optimally

the supervisory control problem using formal and

algebraic characterisations. Finally, the PN controller

synthesis problem has been treated as integer linear

programming problem (Giua and Xie, 2004; Basile

et al., 2007a; Basile et al., 2007b). Nevertheless,

these approaches require a very high time computing.

Although the synthesis problem of PN controller

has been widely treated in the literature, the existing

approaches cannot be exploited directly in our case

because, it is impossible to characterise the forbidden

states by constraints. Indeed, the control speciﬁca-

tion is mainly important to synthesise a controller.

However, we have neither the control speciﬁcation

nor the system description because the identiﬁed

149

Bekrar R., Messai N., Essounbouli N., Hamzaoui A. and Riera B. (2008).

SYNTHESIS METHOD OF A PN CONTROLLER USING FORBIDDEN TRANSITIONS SEQUENCES.

In Proceedings of the Fifth International Conference on Informatics in Control, Automation and Robotics - SPSMC, pages 149-154

DOI: 10.5220/0001493201490154

 SciTePress

PN that we want to control is obtained using only

the measurable inputs and outputs system signals.

Let’s note that, we can determine the forbidden

states uniquely by comparing the observed system’s

states and states reachable by the identiﬁed PN model.

This paper deals with the supervisory control

problem of DES characterised by forbidden states

and modelled by bounded ordinary PN. The PN

herein considered is transitions controllable and

contains measurable and non measurable places.

The latter represent the non measurable outputs

system signals. Indeed, the fact that all transitions are

controllable then, the PN reachability graph does not

contain dangerous markings. In addition, we suppose

that we have not the control speciﬁcation and the

initial marking is the unique marked state. Finally,

we consider that the PN reachability graph does

not contain deadlock states because the input and

the output signals observed during the identiﬁcation

phase represent only the normal behaviours of the

considered system.

The proposed PN controller is synthesised us-

ing the forbidden transitions sequences deduced

from the PN reachability graph. The designed

PN controller permits to avoid the occurrence of

forbidden markings, generated by the non controlled

PN model, and guarantees a set of desired behaviours.

In this paper, we start by presenting the considered

FSP and deﬁning the control speciﬁcations. Then, we

present a procedure to calculate the forbidden transi-

tions sequences from the PN reachability graph and

introduce a new algorithm to design the PN controller

using these sequences. At last, we illustrate the pro-

posed algorithm by an explicative example.

2 CONTROL SPECIFICATIONS

We consider the basic supervisory control problem to

synthesise a PN controller that avoids the occurrence

of forbidden states. In this paper, we assume that

the PN that we want to control is established using

the I/O sequences describing all the system normal

behaviours according to the algorithm presented in

(Bekrar et al., 2006b). Hence, our objective is to add

some control places in order to guarantee that all

the behaviours generated by the identiﬁed PN model

correspond to those generated by the real system.

Note also that, each PN reachable marking M

composed of two parts: M

i ¯m

where, the ﬁrst

one represents the marking of the measurable places

and the second part represents the estimated marking

of the non measurable places.

The control speciﬁcations to be addressed in this

paper are the forbidden states type and the problem

herein considered becomes a FSP. We will treat it as

a FSTP as proved in (Ghaffari et al., 2002). To solve

this problem, we propose an algorithm that consists

in: (1) identifying the set of the forbidden markings,

(2) determining the set of the equivalent forbidden

state transitions, (3) developing a PN controller.

2.1 Identiﬁcation of Forbidden

Markings

Contrary to works proposed to treat the FSP where

the forbidden states are deﬁned explicitly by con-

straints, in our case we must calculate them using the

behaviours of the real system and those of the PN

model. The behaviours of the considered system are

represented by the set of its reachable states called

E . Those generated by the PN modelling the system

are represented by its reachability graph called R

Note that, a marking M

∈ R

reachable by the PN

that we want to control is said forbidden marking if

its measurable part is not equivalent to any state E

in E otherwise, it is a legal marking. Hence, the set

of the forbidden markings can be obtained using the

following procedure:

Input: E and R

Output: M

f r

the set of forbidden markings.

Begin

1. Initialise the set of forbidden markings: M

f r

2. For each marking M

∈ R

do:

(a) If there exists a state E

∈ E such that M

= E

then:

• M

is legal marking.

(b) Else, M

is a forbidden marking.

• Update the set of forbidden markings: M

f r

∪ M

End If.

End For.

End.

Once the set of the forbidden markings is obtained,

we should determine the set of the transitions lead-

ing to or ﬁring from forbidden markings in order to

prevent the ﬁring of these transitions.

ICINCO 2008 - International Conference on Informatics in Control, Automation and Robotics

150

2.2 Forbidden State Transitions

A state transition in the reachability graph of the PN

that we want to control, which ﬁres from a marking

∈ R

and leads to a marking M

∈ R

> M

is said forbidden state transition if and only if one of

the following conditions is veriﬁed:

• (a) M

is forbidden marking or,

• (b) M

is forbidden marking.

These can be reformulated, using E and R

, as fol-

lows: a state transition (M

→ M

) in R

is said for-

bidden iff:

• ∃E

∈ E : M

= E

and @E

∈ E : M

= E

or,

• @E

∈ E : M

= E

and ∃E

∈ E : M

= E

or,

• @E

, E

∈ E : M

= E

and M

= E

Proof : the occurrence of forbidden marking can be

prevented by avoiding the ﬁring of the transition

leading to this marking. Moreover, it is clear that

a transition ﬁring from a forbidden marking is a

forbidden transition. So, each transition leading to

or ﬁring from a forbidden marking is considered as a

forbidden transition.

Therefore, the forbidden state transitions set can be

obtained using the following procedure:

Input: E , R

and M

f r

Output: Ψ the set of forbidden state transitions.

Begin

1. Initialise the set of forbidden state transitions:

Ψ =

2. For each state transition (M

→ M

) ∈ R

such

that M

, M

∈ R

do:

• If (a) or (b) is veriﬁed then: (M

→ M

) is a

forbidden state transition.

– Update Ψ:(Ψ:= Ψ ∪ {(M

→ M

)}).

• Else, (M

→ M

) is a legal state transition.

End If.

End For

End.

Once the forbidden state transitions are determined,

the forbidden transitions sequences will be calculated

and used for designing a PN controller. More details

concerning these steps will be presented in the next

section.

3 THE PROPOSED PN

CONTROLLER

We can solve the forbidden state problem considered

in this paper by adding control places {p

, ..., p

}

to the initial PN model (N, M

). These places are

deﬁned as follows:

Deﬁnition 1: A control place p

of a PN model

(N, M

) is deﬁned by: (i) M

): its initial marking,

(ii) Post(p

, .) and Pre(p

, .): the weighting vectors

of the arcs connecting the transitions of (N, M

) to

and connecting p

to the transitions of (N, M

)

respectively.

Remark 1: Since the considered PN is ordinary then,

the arcs weighting values are equal to 1.

In order to solve this problem, we propose to use the

forbidden transitions sequences, calculated from the

reachability graph of the PN that we want to control,

to determine the control places to be added. Note

that, these sequences have been used by Lee et al.,

(2006) but with the constraint asynchronous reacha-

bility graph.

3.1 The Forbidden Transitions

Sequences

Before introducing the computing procedure of the

forbidden transitions sequences, let us present the

following deﬁnitions that will be used in the PN

controller design algorithm.

Deﬁnition 2: A transitions sequence σ

is said forbid-

den if and only if it allows the ﬁring of at least one

forbidden transition. Thus, σ

= t

, ..., t

is said forbidden iff: ∃t

∈ σ

∗

M(t

) ∈ M

f r

∗

) ∈ M

f r

where

∗

M(t

) and M

∗

) represent

respectively the input and the output marking of t

, otherwise, it is a legal.

The forbidden transitions sequences are calculated

using the PN reachability graph as follows:

Input: R

, Ψ.

Output: S the set of forbidden transitions sequences.

Begin

1. Initialise the set of forbidden transitions se-

quences: S :=

2. Calculate S

the set of all legal transitions se-

quences reachable from the initial marking M

3. Calculate the set of the successors transitions of

each transitions sequence σ

in S

, noted Suc(σ

4. For each transition t

in the set Suc(σ

) do:

SYNTHESIS METHOD OF A PN CONTROLLER USING FORBIDDEN TRANSITIONS SEQUENCES

151

(a) If t

is a forbidden transition then:

• σ

:= σ

is a forbidden transitions sequence.

• Else, stop the successors computation proce-

dure of the transitions sequence σ

(b) Update S (S := S ∪{ σ

}).

End If.

End For

5. Calculate the set of successors transitions of each

transitions sequence σ

in S.

6. Go to 4.

7. End.

Note that, in our case, each forbidden transitions se-

quence σ

is composed of two sub-sequences as fol-

lows: σ

= t

, ..., t

| {z }

, ..., t

| {z }

, with σ

is a legal tran-

sitions sub-sequence authorised to be ﬁred from the

initial marking M

and it can be equal to the empty

string ε (i.e., σ

= ε). Thus, it means that there exists

forbidden states reachable from M

after the ﬁring of

only one transition. σ

is a sub-sequence forbidden

to ﬁring from M

where M

is the marking reachable

after the ﬁring of t

. This sub-sequence represents

the transitions inﬂuenced by the ﬁring of the forbid-

den transition t

. We note by

∗

and σ

in∗

the ﬁrst

and the last transition of σ

respectively. Hence, all

the markings reachable from M

after the ﬁring of the

sub-sequence σ

, transition after transition, are legal

markings. However, the markings reachable from M

after the ﬁring of the sub-sequence σ

, transition af-

ter transition, are forbidden markings as represented

in ﬁgure 1.

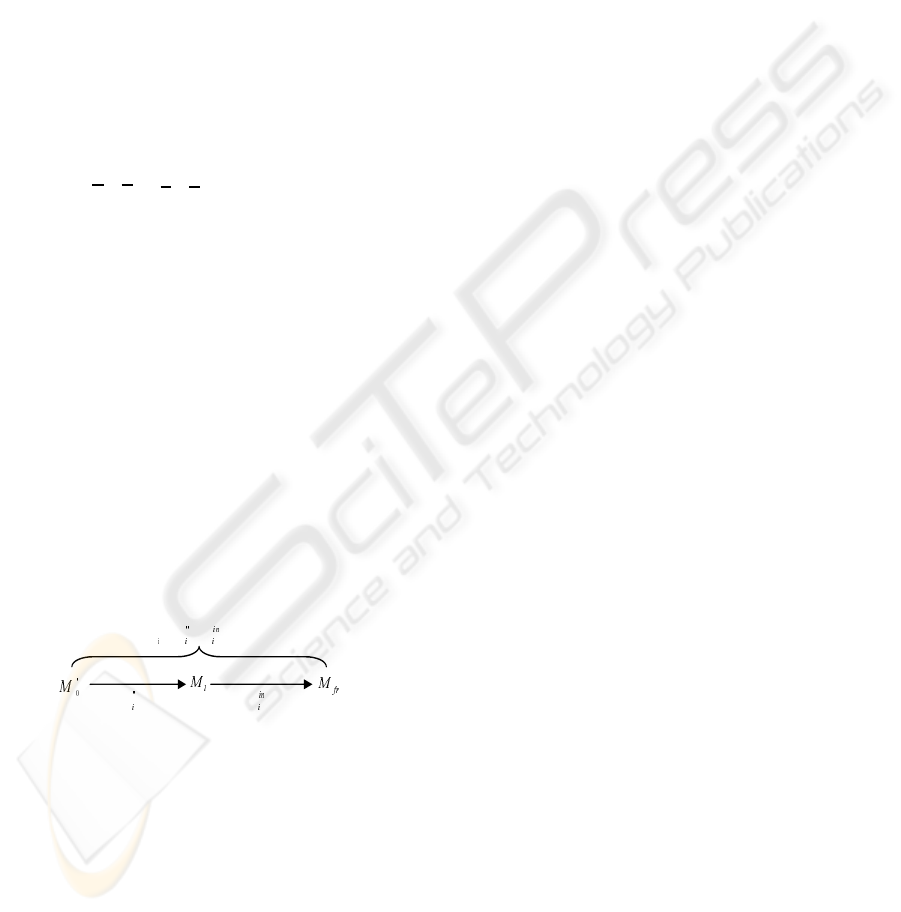
σ σ σ

= +

Figure 1: Legal and forbidden markings.

Deﬁnition 3: Let’s σ

= t

, ..., t

transitions se-

quence. |σ

| designs the length of this sequence,

and it represents the number of transitions in this

sequence.

Remark 2: For each transition t

∈ σ

, we note by

∩ σ

| the number of times that the transition t

appears in σ

Deﬁnition 4: Let’s σ

= t

, ..., t

a transitions

sequence that we consider as a word generated by a

PN. The preﬁx of σ

, noted Pre f (σ

), is a word t

...t

with 0 ≤ j ≤ k and k = |σ

|. For q ∈ N: Pre f (σ

)

(q)

represents a preﬁx of σ

of width equal to q.

Remark 3: Since PN to be controlled is bounded, then

its reachability set is ﬁnite. Also, the language gener-

ated by this PN is ﬁnite preﬁx-closed language.

3.2 Synthesis Algorithm of PN

Controller

Based on previous deﬁnitions and notations, we pro-

pose an algorithm that allows to add control places

to the initial PN model for preventing the reachability

of forbidden markings. This algorithm contains the

following:

1. Construction of the reachability graph of the PN

that we want to control.

2. Identiﬁcation of the forbidden markings and the

determination of the forbidden state transitions by

browsing the PN reachability graph.

3. Determination of the forbidden transitions se-

quences set. These latter, we consider them as for-

bidden words generated by the PN model. Then,

we use these sequences to synthesise a PN con-

troller.

Hence, the PN controller will be synthesised using

the following algorithm:

Input: E the system states set, (N, M

) the PN model.

Output: Controlled PN.

Begin

1. Construct the reachability graph R

of the PN

model to be controlled (N, M

2. Identify the set of forbidden markings noted M

f r

as introduced in subsection 2.1.

3. Determine the set of forbidden state transitions Ψ

by executing the procedure described in subsec-

tion 2.2.

4. Determine the set S of all forbidden transitions

sequences ﬁring from the initial marking M

browsing the reachability graph of PN that we

want to control as shown in subsection 3.1.

5. Update the set of forbidden transitions sequences

S as follows:

(a) For each transitions sequence σ

∈ S do:

i. Calculate the preﬁx set of this sequence noted

Pre f (σ

ii. Compare the preﬁxes of σ

to the preﬁxes of

all the transitions sequences in S as follows:

ICINCO 2008 - International Conference on Informatics in Control, Automation and Robotics

152

A. If there exists at least one transitions se-

quence σ

such that, all the elements of pre-

ﬁxes set of σ

are included in the preﬁxes set

of σ

(Pre f (σ

) ⊂ Pre f ()σ

) then:

• Eliminate σ

from S: S := S\{σ

}

B. Else, σ

still in S.

End If

End For.

6. For each forbidden transitions sequence in S do:

(a) Determine the sub-sequence of legal transitions

and the sub-sequence of forbidden transi-

tions σ

(b) For each sub-sequence of forbidden transitions

, we determine

∗

and σ

in∗

as an input of

∗

and

as an output of σ

in∗

(d) Mark p

with initial marking M

) =

∗

∩ σ

|. Il there exists several forbidden

transitions sub-sequences which have the same

∗

and σ

in∗

then, we add one control place

and we mark it with initial marking equal to

max

i,σ

∈S

∗

∩ σ

|).

End For.

End.

This algorithm allows to complete the PN model,

established using the identiﬁcation approach pro-

posed previously, by adding control places. The anal-

ysis of the algorithm computational complexity shows

that it is linear with the number of places, of transi-

tions and, the length of the largest forbidden transi-

tions sequences.

4 ILLUSTRATIVE EXAMPLE

To illustrate the proposed algorithm, let us consider

the example of a system described by the identiﬁed

PN model of ﬁgure 2:

••

•

Figure 2: The PN model.

The set of its states is given by: E =













| {z }













| {z }













| {z }













| {z }













| {z }













| {z }













| {z }

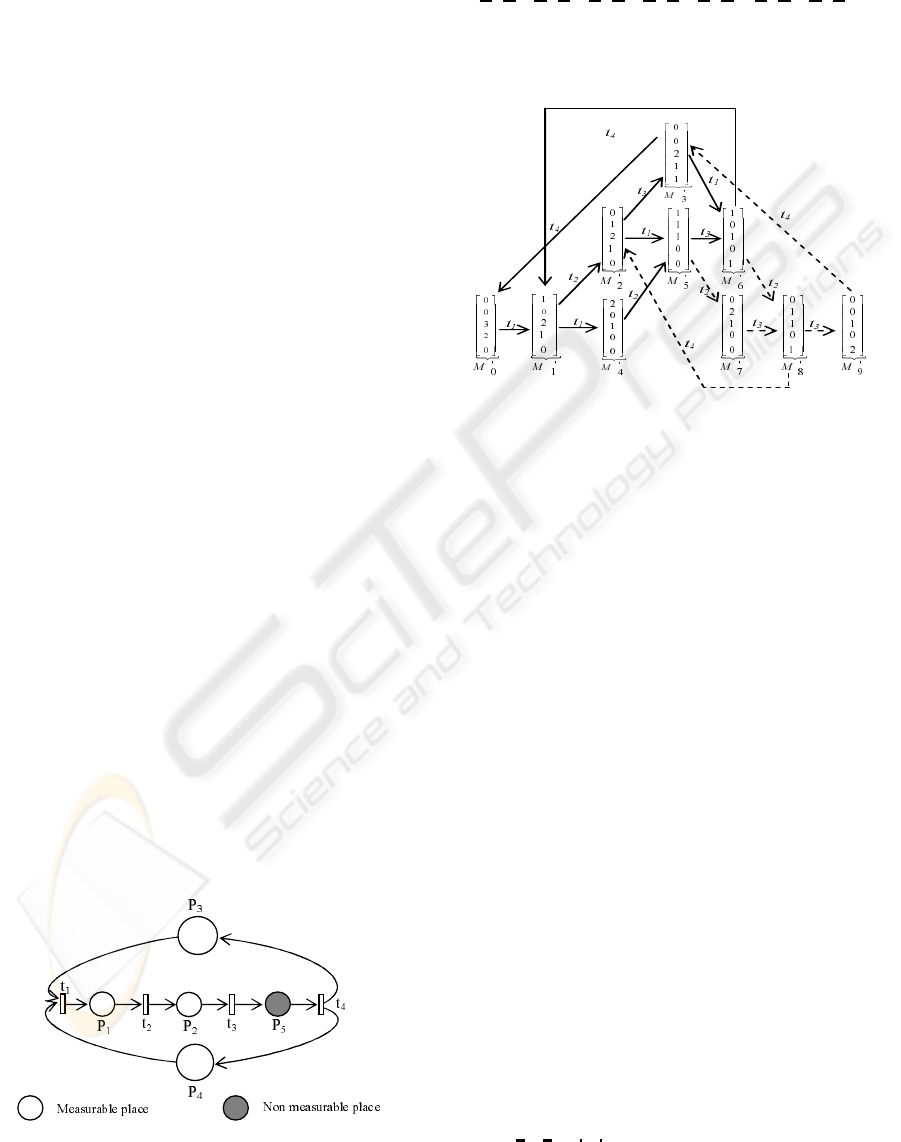
Firstly, we elaborate the PN reachability graph that is

given in ﬁgure 3.

Figure 3: The PN reachability graph.

The set of forbidden markings is: M

f r

, M

}. These markings must be removed

from R

together with their arcs coming from other

markings to these forbidden markings or going from

these forbidden markings to other markings in R

The set of the equivalent forbidden state transi-

tions are: {(M

→ M

), (M

→ M

), (M

→ M

), (M

→ M

), (M

→ M

)}. By ap-

plying the procedure described in subsection 4.1,

the forbidden transitions sequences ﬁring from the

initial marking are: σ

= t

, σ

= t

, σ

= t

, σ

= t

, σ

= t

, σ

= t

, σ

= t

, σ

= t

, σ

= t

. Thus, the

set of the forbidden transitions sequences is

S = {σ

i,i=1,...,13

}. To update S, we must calcu-

late the preﬁx of each sequence in S. We take as

example the sequences σ

and σ

: Pre f (σ

) =

{ε,t

}, Pre f (σ

) =

{ε,t

}. We

note that Pre f (σ

) = Pre f (σ

) then, we eliminate σ

from S: S := S\{σ

}. By repeating the same process

with the remaining sequences, and after updating

S, we have ﬁnally : S = {σ

, σ

Then, for each forbidden transitions sequence in

S we determine the legal transitions sub-sequence

and the forbidden one. We take for example

= t

| {z }

|{z}

. We remark that σ

= t

and

SYNTHESIS METHOD OF A PN CONTROLLER USING FORBIDDEN TRANSITIONS SEQUENCES

153

= t

. Finally, by analysing the remaining for-

bidden sequences, we see that all the forbidden

transitions sub-sequences have the same

∗

= t

and σ

in∗

= t

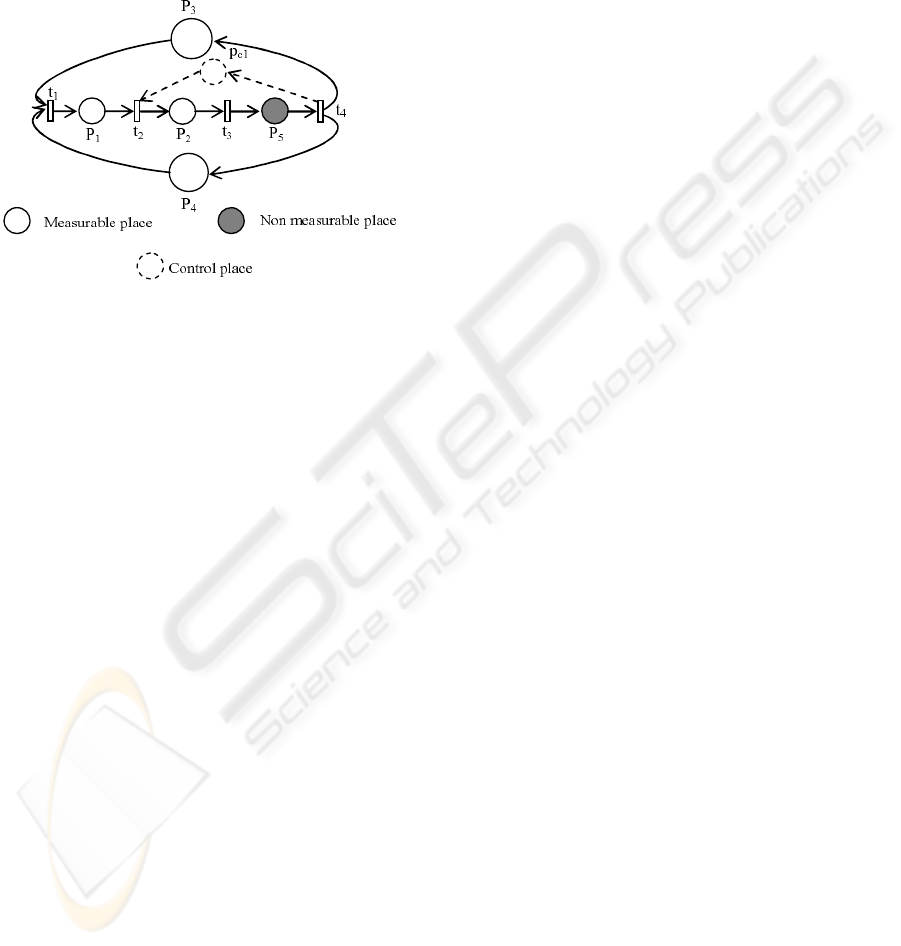
for i ∈ {2, 4, 6, 11, 13}. Therefore,

we add one control place p

with initial marking

) = max(|

∗

∩ σ

|) = 1. This place is an

input of t

and an output of t

as depicted in the ﬁgure

•

Figure 4: The Controlled PN model.

5 CONCLUSIONS

This paper presents a synthesis method of a PN con-

troller to solve a FSP of DES modelled by a bounded

ordinary PN. The model to be controlled is transi-

tions controllable. Using the system behaviours and

those generated by the considered PN model, forbid-

den markings are identiﬁed and the equivalent forbid-

den state transitions are determined. Then, the forbid-

den transitions sequences deduced from the PN reach-

ability graph are used to synthesise a PN controller.

The latter is maximally permissive within the speciﬁ-

cations that guarantees the desired behaviours.

As Future work, we will generalise this method to PN

with uncontrollable transitions.

REFERENCES

Achour, Z. and Rezg, N. (2006). Time ﬂoating general mu-

tual exclusion constraints (tfgmec). In IMACS, Multi-

conference on Computational Engineering in Systems

Applications.

Basile, F., Carbone, C., and Chiacchio, P. (2007a). Feed-

back control logic for backward conﬂict free choice

nets. In IEEE Transaction on Automation and Con-

trol.

Basile, F., Chiacchio, P., and Giua, A. (2006). Suboptimal

supervisory control of Petri nets in presence of uncon-

trollable transitions via monitor places. In Automat-

ica.

Basile, F., P.Chiacchio, and Giua, A. (2007b). An optimi-

sation approach to Petri net monitor design. In IEEE

Transaction on Automation and Control.

Bekrar, R., Messai, N., Essounbouli, N., Hamzaoui, A., and

Riera, B. (2006a). Identiﬁcation of discrete event sys-

tems using ordinary Petri nets. In IAR-ACD’06, Pro-

ceeding of 2006 Workshop on Advanced Control and

Diagnosis.

Bekrar, R., Messai, N., Essounbouli, N., Hamzaoui, A., and

Riera, B. (2006b). Off-line identiﬁcation for a class of

discrete event systems using safe Petri nets. In DES-

Des’06, 3rd IFAC Workshop on Discrete Event System

Design.

Boel, R., Ben-Naoum, L., and Breusegem, V. V. (1995). On

forbidden state problems for a class of controlled Petri

nets. In IEEE Transaction on Automatic and Control.

Ghaffari, A., Rezg, N., and Xie, X. (2002). Algebraic and

geometric characterization of Petri net controllers us-

ing the theory of regions. In WODES’02, Proceedings

of 6

Worksop on Discrete Event Systems.

Ghaffari, A., Rezg, N., and Xie, X. (2003). Feedback

control logic for forbidden state problems of marked

graphs: application to a real manufacturing system. In

IEEE transaction on Automatic and Control.

Giua, A., DiCesare, F., and Silva, M. (1992). Generalized

mutual exclusion constraints on nets with uncontrol-

lable transitions. In Proceedings of IEEE Interna-

tional Conference on System, Man and Cybernitic.

Giua, A. and Xie, X. (2004). Control of safe ordinary Petri

nets with marking speciﬁcations using unfolding. In

WODES’04, Proceedings of the 7

IFAC Workshop

on Discrete Event Systems.

Holloway, L., Guan, X., and Zhang, L. (1996). A general-

ization of state avoidance policies for controlled Petri

nets. In IEEE Transaction on Automatic and Control.

Krogh, B. H. and Holloway, L. E. (1991). Synthesis of feed-

back control logic for discrete manufacturing systems.

In Automatica.

Lee, E. J., Toguyeni, A., and Dangoumau, N. (2006). A

Petri net based approach for the synthesis of parts’

controllers for reconﬁgurable manufacturing systems.

In Proceeding of SICE-ICASE International Joint

Conference.

Moody, J. and Antsaklis, P. (2000). Petri net supervisors for

dbs with uncontrollable and unobservable transitions.

In Proceedings of IEEE International Conference on

System, Man and Cybernitic.

ICINCO 2008 - International Conference on Informatics in Control, Automation and Robotics

154