
SUBJECTIVE PREFERENCES IN FINANCIAL PRODUCTS
Emili Vizuete Luciano and Anna Mª Gil Lafuente
Department of Business Administration, University of Barcelona
Av. Diagonal 690, 08034, Barcelona, Spain
Keywords: Fuzzy Sets, Finance, Subjective Preferences.
Abstract: When the decision maker invests in the banking organizations, he is faced with the need to choose between
apparently different products. The financial advisers have to offer an agile and well-qualified service to be
able to continue counting on the confidence of their customers and to increase their results consequently. In
this paper, presents a further step in justifying such evalutation. Results from the proposed approach present
a better undestanding of each system to decision makers for evaluating justification issues which sometimes
cannot be defined.
1 INTRODUCTION
With increasing frequency it can be seen that new
products appear on the market under many different
forms that, either real or apparent, have different
characteristics. It should not be forgotten that the
strong competition characterising the financial world
obliges those offering payment means to a great
effort of diversification and differentiation of
products that permits them, on the one hand, to
cover the widest range of possible users and, on the
other, provoke a flaw by means of the presentation
of different products with the object of get around
the laws of the perfect market.
Evidently that for each business, and even for
each specific situation, there will be a different
valuation of each one of the characteristics of the
financial products (Zadeh, 1971).
In this context two fundamental elements appear
that make up the problem:
1) Differentiation in the characteristics of
each one of the financial products on offer.
2) Different estimate, by the acquirer, of each of
the characteristics relative to the rest, which
provides an order of preference.
Evidently, the degree of preference for each one
of the characteristics relative to the others may
sometimes be determined by means of
measurements, that is, with an objective nature, but
on other occasions it will be necessary to resort to
subjective numerical situations, that is by means of
valuations.
With all this an attempt is made to arrive at
certain results that express the order of preference
between different financial products to which a
business may opt. The subjective nature of the
estimated values should lead to certain conclusions
that can be expressed by means of fuzzy sets
(Bustince & Herrera, 2008).
2 PROBLEM FORMULATION
We start out from the existence of a finite and re-
countable number of financial products
P
1
,P
2
,..., P
n
,
which each posses certain determined characteristics
C
1
,C
2
,...,C
m
in such a way that for each
characteristic it is possible to establish a quantified
(objective or subjective) relation of preferences.
Therefore for
C
j
we have that: P
1
is preferred
μ
1
/
μ
2
times over P
2
,
μ
1
/
μ
3
times over P
3
, …,
μ
1
/
μ
n
times over
P
n
, …,
P
n
is preferred
μ
n
/
μ
1
times over
P
1
,
μ
n
/
μ
2
times over P
2
, …,
μ
n
/
μ
n
−
1
times over
P
n-1
.
With this previous relation of preferences we
will be able to construct the following matrix, which
will be reflexive and reciprocal by construction:
407
Vizuete Luciano E. and M
a
Gil Lafuente A. (2008).
SUBJECTIVE PREFERENCES IN FINANCIAL PRODUCTS.
In Proceedings of the Tenth International Conference on Enterprise Information Systems - AIDSS, pages 407-410
DOI: 10.5220/0001676904070410
Copyright
c
SciTePress
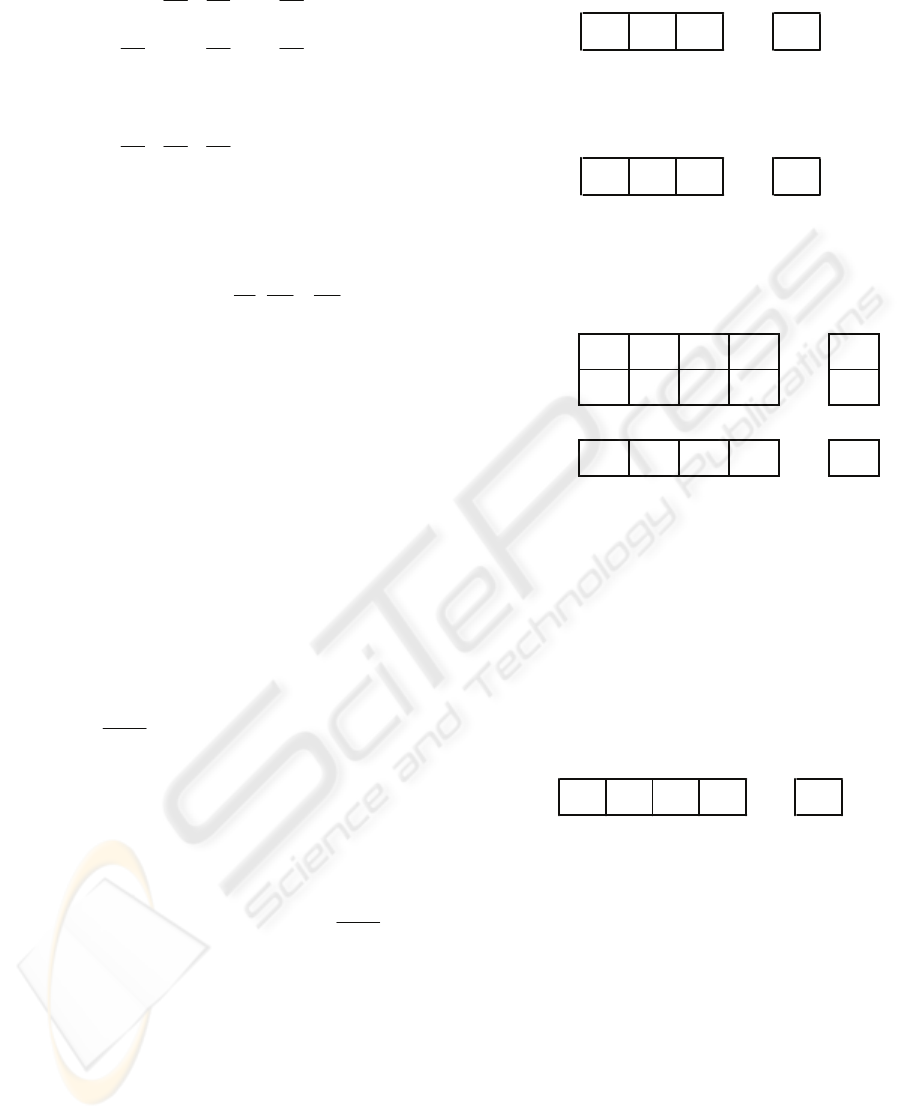
[C
ij
] =
1
μ
1
μ
2
μ
1
μ
3
...
μ
1
μ
n
μ
2
μ
1
1
μ
2
μ
3
...
μ
2
μ
n
... ... ... ... ...
μ
n
μ
1
μ
n
μ
2
μ
n
μ
3
... 1
⎡
⎣
⎢
⎢
⎢
⎢
⎢
⎢
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
⎥
⎥
⎥
⎥
⎥
⎥
(1)
This matrix is also coherent or consistent,
(Dubois & Prade, 1995) since the following is
complied with:
∀i, j,k ∈ {1, 2, ..., n},
μ
i
μ
j
⋅
μ
j
μ
k
=
μ
i
μ
k
(2)
For this reason we are going to consider certain
properties (Vasantha, 2007), those in which all the
elements that are members of
R
0
+
:
a) A positive square matrix posses a
dominant value of its own l real positive which is
unique for which what is complied is that
λ
≥ n,
where n is the order of the square matrix.
b) The vector that corresponds to the dominant
own value is found also formed by positive terms
and when normalised, is unique.
When
λ is a number close to n it is said that the
matrix is nearly coherent; on the contrary it will be
necessary to make an adjustment between the
elements of the matrix (Gil Aluja, 1998 & 1999), if
wanting to use this scheme correctly. It is considered
that
λ−n or
λ−n
n
is an index of coherence. As is
very well known, when a reciprocal matrix is also
coherent it complies with
[C
ij
] ⋅[v
i
]
T
= n ⋅[v
i
]
T
where
[v
i
]
T
is the transpose of row i.
When the reciprocal matrix is not coherent, we
write:
[C
ij
] ⋅[ ′ v
i
]
T
=λ⋅[ ′ v
i
]
T
. We accept [
′
v
i
] as the
result when the index of coherence
λ
−
n
n
is
sufficiently small.
For each characteristic
C
j
, j = 1, 2, ...,
m
the
corresponding reflexive and reciprocal matrix
[C
ij
]
is obtained. Once the m matrices are constructed the
dominant own values
λ
j
and their corresponding
vectors
[
]
nj
X
ij
X ...
must be found for each one,
verifying if they posses sufficient consistency by
means of the «index of coherence».
The elements of each corresponding own vector
will give rise to a fuzzy sub-set:
…
=…
P
1
P
2
P
3
P
n
X
j
x
1j
x
2j
x
3j
x
4j
which once normalised in sum equal to one will
be:
…
=…
P
1
P
2
P
3
P
n
D
j
p
1j
p
2j
p
3j
p
4j
The
m own vectors are regrouped forming a
Matrix 1, the form of which will be:
Matrix 1
C1
C2
C3
C4
Cm
P1
P11
P12
P13
P14
…
P1m
[Pij]=
P2
P21
P22
P23
P24
…
P2m
… …
…
…
…
Pn
Pn1
Pn2
Pn3
Pn4
…
Pnm
Each column of this matrix brings to light the
relative degree in which a characteristic is possessed
by all the financial products. As we have already
pointed out, this can be represented by a normalised
fuzzy sub-set
D
j
. From this perspective there exist
m fuzzy sub-sets (Kao & Liu, 2001). On the other
hand each row expressed, for one product, the
degree in which it possess each one of the
characteristics, which is also represented by a fuzzy
sub-set
Q
i
such as:
…
=…
Q
i
p
i1
p
i2
p
i3
p
i4
p
im
C
1
C
2
C
3
C
4
C
m
On the other hand, each business has a different
appreciation of the importance that each
characteristic has (Gil Lafuente, 2005). Evidently,
this estimate can vary from one moment to another
and its quantification has a basically subjective
sense, therefore will be expressed by means of
valuations.
The establishment of these valuations can be
done by means of a comparison between the relative
importance of a characteristic in relation to the rest.
Therefore, for example, it can be said that a
characteristic is two times as important as another,
or has half the importance of a third.
In this way we can construct a Matrix 2, that
obviously will be square, reflexive and anti-
symmetrical. Since there are n products, its order
will be
m
×
m
:
ICEIS 2008 - International Conference on Enterprise Information Systems
408
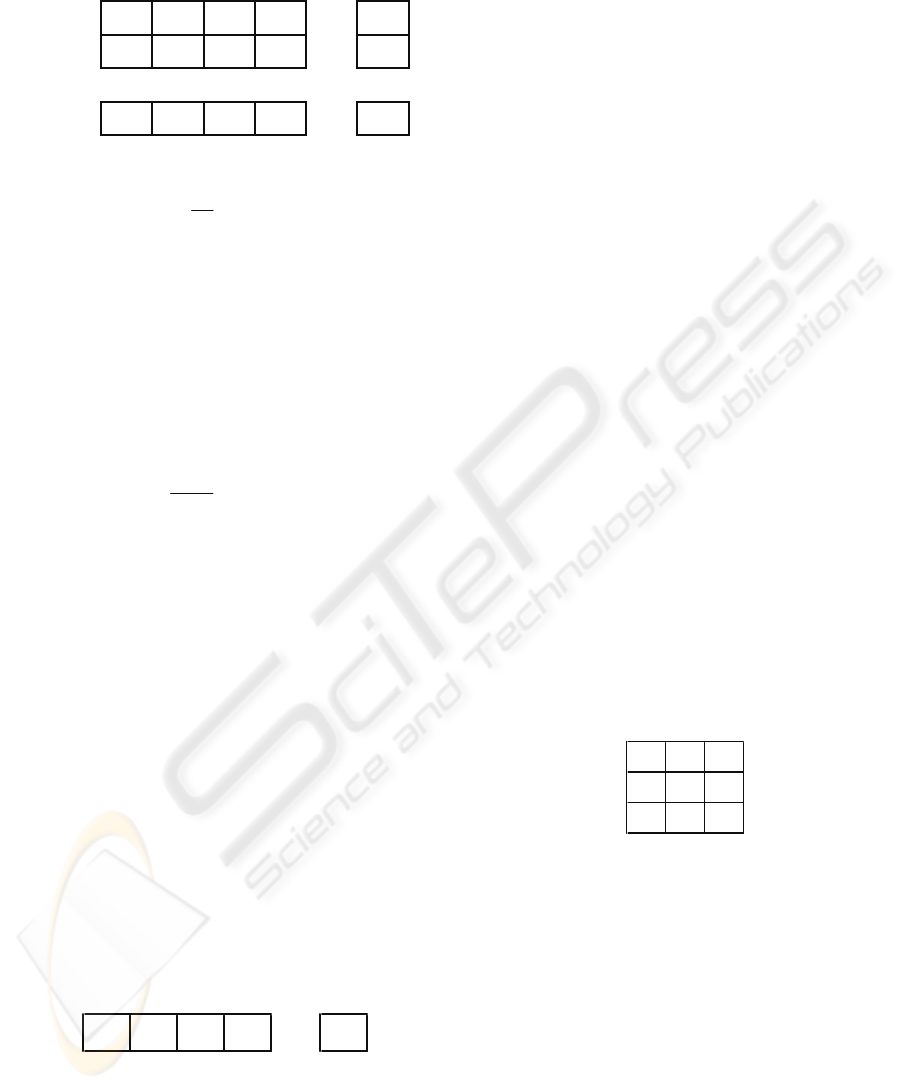
Matrix 2
C1
C2
C3
C4
Cm
C1
1
a12
a13
a14
…
a1m
[Pij]=
C2
a21
1
a23
a24
…
a2m
… …
…
…
…
Cm
am1
am2
am3
am4
…
1
Due to the condition of asymmetry the following
will be complied with:
ij
ij
a
a
1
=
(3)
Once the matrix 2 has been determined, we
proceed to obtain the corresponding dominant value
and vector. This vector will bring to light the
preferences of the business relative to the
characteristics,
[
]
5
4321
yyyyy
j
y =
In order for this vector to be susceptible to being
used as a weighting element, we are going to convert
it into another that possesses the property that the sum
of its elements be equal to the unit. For this we do:
∑
=
=
m
j
j
j
j
y
y
b
1
,
j = 1, 2, ...,
m
(4)
With which we arrive at
[
]
5
4321
bbbbb
j
b =
We are now in a position finally to arrive at the
sought after result, by taking matrix
[p
ij
] and
multiplying it to the right by vector
[b
j
]. The result
will be another vector, which will express the
relative importance of each financial product for the
business, taking into account its preferences for each
one of the characteristics:
p
11
p
12
p
13
... p
1m
p
21
p
22
p
23
... p
2m
p
31
p
32
p
33
... p
3m
... ... ... ... ...
p
n1
p
n2
p
n3
... p
nm
⎡
⎣
⎢
⎢
⎢
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
⎥
⎥
⎥
⋅
b
1
b
2
b
3
...
b
m
⎡
⎣
⎢
⎢
⎢
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
⎥
⎥
⎥
=
d
1
d
2
d
3
...
d
m
⎡
⎣
⎢
⎢
⎢
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
⎥
⎥
⎥
(5)
The result can also be expressed by means of a
normal fuzzy sub-set, by doing:
…
=…
P
1
P
2
P
3
P
m
H
h
1
h
2
h
3
h
m
P
4
h
4
This model on the contrary to all those that use
as the only basis for selection, the price of the
money, has as its greatest advantage the possibility
of incorporating a wide range of elements that, in the
reality of businesses, at times play a decisive role at
the time of taking the decision to select a financial
product from among those offered on the market.
These elements normally do not have the same
weight at the time of making a valuation.
3 APPLICATION OF THE
PROPOSED MODEL
With the object of illustrating the model a case has
been considered which we have linked to the one
shown, in order to cover certain financial
requirements, resorts to three credit institutions
which propose as the most adequate, one financial
product each (Vizuete & Gil Lafuente, 2007).
Therefore there is a choice between three products
P
1
,
P
2
,
P
3
.
The characteristics of these products makes them
different, but in certain aspects some are more
attractive, but in others these are less favourable.
Obviously, in the eyes of the businessman not all the
characteristics have the same weight at the time of
deciding to accept one or another (Kaufmann & Gil
Aluja, 1987 & 1990). The five characteristics
mentioned previously were considered as important:
price of the money, payback period, possibilities for
renewal, fractioning repayments, speed of granting.
1. With regard to the price of the money the
following data is considered: for
P
1
20%, for
P
2
22% and for
P
3
18%. This then is objective data and
it is logical to think that the preference would be for
the lowest price in a proportional manner. In this
way the following matrix can be constructed:
1 11/10 9/10
10/11 1 9/11
10/9 11/9 1
P
1
P
2
P
3
P
1
P
2
P
3
Once this matrix has been constructed the
corresponding dominant own value and vector must
be obtained. Among the various procedures existing
we are going to use the following:
11,10,9
0,9090 1 0,8181
1,1111 1,2222 1
⎡
⎣
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
⋅
1
1
1
⎡
⎣
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
=
3
2,7271
3,3333
⎡
⎣
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
= 3,3333⋅
0, 9
0,8181
1
⎡
⎣
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
11,10,9
0,9090 1 0,8181
1,1111 1,2222 1
⎡
⎣
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
⋅
0, 9
0,8181
1
⎡
⎣
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
=
2,6999
2,4543
2,9998
⎡
⎣
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
= 2,9998⋅
0, 9
0,8181
1
⎡
⎣
⎢
⎢
⎢
⎤
⎦
⎥
⎥
⎥
For normalisation of the sum equal to 1, in this
way arriving at:
SUBJECTIVE PREFERENCES IN FINANCIAL PRODUCTS
409
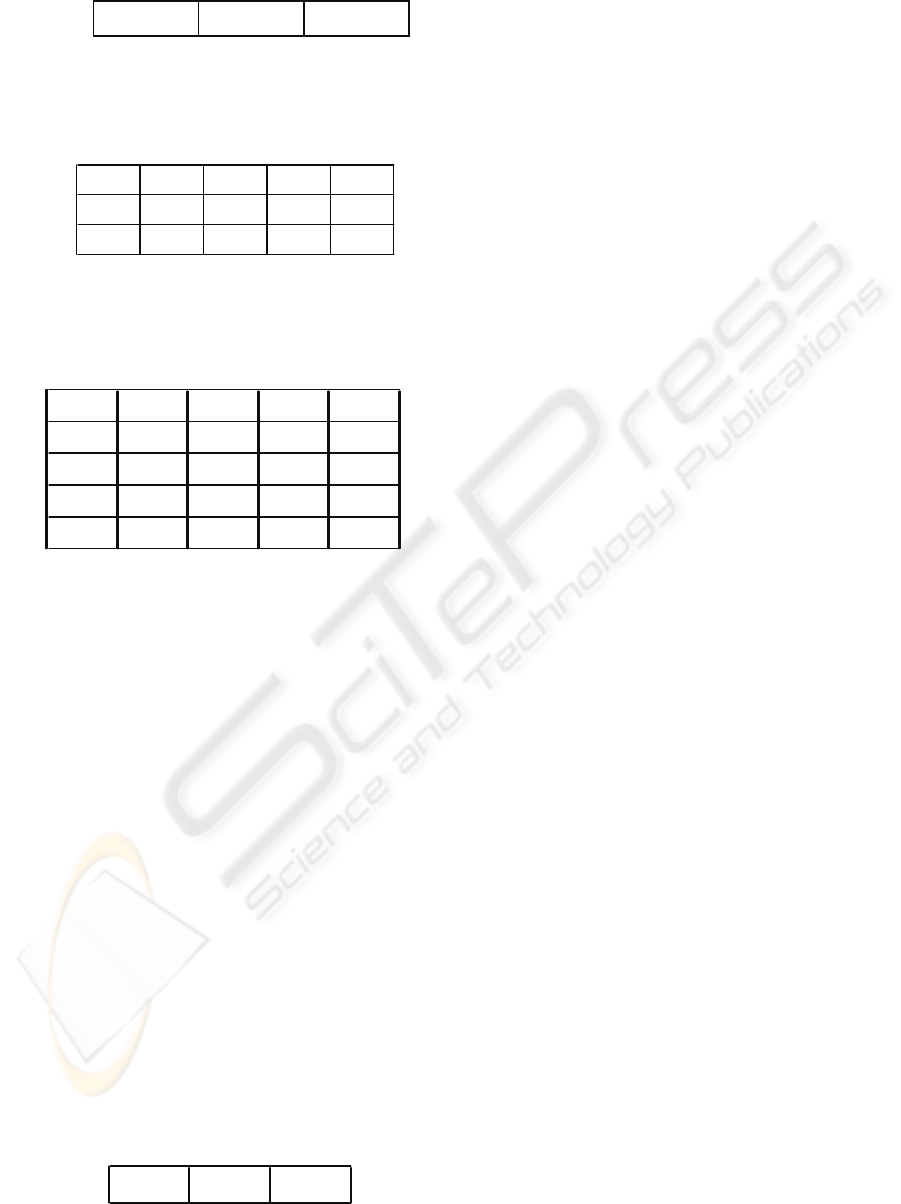
P1
P2
P3
[Pi1]=
0,3311
0,3009
0,3679
The same process should be developed for Pi2,
..., Pi5. Once we have obtained these five vectors
[p
ij
], j = 1, 2, 3, 4 , 5 , we group them and form the
following matrix:
0,3311 0,3333 0,1681 0,4285 0,6483
0,3009 0,4000 0,3572 0,1428 0,2296
0,3679 0,2666 0,4746 0,4285 0,1219
[p
ij
] =
P
1
P
2
P
3
C
1
C
2
C
3
C
4
C
5
With the following square, reflexive and
reciprocal matrix can be arrived at matrix 3:
Matrix 3
12684
1/21462
1/6 1/4 1 3 1/2
1/8 1/6 1/3 1 1/3
1/4 1/2 2 3 1
C
1
C
2
C
3
C
4
C
1
C
2
C
3
C
4
C
5
C
5
In order to obtain the corresponding dominant
own value and vector the same process can be used
as followed before. In this way with the
normalisation in sum equal to one:
[
]
0, 4704 0, 2685 0, 836 0, 0430 0, 1342
j
b =
⎡⎤
⎣⎦
Finally, if we take matrix
[p
ij
] and multiply to
the right by vector
[b
j
], which in short constitutes a
weighting, we arrive at:
0, 4704
0, 3311 0, 3333 0,1681 0, 4285 0, 6483 0, 3647
0, 2685
[d ] = 0, 3009 0, 4000 0, 3572 0,1428 0, 2296 × = 0, 3157
0, 0836
j
0, 3679 0, 2666 0, 4746 0, 4285 0, 1219 0, 3191
0, 0430
0,1342
⎡⎤
⎢⎥
⎡⎤⎡⎤
⎢⎥
⎢⎥⎢⎥
⎢⎥
⎢⎥⎢⎥
⎢⎥
⎢⎥⎢⎥
⎣⎦⎣⎦
⎢⎥
⎢⎥
⎣⎦
Taking into account that we have only
considered four decimal points and the last one has
not been rounded up, the sum of the elements of the
last matrix does not give the unit as the result, which
would have occurred if the rounding up were to have
been done.
The result we have arrived at can also be
expressed by means of a normal fuzzy sub-set, as
follows:
= 1,0000 0,8656 0,8749
P
P
1
P
2
P
3
It will be seen in this fuzzy sub-set that financial
product
P
1
is preferable to products
P
2
and
P
3
,
although not too much. There is very little difference
between
P
2
and P
3
.
4 CONCLUSIONS
In this paper, we have studied an example could be
taken as typical since it shows what happens often in
financial reality, when the decison maker is faced
with the need to choose between apparently different
products but which, when all is said and done, are
very similar. This situation should not come as a
surprise to us if it is thought that financial
institutions attempt to compensate certain
disadvantages of a product relative to other of the
competition, by means of incentives to certain
aspects that make it more attractive and allow in this
way for its placing in the market under conditions of
competitiveness.
REFERENCES
Bustince, H., Herrera, F., 2008. Fuzzy Sets and their
extensions: Representation, aggregation and models.
Springer Verlag Berlin.
Dubois, D., Prade, H., 1995. Fuzzy Relation Equations
and Causal Reasoning, Fuzzy Sets and Systems, 75; p.
119-134.
Gil-Aluja, J., 1999. Elements for a Theory of Decision in
Uncertainty, Kluwer Academic Publishers. Dordrecht,
Boston, London
Gil-Aluja, J., 1998. The interactive management of human
resources in uncertainty. Kluwer Academic
Publishers. Dortrech.
Gil Lafuente, A.M., 2005. Fuzzy logic in financial
analysis. Springer. London.
Kao,C., Liu, S.T., 2001. Fractional programming approach
to fuzzy weighted average. Fuzzy Sets and Systems,
120, p. 435-444.
Kaufmann, A., Gil-Aluja, J., 1990. Las matemáticas del
azar y de la incertidumbre. Editorial Ceura, Madrid,
Spain.
Kaufmann, A., Gil-Aluja, J., 1987, Técnicas operativas de
gestión para el tratamiento de la incertidumbre.
Editorial Hispano Europea, Barcelona, Spain.
Vasantha Kandasamy, W.B., 2007. Elementary Fuzzy
Matrix Theory and Fuzzy Models for Social Scientists.
Indian space research. USA, p. 72-90
Vizuete Luciano, E., Gil Lafuente, A.M., 2007.
Algoritmos para el tratamiento y selección de
productos financieros en al incertidumbre
(Unpublished) Doctoral Thesis in University of
Barcelona. Spain.
Zadeh, L., 1971. Similarity Relations and Fuzzy
Orderings, Inform Sci. 3. p.177-200.
ICEIS 2008 - International Conference on Enterprise Information Systems
410
