THE GENERALIZED HYBRID AVERAGING OPERATOR AND

ITS APPLICATION IN FINANCIAL DECISION MAKING

José M. Merigó and Montserrat Casanovas

Department of Business Administration, University of Barcelona, Av. Diagonal 690, 08034 Barcelona, Spain

Keywords: Aggregation operators, Decision making, Financial decision making.

Abstract: We present the generalized hybrid averaging (GHA) operator. It is a new aggregation operator that

generalizes the hybrid averaging (HA) operator by using the generalized mean. Then, we are able to

generalize a wide range of mean operators such as the HA, the hybrid quadratic averaging (HQA), etc. The

HA is an aggregation operator that includes the ordered weighted averaging (OWA) operator and the

weighted average (WA). Then, with the GHA, we are able to get all the particular cases obtained by using

generalized means in the OWA and in the WA such as the weighted geometric mean, the ordered weighted

geometric (OWG) operator, the weighted quadratic mean (WQM), etc. We further generalize the GHA by

using quasi-arithmetic means. Then, we obtain the quasi-arithmetic hybrid averaging (Quasi-HA) operator.

Finally, we apply the new approach in a financial decision making problem.

1 INTRODUCTION

Different types of aggregation operators are found in

the literature for aggregating the information. A very

common aggregation method is the ordered

weighted averaging (OWA) operator (Yager, 1988).

It provides a parameterized family of aggregation

operators that includes as special cases the

maximum, the minimum and the average criteria.

Since its appearance, the OWA operator has been

used in a wide range of applications (Calvo et al.,

2002; Merigó, 2007; Yager, 1993; Yager and

Kacprzyk, 1997).

In 2003, Xu and Da introduced the hybrid

averaging (HA) operator. It is an aggregation

operator that uses the weighted average (WA) and

the OWA operator at the same time. Then, it is able

to consider in the same problem the attitudinal

character of the decision maker and the subjective

probablity. For further research on the HA operator,

see (Merigó, 2007; Xu, 2004; 2006).

Another interesting aggregation operator is the

generalized OWA (GOWA) operator (Karayiannis,

2000; Yager, 2004). It generalizes the OWA

operator by using generalized means. Then, it

includes as special cases, the maximum, the

minimum and the average criteria, and a wide range

of other means such as the OWA operator itself, the

ordered weighted geometric (OWG) operator, etc.

The GOWA operator has been further generalized

by using quasi-arithmetic means (Beliakov, 2005)

obtaining the Quasi-OWA operator (Fodor et al.,

1995). For further research on the GOWA operator,

see (Merigó, 2007; Merigó and Casanovas, 2007;

Merigó and Gil-Lafuente, 2007).

In this paper, we introduce the generalized

hybrid averaging (GHA) operator. It generalizes the

HA operator by using generalized means. Then, it

includes in the same formulation all the cases

coming from the generalized mean. As a result, we

obtain new aggregation operators such as the hybrid

geometric averaging (HGA) operator, the hybrid

quadratic averaging (HQA) operator, etc. We further

generalize the GHA operator by using quasi-

arithmetic means, obtaining the quasi-HA operator.

We also develop an application of the new approach

in a financial decision making problem where we

can see how it can be implemented in the real life.

In order to do so, this paper is organized as

follows. In Section 2, we briefly review some basic

aggregation operators. In Section 3, we present the

GHA operator. Section 4 studies different families

of GHA operators. Section 5 develops an application

of the new approach in a financial decision making

problem. Finally, in Section 6 we summarize the

main conclusions found in the paper.

467

M. Merigó J. and Casanovas M. (2008).

THE GENERALIZED HYBRID AVERAGING OPERATOR AND ITS APPLICATION IN FINANCIAL DECISION MAKING.

In Proceedings of the Tenth International Conference on Enterprise Information Systems - AIDSS, pages 467-471

DOI: 10.5220/0001692204670471

 SciTePress

2 AGGREGATION OPERATORS

2.1 Hybrid Averaging Operator

The HA operator (Xu and Da, 2003) is an

aggregation operator that uses the WA and the OWA

operator in the same formulation. It can be defined

as follows.

Definition 1. An HA operator of dimension n is a

mapping HA:R

→R that has an associated weighting

vector W of dimension n such that the sum of the

weights is 1 and w

∈ [0,1], then:

HA(a

, a

…, a

) =

∑

(1)

where b

is the jth largest of the â

(â

= n

, i =

1,2,…,n),

= (

, …,

)

is the weighting

vector of the a

, with

∈ [0, 1] and the sum of the

weights is 1.

2.2 Generalized OWA Operator

The GOWA operator (Karayiannis, 2000; Yager

2004) is a generalization of the OWA operator by

using generalized means. It is defined as follows.

Definition 2. A GOWA operator of dimension n is a

mapping GOWA:R

→R that has an associated

weighting vector W of dimension n such that the

sum of the weights is 1 and w

∈ [0,1], then:

GOWA(a

, a

,…, a

) =

⎟

⎠

⎞

⎜

⎝

⎛

∑

(2)

where b

is the jth largest of the a

, and

is a

parameter such that

∈ (−∞, ∞).

3 THE GENERALIZED HYBRID

AVERAGING OPERATOR

The GHA operator is a generalization of the HA

operator by using generalized means. It includes in

the same formulation the weighted generalized mean

and the GOWA operator. Then, this operator

includes the WA, the OWA and the OWG operator

as special cases. It is defined as follows.

Definition 3. A GHA operator of dimension n is a

mapping GHA:R

→R that has an associated

weighting vector W of dimension n such that the

sum of the weights is 1 and w

∈ [0,1], then:

GHA(a

, a

…, a

) =

⎟

⎠

⎞

⎜

⎝

⎛

∑

(3)

where b

is the jth largest of the â

(â

= n

, i =

1,2,…,n),

= (

, …,

)

is the weighting vector

of the a

, with

∈ [0, 1] and the sum of the weights

is 1, and λ is a parameter such that λ ∈ (−∞, ∞).

From a generalized perspective of the reordering

step, we can distinguish between the descending

GHA (DGHA) operator and the ascending GHA

(AGHA) operator. The weights of these operators

are related by w

= w*

−

j+1

, where w

is the jth weight

of the DGHA and w*

−

j+1

the jth weight of the

AGHA operator.

The GHA operator is monotonic, commutative

and idempotent. Note that this operator is not

bounded by the maximum and the minimum because

for some special situations it can be higher and

lower than them.

Another interesting issue to consider are the

measures for characterizing the weighting vector W

of the GHA operator such as the attitudinal

character, the entropy of dispersion, the divergence

of W and the balance operator (Merigó, 2007).

4 FAMILIES OF GHA

OPERATORS

In the GHA operator we find different families of

aggregation operators. Mainly, we can classify them

in two types. The first type represents all the

families found in the weighting vector W and the

second type, the families found in the parameter λ.

4.1 Analysing the Weighting Vector W

By choosing a different manifestation of the

weighting vector in the GHA operator, we are able

to obtain different types of aggregation operators.

For example, we can obtain the hybrid maximum,

the hybrid minimum, the generalized mean (GM),

the weighted generalized mean (WGM) and the

GOWA operator.

The hybrid maximum is obtained if w

= 1 and

= 0, for all j ≠ 1. The hybrid minimum is obtained

ICEIS 2008 - International Conference on Enterprise Information Systems

468

if w

= 1 and w

= 0, for all j ≠ n. More generally, if

= 1 and w

= 0, for all j ≠ k, we get for any

GHA(a

, a

…, a

) = b

, where b

is the kth largest

argument a

. The GM is found when w

= 1/n, and ω

= 1/n, for all a

. The WGM is obtained when w

1/n, for all a

. The GOWA is found when ω

= 1/n,

for all a

Following a similar methodology as it has been

developed in (Merigó, 2007; Yager, 1993), we could

study other particular cases of the GHA operator

such as the step-GHA, the window-GHA, the

olympic-GHA, the S-GHA operator, the median-

GHA, the maximal entropy GHA weights, the

minimal variability GHA, etc.

For example, when w

= 1/m for k ≤ j* ≤ k + m −

1 and w

= 0 for j* > k + m and j* < k, we are using

the window-GHA operator. Note that k and m must

be positive integers such that k + m − 1 ≤ n.

The olympic-GHA, based on the olympic

average (Yager, 1996), is found when w

= w

= 0,

and for all others w

= 1/(n − 2). Note that if n = 3 or

n = 4, the olympic-GHA is transformed in the

median-GHA and if m = n − 2 and k = 2, the

window-GHA is transformed in the olympic-GHA.

We note that the median can also be used as

GHA operators. For the median-GHA, if n is odd we

assign w

(n + 1)/2

= 1 and w

= 0 for all others. If n is

even we assign for example, w

n/2

= w

(n/2) + 1

= 0.5 and

= 0 for all others.

For the weighted median-GHA, we select the

argument b

that has the kth largest argument such

that the sum of the weights from 1 to k is equal or

higher than 0.5 and the sum of the weights from 1 to

k − 1 is less than 0.5.

A further interesting family is the S-GHA

operator based on the S-OWA operator (Yager,

1993; Yager and Filev, 1994). It can be subdivided

in three classes: the “orlike”, the “andlike” and the

generalized S-GHA operator. The “orlike” S-GHA

operator is found when w

= (1/n)(1 −

) +

, and w

= (1/n)(1 −

) for j = 2 to n with

∈ [0, 1]. The

“andlike” S-GHA operator is found when w

(1/n)(1 −

) +

and w

= (1/n)(1 −

) for j = 1 to n −

1 with

∈ [0, 1]. Finally, the generalized S-GHA

operator is obtained when w

= (1/n)(1 − (

)) +

, w

= (1/n)(1 − (

)) +

, and w

= (1/n)(1 − (

)) for j = 2 to n − 1 where

∈ [0, 1] and

≤ 1. Note that if

= 0, the generalized S-GHA

operator becomes the “andlike” S-GHA operator and

= 0, it becomes the “orlike” S-GHA operator.

Other families of GHA operators could be

studied such as the centered-GHA, the EZ-GHA

weights, the Gaussian GHA weights, the

nonmonotonic GHA operator, etc. For more

information, see (Merigó, 2007).

4.2 Analysing the Parameter λ

If we analyze different values of the parameter

, we

obtain another group of particular cases such as the

usual HA, the hybrid geometric averaging (HGA),

the hybrid harmonic averaging (HHA) and the

hybrid quadratic averaging (HQA) operator.

When

= 1, we get the HA operator.

GHA(a

, a

…, a

) =

∑

(4)

From a generalized perspective of the reordering

step we can distinguish between the DHA operator

and the AHA operator. Note that if w

= 1/n, for all

, we get the WA and if ω

= 1/n, for all a

, we get

the OWA operator. If w

= 1/n, and ω

= 1/n, for all

, then, we get the arithmetic mean (AM).

When

= 0, we get the HGA operator.

GHA(a

, a

…, a

) =

∏

(5)

Note that it is possible to distinguish between

descending (DHGA) and ascending (AHGA) orders.

Note that if w

= 1/n, for all a

, we get the WGM and

if ω

= 1/n, for all a

, we get the OWG operator.

When

= −1, we get the HHA operator.

GHA(a

, a

…, a

) =

∑

(6)

In this case, we get the descending HHA

(DHHA) operator and the ascending HHA (AHHA)

operator.

When

= 2, we get the HQA operator.

GHA(a

, a

…, a

) =

2/1

⎟

⎠

⎞

⎜

⎝

⎛

∑

(7)

In this case, we also get the descending HQA

(DHQA) operator and the ascending HQA (AHQA)

operator. If w

= 1/n, for all a

, we get the WQM and

if ω

= 1/n, for all a

, we get the OWQA operator. If

= 1/n, and ω

= 1/n, for all a

, then, we get the

quadratic mean (QM).

THE GENERALIZED HYBRID AVERAGING OPERATOR AND ITS APPLICATION IN FINANCIAL DECISION

MAKING

469

Note that we could analyze other families by

using different values of the parameter λ. Also note

that it is possible to study these families

individually.

5 QUASI-ARITHMETIC MEANS

IN THE HA OPERATOR

Going a step further, it is possible to generalize the

GHA operator by using quasi-arithmetic means in a

similar way as it was done for the GOWA operator

(Beliakov, 2005). The result is the Quasi-HA

operator which is a hybrid version of the Quasi-

OWA operator (Fodor et. al., 1995). It can be

defined as follows.

Definition 4. A Quasi-HA operator of dimension n

is a mapping QHA: R

→ R that has an associated

weighting vector W of dimension n such that the

sum of the weights is 1 and w

∈ [0,1], then:

Quasi-HA(a

, …, a

) =

()

⎟

⎠

⎞

⎜

⎝

⎛

∑

−

bgwg

(8)

where

is the jth largest of the â

(â

= n

, i =

1,2,…,

n),

= (

, …,

)

is the weighting

vector of the

, with

∈ [0, 1] and the sum of the

weights is 1.

As we can see, we replace

with a general

continuous strictly monotone function

g(b). In this

case, the weights of the ascending and descending

versions are also related by

= w*

−

j+1

, where w

the

jth weight of the Quasi-DHA and w*

−

j+1

the jth

weight of the Quasi-AHA operator.

Note that all the properties and particular cases

commented in the GHA operator, are also included

in this generalization (Merigó, 2007).

6 APPLICATION IN FINANCIAL

DECISION MAKING

Now, we are going to develop an application of the

new approach in a decision making problem. We

will analyze an investment selection problem where

an investor is looking for an optimal investment.

We will develop the analysis considering a wide

range of particular cases of the GHA operator such

as the arithmetic mean (AM), the WA, the OWA,

the OWQA, the HA, the AHA, the HQA and the

HGA. Note that we do not consider the hybrid

maximum and the hybrid minimum because

sometimes its results are inconsistent.

Assume an investor wants to invest some money

in an enterprise in order to get high profits. Initially,

he considers five possible alternatives.

In order to evaluate these investments, the

investor uses a group of experts. This group of

experts considers that the key factor is the economic

environment of the economy. After detailed

analysis, they consider five possible situations for

the economic environment:

= Very bad, S

= Bad,

= Normal, S

= Good, S

= Very good. The

expected results depending on the state of nature S

and the alternative A

are shown in Table 1.

Table 1: Payoff matrix.

30 60 50 80 20

30 30 90 60 40

70 40 50 20 60

50 70 30 40 50

90 10 10 70 70

In this example, we assume the following

weighting vector for all the cases of the WA and the

OWA operator:

W = (0.1, 0.1, 0.2, 0.3, 0.3).

With this information, it is possible to aggregate

it in order to take a decision. First, we consider the

results obtained with some basic aggregation

operators. The results are shown in Table 2.

Table 2: Aggregated results 1.

Max Min AM WA OWA

80 20 48 49 39

90 30 50 54 44

70 20 48 45 41

70 30 48 45 40

90 10 50 54 36

As we can see, the optimal investment is

different depending on the operator used.

In the following, we will consider other

particular cases of the GHA operator with more

complexity. The results are shown in Table 3.

Table 3: Aggregated results 2.

OWQ HA AHA HQA HGA

43.4 36.5 61.5 46.9 29.4

45.0 39 69 49.7 28.3

44.1 36 54 41.1 32.1

44.6 37 53 40.3 34.4

48.3 34.5 73.5 51.4 17.5

ICEIS 2008 - International Conference on Enterprise Information Systems

470

Again, we can see that the optimal investment is

not the same for all the aggregations used. Note that

other types of GHA operators may be used in the

analysis such as the ones explained in Section 4.

A further interesting issue is to establish an

ordering of the investments. This is very useful

when the investor wants to consider more than one

alternative. The results are shown in Table 4.

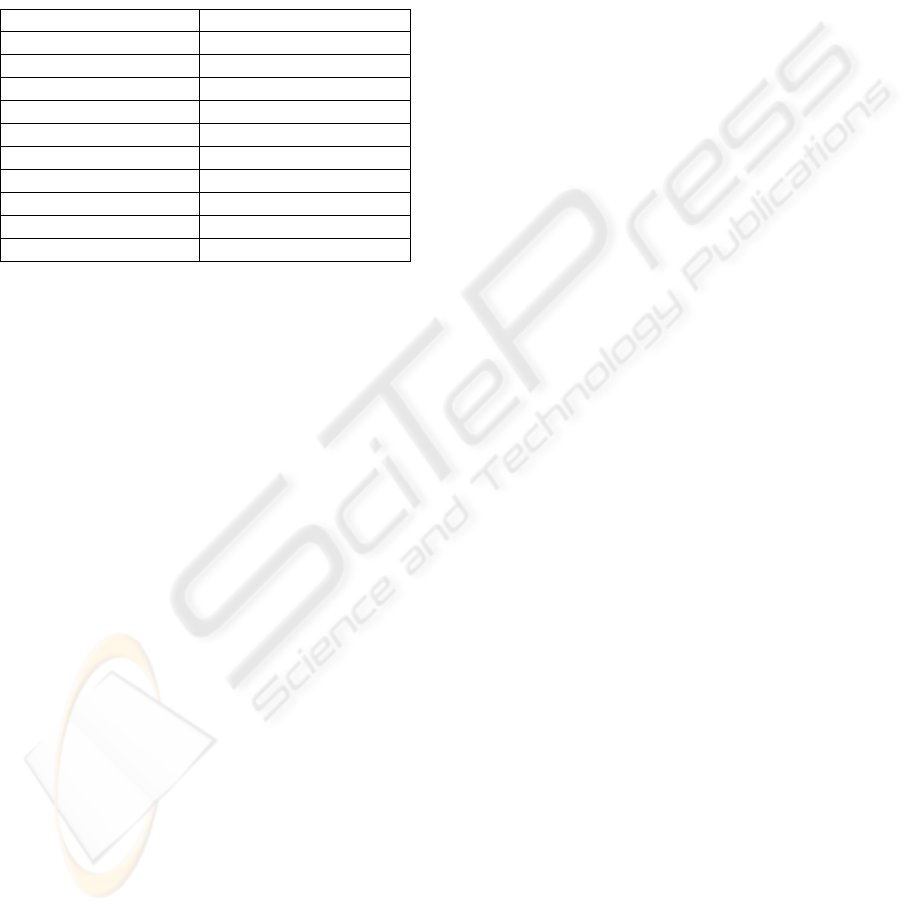
Table 4: Ordering of the investments.

Ordering

Max

⎬A

Min

⎬A

OWA

⎬A

OWQA

⎬A

AHA

⎬A

HQA

⎬A

HGA

⎬A

As we can see, we get different orderings of the

investments depending on the aggregation operator

used.

7 CONCLUSIONS

We have introduced the generalized hybrid

averaging (GHA) operator. It is a generalization of

the hybrid averaging (HA) operator by using

generalized means. We have seen that it is very

useful when we want to consider subjective

probabilities and the attitudinal character of the

decision maker in the same problem. With this

generalization we have found different special cases

such as the hybrid geometric averaging (HGA), the

hybrid quadratic averaging (HQA), the WA, the

OWA operator, the OWG operator, etc. We have

further generalized the GHA operator by using

quasi-arithmetic means. Then, we have obtained the

quasi-HA operator.

We have ended the paper with an application of

the new approach in a decision making problem. In

this case, we have focussed in a financial problem

where we have seen the usefulness of the new

approach in the selection of investments.

In future research, we expect to develop further

extensions to the GHA operator by adding new

characteristics in the problem such as the use of

inducing variables.

REFERENCES

Beliakov, G., 2005. Learning Weights in the Generalized

OWA Operators. Fuzzy Optimization and Decision

Making, 4, pp. 119-130.

Calvo, T., Mayor, G., Mesiar, R., 2002. Aggregation

Operators: New Trends and Applications. Physica-

Verlag, New York.

Fodor, J., Marichal, J.L., Roubens, M., 1995. Characte-

rization of the ordered weighted averaging operators.

IEEE Transactions on Fuzzy Systems, 3, pp. 236-240.

Karayiannis, N., 2000. Soft Learning Vector Quantization

and Clustering Algorithms Based on Ordered

Weighted Aggregation Operators. IEEE Transactions

on Neural Networks, 11, pp. 1093-1105.

Merigó, J.M., 2007. New extensions to the OWA operator

and its application in business decision making.

Unpublished thesis (in Spanish), Department of

Business Administration, University of Barcelona.

Merigó, J.M. Casanovas, M., 2007. The fuzzy generalized

OWA operator. In Proceedings of the XIVth Congress

of International Association for fuzzy-set management

and economy (SIGEF). Poiana-Brasov, Romania, pp.

504-517.

Merigó, J.M., Gil-Lafuente, A.M., 2007. The induced

generalized OWA operator. In Proceedings of the 5th

EUSFLAT Conference. Ostrava, Czech Republic, vol.

2, pp. 463-470.

Xu, Z.S., 2004. A method based on linguistic aggregation

operators for group decision making with linguistic

preference relations. Information Sciences, 166, pp.

19-30.

Xu, Z.S., 2006. A Note on Linguistic Hybrid Arithmetic

Averaging Operator in Multiple Attribute Group

Decision Making with Linguistic Information. Group

Decision and Negotiation, 15, pp. 593-604.

Xu, Z.S., Da, Q.L., 2003. An overview of operators for

aggregating the information. International Journal of

Intelligent Systems, 18, pp. 953-969.

Yager, R.R., 1988. On Ordered Weighted Averaging

Aggregation Operators in Multi-Criteria Decision

Making. IEEE Transactions on Systems, Man and

Cybernetics, B 18, pp. 183-190.

Yager, R.R., 1993. Families of OWA operators. Fuzzy Sets

and Systems, 59, pp. 125-148.

Yager, R.R., 1996. Quantifier guided aggregation using

OWA operators. International Journal of Intelligent

Systems, 11, pp. 49-73.

Yager, R.R., 2004. Generalized OWA Aggregation

Operators. Fuzzy Optimization and Decision Making,

3, pp. 93-107.

Yager, R.R., Filev, D.P., 1994. Parameterized andlike and

orlike OWA Operators. International Journal of

General Systems, 22, pp. 297-316.

Yager, R.R., Kacprzyk, J., 1997. The Ordered Weighted

Averaging Operators: Theory and Applications.

Kluwer Academic Publishers, Norwell, MA.

THE GENERALIZED HYBRID AVERAGING OPERATOR AND ITS APPLICATION IN FINANCIAL DECISION

MAKING

471