FUZZY INDUCED AGGREGATION OPERATORS IN DECISION
MAKING WITH DEMPSTER-SHAFER BELIEF STRUCTURE
José M. Merigó and Montserrat Casanovas
Department of Business Administration, University of Barcelona, Av. Diagonal 690, 08034 Barcelona, Spain
Keywords: Aggregation operators, Decision making, Dempster-Shafer theory of evidence, Financial decision making.
Abstract: We develop a new approach for decision making with Dempster-Shafer theory of evidence when the
available information is uncertain and it can be assessed with fuzzy numbers. With this approach, we are
able to represent the problem without losing relevant information, so the decision maker knows exactly
which are the different alternatives and their consequences. For doing so, we suggest the use of different
types of fuzzy induced aggregation operators in the problem. As a result, we get new types of fuzzy induced
aggregation operators such as the belief structure – fuzzy induced ordered weighted averaging (BS-FIOWA)
operator. We also develop an application of the new approach in a financial decision making problem.
1 INTRODUCTION
The Dempster-Shafer (D-S) theory of evidence
(Dempster, 1967; Shafer, 1976) provides a unifying
framework for representing uncertainty because it
includes the situations of risk and ignorance as
special cases. For further reading on the D-S theory,
see (Yager and Liu, 2008).
Usually, when using the D-S theory it is assumed
that the available information are exact numbers
(Engemann et al., 1994; Merigó and Casanovas,
2007; Yager, 1992; 2004). However, this may not be
the real situation found in the decision making
problem because often, the available information is
vague or imprecise and it is not possible to analyze it
with exact numbers. Then, a better approach may be
the use of fuzzy numbers (FN) because it considers
the best and worst possible scenarios and a lot of
others that could occur. When using FNs, we will
follow the ideas of (Chang and Zadeh, 1972; Dubois
and Prade, 1980; Kaufmann and Gupta, 1985).
Going a step further, the aim of this paper is to
suggest the use of different types of fuzzy induced
aggregation operators for aggregating the informa-
tion in decision making with D-S theory. The reason
for using various types of aggregation operators is
that we want to show that the fuzzy decision making
problem with D-S theory can be modelled in
different ways depending on the interests of the
decision maker. We will use the fuzzy induced
ordered weighed averaging (FIOWA) operator
because it provides a parameterized family of
aggregation operators that include the fuzzy
maximum, the fuzzy minimum, the fuzzy average
(FA), the fuzzy weighted average (FWA) and the
fuzzy OWA (FOWA), among others. Then, we will
get a new aggregation operator that we will call the
belief structure - FIOWA (BS-FIOWA) operator.
We also develop an application of this new model in
a business decision making problem.
In order to do so, the remainder of the paper is
organized as follows. In Section 2, we briefly
describe some basic concepts. In Section 3, we
present the new approach about using fuzzy induced
aggregation operators in decision making with D-S
theory. Finally, in Section 4 we develop an
application of the new approach.
2 PRELIMINARIES
2.1 Fuzzy Numbers
The FN was introduced by (Chang and Zadeh,
1972). Since then, it has been studied by a lot of
authors such as (Kaufmann and Gupta, 1985).
A FN is a fuzzy subset (Zadeh, 1965) of a
universe of discourse that is both convex and normal
(Kaufmann and Gupta, 1985). Note that the FN may
be considered as a generalization of the interval
number (Moore, 1966) although it is not strictly the
548
M. Merigó J. and Casanovas M. (2008).
FUZZY INDUCED AGGREGATION OPERATORS IN DECISION MAKING WITH DEMPSTER-SHAFER BELIEF STRUCTURE.
In Proceedings of the Tenth International Conference on Enterprise Information Systems - AIDSS, pages 548-552
DOI: 10.5220/0001711105480552
Copyright
c
 SciTePress

same because the interval numbers may have
different meanings.
In the literature, we find a wide range of FNs
(Kaufmann and Gupta, 1985). For example, a
trapezoidal FN (TpFN) A of a universe of discourse
R can be characterized by a trapezoidal membership
function
),( aaA = such that
).()(
),()(
344
121
aaaa
aaaa
−−=
−+=
αα
αα
(1)
where
α ∈ [0, 1] and parameterized by (a
1
, a
2
, a
3
,
a
4
) where a
1
≤ a
2
≤ a
3
≤ a
4
, are real values. Note that
if a
1
= a
2
= a
3
= a
4
, then, the FN is a crisp value and
if a
2
= a
3
, the FN is represented by a triangular FN
(TFN). Note that the TFN can be parameterized by
(a
1
, a
2
, a
4
).
2.2 Fuzzy Induced OWA Operator
The FIOWA (or FN-IOWA) operator was
introduced by S.J. Chen and S.M. Chen (2003). It is
an extension of the OWA operator (Yager, 1988;
Yager and Kacprzyk, 1997) that uses uncertain
information represented by FNs. It also uses a
reordering process different from the values of the
arguments. In this case, the reordering step is based
on order inducing variables. It is defined as follows.
Definition 1. Let Ψ be the set of FN. A FIOWA
operator of dimension n is a mapping FIOWA: Ψ
n
→ Ψ that has an associated weighting vector W of
dimension n such that w
j
∈ [0, 1] and
∑
=
=
n
j
j
w
1
1
,
then:
FIOWA(〈u
1
,ã
1
〉, …, 〈u
n
,ã
n
〉) =
∑
=
n
j
jj
bw
1
(2)
where b
j
is the ã
i
value of the FIOWA pair 〈u
i
, ã
i
〉
having the jth largest u
i
, u
i
is the order inducing
variable and ã
i
is the argument variable represented
in the form FN.
2.3 Dempster-Shafer Theory of
Evidence
The D-S theory provides a unifying framework for
representing uncertainty as it can include the
situations of risk and ignorance as special cases. It is
defined as follows.
Definition 2. A D-S belief structure defined on a
space X consists of a collection of n nonnull subsets
of X, B
j
for j = 1,…,n, called focal elements and a
mapping m, called the basic probability assignment,
defined as, m: 2
X
→ [0, 1] such that:
(1) m(B
j
) ∈ [0, 1].
(2)
)(
1
∑
=
n
j
j
Bm = 1. (3)
(3) m(A) = 0, ∀ A ≠ B
j.
.
3 FIOWA OPERATORS IN
DECISION MAKING WITH D-S
THEORY OF EVIDENCE
In this Section, we describe the process to follow
when using fuzzy induced aggregation operators in
decision making with D-S theory.
3.1 Decision Making Approach
A new method for decision making with D-S theory
is possible by using FN aggregation operators in the
problem. Going a step further, we see that it is
possible to use fuzzy induced aggregation operators
such as the FIOWA operator. Note it is also possible
to consider other cases such as the use of different
types of fuzzy induced generalized means and fuzzy
induced quasi-arithmetic means. The motivation for
using FNs appears because sometimes, the available
information is not clear and it is necessary to assess
it with another approach such as the use of FNs.
Although the information is uncertain and it is
difficult to take decisions with it, at least we can
represent the best and worst possible scenarios and
the possibility that the internal values of the fuzzy
interval will occur. The decision process can be
summarized as follows.
Assume we have a decision problem in which
we have a collection of alternatives {A
1
, …, A
q
} with
states of nature {S
1
, …, S
n
}. ã
ih
is the uncertain
payoff, given in the form of FNs, to the decision
maker if he selects alternative A
i
and the state of
nature is S
h
. The knowledge of the state of nature is
captured in terms of a belief structure m with focal
elements B
1
, …, B
r
and associated with each of these
focal elements is a weight m(B
k
). The objective of
the problem is to select the alternative which gives
the best result to the decision maker. In order to do
so, we should follow the following steps:
Step 1: Calculate the uncertain payoff matrix.
Step 2: Calculate the belief function m about the
states of nature.
FUZZY INDUCED AGGREGATION OPERATORS IN DECISION MAKING WITH DEMPSTER-SHAFER BELIEF
STRUCTURE
549

Step 3: Calculate the attitudinal character of the
decision maker
α (W) (Yager, 1988).
Step 4: Calculate the collection of weights, w, to
be used in the FIOWA aggregation for each different
cardinality of focal elements. (Merigó, 2007; Yager,
1988; 1993).
Step 5: Determine the uncertain payoff
collection, M
ik
, if we select alternative A
i
and the
focal element B
k
occurs, for all the values of i and k.
Hence M
ik
= {a
ih
| S
h
∈ B
k
}.
Step 6: Calculate the fuzzy induced aggregated
payoff, V
ik
= FIOWA(M
ik
), using Eq. (2), for all the
values of i and k.
Step 7: For each alternative, calculate the
generalized expected value, C
i
, where:
∑
=
=
r
r
kiki
BmVC
1
)(
(4)
Step 8: Select the alternative with the largest C
i
as the optimal.
3.2 Using FIOWA Operators in Belief
Structures
Analyzing the aggregation in Steps 6 and 7 of the
previous subsection, it is possible to formulate in
one equation the whole aggregation process. We will
call this process the belief structure – FIOWA (BS-
FIOWA) aggregation. It can be defined as follows.
Definition 3. A BS-FIOWA operator is defined by
∑∑
=
==
r
k
q
j
jjki
k
k
kk
bwBmC
11
)(
(5)
where w
j
k
is the weighting vector of the kth focal
element such that
1
1
=
∑
=
n
j
j
k
w and w
j
k
∈ [0,1], b
j
k
is the j
k
th largest of the ã
i
k
and the ã
i
k
are FNs, and
m(B
k
) is the basic probability assignment.
Note that q
k
refers to the cardinality of each focal
element and r is the total number of focal elements.
The BS-FIOWA operator is monotonic, commu-
tative, bounded and idempotent.
Note that it is possible to distinguish between
descending (BS-DFIOWA) and ascending (BS-
AFIOWA) orders.
3.3 Families of BS-FIOWA Operators
By using a different manifestation in the weighting
vector of the FIOWA operator, we are able to deve-
lop different families of FIOWA and BS-FIOWA
operators. As we can see in Definition 3, each focal
element uses a different weighting vector in the
aggregation with the FIOWA operator. Therefore,
the analysis needs to be done individually.
For example, the maximum is obtained if w
p
= 1
and w
j
= 0, for all j ≠ p, and u
p
= Max{ã
i
}. The fuzzy
minimum is obtained if w
p
= 1 and w
j
= 0, for all j ≠
p, and u
p
= Min{ã
i
}. The FA is found when w
j
= 1/n,
for all ã
i
. The FWA is obtained if u
i
> u
i+1
, for all i,
and the FOWA operator is obtained if the ordered
position of u
i
is the same than the ordered position of
b
j
such that b
j
is the jth largest of ã
i
.
Other families of FIOWA operators could be
used in the BS-FIOWA operator such as the step-
FIOWA, and the olympic-FIOWA, among others.
For more information, see (Merigó, 2007).
For example, the step-FIOWA operator is found
when w
k
= 1 and w
j
= 0, for all j ≠ k. The olympic-
FIOWA operator is found if w
1
= w
n
= 0, and for all
others w
j
= 1/(n − 2).
Finally, if we assume that all the focal elements
use the same weighting vector, then, we can refer to
these families as the BS-fuzzy maximum, the BS-
fuzzy minimum, the BS-FA, the BS-FWA, the BS-
S-FIOWA, the BS-olympic-FIOWA, etc.
4 APPLICATION IN FINANCIAL
DECISION MAKING
In the following, we are going to develop an
application of the new approach in a decision
making problem. We will analyze the selection of
financial strategies where an enterprise is looking for
its optimal financial strategy for the next year. Note
that other applications could be developed such as
the selection of human resources, etc.
Assume an enterprise is planning its financial
strategy for the next year and considers 4 possible
financial strategies to follow: {A
1
, A
2
, A
3
, A
4
}.
In order to evaluate these financial strategies, the
group of experts considers that the key factor is the
economic situation of the company for the next year.
After careful analysis, the experts have considered
five possible situations that could happen in the
future: S
1
= Very bad, S
2
= Bad, S
3
= Regular, S
4
=
Good, S
5
= Very good.
ICEIS 2008 - International Conference on Enterprise Information Systems
550

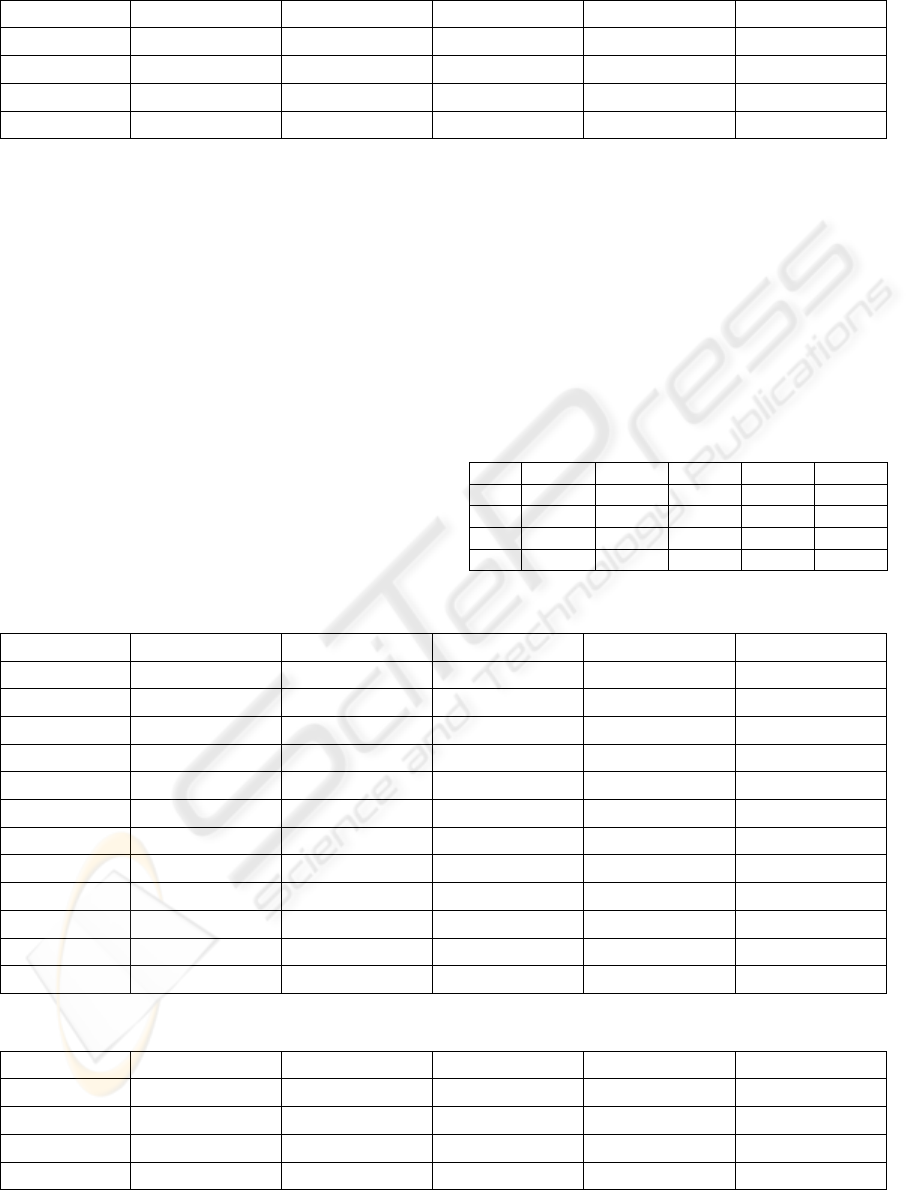
Table 1: Fuzzy payoff matrix.
S
1
S
2
S
3
S
4
S
5
A
1
(50,60,70) (30,40,50) (30,40,50) (60,70,80) (40,50,60)
A
2
(10,20,30) (20,30,40) (50,60,70) (50,60,70) (80,90,100)
A
3
(30,40,50) (50,60,70) (40,50,60) (40,50,60) (40,50,60)
A
4
(60,70,80) (40,50,60) (30,40,50) (30,40,50) (30,40,50)
Depending on the uncertain situations that could
happen in the future, the experts establish the payoff
matrix. As the future states of nature are very
imprecise, the experts cannot determine exact
numbers in the payoff matrix. Instead, they use FNs
to calculate the future benefits of the enterprise
depending on the state of nature that happens in the
future and the financial strategy selected. Note that
in this example the experts use TFN. Then, they can
calculate the best and worst possible scenarios and
represent all the internal results with a membership
level. The results are shown in Table 1.
After careful analysis of the information, the
experts have obtained some probabilistic informa-
tion about which state of nature will happen in the
future. This probabilistic information is represented
by the following belief structure about the states of
nature.
Focal element
B
1
= {S
1
, S
2
, S
3
} = 0.3
B
2
= {S
3
, S
4
, S
5
} = 0.3
B
3
= {S
2
, S
3
, S
4
, S
5
} = 0.4
The attitudinal character of the enterprise is very
complex because it involves the opinion of different
members of the board of directors. Therefore, the
experts use order inducing variables for analysing
the attitudinal character of the enterprise. The results
are shown in Table 2.
Table 2: Inducing variables.
S
1
S
2
S
3
S
4
S
5
A
1
7 6 4 9 2
A
2
1 5 7 9 3
A
3
4 3 8 6 5
A
4
2 5 6 7 8
Table 3: Fuzzy aggregated results.
FA FWA FOWA FIOWA AFIOWA
V
11
(36.6,46.6,56.6) (36,46,56) (36,46,56) (36,46,56) (38,48,58)
V
12
(43.3,53.3,63.3) (43,53,63) (42,52,62) (43,53,63) (45,55,65)
V
13
(40,50,60) (42,52,62) (38,48,58) (39,49,59) (41,51,61)
V
21
(26.6,36.6,46.6) (29,39,49) (25,35,45) (25,35,45) (29,39,49)
V
22
(60,70,80) (62,72,82) (59,69,79) (62,72,82) (59,69,79)
V
23
(50,60,70) (53,63,73) (47,57,67) (50,60,70) (50,60,70)
V
31
(40,50,60) (40,50,60) (39,49,59) (41,51,61) (40,50,60)
V
32
(40,50,60) (40,50,60) (40,50,60) (40,50,60) (40,50,60)
V
33
(42.5,52.5,62.5) (42,52,62) (42,52,62) (43,53,63) (43,53,63)
V
41
(43.3,53.3,63.3) (42,52,62) (42,52,62) (45,55,65) (42,52,62)
V
42
(30,40,50) (30,40,50) (30,40,50) (30,40,50) (30,40,50)
V
43
(32.5,42.5,52.5) (32,42,52) (32,42,52) (33,43,53) (32,42,52)
Table 4: Fuzzy generalized expected value.
FA FWA FOWA FIOWA AFIOWA
A
1
(40,50,60) (40.5,50.5,60.5) (38.6,48.6,58.6) (39.3,49.3,59.3) (41.3,51.3,61.3)
A
2
(46,56,66) (48.5,58.5,68.5) (44,54,64) (46.1,56.1,66.1) (46.4, 56.4, 66.4)
A
3
(41,51,61) (40.8,50.8,60.8) (40.5,50.5,60.5) (41.5,51.5,61.5) (41.2,51.2,61.2)
A
4
(35,45,55) (34.4,44.4,54.4) (34.4,44.4,54.4) (35.7,45.7,55.7) (34.4,44.4,54.4)
FUZZY INDUCED AGGREGATION OPERATORS IN DECISION MAKING WITH DEMPSTER-SHAFER BELIEF
STRUCTURE
551

The experts establish the following weighting
vectors for the FIOWA:
Weighting vector
W
3
= (0.3, 0.3, 0.4)
W
4
= (0.2, 0.2, 0.3, 0.3)
W
5
= (0.1, 0.2, 0.2, 0.2, 0.3)
With this information, we can obtain the aggre-
gated payoffs. The results are shown in Table 3.
Once we have the aggregated results, we have to
calculate the fuzzy generalized expected value. The
results are shown in Table 4.
As we can see, depending on the fuzzy aggre-
gation operator used, the results and decisions may
be different. Note that in this case, our optimal
choice is the same for all the aggregation operators
but in other situations we may find different
decisions between each aggregation operator.
A further interesting issue is to establish an ordering
of the financial strategies. Note that this is very
useful when the decision maker wants to consider
more than one alternative. As we can see, depending
on the aggregation operator used, the ordering of the
financial strategies may be different. Note that in
this example the results are clear being A
2
the
optimal choice and the ordering: A
2
⎬A
3
⎬A
1
⎬A
4
excepting for the AFIOWA operator, where the
ordering is:
A
2
⎬A
1
⎬A
3
⎬A
4
.
5 CONCLUSIONS
We have studied the D-S theory of evidence in
decision making with uncertain information
represented in the form of FNs. With this approach,
we have been able to assess the information in a
more complete way because in this model we
consider the different scenarios that could happen in
the problem. For doing so, we have used different
types of fuzzy induced aggregation operators in the
decision process such as the FIOWA operator. Then,
we have obtained the BS-FIOWA operator.
We have also developed an application of the
new approach in a business decision making
problem about selection of financial strategies. We
have seen the usefulness of this approach about
using probabilities and FIOWAs in the same
problem. We have also seen that depending on the
fuzzy induced aggregation operator used the results
may lead to different decisions.
In future research, we expect to develop further
extensions to this approach by adding new
characteristics in the problem and applying it to
other decision making problems.
REFERENCES
Chang, S.S.L., Zadeh, L.A., 1972. On fuzzy mapping and
control, IEEE Transactions on Systems, Man and
Cybernetics, 2, pp. 30-34.
Chen, S.J., Chen, S.M., 2003. A new method for handling
multi-criteria fuzzy decision making problems using
FN-IOWA operators, Cybernetics and Systems, 34, pp.
109-137.
Dempster, A.P., 1967. Upper and lower probabilities
induced by a multi-valued mapping, Annals of
Mathematical Statistics, 38, pp. 325-339.
Dubois, D., Prade, H., 1980. Fuzzy Sets and Systems:
Theory and Applications, Academic Press, New York.
Engemann, K.J., Miller, H.E., Yager, R.R., 1996. Decision
making with belief structures: an application in risk
management, International Journal of Uncertainty,
Fuzziness and Knowledge-Based Systems, 4, pp. 1-26.
Kaufmann, A., Gupta, M.M., 1985. Introduction to fuzzy
arithmetic, Publications Van Nostrand, Rheinhold.
Merigó, J.M., 2007. New extensions to the OWA operator
and its application in business decision making.
Unpublished thesis (in Spanish), Department of
Business Administration, University of Barcelona.
Merigó, J.M., Casanovas, M. 2007. Decision making with
Dempster-Shafer theory using induced aggregation
operators. In Proceedings of the 4th International
Summer School on AGOP, Ghent, Belgium, pp. 95-
100.
Moore, R.E,, 1966. Interval Analysis, Prentice Hall,
Englewood Cliffs, NJ.
Shafer, G.A., 1976. Mathematical Theory of Evidence,
Princeton University Press, Princeton, NJ.
Yager, R.R., 1988. On Ordered Weighted Averaging
Aggregation Operators in Multi-Criteria Decision
Making. IEEE Transactions on Systems, Man and
Cybernetics, B 18, pp. 183-190.
Yager, R.R., 1992. Decision Making Under Dempster-
Shafer Uncertainties, International Journal of General
Systems, 20, pp. 233-245.
Yager, R.R., 1993. Families of OWA operators. Fuzzy Sets
and Systems, 59, pp. 125-148.
Yager, R.R., 2004. Uncertainty modeling and decision
support, Reliability Engineering and System Safety,
85, pp. 341-354.
Yager, R.R., Kacprzyk, J., 1997. The Ordered Weighted
Averaging Operators: Theory and Applications.
Kluwer Academic Publishers, Norwell, MA.
Yager, R.R., Liu, L. 2008. Classic Works in the Dempster-
Shafer Theory of Belief Functions. Springer-Verlag,
Berlin.
Zadeh, L.A., 1965. Fuzzy Sets, Information and Control,
8, pp. 338-353.
ICEIS 2008 - International Conference on Enterprise Information Systems
552