MAGNETOMETRY USING ELECTROMAGNETICALLY INDUCED

TRANSPARENCY IN A ROOM TEMPERATURE VAPOUR CELL

Developing an Optical Magnetometer that Utilises the Steep Dispersion Curve

Observed in EIT to Detect Time Varying Magnetic Fields

Melody R. Blackman and Benjamin T. H. Varcoe

School of Physics and Astronomy, University of Leeds, Leeds LS2 9JT, U.K.

Keywords:

Magnetometry, EIT.

Abstract:

The physiological importance of magnetic signals within biological systems has been investigated with ever in-

creasing sensitivities over the last decade. Currently superconducting quantum interference devices (SQUIDs)

are at the forefront of bio-magnetic diagnostics. In this research we aim to build an optics based magnetometer

that can compete with the sensitivity of the SQUID but that runs at a lower start up and operational cost. To

do this we intend to use the steep dispersion curve observed in the atomic physics effect electromagnetically

induced transparency. This magnetometer can operate at room temperature, its design is a convenient method

for monitoring bio-magnetic ﬁelds, making this technology an affordable technique for further bio-magnetic

diagnostics.

1 INTRODUCTION

Bio-magnetism is a fast developing ﬁeld of research

as its non-invasive applications make it a very desir-

able diagnostic tool. The presence of time varying

electric ﬁelds in the human body has been greatly

studied over the last hundred years. Electrocardio-

graphs (ECG) measure these ﬁelds as potential dif-

ferences on the skin’s surface, with emphasis on the

torso (Nash et al., 2002; Ramanathan et al., 2004).

However each of these electric ﬁelds is accompanied

by a weak magnetic ﬁeld that also holds information

on the condition of the organ. Unlike electric ﬁelds

the magnetic, do not suffer from varying attenuation

at they travel through the different types of tissue. An-

other advantage of measuring the magnetic ﬁeld is

the ability to make direct measurements of an organ’s

ﬁeld map instead of the potential difference between

two points.

The largest magneto-physiological signal in the

body is created by the heart’s QRS peak which corre-

sponds to the contraction and relaxation of the cardiac

muscle during a single heart beat. The magnetic ﬁelds

associated with this process are of the order of tens

of picotesla, although the more clinically important

signals that are unobservable with ECG are formed

by the cardiac conducting system, which are super-

imposed on the ﬁeld and are less than 1 pT (Fenici

et al., 1983).

Currently at the forefront of magnetometer clini-

cal trials are Superconducting Quantum Interference

Devices(SQUIDs), which have displayed sensitivities

as high a few fT/

√

Hz (Vodel and Makiniemi, 1992).

Since their implementation they have been used to

form dynamic mapping of both the brain and heart’s

magnetic ﬁelds (H¨am¨al¨ainen et al., 1993; Fagaly,

2006). The limitations with SQUIDs come from their

operational requirements, starting with their need for

a cryostat, crucial for the 4 Kelvin cooling of the su-

perconducting materials that make up the Josephson

junctions used in the detector heads. This causes the

overall cost of the SQUIDs to be very high (in the

order of hundreds of thousands of pounds) meaning

there is a place in the market for cheaper alternatives.

It is in the ﬁeld of optical magnetometry where

the most promising competition to SQUIDs can be

found (Bloom, 1962; Cohen-Tannoudji et al., 1969;

Nagel et al., 1998; Budker et al., 2000; Bison et al.,

2003; Kominis et al., 2003) and it is in this ﬁeld that

our research is based. These methods allow for low

cost, non-invasive, non-contact highly sensitive mag-

netic ﬁeld detectors that can be made and operated

at a fraction of the cost of a SQUID. The ability to

measure bio-magnetic signals without contact makes

173

R. Blackman M. and T. H. Varcoe B. (2009).

MAGNETOMETRY USING ELECTROMAGNETICALLY INDUCED TRANSPARENCY IN A ROOM TEMPERATURE VAPOUR CELL - Developing an

Optical Magnetometer that Utilises the Steep Dispersion Curve Observed in EIT to Detect Ti.

In Proceedings of the International Conference on Biomedical Electronics and Devices, pages 173-177

DOI: 10.5220/0001434501730177

 SciTePress

it very useful for fetal cardiac diagnostics (Comani

et al., 2004) as well as the more standard magneto-

cardiographs (MCG) and in the case of burns victims

where contact is not an option.

When making observations of magnetic ﬁelds us-

ing atom-light interactions one may start by thinking

of the Zeeman effect. The Zeeman effect explains the

perturbation of atomic energy levels by means of an

applied magnetic ﬁeld, which couples to the magnetic

moment of the atomic electrons. Here we still utilise

the Zeeman effect but we need to go further into the

detail of the energy level shifts by discussing the ac-

Stark shift (also called the Autler-Townes effect) as

these are essential to the mechanism needed for mak-

ing our magnetometer more sensitive than a device

utilising just the Zeeman effect.

In this experiment we use a form of coherent pop-

ulation trapping (CPT) (Belﬁ et al., 2007) called Elec-

tromagnetically Induced Transparency (EIT) (Boller

et al., 1991; Scully, 1991; Harris, 1997). EIT is

a quantum interference effect whereby an opaque

medium is made transparent to a probe laser under

certain resonance conditions. To understand how the

many mechanisms that create EIT work together we

shall start by considering a three level system in the Λ

conﬁguration. A depiction of the Λ transition used in

this experiment is displayed in ﬁgure 1. In this case

the ground states are degenerate, resulting in a sym-

metric transition so the coupling and probe beams can

be resonant with either m

= ±1 levels by switching

the sign of the circular polarisation (σ

+/−

The intense coupling beam drives the transition

into the excited state; at this point an ac Stark shift

occurs at the upper energy level, whereby the atomic

absorption line splits into an Autler-Townes doublet,

symmetric about the transition’s unshifted resonance.

We now consider the processes of EIT in more de-

tail. We are fortunate that a thorough mathematical

understanding of EIT is available allowing us to sim-

ulate the system before building an experiment. This

is presented in the following paragraphs.

The following time dependent interaction Hamil-

tonian describes the atom-light coupling for a system

with EIT;

int

= −

[Ω

(t)

i∆

+ Ω

(t)

i∆

+ H.c]

(1)

where the Ω

c/p

(t) are the Rabi frequencies of the

coupling and probe ﬁelds respectively,

= |iihj| is

the atomic projection operator, ∆ is the detuning and

H.c is the Hermitian conjugate. It is this Hamilto-

nian that is inserted into the Master equation and al-

lows us to calculate the atomic density operators (ρ).

The solutions of the Master equation are fundamental

Figure 1: The Λ conﬁguration for our

Rb transition show-

ing the possible detuning (∆) of the m

levels in the pres-

ence of a coupling and probe beam. σ

+/−

denotes the sign

of the circular polarisation. As the applied magnetic ﬁeld

oscillates our system will rotate through these three Λ sys-

tems.

to understanding how the photons are no longer ab-

sorbed at the resonance peak, showing us that when

the coupling and probe beams interact they can cancel

each others absorptive terms producing a dark state by

means of destructive quantum interference. How this

affects the absorption can be seen by looking at the

real and imaginary parts of the linear susceptibility

(χ), given by;

χ(−ω

,ω

) =

|µ

atom

4δ(|Ω|

−4δ∆) −4∆γ

||Ω|

+ (γ

+ i2∆)(γ

+ i2δ)|

8δ

+ 2γ

(|Ω|

+ γ

)

||Ω|

+ (γ

+ i2∆)(γ

+ i2δ)|

(2)

where the two photon detuning, δ = ∆

− ∆

−(ω

−ω −c) and is derived from the single

photon detuning, ∆ = ∆

= ω

−ω

. The coherent

decay between states is γ, in this case γ

= 0. The

probe beam term (γ

) is neglected from the equa-

tion as it is assumed it has no observable effect on the

atom’s behaviour, this is in part due to the two photon

Raman resonance which occurs in the presence of the

dark states (Fleischhauer et al., 2005).

The linear susceptibility, as seen in equation 2

holds all the important information about the atom’s

absorption and refractive index and as these are both

BIODEVICES 2009 - International Conference on Biomedical Electronics and Devices

174

−1 −0.5 0 0.5 1

−0.02

−0.01

0.01

0.02

Linear susceptibility (χ) of a system with EIT

∆/γ

Re[χ]

−1 −0.5 0 0.5 1

0.01

0.02

0.03

∆/γ

Im[χ]

Figure 2: This ﬁgure shows ﬁrst the real Re[χ] then the

imaginary Im[χ] parts of the susceptibility calculated for the

D1 line of

Rb using equation 2.

altered in a EIT system it gives us a clear picture of

how the atom-light interaction has changed. Figure 2

displays how all these effects alter the real (Re[χ]) and

the imaginary (Im[χ]) part of χ.

∆ B

∆ V

Figure 3: The top line is a representation of Re[χ] for a

standard saturation spectroscopy dispersion curve, while the

lower is Re[χ] in the presence of EIT. The ﬁgure displays

how the sensitivity to magnetic ﬁeld is increased propor-

tionally to the gradient of the dispersion curve, where ∆B is

the amplitude of the input and ∆V is the amplitude of the

response.

The key to why a system with EIT makes for a

good magnetometer is the steep dispersion curve. Fig-

ure 3 shows a representation of the difference that the

steep dispersion curve has on the atom’s response to

magnetic ﬁelds. Therefore the steeper one can make

the dispersion curve the better the magnetic detector

will be produced from it.

2 EXPERIMENTAL SETUP

The experiment currently runs using free space op-

tics and a diode laser. The laser is tuned to

794.985nm, which is the transition frequency of

the D1 (5

1/2(F=2)

1/2(F=1)

) line of rubidium 87

(

Rb). The

Rb is contained within vapour cell with

a buffer gas of, neon at 30Torr. We use

Rb as it al-

lows us the run the experiment at room temperature

with low powered laser beams. Figure 4 displays the

apparatus used in this experiment.

The laser is a commercially available diode laser,

from which beams are tapped off and sent to the di-

agnostic and stabilisation section of the experiment.

Contained within this setup is the reference

85/87

vapour cell, arranged in a saturation spectroscopy

retro-reﬂection conﬁguration. Using this along with

a wavemeter and a lock-in ampliﬁer we can lock the

laser to the resonance peak of the transition, stabilis-

ing the laser system.

Laser

Amplifier

Lock−in

Wavemeter

Lock−in

Oscilloscope

Signal

Generator

Diagnostics

Laser Stabilisation

50:50

PBS

50:50

Rb cell

ND filter

Data Collection

λ/2

λ/4

filter

Amplifier

3 layer

− metal

Rb cell

2 solenoids

Figure 4: A schematic of the apparatus used in this experi-

ment.

The main design of the experiment is a Sagnac in-

terferometer, which starts a single beam that is then

split, these two beams then counterpropagate around

a ring. The beams return to their entry point and leave

the interferometer where they interact and produce in-

terference fringes.

Before the light enters the interferometer it is cou-

pled into a single mode optical ﬁbre to clean the mode

MAGNETOMETRY USING ELECTROMAGNETICALLY INDUCED TRANSPARENCY IN A ROOM

TEMPERATURE VAPOUR CELL - Developing an Optical Magnetometer that Utilises the Steep Dispersion Curve

Observed in EIT to Detect Time Varying Magnetic Fields

175

for the experiment. We use a series of optics to pre-

pare the polarisations before splitting the circular po-

larised light with a 50:50 beam splitter into the in-

terferometer. It is here we obtain the σ

+/−

terms as

displayed in ﬁgure 1. Before entering the vapour cell

one of the beams is attenuated with a neutral density

ﬁlter (ND ﬁlter), making it into our weak probe beam.

Two solenoids surround the vapour cell within

three layers of µ-metal shielding. These are driven by

an arbitrary signal generator. The ﬁrst (modulation)

solenoid is driven with a square wave correspond-

ing to approximately 1nT of between 250-300KHz,

which is used as the reference for the signal recovery

lock-in ampliﬁer. The second solenoid is also con-

nected to the arbitrary signal generator and is driven

with a sine wave with frequencies between 2-8Hz.

The human heart has a frequency range of 1-1.67Hz,

with higher frequency components.

The raw signal is collected via a photodiode and

then connected to the input of the signal recovery

lock-in ampliﬁer. The lock-in ampliﬁer allows very

small signals to be extracted from the large amounts

of noise, using this system we have gained sensitiv-

ities in the range of a fetal heart beat. An example

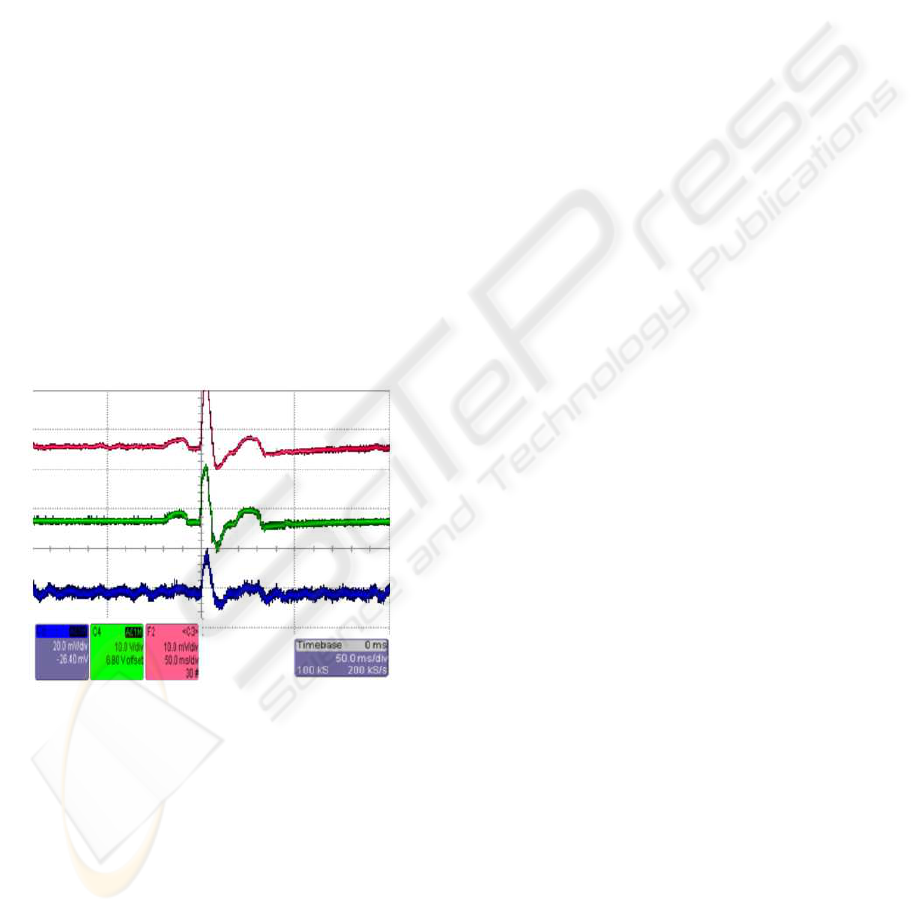
of test data taken using a simulated heartbeat is dis-

played in ﬁgure 5.

Figure 5: A sceen dump of data take on the experiment.

The centre trace is the raw input signal, a simulated QRS,

the lower trace is the raw data from the photodiode and the

upper trace is the 20 trace avarage.

3 CONCLUSIONS

With current data we can measure magnetic ﬁelds of

the order of a fetal heart beat. The experiment is

still at a very early stage of development, therefore

the current data acquisition method is not suitable for

clinical applications. However even at this stage the

experiment has shown a great deal of promise as a

potential MCG device. We expect, with some calibra-

tion, to obtain at least femtotelsa sensitivities (Fleis-

chhauer and Scully, 1994), with the possibility of us-

ing more optical ﬁbres and replacing the modulation

solenoid with an alternative scheme such as an acous-

tic or electro-optic modulator (AOM/EOM). These

improvements will move this research closer to being

a competitive device for performing clinical MCG tri-

als.

ACKNOWLEDGEMENTS

This work is funded by an EPSRC DTA.

REFERENCES

Belﬁ, J., Bevilacqua, G., Biancalana, V., Dancheva, Y., and

Moi, L. (2007). All optical sensor for automated mag-

netometry based on coherent population trapping.

Bison, G., Wynands, R., and Weis, A. (2003). Dynami-

cal mapping of the human cardiomagnetic ﬁeld with a

room-temperature, laser-optical sensor. Opt. Express,

11(8):904–909.

Bloom, A. L. (1962). Principles of operation of the rubid-

ium vapor magnetometer. Appl. Opt., 1(1):61–68.

Boller, K. J., Imamolu, A., and Harris, S. E. (1991). Obser-

vation of electromagnetically induced transparency.

Physical Review Letters, 66(20):2593+.

Budker, D., Kimball, D. F., Rochester, S. M., Yashchuk,

V. V., and Zolotorev, M. (2000). Sensitive magne-

tometry based on nonlinear magneto-optical rotation.

Physical Review A, 62(4):043403+.

Cohen-Tannoudji, C., Dupont-Roc, J., Haroche, S., and

Lalo¨e, F. (1969). Detection of the static magnetic ﬁeld

produced by the oriented nuclei of optically pumped

he3 gas. Physical Review Letters, 22(15):758+.

Comani, S., Mantini, D., Alleva, G., Di, L. S., and Romani,

G. L. (2004). Fetal magnetocardiographic mapping

using independent component analysis. Institute of

Physics Publishing Physiological Measurement.

Fagaly, R. L. (2006). Superconducting quantum interfer-

ence device instruments and applications. Review of

Scientiﬁc Instruments, 77(10).

Fenici, R., Romani, G., and Ern´e, S. (1983). High-

resolution magnetic measurements of human cardiac

electrophysiological events. Il Nuovo Cimento D,

2(2):231–247.

Fleischhauer, M., Imamoglu, A., and Marangos, J. P.

(2005). Electromagnetically induced transparency:

Optics in coherent media. Reviews of Modern Physics,

77(2).

Fleischhauer, M. and Scully, M. O. (1994). Quantum

sensitivity limits of an optical magnetometer based

on atomic phase coherence. Physical Review A,

49(3):1973+.

BIODEVICES 2009 - International Conference on Biomedical Electronics and Devices

176

H¨am¨al¨ainen, M., Hari, R., Ilmoniemi, R. J., Knuutila, J.,

and Lounasmaa, O. V. (1993). Magnetoencephalog-

raphytheory, instrumentation, and applications

to noninvasive studies of the working human brain.

Reviews of Modern Physics, 65(2):413+.

Harris, S. E. (1997). Electromagnetically induced trans-

parency. In Quantum Electronics and Laser Science

Conference, 1997. QELS ’97., Summaries of Papers

Presented at the, page 25.

Kominis, I. K., Kornack, T. W., Allred, J. C., and Romalis,

M. V. (2003). A subfemtotesla multichannel atomic

magnetometer. Nature, 422(6932):596–599.

Nagel, A., Graf, L., Naumov, A., Mariotti, E., Biancalana,

V., Meschede, D., and Wynands, R. (1998). Experi-

mental realization of coherent dark-state magnetome-

ters. Europhysics Letters, pages 31–36.

Nash, M. P., Bradley, C. P., Kardos, A., Pullan, A. J., and

Paterson, D. J. (2002). An experimental model to cor-

relate simultaneous body surface and epicardial elec-

tropotential recordings in vivo. Chaos, Solitons &

Fractals, 13(8):1735–1742.

Ramanathan, C., Ghanem, R. N., Jia, P., Ryu, K., and Rudy,

Y. (2004). Noninvasive electrocardiographic imag-

ing for cardiac electrophysiology and arrhythmia. Nat

Med, 10(4):422–428.

Scully, M. O. (1991). Enhancement of the index of refrac-

tion via quantum coherence. Physical Review Letters,

67(14):1855+.

Vodel, W. and Makiniemi, K. (1992). An ultra low noise dc

squid system for biomagnetic research. Measurement

Science and Technology, 3(12):1155–1160.

MAGNETOMETRY USING ELECTROMAGNETICALLY INDUCED TRANSPARENCY IN A ROOM

TEMPERATURE VAPOUR CELL - Developing an Optical Magnetometer that Utilises the Steep Dispersion Curve

Observed in EIT to Detect Time Varying Magnetic Fields

177