
HIGHER ORDER SLIDING MODE CONTROL FOR CONTINUOUS
TIME NONLINEAR SYSTEMS BASED ON OPTIMAL CONTROL
Zhiyu Xi and Tim Hesketh
School of Electrical Engineering & Telecommunications, University of New South Wales, Kensington, NSW, Australia
Keywords:
Sliding mode, High order, Nonlinear, Optimal control.
Abstract:
This paper addresses higher order sliding mode control for continuous nonlinear systems. We propose a new
method of reaching control design while the sliding surface and equivalent control can be designed conven-
tionally. The deviations of the sliding variable and its high order derivatives from zero are penalized. This is
realized by minimizing the amplitudes of the higher order derivatives of the sliding variable. An illustrative
example— a field-controlled DC motor— is given at the end.
1 INTRODUCTION
Variable structure systems (VSS) have been exten-
sively used for control of dynamic industrial pro-
cesses. The essence of variable structure control
(VSC) is to use a high speed switching control scheme
to drive the plant’s state trajectory onto a specified
and user chosen surface in the state space which is
commonly called the sliding surface or switching sur-
face, and then to keep the plant’s state trajectory mov-
ing along this surface (Utkin, 1992), (Utkin, 1977).
VSS has attracted attention during the past decades
because of its superior capability to eliminate the im-
pact of uncertainties.
Standard sliding mode controllers reveal draw-
backs: high frequency vibration of the controlled sys-
tem, which is also called “chattering”, and sensitiv-
ity to disturbances during reaching mode. In recent
years, a new method, so-called “higher order sliding
mode (HOSM)” has been proposed (Levant, 1996),
(Levant, 2007), (Glumineaus, 2006) for nonlinear
sliding mode design. In higher order sliding mode
problems, the switching controller also influences the
higher order derivatives of the sliding variable rather
than just the first order derivative. Under certain cir-
cumstances, for instance, the control u is treated as
an additional state variable while its derivative
.
u is
employed as the actual control (Levant, 1996), (Zi-
nober). The most popular higher order sliding mode
controllers are the so called “twisting controller” and
“super-twisting controller” which are derived based
on bang-bang control theory. A number of papers
report the derivation and performance of these con-
trollers (Levant, 1996), (Levant, 2007), (Glumineaus,
2006), (Castellanos, 2004). As discussed by Boiko,
Fridman and Castellanos (2004), if the actuator is of
second or higher order there is an opportunity for re-
duction of the amplitude of chattering in the control
signal when using twisting as a filter algorithm, com-
pared with first order SM control. In other words,
higher order sliding mode control contributes to sup-
pressing the chattering effect although not completely
eliminating it. Furthermore, a new concept, “integral
sliding mode control (ISMC)” has been developed re-
cently (Shi, 1996). With an integral sliding mode con-
trol scheme, the reaching phase is eliminated so that
robustness is guaranteed right from the initial time in-
stant.
The aim of this paper is to provide an effective
and more convenient way to solve nonlinear higher
order sliding mode problems. Nonlinear continuous
systems are worked on and second or even higher
order sliding mode control concepts are developed.
With this method, a sliding mode is reached by forc-
ing the sliding variable and its higher order deriva-
tives to zero in finite time rather than working on
nonlinear inequalities based on high order differen-
tial equations, which is inevitable in “super-twisting”
controller design. The resulting reaching controller
does not contain any high frequency switching com-
ponent which evokes high frequency responses of the
system. This idea is borrowed from optimal control
laws. The derivation of equivalent control is different
from that of normal sliding mode. Meanwhile, the
54
Xi Z. and Hesketh T.
HIGHER ORDER SLIDING MODE CONTROL FOR CONTINUOUS TIME NONLINEAR SYSTEMS BASED ON OPTIMAL CONTROL.
DOI: 10.5220/0002168100540059
In Proceedings of the 6th International Conference on Informatics in Control, Automation and Robotics (ICINCO 2009), page
ISBN: 978-989-674-001-6
Copyright
c
2009 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved

sliding surface design may employ various methods.
In Section III B, we address the problem of the
reachability of the sliding surface. To avoid chatter-
ing, whateverthe initial state of the system is, both the
sliding variable and its derivatives have to be driven
to zero (not necessarily with the same convergence
speed). They should also be kept at zero after the
sliding surface is reached. In this paper, the reach-
ing controller is expected to be a continuous nonlin-
ear function with respect to the state variables. The
form of the nonlinearity is determined by the solu-
tion of a minimization problem which is analogous to
that which occurs in optimal control. What is to be
minimized is the amplitude of the vector the entries
of which are the sliding variable and its derivatives.
If an q -th order sliding mode is pursued, the sliding
variable and its derivatives up to order (q − 1) will be
contained in the state vector. This method leads to a
very smooth system trajectory. The reachablility of
the sliding surface is guaranteed by the existence of a
solution to the minimization operation. Furthermore,
the minimization algorithm promises good robustness
while the precision of high order sliding mode is kept.
At the end of this paper, a field controlled DC mo-
tor is considered. The performance of the proposed
control scheme is shown applied to this third order
system.
2 THE PROBLEM STATEMENT
Consider a continuous nonlinear system of the form
.
x(t) = f(x(t)) + Bu(t), t ≥ t
0
(1)
x(t
0
) = x
0.
(2)
where x(t) ∈ R
n×1
, u ∈ R
1×1
is the control input, σ is
the sliding variable. B
n×1
, C, D are matrices or vectors
of proper dimensions and n is known. It is assumed
that f(x(t)) is Lipschitz continuous and differentiable
with respect to x(t) to any order. In this paper, the
sliding variable is restrained to be a linear combina-
tion of the states, which has the following form:
σ(t) = Sx(t) = s
1
x
1
(t) + s
2
x
2
(t) + ... + s
n
x
n
(t) (3)
Calculate the first and second order derivative of
the sliding variable and we have
.
σ(t) = S
.
x(t) = S f(x(t))+ SBu(t) (4)
..
σ(t) = S
..
x(t) = S
∂f(x(t))
∂x(t)
f(x(t))
+S
∂f(x(t))
∂x(t)
Bu(t)+ SB
.
u(t) (5)
3 SECOND ORDER SLIDING
MODE CONTROL DESIGN
3.1 Sliding Surface Design
For system (1), perform a similarity transformation
defined by an orthogonal matrix P
n×n
:
x
l
= Px = [x
l1
: x
l2
]
T
, B
l
= PB =
0
k×1
B
2
, (6)
f
l
(x(t)) = f(x
l
(t)) =
f
l1
(x(t))
f
l2
(x(t))
. (7)
where x
l1
∈ R
k×1
, x
l2
∈ R
(n−k)×1
, B
(n−k)×1
2
and x
l1
does not have direct dependence on the input. Sliding
surface design may be undertaken considering only
x
l1
, treating x
l2
as an “input” to the partitioned system.
In this way, the input may be ignored while determin-
ing the sliding surface and this reduces the complexity
of the sliding surface design.
The partitioned state equations corresponding to
(1) may now be expressed in the following way:
.
x
l1
(t) = f
l1
(x
l1
(t), x
l2
(t)) (8)
.
x
l2
(t) = f
l2
(x
l1
(t), x
l2
(t)) + B
2
u(t). (9)
Suppose
Sx(t) = [
s
1
s
2
· · · s
n
]x(t)
= wPx(t) = wx
l
(t)
= w
l1
x
l1
(t) + w
l2
x
l2
(t)
in which
w
p×k
l1
=
w
1
w
2
· · · w
k
,
w
p×(n−k)
l2
=
w
k+1
w
k+2
· · · w
n
,
and Sx(t) is the sliding variable, then the condition for
the sliding mode to exist is
w
l1
x
l1
(t) + w
l2
x
l2
(t) = 0,
which yields
x
l2
(t) = −w
−1
l2
w
l1
x
l1
(t). (10)
When w
l2
is non-square, w
−1
l2
in (10) should be its
pseudo inverse.
Substituting (10) into (8) we have,
x
l1
(t + 1) = f
l1
(x
l1
(t), −w
−1
2
w
1
x
l1
(t)) (11)
= F(x
l1
(t)) (12)
where F(·) denotes the nonlinear function about x
l1
(t)
after tidying (11) up.
The goal of the next step is to fix the relation-
ship between x
l2
(t) and x
l1
(t) to prescribe desirable
HIGHER ORDER SLIDING MODE CONTROL FOR CONTINUOUS TIME NONLINEAR SYSTEMS BASED ON
OPTIMAL CONTROL
55

performance for the nominal sliding mode dynam-
ics. Any standard design algorithm which produces
a linear state feedback controller for a nonlinear dy-
namic system can be used to determine F(x
l1
(t))
and achieve desired performance through selection of
sliding mode dynamics (Spurgeon, 1992). It is also
assumed here that (12) is stabilizable. The controller
gain derived is:
x
l2
(t) = −kx
l1
(t) (13)
which means that
σ(x
l
(t)) =
h
k
.
.
. I
i
x
l
(t) (14)
while I represents the identity matrix with proper di-
mension.
Note that inversion of the similarity transforma-
tion (using P) is needed to recover x(t) from x
l
(t).
Then Sx(t) = 0 is the desired sliding surface.
3.2 Higher Order Sliding Mode Design
3.2.1 Reaching Control Design
As the reaching condition implies, the sliding vari-
able has to converge to zero in finite time. Further-
more, as an q-th order sliding mode is expected,
.
σ,
..
σ......σ
(q−1)
are also desired to approach zero. Derive
a vector containing σ,
.
σ,
..
σ......σ
(q−1)
and extend (4),
(5) to describe this vector
.
σ(t) = S
.
x(t) = S f(x(t))+ SBu(t) (15)
..
σ(t) = S
..
x(t) = S
∂f(x(t))
∂x(t)
f(x(t))
+S
∂f(x(t))
∂x(t)
Bu(t)+ SB
.
u(t) (16)
σ
(3)
(t) = Sx
(3)
(t)
= S(
∂
2
f(x(t))
∂x
(2)
(t)
+ (
∂f(x(t))
∂x(t)
)
2
) f(x(t)
+S(
∂
2
f(x(t))
∂x
(2)
(t)
+ (
∂f(x(t))
∂x(t)
)
2
)Bu(t)
+S
∂f(x(t))
∂(t)
B
.
u(t) + SBu
(2)
(t), (17)
......
which is equivalent to
z(t) = G(x(t))+ H(x(t))U(t). (18)
where
z(t) =
σ(t)
.
σ(t)
...
σ
(q−1)
(t)
, U(t) =
u(t)
.
u(t)
...
u
(q−1)
(t)
,
H(x(t)) =
0 0 ... 0
SB 0 ... 0
S
∂f(x(t))
∂x(t)
B SB ... 0
S(
∂
2
f(x(t))
∂x
(2)
(t)
+ (
∂f(x(t))
∂x(t)
)
2
)B ... ... ...
... ... ... SB
,
G(x(t)) =
Sx(t)
Sf(x(t))
S
∂f(x(t))
∂x(t)
f(x(t))
S(
∂
2
f(x(t))
∂x
(2)
(t)
+ (
∂f(x(t))
∂x(t)
)
2
) f(x(t))
...
(19)
Here, the following conditions are assumed:
Assumption I:
z(t) ∈ Z, Z
contains the origin.
Assumption II: The set
Z
is reachable in finite
time from any initial state and from any point in the
generated trajectories.
As the purpose of reaching control design is to
find some u(t) which regulates z(t) to zero in finite
time, we define a cost function which is
J(t) = z
T
(t)z(t) + λU
T
(t)U(t) (20)
with a weighting factor λ. Then U(t) is determined to
minimize J(t).
Taking the partial derivative of J(t) with respect
to U(t) we have:
∂J(t)
∂U(t)
=
∂(z
T
(t)z(t) + λU
T
(t)U(t))
∂U(t)
. (21)
Let
∂J(t)
∂U(t)
= 0 and derive:
U(t) = M(x(t))
= −(H(x(t))
T
H(x(t)) + λI)
−1
H(x(t))
T
G(x(t))
(22)
(I here again represents the identity matrix if certain
dimension.)
It should be noticed that the derivation of (22) re-
duces to a Tikhonov regularization problem therefore
the detail is omitted here.
REMARK: Here, we assume the minimization
over an infinite horizon results in a control U
∗
(t).
This control input will be implemented only until the
ICINCO 2009 - 6th International Conference on Informatics in Control, Automation and Robotics
56

next measurement becomes available. Then the up
to date system information will be taken into account
and a new value of U
∗
(t) is computed. Introduce
J(t + h) = z
T
(t)z(t) + λU
∗T
(t)U
∗
(t) (23)
≤ z
T
(t)z(t) + λU
T
(t)U(t) = J(t).(24)
where J(t) stands for the cost observed at time t and
h is a sufficiently small positive number. The final
cost J(∞) is a finite non-negative number as J(t) is
non-increasing. In other words, J(t) decreases due to
the effect of U
∗
(t) until reaches zero. Then the next
value (final value) of U
∗
(t) is zero which indicates
that the reaching mode is complete. Meanwhile, the
final value of z
T
(t)z(t) is zero. By choosing λ to be
a small positive weighting factor, non-zero z
T
(t)z(t)
will be relatively heavily punished and so z(t) con-
verges to zero more quickly.
The reaching control law u
r
(t) can be obtained
from the equation which forms the first row of (22)
(Wertz, 1990)
u
r
(t) =
1 0 ... ... 0
M(x(t)) = M
1
(x(t))
(25)
where M
1
(x(t)) stands for the first element of vector
M(x(t)).
3.2.2 Robustness Issue
By substituting (22) into (18) we have
z(t) = G(x(t)) + H(x(t))M(x(t)). (26)
Now, assume that due to modelling errors, the real
system is
.
x(t) = f
real
(x(t)) + B
real
u(t), t ≥ t
0
(27)
which leads to
z(t) = G
real
(x(t)) + H
real
(x(t))U(t). (28)
The robustness of the reaching mode relies on
•
Assumption I and II
for z(t) in (28)
• The satisfaction of (29)
J(G
real
, H
real
, U
∗
(t), t) ≤ J(G, H, t). (29)
3.2.3 Equivalent Control Design
After the sliding mode is reached, the system dy-
namic is dominated by the equivalent controller. To
ensure q− th order sliding, the equivalent control has
to maintain σ(t),
.
σ(t)...σ
(q−1)
(t) at zero. By extend-
ing (1), (3) we have
σ
(q−1)
(t) = P( f(x)) + Q(u(t)). (30)
where P(·) and Q(·) are both nonlinear functions.
The equivalent control u
eq
(t) should be derived
according to the following
σ
(q−1)
(t) = P( f(x)) + Q(u
eq
(t)) = 0. (31)
As introduced in (Matthews, 1988), the complete slid-
ing mode controller is
u(t) = u
eq
(t) + u
r
(t) (32)
where u
r
(t) is from (25).
4 EXAMPLE AND SIMULATION
RESULTS
4.1 Field controlled DC Motor
and controller Design
Consider the example of a field-controlled DC motor.
DC motors are widely used by almost all industries
and can be highly nonlinear in field controlled config-
urations. The mathematical model of a DC motor can
Figure 1: Structure of a DC motor.
be expressed in the following way.
.
x
1
(t) = −ax
1
(t) + u(t) (33)
.
x
2
(t) = −bx
2
(t) + ρ− cx
1
(t)x
3
(t) (34)
.
x
3
(t) = θx
1
(t)x
2
(t) (35)
y(t) = x
3
(t). (36)
The physical meanings of the variables in the above
equations are:
x
1
(t) Field current
x
2
(t) Armature current
x
3
(t) Angular velocity
u(t) Field voltage
ρ Armature voltage,
,
HIGHER ORDER SLIDING MODE CONTROL FOR CONTINUOUS TIME NONLINEAR SYSTEMS BASED ON
OPTIMAL CONTROL
57
with a, b, c, θ, ρ positive constants.
The equilibria of the system are
x
1
= 0, x
2
=
ρ
b
and x
3
= ω
0
,
where ω
0
is a desired setpoint for the angular velocity.
In this paper, we choose
a = b = c = θ = ρ = 1
for simplicity (?).
The partitioned system matrices are
f
l1
(x
l1
, x
l1
) = −x
1
(t) (37)
.
f
l2
(x
l1
, x
l1
) =
−x
2
(t) + 1− x
1
(t)x
3
(t)
x
1
(t)x
2
(t)
(38)
B
l
=
B
1
0
2×1
=
1
0
0
(39)
It is seen that (33)-(35) are already in the same
form as (6)-(7). Hence the transformation matrix P is
identity.
Suppose
Sx(t) = [
s
1
s
2
s
3
]x(t) = w
l1
x
l1
(t) + w
l2
x
l2
(t),
The values of w
1
and w
2
must be chosen to ensure the
following system has satisfactory closed loop behav-
ior:
x
l2
(t) = Kx
l1
(t) = −w
−1
l2
w
l1
x
l1
(t)
.
x
l1
(t) = f
l1
(x
l1
(t), −w
−1
l2
w
l1
x
l1
(t)) (40)
= Fx
l1
(t). (41)
One of the proper selections of w
1
and w
2
leads to:
K =
0 −1
which produces a sliding variable:
σ(t) = Sx(t) = x
1
(t) + x
3
(t) − x
3desired
In this case, x
3
(t) − x
3desired
is treated as the state
of the system rather than x
3
(t) in certain design steps
because the final value of x
3
is not expected to be
zero but a desired value. This desired value should
be involved in the sliding surface design. Similarly,
x
2desired
should be considered at some stage as well.
(In this case, x
2desired
= 0.95 and x
3desired
= 2.05.)
Accordingly, we have
u
eq
(t) =
2x
2
1
(t)x
3
(t) + 2x
1
(t)x
3
(t) − x
3
1
(t)x
2
(t) − 4x
1
(t)
3x
2
(t) + x
1
(t)x
3
(t) + 2x
3
(t) − 3
which is derived by letting
σ
(3)
(t) = Sx
(3)
(t) = 0.
Now we proceed to design the reaching control.
In this case, take the derivatives of σ(t) up to order
3 into account in the cost function definition. Then
G(x(t)) and H(x(t)) can be calculated from (19). As
the result of the minimization, the reaching control
will be expressed in the form of a nonlinear function
of state variables:
u
r
(t) = W(x(t)).
W(x(t) is derived from (22) and (25). Computing
W(x(t)) is reduced to a numerical calculation with-
out necessity of pursuing the algebraic description of
W(x(t)). Finally the complete control law u(t) is de-
rived using (32) with the equivalent control derived
according to (31).
−0.05 −0.04 −0.03 −0.02 −0.01 0 0.01 0.02 0.03 0.04 0.05
−2.5
−2
−1.5
−1
−0.5
0
0.5
sliding variable
1−derivative
Figure 2: Sliding variable and its derivative.
4.2 Simulation Results
The integration step size is chosen to be 1ms. In all
the figures below, the unit of time axis is in second.
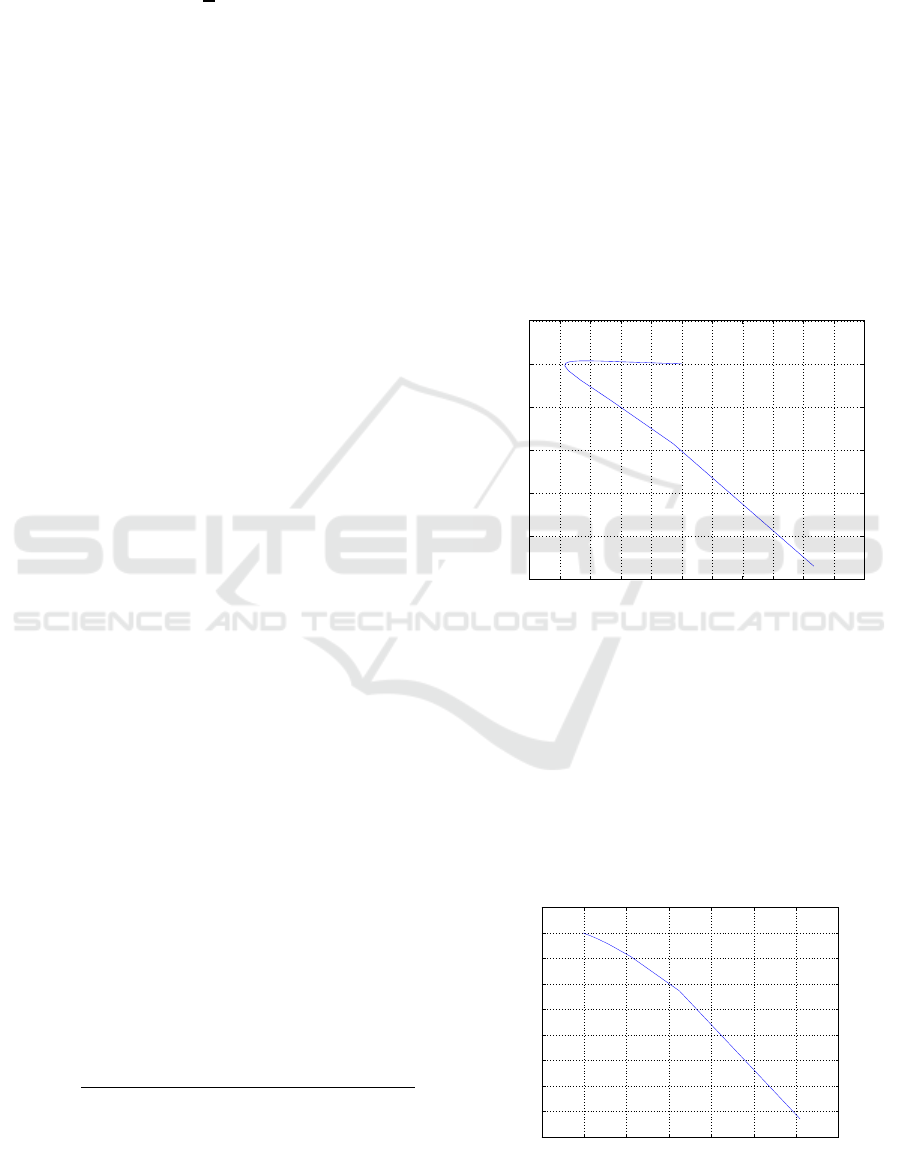
The process depicting the sliding variable and its
derivative as they approach zero is shown in Fig. 2
The trajectory travels smoothly on the plane until it
reaches the origin without overshooting. From Fig. 3
−2 0 2 4 6 8 10 12
−400
−350
−300
−250
−200
−150
−100
−50
0
50
3−derivative
2−derivative
Figure 3: Higher order derivatives of the sliding variable.
ICINCO 2009 - 6th International Conference on Informatics in Control, Automation and Robotics
58
we can see that the second and third order deriva-
tives of the sliding variable also behave as a smooth
curve which ends up at the origin. Figure 4 shows
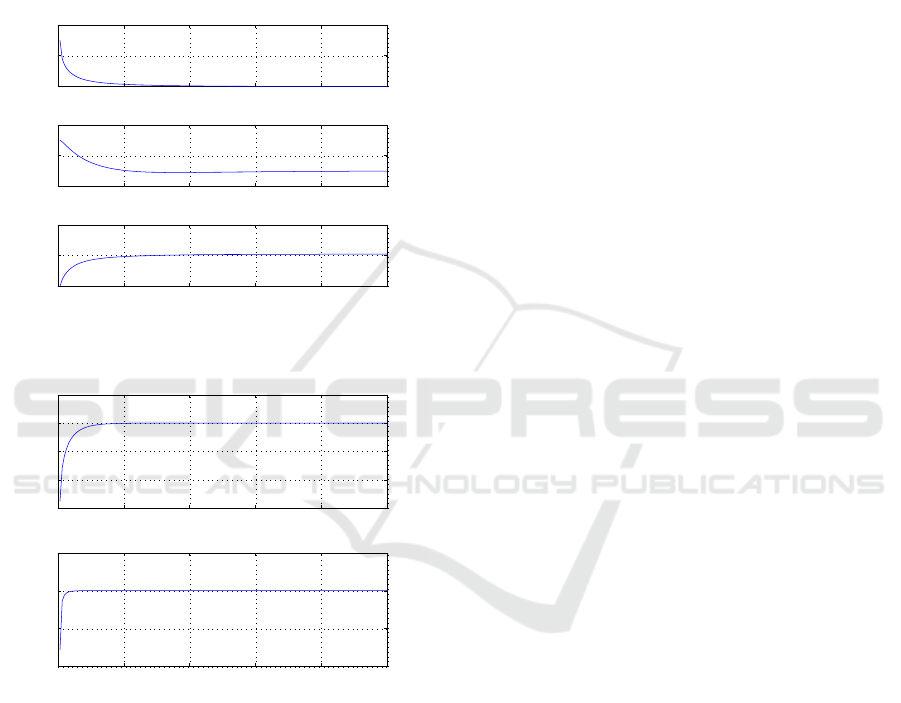
the convergence performance of the state variables. It
is shown that x
1
(t) converges to zero while x
2
(t) and
x
3
(t) each approach their desired value. The trajec-
tories are smooth and there is no overshoot or oscil-
lation. The whole process settles quickly within 0.5
seconds.
0 0.3 0.6 0.9 1.2 1.5
0
2
4
t
x1
0 0.3 0.6 0.9 1.2 1.5
0
2
4
t
x2
0 0.3 0.6 0.9 1.2 1.5
0
2
4
t
x3
Figure 4: Convergence of the states.
0 0.3 0.6 0.9 1.2 1.5
−6
−4
−2
0
2
t
reaching control
0 0.3 0.6 0.9 1.2 1.5
−20
−10
0
10
t
equivalent control
Figure 5: Control signal u.
The variation of the control signal u during the pe-
riod is plotted in Figure 5.
As shown above, a good performance is achieved.
A higher order sliding behavior is shown.
5 CONCLUSIONS
In this paper, a new method of designing a higher or-
der sliding mode controller for a continuous nonlin-
ear dynamic system is reported. Retaining the advan-
tages of higher order sliding mode control, i.e. chat-
tering reduction, the complexity of nonlinear design is
greatly reduced with this method especially in reach-
ing control design. A field-controlled DC motor is
given as an illustrative example to show the effective-
ness of this method.
REFERENCES
Vadim I. Utkin (1992). Sliding Modes in Control and Opti-
mization. Springer-Verlag New York, Inc.
Vadim I. Utkin (1977). Variable Structure Systems with
Sliding Modes. IEEE Transactions on Automatic Con-
trol, Volume AC-22, No. 2, April.
S. V. Emeryanov, S. K. Korovin, and A. Levant (1996).
Higher-order Sliding Modes in Control Systems.
Computational Mathematics and Modeling, Vol. 7,
No. 3.
A. Levant (2007). Principles of 2-sliding mode design. Au-
tomatica Vol. 43, pp. 576 – 586.
S. Laghrouche, F. Plestan, and A. Glumineau (2005). Multi-
variable practical higher order sliding mode control.
Proceedings of the 44th IEEE Conference on Decision
and Control, and the European Control Conference.
A.J. Koshkouei, K.J. Burnham and A.S.I. Zinober. Dynamic
sliding mode control design. IEE Proceedings online
no. 20055133.
I. Boiko, L. Fridman, and I. M. Castellanos (2004). Anal-
ysis of second-order sliding-mode algorithms in the
frequency domain. IEEE Transaction on Automatic
Control, Vol. 49, No. 6, pp. 946–950, Jun.
Vadim Utkin and Jingxin Shi(1996). Integral Sliding Mode
in Systems Operating under Uncertainty Conditions.
Proceedings of the 35th Conference on Decision and
Control, Kobe, Japan, December.
S. K. Spurgeon (2004). Temperature Control of Industrial
Process using a Variable Structure Design Philoso-
phy. Transactions of the Institute of Measurement and
Control
R. R. Bitmead, M. Gevers, and V. Wertz (1990). Adaptive
Optimal Control: The Thinking Man’s GPC. Engle-
wood Cliffs, NJ: Prentice-Hall.
Raymond, A. DeCarlo, Stanislaw H. Zak, Gregory P.
Matthews(1988). Variable Structure Control of Non-
linear Multivariable Systems: A Tutorial. Proceedings
of The IEEE, Vol. 76, No. 3, March.
Christopher Edwards and Sarah K. Spurgeon (1998). Slid-
ing Mode Control, Theory And Applications. CRC
Press, Taylor & Francis Group.
HIGHER ORDER SLIDING MODE CONTROL FOR CONTINUOUS TIME NONLINEAR SYSTEMS BASED ON
OPTIMAL CONTROL
59
