
ANALOG REALIZATIONS OF FRACTIONAL-ORDER
INTEGRATORS/DIFFERENTIATORS
A Comparison
Guido Maione
DEESD, Technical University of Bari, Via de Gasperi, snc, I-74100, Taranto, Italy
Keywords: Non-integer-order operators, Fractional-order controllers, Rational approximation, Interlaced singularities.
Abstract: Non-integer differential or integral operators can be used to realize fractional-order controllers, which
provide better performance than conventional PID controllers, especially if controlled plants are of non-
integer-order. In many cases, fractional-order controllers are more flexible than PID and ensure robustness
for high gain variations. This paper compares three different approaches to approximate fractional-order
differentiators or integrators. Each approximation realizes a rational transfer function characterized by a
sequence of interlaced minimum-phase zeros and stable poles. The frequency-domain comparison shows
that best approximations have nearly the same zero-pole locations, even if they are obtained starting from
different points of view.
1 INTRODUCTION
Originally, the investigation of integrals and
derivatives of any order was a topic known as
fractional calculus. In recent years, however,
considerable attention has been paid to the concept
of non-integer derivative and integral to model
systems in various fields of science and engineering.
In the research area of control theory, several
authors have provided generalizations of classical
controllers introducing various types of Fractional-
Order Controllers (FOC). For example, the CRONE
(French acronym for “Commande Robuste d’Ordre
Non Entièr”) controller (Oustaloup, 1991;
Oustaloup, 1995) and Fractional-Order Proportional-
Integral-Derivative (FOPID) controllers PI
λ
D
μ
(Podlubny, 1999a; Podlubny, 1999b) have been
recently considered. Moreover, FOC have been
successfully applied in rigid robots, both for position
control and for hybrid position-force-control
(Tenreiro Machado and Azenha, 1998; Valerio and
Sá da Costa, 2003). In general, FOC provide better
performance than PID controllers, if the controlled
plants are of non-integer-order. In other cases, FOC
show high flexibility and can ensure high robustness
for high gain variations. More particularly, in SISO
systems, they can make the phase margin nearly not
changing in a wide range around the gain crossover
frequency, even if high gain variations produce high
changes in gain crossover frequency. Applications in
mechatronics are testified by several papers (Canat
and Faucher, 2005; Li and Hori, 2007; Ma and Hori,
2004a; Ma and Hori, 2004b; Ma and Hori, 2007;
Melchior et al., 2005).
The basic element of transfer functions of
FOPID controllers is the fractional
differentiator/integrator s
ν
, with ν positive or
negative real number. This operator is infinite
dimensional, even if it can be approximated by
finite-dimension transfer functions, whose
coefficients depend on the non-integer exponent ν. A
good rational approximation can be obtained by
truncating the continued fractions expansion (CFE)
of s
ν
(Maione, 2006; Maione, 2008). Recently, in
(Barbosa et al., 2006), least-squares-based methods
are used for obtaining Fractional-Order Differential
Filters (FODF) approximating s
ν
.
In this paper, a novel approach is compared to
two commonly used methods to realize a rational
approximation of fractional-order differentiators or
integrators. These operators are the basic elements in
fractional-order controllers of mechatronic systems.
Section 2 revisits the three different methods
systematically. Section 3 compares them in the
frequency domain. Section 4 draws the conclusion
with some remarks.
184
Maione G.
ANALOG REALIZATIONS OF FRACTIONAL-ORDER INTEGRATORS/DIFFERENTIATORS - A Comparison.
DOI: 10.5220/0002173901840189
In Proceedings of the 6th International Conference on Informatics in Control, Automation and Robotics (ICINCO 2009), page
ISBN: 978-989-674-001-6
Copyright
c
2009 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved

2 REVISITING THREE
RATIONAL APPROXIMATIONS
In this section, three methods are compared. They
are shortly revisited, for making a direct comparison
based on transfer functions putted in the same form.
All the considered realizations are known to be
minimum-phase and stable, with poles interlacing
zeros along the negative real half-axis of the s-plane.
This property is enlightened by the form of the three
transfer functions, which explicitly shows the
frequencies corresponding to the alternated zeros
and poles. The interlacing property is important for
comparison purposes, because the position of the
zero-pole pairs determines the quality of the models
approximating phase and magnitude of the irrational
operator (jω)
ν
. Hence, for comparison purpose,
realizations are constrained to have both their zeros
with minimum module and their poles with
maximum module approximately equal. All the
approximating transfer functions are in a factorized
form, which puts in evidence the break frequencies.
Then, the lowest and highest break frequencies of
the proposed method are taken as reference.
2.1 The Proposed CFE Approximation
The starting point is the following continued
fractions expansion (CFE):
()
1
2
2
1
1
0
+++
+≅+
j
j
b
a
b
a
b
a
bx
ν
(1)
with b
0
= b
1
= 1, a
1
= ν x and:
a
j
= n (n–ν) x, b
j
= 2n (2)
a
j
+1
= n (n+ν) x, b
j
+1
= 2n+1 (3)
for j = 2n, with n natural number (Khovanskii,
1965). The analog approximation for the operator s
ν
,
with 0 < ν < 1, is given in (Maione, 2008), where
x = s–1 is used in (1) to obtain the (2N)-th
convergent of the resulting CFE as approximating
transfer functions:
)( )( )(
)( )( )(
),(
~
1
10
1
10
ννν
ννν
ν
NN
N
N
N
N
NN
N
N
N
N
qsqsq
pspsp
sG
+++
+++
=
=
−
−
(4)
where
p
Nj
(ν) = q
N,N-j
(ν) =
= (–1)
j
C(N, j) (ν+j+1)
(
N
-
j
)
(ν– N)
(
j
)
(5)
and
)!(!
!
),(
jNj
N
jNC
−
=
is the binomial
coefficient. Moreover:
(ν+j+1)
(
N
-
j
)
= (ν+j+1) (ν+j+2) … (ν+N) (6)
(ν–N)
(
j
)
= (ν–N) (ν–N+1) … (ν–N+j+1) (7)
define the Pochammer functions with (ν–N)
(0)
= 1
(Spanier and Oldham, 1987). As it is easily noted, in
this method the coefficients p
Nj
(ν) and q
Nj
(ν) are
explicitly given in terms of the fractional order ν.
Obviously, the positions of zeros and poles in the s-
plane also depend on ν. So,
),(
~
sG
ν
can be written
in the form:
∏
=
+
+
≅
N
i
p
z
i
i
s
s
ksG
1
~
1
~
1
~
),(
~
ω
ω
ν
.
(8)
As it is proved in (Maione, 2008), zeros
)
~
(
i
z
ω
−
and poles
)
~
(
i
p
ω
−
of ),(
~
sG
ν
are all real and
interlace along the negative real half-axis in the
s-plane, with:
NN
pzpzpz
ω
ω
ω
ω
ω
ω
~
~
~
~
~
~
2211
<<
<
<
<
<
.
(9)
2.2 Oustaloup’s Recursive
Approximation
The CRONE controller is an integer-order frequency
domain approximation of s
ν
in the form:
∏
=
+
+
≅
N
i
p
z
i
i
s
s
ksG
1
1
1
),(
ω
ω
ν
.
(10)
The gain k is adjusted so that G(ν,s) has the same
crossover frequency as the ideal operator s
ν
. The
number N of zeros and poles of the approximating
transfer function is chosen in advance. They
alternate on the negative real half-axis of the s-plane
so that the frequencies satisfy:
NN
pzpzpz
ω
ω
ω
ω
ω
ω
<<
<
<
<
<
2211
.
(11)
ANALOG REALIZATIONS OF FRACTIONAL-ORDER INTEGRATORS/DIFFERENTIATORS - A Comparison
185

In this way, zeros and poles interlace on the
negative real half-axis, leading to a gain which is,
approximately, a linear function of the logarithm of
frequency. The phase is nearly constant and
approximates ν π / 2. The parameters ω
z
i
and ω
p
i
are
determined by placing zeros and poles as follows:
ηωω
ω
ω
η
ω
ω
α
νν
Lz
N
L
H
N
L
H
=
⎟
⎠
⎞
⎜
⎝
⎛
=
⎟
⎠
⎞
⎜
⎝
⎛
=
−
1
; ;
1
(12)
α
ω
ω
ii
zp
= i = 1, ..., N
(13)
η
ω
ω
1 ii
pz
=
+
i = 1, ..., N–1.
(14)
The frequencies
ω
L
and ω
H
are appropriately
chosen as
1
~
zL
ω
ω
< and
N
pH
ω
ω
~
> , so that it holds
11
~
zz
ω
ω
≅ and
NN
pp
ω
ω
~
≅ .
2.3 Matsuda’s Approximation
The Matsuda’s method approximates the operator s
ν
from its gain
ω
ν
. The gain is determined at 2N+1
frequencies
ω
0
, ω
1
, …, ω
2N
, which are taken
logarithmically spaced in the approximation interval.
The interval [
ω
0
, ω
2N
] is chosen so that the lowest
break frequency
1
ˆ
z
ω
and the highest break
frequency
N
p
ω
ˆ
in the model satisfy:
11
~
ˆ
zz
ω
ω
≅ and
NN
pp
ω
ω
~
ˆ
≅
, respectively. Note that, usually, an odd
value of
N is used, so that the resulting
approximation is proper. Then, the following
functions are defined:
)()(
)(
;
)()(
)( ;
;
)()(
)( ;)(
121212
12
2
111
1
000
0
10
−−−
−
−−−
−
−
−
=
−
−
=
−
−
==
NNN
N
N
kkk
k
k
mm
m
mm
m
mm
mm
ωω
ωω
ω
ωω
ωω
ω
ωω
ωω
ωωω
ν
……
…
(15)
from which the following set of parameters are
obtained:
()
ν
ωα
00
=
(16)
)()(
111
1
−−−
−
−
−
=
kkkk
kk
k
mm
ωω
ωω
α
(17)
for
k = 1, 2, …, 2N.
Using the
ω
k
and α
k
, the CFE can be written as:
3
2
2
1
1
0
0
+
−
+
−
+
−
+≅
α
ω
α
ω
α
ω
α
ν
sss
s
(18)
whose convergents provide the rational
approximations to the irrational operator s
ν
. The
(2
N)-th convergent of (18) can be easily converted
into the rational approximation, as the ratio
),(
ˆ
sG
ν
of two polynomials with degree
N. Then, the
factorization of these polynomials leads to:
∏
=
+
+
≅
N
i
p
z
i
i
s
s
ksG
1
ˆ
1
ˆ
1
ˆ
),(
ˆ
ω
ω
ν
.
(19)
Numerical experiments show that, also in this
case, it holds:
NN
pzpzpz
ω
ω
ω
ω
ω
ω
ˆˆˆˆˆˆ
2211
<<
<
<
<
<
.
(20)
3 A COMPARISON BETWEEN
THREE METHODS
The approaches of the previous sections are here
compared, by choosing
N = 3 and then N = 4. These
values are chosen to make the order of the FOC
realizations as low as possible, compatibly with
good performances. Figures 1, 2, 3 and 4 show the
Bode plots of phase and amplitude, for the typical
fractional order
ν = 0.5. Other values of the integer
N and of ν, with 0 < ν < 1, can be considered. As
previously stated, the approximation is performed so
that
),(
~
sG
ν
, G(ν,s) and ),(
ˆ
sG
ν
have their first
zero-frequency and their last pole-frequency nearly
equal. Hence, the zero-frequency
1
~
z
ω
and the pole-
frequency
3
~
p
ω
or
4
~
p
ω
of ),(
~
sG
ν
are assumed as
reference. In conclusion, it must nearly hold:
11
~
zz
ω
ω
≅
,
11
~
ˆ
zz
ω
ω
≅
,
33
~
pp
ω
ω
≅ , and
33
~
ˆ
pp
ω
ω
≅
,
when
N = 3, and
11
~
zz
ω
ω
≅
,
11
~
ˆ
zz
ω
ω
≅ ,
44
~
pp
ω
ω
≅
,
and
44
~
ˆ
pp
ω
ω
≅
, when N = 4.
First, the parameters of
),(
~
sG
ν
are determined.
For
ν = 0.5 and N = 3, formula (8) gives:
0.0521 =
~
1
z
ω
, 0.6360 =
~
2
z
ω
, 4.3119
~
3
=
z
ω
,
ICINCO 2009 - 6th International Conference on Informatics in Control, Automation and Robotics
186
0.2319 =
~
1
p
ω
, 1.5724 =
~
2
p
ω
, 19.1957
~
3
=
p
ω
, and
0.1429
~
=k . These values clearly indicate that
),(
~
sG
ν
is minimum-phase, stable, with interlacing
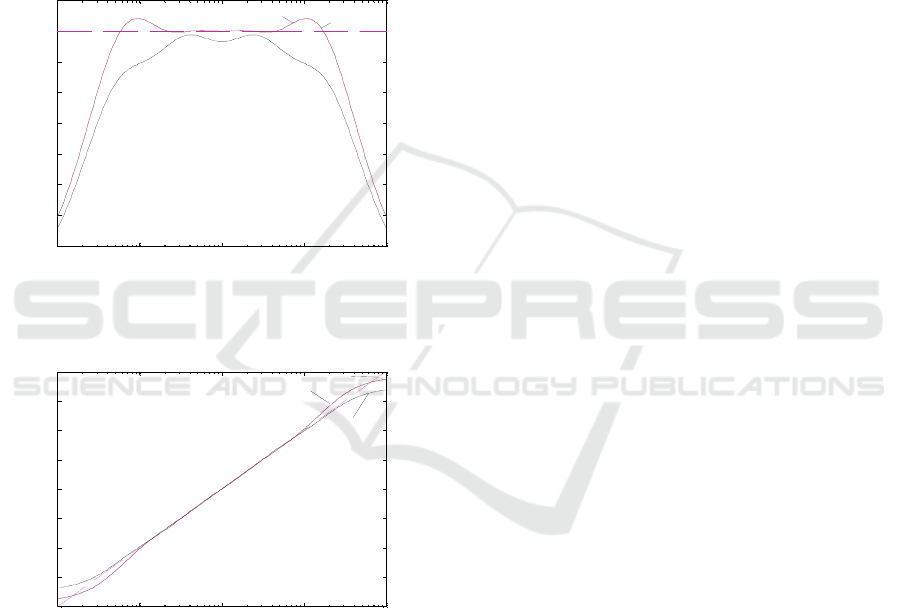
zeros and poles. Figure 1 reports the phase Bode
diagram of
)],(
~
[
ων
jGarg (Maione’s curve).
10
-2
10
-1
10
0
10
1
10
2
5
10
15
20
25
30
35
40
45
50
Frequency (rad/s)
Phase (degrees)
Ideal
Oustaloup
Matsuda
Maione
Figure 1: Phase Bode diagram for the approximations of
order 3 a fractional-order differentiator, ν = 0.5.
Now, the procedure for determining the function
G(ν,s) is considered. With reference to (12), the
interval [
ω
L
, ω
H
] is chosen larger than ]
~
,
~
[
31
pz
ω
ω
.
More precisely,
1
~
1
λ
ω
ω
zL
= and
2
~
3
λ
ω
ω
pH
=
,
where
λ
1
and λ
2
are coefficients to be fixed so that
the Oustaloup’s algorithm leads to
11
~
zz
ω
ω
≅ and
33
~
pp
ω
ω
≅ . These coefficients are chosen by a rule
of thumb. Since
0521.0
~
1
=
z
ω
and
1957.19
~
3
=
p
ω
,
simple computer experiments in MATLAB
®
show
that choosing
λ
1
= 0.55 and λ
2
= 1.8 yields:
0518.0
1
=
z
ω
, 5509.0
2
=
z
ω
, 8634.5
3
=
z
ω
,
1688.0
1
=
p
ω
, 7972.1
2
=
p
ω
, 1293.19
3
=
p
ω
, k =
0.1692. As it is noted, the constraints
11
~
zz
ω
ω
≅
and
33
~
pp
ω
ω
≅ are respected. In Figure 1, arg[G(ν, jω)]
is also reported (Oustaloup’s curve).
Finally, for applying the Matsuda’s method, the
sampling frequencies are logarithmically distributed
inside the approximation interval, so that it must
result:
11
~
ˆ
zz
ω
ω
≅ and
33
~
ˆ
pp
ω
ω
≅
, as requested. This
result is achieved by choosing
1
~
2 zN
ω
λ
ω
= and
λ
ω
ω
/
~
3
0 p
= . The parameter λ is fixed by computer
experiments to
λ = 45. Namely, the following
breaking frequencies of
),(
ˆ
ων
jG result:
0.0485 =
ˆ
1
z
ω
, 0.6248 =
ˆ
2
z
ω
, 4.5311 =
ˆ
3
z
ω
,
0.2207 =
ˆ
1
p
ω
, 1.6004 =
ˆ
2
p
ω
, 0.6273 2=
ˆ
3
p
ω
, and
0.1373=
ˆ
k . These values show that the constraints
11
~
ˆ
zz
ω
ω
≅
and
33
~
ˆ
pp
ω
ω
≅
are also satisfied. As it
can be easily observed, however, all the remaining
frequencies and the gain of the Matsuda’s model are
nearly equal to those of the author’s approximating
transfer function. This fact is confirmed by the
behaviour of
)],(
ˆ
[
ων
jGarg in Figure 1 (Matsuda’s
curve). The Bode plot, indeed, is nearly
indistinguishable from the plot of
)],(
~
[
ων
jGarg .
In conclusion, Figure 1 shows that
)],(
ˆ
[
ων
jGarg and )],(
~
[
ων
jGarg are nearly flat
and give a good approximation of
2 / ])[(
πνω
ν
=jarg
. The plot of )],(
ˆ
[
ων
jGarg
yields a slightly worst approximation. Figure 2
confirms that the magnitude plots of
),(
ˆ
sG
ν
and
),(
~
sG
ν
are nearly coincident. They give a better
approximation of
ω
ν
than G(ν,s), also in this case.
10
-2
10
-1
10
0
10
1
10
2
-20
-15
-10
-5
0
5
10
15
20
Frequency (rad/s)
Amplitude (dB)
Maione
Oustaloup
Mats uda
Ideal
Figure 2: Amplitude Bode diagram for the approximations
of order 3 of a fractional-order differentiator, ν = 0.5.
Now, let us consider a different approximation
obtained by using
N = 4 and the same procedure.
For
ν = 0.5, formula (8) gives: 0.0311 =
~
1
z
ω
,
0.3333 =
~
2
z
ω
, 1.4203
~
3
=
z
ω
, 7.5486
~
4
=
z
ω
,
0.1325 =
~
1
p
ω
,
0.7041 =
~
2
p
ω
,
3.0000
~
3
=
p
ω
,
32.1634
~
3
=
p
ω
, and 0.1111
~
=k . Then, ),(
~
sG
ν
is
minimum-phase, stable, with interlacing zeros and
poles. Figure 3 shows the phase Bode diagram of
)],(
~
[
ων
jGarg (Maione’s curve).
For the Oustaloup’s approximation,
λ
1
= 0.61 and
λ
2
= 1.64 yield: 0311.0
1
=
z
ω
, 2261.0
2
=
z
ω
,
6419.1
3
=
z
ω
, 9237.11
4
=
z
ω
,
0839.0
1
=
p
ω
,
6093.0
2
=
p
ω
, 4247.4
3
=
p
ω
, 1323.32
3
=
p
ω
, and
k = 0.1377. The constraints
11
~
zz
ω
ω
≅ and
ANALOG REALIZATIONS OF FRACTIONAL-ORDER INTEGRATORS/DIFFERENTIATORS - A Comparison
187
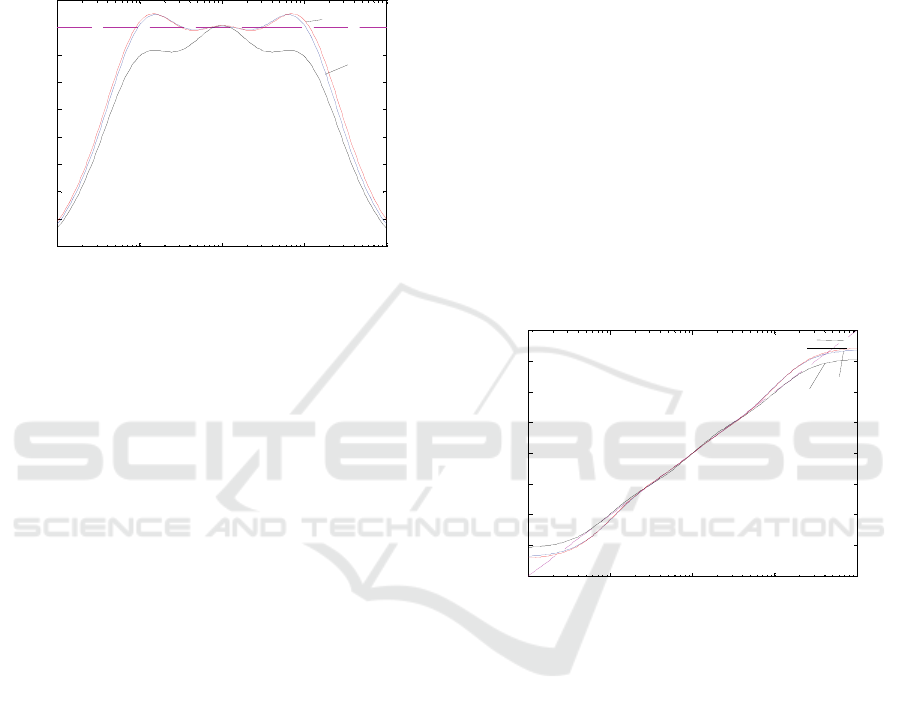
44
~
pp
ω
ω
≅ are respected. In Figure 3, arg[G(ν, jω)]
is also reported (Oustaloup’s curve).
For the Matsuda’s approximation,
λ = 39 gives:
0.0310 =
ˆ
1
z
ω
, 0.3327 =
ˆ
2
z
ω
, 1.4211 =
ˆ
3
z
ω
,
7.5702 =
ˆ
4
z
ω
, 0.1321 =
ˆ
1
p
ω
, 0.7035 =
ˆ
2
p
ω
,
3.0055=
ˆ
3
p
ω
, 32.2772=
ˆ
4
p
ω
, and 0.1109=
ˆ
k .
For
N = 4, the frequency response of )],(
ˆ
[
ων
jGarg
is practically indistinguishable from that of
)],(
~
[
ων
jGarg
(Matsuda’s and Maione’s curves are
practically the same).
10
-2
10
-1
10
0
10
1
10
2
10
15
20
25
30
35
40
45
50
Frequency (rad/s)
Phase (degrees)
Ideal
Maione
Matsuda
Oustaloup
Figure 3: Phase Bode diagram for the approximations of
order 4 of a fractional-order differentiator, ν = 0.5.
10
-2
10
-1
10
0
10
1
10
2
-20
-15
-10
-5
0
5
10
15
20
Frequency (rad/s)
Amplitude (dB)
Ideal
Maione
Matsuda
Oustaloup
Figure 4: Amplitude Bode diagram for the approximations
of order 4 of a fractional-order differentiator, ν = 0.5.
Figure 4 confirms that the magnitude plots of
),(
ˆ
sG
ν
and ),(
~
sG
ν
are nearly the same and give a
better approximation of
ω
ν
than G(ν,s), for N = 4.
4 CONCLUDING REMARKS
This paper compared three different methods to
approximate non-integer-order differential or
integral operators in fractional-order controllers:
these methods are the author’s, the Oustaloup’s, and
the Matsuda’s, respectively. All approximations of
the irrational operator
s
ν
were realized through
analog transfer functions characterized by stable
poles and minimum-phase zeros. In particular, zeros
and poles were interlaced along the negative real
half-axis of the
s-plane, and the first and last
singularities were constrained to be nearly the same
in all approximations. The interlacing property
allowed us the comparison to find the best
distribution of singularities. Namely, a frequency
domain analysis of the phase diagrams showed that
the author’s and Matsuda’s approximations
outperformed the well-known by Oustaloup.
Note that all realizations were limited to the
lowest order that could guarantee good performance.
The better results achieved by the proposed
approximation are due to a better distribution of
interlaced zeros and poles. It is also interesting to
note how the proposed approximation achieves
nearly the same zero-pole pairs of the Matsuda’s
approximation, even if the starting points of the two
methods are completely different.
REFERENCES
Barbosa, R.S., Tenreiro Machado, J.A., Silva, M.F., 2006.
Time domain design of fractional differintegrators
using least-squares. Signal Processing, Vol. 86, No.
10, pp. 2567-2581.
Canat, S., Faucher, J., 2005. Modeling, identification and
simulation of induction machine with fractional
derivative. In Fractional Differentiation and its
Applications, Le Mehauté, A., Tenreiro Machado,
J.A., Trigeassou, J.C., Sabatier, J. (Eds.), Ubooks
Verlag Ed., Neusäß, Vol. 2, pp. 195-206.
Khovanskii, A.N., 1965. Continued fractions. In
Lyusternik, L.A., Yanpol’skii, A.R. (Eds.):
Mathematical Analysis - Functions, Limits, Series,
Continued Fractions, chap. V, Pergamon Press.
Oxford, International Series Monographs in Pure and
Applied Mathematics (transl. by D. E. Brown).
Li, W., Hori, Y., 2007. Vibration suppression using single
neuron-based PI fuzzy controller and fractional-order
disturbance observer. IEEE Transactions on Industrial
Electronics, Vol. 54, No. 1, pp. 117-126.
Ma, C., Hori, Y., 2004a. Backlash vibration suppression
control of torsional system by novel fractional-order
PID
k
controller. Transactions of IEE Japan on
Industry Application, Vol. 124, No. 3, pp. 312-317.
Ma, C., Hori, Y., 2004b. Fractional order control and its
application of PI
α
D controller for robust two-inertia
speed control. In Proceedings of the 4th International
Power Electronics and Motion Control Conference
ICINCO 2009 - 6th International Conference on Informatics in Control, Automation and Robotics
188

(IPEMC 04), Xi'an, China, 14-16 Aug. 2004, Vol. 3,
pp. 1477-1482.
Ma, C., Hori, Y., 2007. Fractional-order control: theory
and applications in motion control (past and present).
IEEE Industrial Electronics Magazine, Winter 2007,
Vol. 1, No. 4, pp. 6-16.
Maione, G., 2006. Concerning continued fractions
representation of noninteger order digital
differentiators. IEEE Signal Processing Letters, Vol.
13, No. 12, pp. 725-728.
Maione, G., 2008. Continued fractions approximation of
the impulse response of fractional order dynamic
systems. IET Control Theory & Applications, Vol. 2,
No. 7, pp. 564-572.
Melchior, P., Sabatier, J., Duboy, D., Ferragne, H.,
Amagat, C., 2005. CRONE position controller for
pneumatic butterfly valve controller by on-off valves.
In Fractional Differentiation and its Applications, Le
Mehauté, A., Tenreiro Machado, J.A., Trigeassou,
J.C., Sabatier, J. (Eds.), Ubooks Verlag Ed., Neusäß,
Vol. 3, Chap. 16, pp. 721-734.
Oustaloup, A., 1991. La Commande CRONE. Commande
Robuste d’Ordre Non Entièr, Editions Hermès. Paris,
France.
Oustaloup, A., 1995. La Dérivation non Entière: Théorie,
Synthèse et Applycations, Editions Hermès, Serie
Automatique. Paris, France.
Podlubny, I., 1999a. Fractional Differential Equations,
Academic Press. San Diego, CA, USA.
Podlubny, I., 1999b. Fractional-order systems and PI
λ
D
μ
controllers. IEEE Transactions on Automatic Control,
Vol. 44, No. 1, pp. 208-214.
Spanier, J., Oldham, K.B., 1987. An atlas of functions,
Hemisphere Publishing Co.. New York, 1987.
Tenreiro Machado, J.A., Azenha, A., 1998. Fractional-
order hybrid control of robot manipulators. In IEEE
SMC’98, Proceedings of the 1998 IEEE International
Conference on Systems, Man, and Cybernetics, Hyatt
La Jolla, San Diego (CA), USA, 11-14 Oct. 1998, pp.
788-793.
Valerio D., Sá da Costa, J., 2003. Digital implementation
of non-integer control and its application to a two link
control arm. In Proceedings of the European Control
Conference, Cambridge, UK, 1-4 Sept. 2003.
ANALOG REALIZATIONS OF FRACTIONAL-ORDER INTEGRATORS/DIFFERENTIATORS - A Comparison
189
