A NOVEL DUAL ADAPTIVE NEURO-CONTROLLER BASED ON

THE UNSCENTED TRANSFORM FOR MOBILE ROBOTS

Marvin K. Bugeja and Simon G. Fabri

Department of Systems and Control Engineering, University of Malta, Msida, MSD2080, Malta

Keywords:

Dual adaptive control, Stochastic control, Neural networks, Unscented Kalman ﬁlter, Mobile robots.

Abstract:

This paper proposes a novel dual adaptive neuro-control scheme based on the unscented transform for the

dynamic control of nonholonomic wheeled mobile robots. The controller is developed in discrete time and

the robot nonlinear dynamic functions are unknown to the controller. A multilayer perceptron neural network

is used to approximate the nonlinear robot dynamics. The network is trained online via a speciﬁcally devised

unscented Kalman predictor. In contrast to the majority of adaptive control techniques hitherto proposed in the

literature, the controller presented in this paper does not rely on the heuristic certainty equivalence assumption,

but accounts for the estimates’ uncertainty via the principle of dual adaptive control. Moreover, the novel dual

adaptive control law employs the unscented transform to improve on the ﬁrst-order Taylor approximations

inherent in previously published dual adaptive schemes. Realistic simulations, including comparative Monte

Carlo tests, are used to illustrate the effectiveness of the proposed approach.

1 INTRODUCTION

Many publications on the control of nonholonomic

wheeled mobile robots (WMRs) (Kanayama et al.,

1990; Canudas de Wit et al., 1993) completely ig-

nore the robot dynamics and rely on the assumption

that the control inputs, usually motor voltages, in-

stantaneously establish the desired wheel velocities.

Others, explicitly account for the robot dynamics due

to its mass, friction and inertia (Fierro and Lewis,

1995; Corradini and Orlando, 2001) show that dy-

namic control leads to an improvement in perfor-

mance. However, as argued by Fierro and Lewis

(1995), perfect knowledge of the robot dynamics is

unavailable in practice. In addition, these parame-

ters can also vary over time due to loading, wear and

ground conditions. These issues inspired the develop-

ment of several robust and adaptive WMR controllers

over the last decade. These include: pre-trained ar-

tiﬁcial neural network (ANN) based controllers and

robust sliding-mode methods (Corradini and Orlando,

2001), parametric adaptive schemes (Wang and Tsai,

2004), and functional adaptive controllers (Bugeja

and Fabri, 2008).

Yet, all these adaptive controllers rely on the

heuristic certainty equivalence (HCE) assumption.

This means that the estimated functions are used by

the controller as if they were the true ones, thereby

ignoringcompletely the inherent uncertaintyin the es-

timations. When the uncertainty is large, for instance

during startup or when the functions are changing,

HCE often leads to large tracking errors and exces-

sive control actions, which can excite unmodelled dy-

namics or even lead to instability (

Astr¨om and Witten-

mark, 1995). In contrast, the so-called dual adaptive

controllers based on the dual control principle intro-

duced by Fel’dbaum (1965), do not rely on the HCE

assumption but account for the estimates’ uncertainty

in the control design. Speciﬁcally, a dual adaptive

control law is designed with two aims in mind: (i) to

ensure that the output tracks the reference signal with

due consideration given to the estimates’ uncertainty;

(ii) to excite the plant input sufﬁciently so as to accel-

erate the estimation process, thereby reducing quickly

the uncertainty in future estimates. These two fea-

tures are known as caution and probing respectively

(

Astr¨om and Wittenmark, 1995; Fabri and Kadirka-

manathan, 2001).

Of the few dual adaptive controllers proposed in

recent years, only our work presented in (Bugeja

and Fabri, 2009) focuses on the dynamic control of

WMRs. However, the multilayer perceptron (MLP)

dual adaptive scheme employed in this work, not only

uses the extended Kalman ﬁlter (EKF) (which inher-

ently involves a ﬁrst order approximation) as a neuro-

estimator,butthe control law itself is based on another

355

K. Bugeja M. and G. Fabri S. (2009).

A NOVEL DUAL ADAPTIVE NEURO-CONTROLLER BASED ON THE UNSCENTED TRANSFORM FOR MOBILE ROBOTS.

In Proceedings of the International Joint Conference on Computational Intelligence, pages 355-362

DOI: 10.5220/0002313503550362

 SciTePress

ﬁrst-order Taylor approximation of the measurement

model. In contrast, the novelty of the control scheme

presented in this paper comprises: the use of a specif-

ically devised form of the unscented Kalman ﬁlter

(UKF) (Julier and Uhlmann, 1997; Wan and van der

Merwe, 2001) as a recursive weight tuning algorithm

instead of the EKF; and more importantly, the devel-

opment of a novel dual adaptive control law based on

the unscented transform (UT) (Julier and Uhlmann,

1997), instead of the ﬁrst-order Taylor approxima-

tion. Together, these novel developments lead to a sig-

niﬁcant improvement in performance over the EKF-

based scheme in (Bugeja and Fabri, 2009). To the best

of our knowledge, this is the ﬁrst time that the UT is

being used in the context of dual adaptive control.

The rest of the paper is organized as follows. Sec-

tion 2 presents the dynamic model of the WMR. In

Section 3 we present the novel UT-based dual adap-

tive dynamic control scheme. Simulation results, in-

cluding those from a Monte Carlo comparative test,

are presented in Section 4, which is followed by a

brief conclusion in Section 5.

2 PLANT MODEL

Figure 1 depicts the differentially driven wheeled

mobile robot considered in this paper. The following

notation is adopted throughout the article:

: midpoint on the driving axle

: centre of mass without wheels

d: distance from P

to P

b: distance from each wheel to P

r: radius of each wheel

: mass of the platform without wheels

: mass of each wheel

: angular mass of the platform about P

: angular mass of wheel about its axle

: angular mass of wheel about its diameter

The robot state vector is given by q = [x y φ θ

]

where (x, y) is the Cartesian coordinate of P

, φ is

the robot’s orientation with reference to the xy frame,

and θ

, θ

are the angular displacements of the right

and left driving wheels respectively. The pose of the

robot refers to the vector p = [x y φ].

2.1 Kinematics

Assuming that the wheels roll without slipping, the

kinematic model of this WMR, detailed by Bugeja

and Fabri (2009), is given by:

˙q = S(q)ν, (1)

Driving wheels

Centre of mass

Geometric centre

Passive wheels

Figure 1: Differentially driven wheeled mobile robot.

where the velocity ν , [ν

]





and

S =







cosφ

sinφ

−

1 0

0 1







2.2 Dynamics

The WMR dynamic model, also detailed in (Bugeja

and Fabri, 2009), is given by:

M ˙ν +

V ( ˙q)ν +

F ( ˙q) = τ , (2)

where:

M =

(mb

+ I) + I

(mb

− I)

(mb

− I)

(mb

+ I) + I

V ( ˙q) =

F ( ˙q) = S

(q)F ( ˙q) ,

I = (I

+ m

) + 2(I

+ m

), m = m

+ 2m

F ( ˙q) is a vector of frictional forces, and τ = [τ

]

with τ

and τ

being the torques applied to the right

and left wheel respectively.

To account for the fact that the controller is im-

plemented on a digital computer, the continuous-time

dynamics (2) are discretized through a ﬁrst order for-

ward Euler approximation with a sampling interval of

T seconds, resulting in

− ν

k−1

= f

k−1

+ G

k−1

, (3)

where subscript k denotes that the corresponding vari-

able is evaluated at kT seconds, and vector f

k−1

and

matrix G

k−1

, which together encapsulate the WMR

dynamics, are given by

k−1

= −T

−1

k−1



k−1



k−1

= T

−1

k−1

(4)

The following condition is assumed.

IJCCI 2009 - International Joint Conference on Computational Intelligence

356

Assumption 2.1. The control input vector τ remains

constant over a sampling interval of T seconds, which

is chosen low enough for the Euler approximation er-

ror to be negligible.

3 CONTROL DESIGN

The trajectory tracking task of a nonholonomic WMR

is chosen as a test problem in this paper. In trajectory

tracking the robot is required to track a nonstationary

kinematically identical virtual vehicle, in both pose

and velocity at all times, by minimizing the tracking

error vector e

(Kanayama et al., 1990) deﬁned as





cosφ

sinφ

−sinφ

cosφ

0 0 1





− p

), (5)

where p

= [x

]

denotes the virtual vehi-

cle pose vector. Hence, the kinematic control task is

to make e converge to zero so that p converges to p

3.1 Kinematic Control

To address the trajectory tracking problem we em-

ploy a discrete-time version of the well-established

trajectory tracking controller originally proposed in

(Kanayama et al., 1990), given by



−



cose

+ k

sine



where ν

is the wheel velocity command vector

computed by the kinematic controller, k

, k

, and k

are positive design parameters, v

and ω

are the

translational and angular reference velocities respec-

tively corresponding to the desired trajectory, and e

, e

are the elements of e

in (5).

If one assumes perfect velocity tracking (i.e. ν

∀ k), hence ignoring the WMR dynamics ex-

pressed in (2), then this kinematic control law alone

solves the trajectory tracking problem. However, as

pointed out earlier, mere kinematic control rarely suf-

ﬁces and often leads to substantial degradation in per-

formance (Fierro and Lewis, 1995).

3.2 UT-based Dual Adaptive Control

If the nonlinear dynamic functions f

and G

are as-

sumed to be perfectly known, a simple feedback lin-

earizing control law, like the one detailed in (Bugeja

and Fabri, 2009), solves the dynamic control problem

(i.e. assuring that ν

tracks ν

∀k). However, it is an

undeniable fact that in practice the robot dynamics;

dependent on mass, inertia, friction and possibly sev-

eral unmodelled phenomena; are typically unknown

and may even change over time. In addition perfect

sensor measurements are never available.

To address these complex issues of uncertainty,

we propose a novel dual adaptive controller employ-

ing a MLP ANN trained online via an UKF algo-

rithm in prediction mode. In contrast to the hitherto

proposed innovation-based suboptimal dual adaptive

laws (Fabri and Kadirkamanathan, 2001; Bugeja and

Fabri, 2009), the control law we propose here em-

ploys the UT to approximate better the mean and co-

variance terms arising in the chosen cost function.

Hence, the envisaged improvement is not solely due

to the superior stochastic estimator employed to train

the ANN (the UKF instead of the EKF), but also due

to the dual adaptive law itself, as clariﬁed further in

the following sections.

3.2.1 Neuro-Stochastic Estimator

To deal with the uncertainty and/or time-varying na-

ture of the dynamic functions f

and G

, we opt to

assume that they are completely unknown to the con-

troller and employ a stochastically trained ANN algo-

rithm for their approximation in real-time.

A sigmoidal MLP ANN with one hidden layer is

used to approximate the nonlinear vector f

k−1

, as de-

picted in Figure 2. Its output is given by

k−1



k−1

, ˆa

) ˆw

k−1

, ˆa

) ˆw



, (6)

in the light of the following statements:

Deﬁnition 3.1. x

k−1

= [ν

k−1

1] denotes the ANN in-

put. The augmented constant serves as a bias input.

Deﬁnition 3.2. φ(·,·) is the vector of sigmoidal

activation functions, whose i

element is given by

= 1/



1+ exp



−ˆs



, where ˆs

is i

vector el-

ement in the group vector ˆa; i.e. ˆa =



ˆs

·· · ˆs



where L denotes the number of neurons. The time in-

dex has been dropped for clarity, and throughout the

paper theˆ notation indicates that the operand is un-

dergoing estimation.

Deﬁnition 3.3. ˆw

represents the synaptic weight

estimate vector of the connection between the neuron

hidden layer and the i

output element of the ANN.

Assumption 3.1. The input vector x

k−1

is contained

within a known, arbitrarily large compact set χ ⊂ R

It is known that G

k−1

is a state-independent ma-

trix with unknown elements (refer to (4)). Hence, its

estimation does not require the use of an ANN. More-

over it is a symmetric matrix, a property which is ex-

A NOVEL DUAL ADAPTIVE NEURO-CONTROLLER BASED ON THE UNSCENTED TRANSFORM FOR MOBILE

ROBOTS

357







k−1

, ˆa

) ˆw

k−1

, ˆa

) ˆw







k−1







ˆw







)

Figure 2: Sigmoidal Multilayer Perceptron neural network.

ploited to construct its estimate as follows

k−1



ˆg

k−1

ˆg

k−1

ˆg

k−1

ˆg

k−1



, (7)

where ˆg

k−1

and ˆg

k−1

represent the estimates of the

unknown elements in G

k−1

The ANN online weight-tuning algorithm is de-

veloped next. The following formulation is required

in order to proceed.

Deﬁnition 3.4. The unknown parameters requir-

ing estimation are grouped in a single vector

ˆz



ˆr

ˆg



, where ˆr



ˆw

ˆa



and

ˆg

= [ ˆg

k−1

ˆg

k−1

]

Deﬁnition 3.5. The measured output in the dynamic

model (3) is denoted by y

= ν

− ν

k−1

Assumption 3.2. By the Universal Approximation

Theorem of ANN, inside the compact set χ, the ANN

approximation error is negligibly small when the es-

timate ˆr

is equal to some unknown optimal vector

denoted by r

∗

. The

∗

notation denotes optimality.

In view of the stochastic adaptive approach taken

in this work, the unknown optimal parameter vector

∗

is treated as a random variable, with the initial con-

dition p(z

∗

) ∼ N (ˆz

), where the covariance P

reﬂects the conﬁdence in the initial guess ˆz

. More-

over, z

∗

is characterized as a stationary process cor-

rupted by an artiﬁcial process noise ρ

, which aids

convergence and tracking during estimation. In ad-

dition, observation uncertainty is catered for by aug-

menting a random measurement noise ǫ

to y

By (6), (7), all previous deﬁnitions and assump-

tions; it follows that the model in (3) can be repre-

sented in the following stochastic state-space form

∗

k+1

= z

∗

+ ρ

= h



k−1

,τ

k−1

∗



+ ǫ

(8)

where the vector function h



k−1

,τ

k−1

∗



is non-

linear in the unknown optimal parameter vector z

∗

and is given by

h(·) =

f(x

k−1

∗

) +

G(g

∗

)τ

k−1

. (9)

Since the resulting measurement model (8) is nonlin-

ear (due to the MLP network), in a stochastic frame-

work one has to a employ a nonlinear estimator, con-

ventionally the suboptimal EKF, to train the ANN in

real-time. However as shown in (Wan and van der

Merwe, 2001), the UKF can be a better alternative for

stochastic nonlinear estimation. Its beneﬁts over the

EKF include, a derivative-free algorithm and superior

accuracy in its approximations. For this reason, we

opt to employ the UKF in predictive mode for the es-

timation of z

∗

k+1

, as detailed below and in Lemma 3.1.

Deﬁnition 3.6. The information state denoted by I

consists of all output measurements up to instant k

and all the previous inputs; Y

and U

k−1

respectively.

Assumption 3.3. ǫ

and ρ

are both zero-mean white

Gaussian processes with covariances R

and Q

re-

spectively. Moreover, ǫ

, ρ

and z

∗

are mutually in-

dependent ∀k.

We propose the use of an unscented Kalman predic-

tor, detailed in Algorithm ??, to generate recursively

estimates for the mean and covariance of z

∗

k+1

condi-

tioned on I

, denoted respectively by ˆz

k+1

and P

k+1

This leads to the following lemma:

Lemma 3.1. In the light of (8), Deﬁni-

tion 3.6, and Assumption 3.3, it follows that

p(z

∗

k+1

) ≈ N (ˆz

k+1

), and so ˆz

k+1

is con-

sidered to be the estimate of z

∗

k+1

conditioned on

Proof. The proof follows directly by applying a pre-

dictive type UKF (additive noise version) on the non-

linear stochastic state-space model in (8). The pre-

dictive UKF is effectively the standard UKF algo-

rithm as presented in (Wan and van der Merwe, 2001)

for parameter estimation, with the difference that

the measurement-update step precedes that for time-

update. In addition, the time-update step is advanced

by one sample to obtain ˆz

k+1|k

at instant k.

Lemma 3.2. On the basis of Lemma 3.1, it follows

that p(y

k+1

) is approximately Gaussian with mean

ˆy

k+1

and covariance P

k+1

given by:

ˆy

k+1

, (10)

where,

∑

i=0

i,k+1|k

G(ˆg

k+1

) (11)

and the covariance

k+1

= (12)

∑

i=0



+ D



+ D



+ R

where, D

= F

i,k+1|k

−

, D

= G

i,k+1|k

−

IJCCI 2009 - International Joint Conference on Computational Intelligence

358

Algorithm 3.1. The unscented Kalman predictor algorithm.

Proof. The proof for

in (11) follows directly

by applying the UT to estimate the mean of



f(x

∗

k+1

)|I



. The equation for

in (11) is

simply an application of the basic results in linear

probability theory, i.e. p(Ax) = A¯x. It can be ap-

plied since

G is linear in the parameters. To derive the

equation of P

k+1

in (12) one needs to advance the

equation for P

in Algorithm 3.1. by one sampling

instant, and substitute for Y

i,k+1|k

and ˆy

k+1

, using the

relations leading to (10) in the same algorithm.

3.2.2 UT-based Dual Adaptive Control Law

The stochastic formulation in Lemmas 3.1 and 3.2

constitutes the weight adaptation law for the proposed

MLP dual adaptive scheme. In addition, it provides a

real-time update of the density p(y

k+1

). This in-

formation is crucial in dual control since unlike HCE

schemes, dual adaptive controllers do not ignore the

uncertainty of the estimates, but employ it in the de-

velopment of the control law itself, as follows.

The explicit-type suboptimal innovation-based

performance index J

inn

, adopted from (Fabri and

Kadirkamanathan, 2001), and modiﬁed to ﬁt

the multiple-input-multiple-output (MIMO) nonlin-

ear scenario at hand is given by

inn

= E

k+1

− y

k+1

)

k+1

− y

k+1

)







k+1





, (13)

where E



·|I



is the mathematical expectation con-

ditioned on I

, and the following deﬁnitions apply:

Deﬁnition 3.7. y

k+1

is the reference vector of y

k+1

and is given by y

k+1

= ν

k+1

− ν

To obtain ν

k+1

at instant k we advance the kinematic

control law by one sampling interval as explained in

(Bugeja and Fabri, 2009).

Deﬁnition 3.8. Design parameters Q

, Q

and Q

are diagonal and ∈ R

2×2

. Additionally: Q

positive deﬁnite, Q

is positive semi-deﬁnite, and

−Q

≤ Q

≤ 0 (element-wise).

Remark 3.1. The design parameter Q

is introduced

to penalize tracking errors, Q

induces a penalty on

large control inputs, and Q

affects the innovation

vector so as to induce the dual adaptive feature char-

acterizing this stochastic control law.

The UT-based dual adaptive control law proposed

in this paper is given in the following theorem.

Theorem 3.1. The control law minimizing perfor-

mance index J

inn

in (13), subject to: the WMR dy-

namic model in (3), all previous deﬁnitions, assump-

tions and lemmas, is given by



+ Q

+ N

k+1



−1





k+1

−



− n

k+1



(14)

where, N

k+1

∑

i=0

k+1

∑

i=0

= Q

+ Q

A NOVEL DUAL ADAPTIVE NEURO-CONTROLLER BASED ON THE UNSCENTED TRANSFORM FOR MOBILE

ROBOTS

359

Proof. Given the approximate Gaussian distribution

of p(y

k+1

) speciﬁed in Lemma 3.2, and several

standard results from multivariate probability theory,

it follows that cost function (13) can be rewritten as

inn

= (ˆy

k+1

− y

k+1

)

(ˆy

k+1

− y

k+1

)

+ τ

+ tr



k+1



. (15)

By substituting for ˆy

k+1

and P

k+1

in (15), using the

relations in (10) and (12) respectively, it is possible to

factorize completely in terms of τ

. The resulting ex-

pression is then differentiated with respect to τ

and

equated to zero, in order to get the dual control law in

(14). The resulting second order partial derivative of

inn

with respect to τ

, the Hessian matrix, is given by



+ Q

+ N

k+1



. By Deﬁnition 3.8

and (15), it is clear that the Hessian matrix is posi-

tive deﬁnite, meaning that τ

in (14) minimizes the

dual performance index in (13) uniquely. Moreover,

the latter implies that the inverse term in (14) exists

without exceptions.

Remark 3.2. Q

which appears in the control law

via Q

acts as a weighting factor, where at one ex-

treme, with Q

= −Q

, the controller completely ig-

nores the estimates’ uncertainty, resulting in HCE

control, and at the other extreme, with Q

= 0, it

gives maximum attention to uncertainty, which leads

to cautious control. For intermediate settings of Q

the controller strikes a compromise and operates in

dual adaptive mode. It is well known that HCE con-

trol leads to large tracking errors and excessive con-

trol actions when the estimates’ uncertainty is rela-

tively high. On the other hand, cautious control is

notorious for sluggish response and control turn-off

(Fabri and Kadirkamanathan, 2001). Consequently,

dual control exhibits superior performance by strik-

ing a balance between the two extremes.

4 SIMULATION RESULTS

This section presents a number of MATLAB

sim-

ulation results demonstrating the effectiveness of the

UT-based dual adaptive control scheme proposed in

this paper. Given the non-deterministic nature of

the stochastic system in question, one cannot rely

solely on a single simulation trial to validate the con-

troller under test. Moreover, the analytical proof of

strict convergence and stability for a dual adaptive

controller for a nonlinear system, is still considered

an open problem. For these reasons, a comprehen-

sive Monte Carlo comparative analysis is also pre-

sented. This renders the performance evaluation pro-

cess much more objective and reliable. In this anal-

ysis the proposed UT-based dual adaptive controller

detailed in Section 3 is compared to the recently pro-

posed EKF-based dual adaptive controller in (Bugeja

and Fabri, 2009).

4.1 Simulation Scenario

The differential WMR under study was simulated us-

ing the continuous-time dynamic model detailed in

(Bugeja and Fabri, 2009). To render the simulations

more realistic, a number of model parameters, namely

d, m

, I

and F ( ˙q), were allowed to vary about a

set of nominal values from one simulation trial to

another. These variations adhere to the physics of

realistic randomly generated scenarios, which repre-

sent various load conﬁgurations and surface frictional

conditions. The nominal parameter values used for

simulations correspond to those of Neurobot, the real

WMR we presented in (Bugeja and Fabri, 2008), with

a typical load. These are: b = 22.95cm, r = 6.25cm,

d = 10cm, m

= 32kg, m

= 1kg, I

= 0.84kgm

= 0.002kgm

, and I

= 0.005kgm

. Moreover,

viscous friction was included in the model by set-

ting F ( ˙q) = F

˙q, where F

is a diagonal matrix

of coefﬁcients, with nominal diagonal values set to

[2.6,2.6,0.35,0.3,0.3]. The control sampling interval

T was set to 50ms, and a zero-mean Gaussian mea-

surement noise with covariance 10

−4

I, where I de-

notes the identity matrix, was included.

Each simulation trial consists of eight consecutive

simulations. The ﬁrst six of these correspond to the

three modes of operation; i.e. HCE (Q

= −Q

cautious (Q

= 0) and dual (Q

= −0.8Q

); for

each of the two adaptive schemes being compared.

The remaining two trials correspond to: (1) a nomi-

nally tuned non-adaptive (NT-NA) controller, which

represents a non-adaptive dynamic controller that as-

sumes the model parameters to be equal to their nom-

inal values. This is the best a non-adaptive controller

can do when the exact robot parameters are unknown

(very realistic); (2) a non-adaptive controller which

is perfectly tuned (PT-NA) to the exact values of the

model parameters. The latter is not realistic, and is

used solely for the purpose of relative comparisons.

In contrast, the HCE, cautious and dual adaptive con-

trollers assume no preliminary information about the

robot whatsoever, since closed-loop control is acti-

vated immediately with the initial parameter estimate

vector ˆz

selected at random from a zero-mean, Gaus-

sian distribution with variance 0.0025.

For the sake of fair comparison the same noise se-

quence, reference trajectory, initial conditions, initial

ﬁlter covariance matrix (P

= 0.1I), artiﬁcial pro-

cess noise covariance (Q

= 10

−6

I), tracking er-

IJCCI 2009 - International Joint Conference on Computational Intelligence

360

ror penalty (Q

= I

), and control input penalty

= 0), are used in each simulation in a particu-

lar trial. In addition, the sigmoidal MLP ANN used

in each of the two schemes under test contained ﬁve

neurons (L = 5 ⇒ N = 27). Our experiments indi-

cated that adding more neurons did not improve the

control performance signiﬁcantly. In the UT-based

scheme, the UKF scaling parameters were set to α =

1,κ = 0 and β = 2. The noise sequence is randomly

generated afresh for each trial.

4.2 Single Trial Results

A number of simulation results, typifying the perfor-

mance of the three control modes of the UT-based

adaptive scheme are presented in Figure 3.

0 5 10 15 20

time (s)

|u| (Nm)

0 20 40 60

0.05

0.1

0.15

0.2

time (s)

− p|

0 5 10 15 20

0.1

0.2

0.3

0.4

0.5

time (s)

− p|

−2 0 2

−3

−2

−1

x (m)

y (m)

t = 60s

(d)

(c)

(a)

(b)

t = 0s

CAUTIOUS

HCE

DUAL

EKF dual adaptive scheme

UT-based dual adaptive scheme

HCE

CAUTIOUS

& DUAL

The HCE shoots up to 140Nm

Figure 3: (a): reference (×) and actual () trajectories

(UT-based dual); (b): control input (UT-based 3 modes);

(c): transient tracking error (UT-based 3 modes); (d): tran-

sient tracking error (UT-based dual vs EKF-based dual).

Plot (a) depicts the WMR tracking a demanding ref-

erence trajectory, with a non-zero initial tracking er-

ror, controlled by the proposed UT-based dual adap-

tive controller. It depicts the good tracking perfor-

mance of the proposed scheme, even when the trajec-

tory reaches high speeds of around 1m/s. Plots (b) to

(d) correspond to another simulation trial; purposely

initiated with zero error conditions, so that any initial

transient errors can be fully attributed to the conver-

gence of the estimators. Plot (b) compares the Eu-

clidian norm of the control input vector, for the three

modes of the UT-based controller, during the ﬁrst 20s.

The very high transient control inputs of the HCE

controller reﬂect the aggressive and incautious nature

of this mode, which ignores completely the high un-

certainty in the initial estimates. Plot (c) compares the

Euclidian norm of the pose error vector, for the three

modes of the UT-based controller, during the ﬁrst 20s.

This plot shows clearly how the HCE mode typically

EKF (HCE) EKF (CAU) EKF (DUA) UT (HCE) UT (CAU) UT (DUA) NT−NA PT−NA

200

400

600

800

1000

1200

1400

COST

HCE: HCE MODE

CAU: CAUTIOUS MODE

DUA: DUAL MODE

NT-NA: NOMINALLY TUNED NON-ADAPTIVE

PT-NA: PERFECTLY TUNED NON-ADAPTIVE

Figure 4: Monte Carlo analysis cost distributions (100runs).

leads to high initial transient errors, while the dual

mode exhibits the best transient performance. This

is in accordance with Remark 3.2. Plot (d) compares

the UT-based dual adaptive controller with the EKF-

based dual adaptive controller. The plot indicates that

the former has better transient performance, while in

steady-state the two controllers lead to the same error.

In addition, with minimal code optimization the

computation time for the proposed UT-based dual

controller is around 30% more than that of the EKF-

based dual controller. This is not unexpected, mainly

due to the time-intensive sigma-points propagation

within the UKF algorithm. Yet, the computation time

of the UT-based controller is still around 12% of the

whole sampling period.

4.3 Monte Carlo Comparative Results

A Monte Carlo simulation involving 100 simulation

trials was performed. Each of the eight simulations

in a trial corresponds to a trajectory time horizon of

one minute in real time under the simulation condi-

tions speciﬁed earlier (and with zero error initial con-

ditions). After each simulation the following cost

COST =

fin

∑

k=1

− p| is calculated. This serves as a

performance measure for each of the eight controllers

operating under the same conditions, where lowerval-

ues of COST are naturally preferred.

The salient statistical features of the eight cost dis-

tributions resulting from the Monte Carlo analysis,

are depicted in the boxplot of Figure 4. Additionally,

the mean and variance of each of these cost distribu-

tions are listed and ranked in Table 1. These results in-

dicate clearly that in general the UT-based dual adap-

tive controller brings about a signiﬁcant improvement

in tracking performance, not only over non-adaptive

controllerswhich assume nominal values for the robot

parameters, but also over the EKF-based dual con-

troller presented in (Bugeja and Fabri, 2009). More-

A NOVEL DUAL ADAPTIVE NEURO-CONTROLLER BASED ON THE UNSCENTED TRANSFORM FOR MOBILE

ROBOTS

361

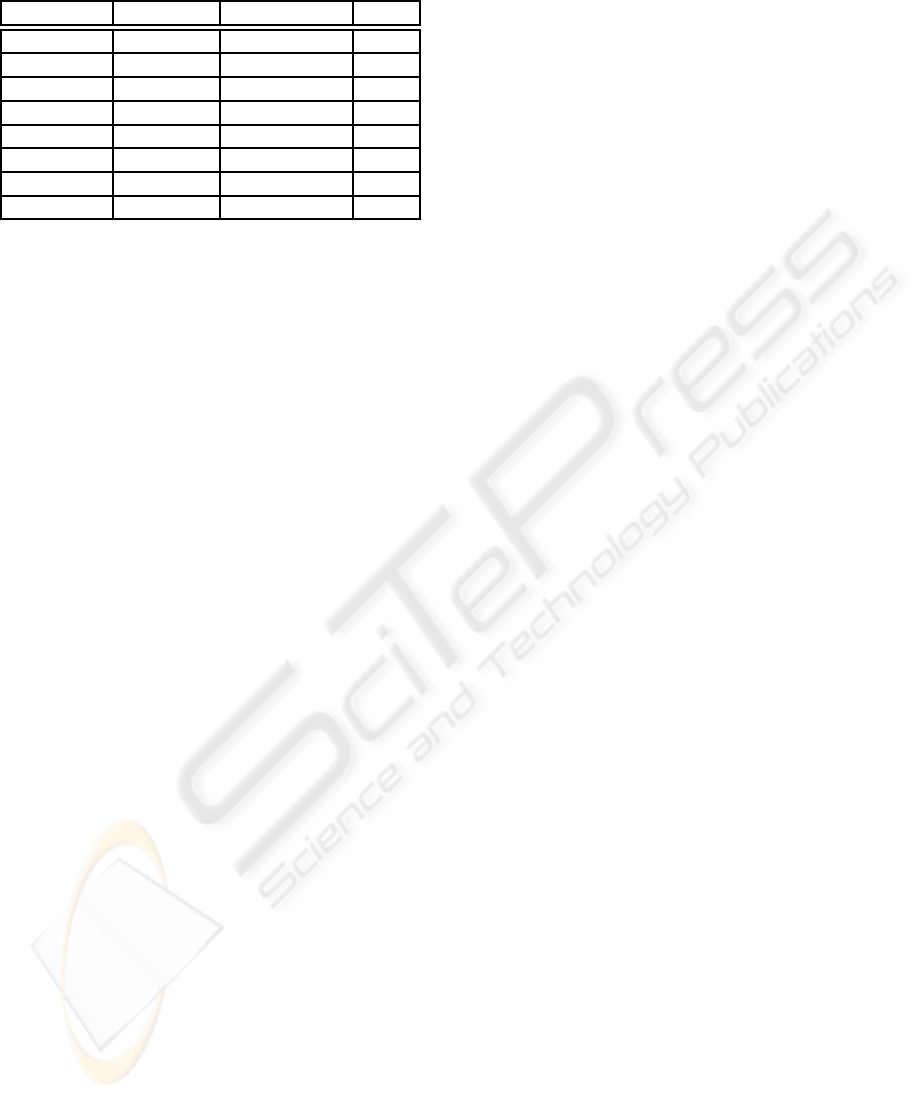
Table 1: Mean and Variance of the cost distributions.

Controller Mean cost Cost variance Rank

EKF-HCE 192 40225 6

EKF-CAU 67 3847 4

EKF-DUA 61 731 3

UT-HCE 140 32813 5

UT-CAU 48 30 2

UT-DUA 47 26 1

NT-NA 372 59614 7

PT-NA 39 5 -na-

over, it is just as evident that within each of the two

schemes, the dual control mode is even better than

the cautious mode, as anticipated in Remark 3.2. This

complies with the dual control philosophy that a bal-

ance between caution and probing yields the best per-

formance in adaptive control. It is also not surprising

that the performance of the HCE modes is character-

ized by a high cost variance and several extreme out-

liers. This is the result of the complete lack of caution

in the presence of high initial uncertainty, leading to

high transient errors. An important observation is that

each mode in the UT-based scheme is superior to the

corresponding mode in the EKF-based scheme. We

associate this to the superior (second order) approxi-

mations introduced by the UT when compared to the

EKF (ﬁrst order).

5 CONCLUSIONS

The novelty in this paper comprises the use of the

UT to improve on the EKF-based dual-adaptive dy-

namic controller recently proposed in (Bugeja and

Fabri, 2009). Speciﬁcally the proposed UT-based

dual-adaptive scheme employs the UKF (in predic-

tive mode) as a recursiveweight tuning algorithm, and

in addition includes a novel dual-adaptive control law

that uses the UT to propagate nonlinear mappings of

distributions, rather that the ﬁrst order approximations

involved in the EKF-based law.

The presented results show clearly that the proposed

novel dual controller exhibits signiﬁcant improve-

ments in performance, not only over the EKF-based

dual scheme, but also on all other non-dual and non-

adaptive controllers tested in this paper.

Recently we have also implemented this novel con-

troller successfully on Neurobot. The obtained exper-

imental results validate the proposed scheme on a real

mobile robot for the ﬁrst time and will soon be pub-

lished elsewhere.

REFERENCES

Astr¨om, K. J. and Wittenmark, B. (1995). Adaptive Control.

Addison-Wesley, Reading, MA, 2nd edition.

Bugeja, M. K. and Fabri, S. G. (2008). Multilayer per-

ceptron adaptive dynamic control of mobile robots:

experimental validation. In H. Bruyninckx, L. P.

and Kulich, M., editors, European Robotics Sympo-

sium 2008 (EUROS’08),Prague, Springer Tracts in

advanced Robotics (STAR), pages 165–174. Springer.

Bugeja, M. K. and Fabri, S. G. (2009). Dual adaptive dy-

namic control of mobile robots using neural networks.

IEEE Trans. Syst., Man, Cybern. B, 39(1):129–141.

Canudas de Wit, C., Khennoul, H., Samson, C., and Sor-

dalen, O. J. (1993). Nonlinear control design for mo-

bile robots. In Zheng, Y. F., editor, Recent Trends

in Mobile Robots, Robotics and Automated Systems,

chapter 5, pages 121–156. World Scientiﬁc.

Corradini, M. L. and Orlando, G. (2001). Robust tracking

control of mobile robots in the presence of uncertain-

ties in the dynamic model. Journal of Robotic Sys-

tems, 18(6):317–323.

Fabri, S. G. and Kadirkamanathan, V. (2001). Functional

Adaptive Control: An Intelligent Systems Approach.

Springer-Verlag, London, UK.

Fierro, R. and Lewis, F. L. (1995). Control of a nonholo-

nomic mobile robot: Backstepping kinematics into

dynamics. In Proc. IEEE 34th Conference on Deci-

sion and Control (CDC’95), pages 3805–3810, New

Orleans, LA.

Julier, S. J. and Uhlmann, J. K. (1997). A new ex-

tention of the Kalman ﬁlter to nonlinear systems.

In Proc. of AeroSense: The 11th Int. Symp. on

Aerospace/Defence Sensing, Simulation and Controls.

Kanayama, Y., Kimura, Y., Miyazaki, F., and Noguchi, T.

(1990). A stable tracking control method for an au-

tonomous mobile robot. In Proc. IEEE International

Conference of Robotics and Automation, pages 384–

389, Cincinnati, OH.

Wan, E. A. and van der Merwe, R. (2001). The unscented

kalman ﬁlter. In Haykin, S., editor, Kalman Filtering

and Neural Networks, Adaptive and Learning Systems

for Signal Processing, Communications, and Control,

chapter 7, pages 221–280. John Wiley & Sons, Inc.

Wang, T.-Y. and Tsai, C.-C. (2004). Adaptive trajectory

tracking control of a wheeled mobile robot via lya-

punov techniques. In Proc. 30th Annual Conference

of the IEEE Industrial Electronics Society, pages 389–

394, Busan, Korea.

IJCCI 2009 - International Joint Conference on Computational Intelligence

362