
FREQUENCY EXTRACTION BASED ON ADAPTIVE FOURIER
SERIES
Application to Robotic Yoyo
Tadej Petriˇc, Andrej Gams and Leon
ˇ
Zlajpah
Department of Automation, Biocybernetics and Robotics, Joˇzef Stefan Institute, Jamova cesta 39, 1000 Ljubljana, Slovenia
Keywords:
Fourier series, Nonlinear dynamical systems, Adaptive frequency oscillators, Imitation, Yo-yo.
Abstract:
We present a novel method to obtain the basic frequency of an unknown periodic signal with an arbitrary
waveform. The method originates from nonlinear dynamical systems for frequency extraction, which are
based on adaptive frequency oscillators in a feedback loop. While using several adaptive frequency oscillators
in a loop results in extraction of separate frequency components, our method extracts the basic frequency of
the input signal without any additional logical operations. The proposed method uses a whole Fourier series
representation in the feedback loop. In this way it can extract the frequency and the phase of an unknown
periodic signal, in real-time, and without any additional signal processing or preprocessing. The method also
determines the Fourier series coefficients and can be used for dynamic Fourier series implementation. It can
be used for the control of rhythmic robotic tasks, where only the extraction of the fundamental frequency is
crucial. This is demonstrated on a highly nonlinear and dynamic task of playing the robotic yo-yo.
1 INTRODUCTION
Controlling rhythmic robotic tasks that require syn-
chronization with the actuated device or interac-
tion with the external environment is a difficult
task, and requires complex sensory systems and ad-
vanced knowledge (Petriˇc et al., 2009). For example,
such rhythmic tasks include handshaking (Kasuga
and Hashimoto, 2005), locomotion (Ijspeert, 2008),
drumming(Degallier et al., 2008), or playing with dif-
ferent toys, like the yo-yo(
ˇ
Zlajpah, 2006) or the gyro-
scopic device called Powerball (Gams et al., 2007).
Controlling these tasks with robots requires both ac-
curate trajectory generation and frequency tuning.
Determining the fundamental frequency of a task
is a complex problem and can be achieved in differ-
ent ways, e.g. with signal processing methods, such
as FFT, or with the use of nonlinear oscillators (Mat-
suoka et al., 2005). Furthermore, trajectory genera-
tion and modulation are still difficult tasks in robotics.
One possible approach to trajectory generation and
the modulation is the imitation (Schaal, 1999), which
can be preformed in several different ways, using
encoding methods like splines (Ude et al., 2000) or
dynamic movement primitives (DMP) (Schaal et al.,
2007).
Not many approaches that combine both frequency
extraction and waveform learning exist. One of them
is the use of a two-layered imitation system based on
nonlinear dynamical systems (Gams et al., 2009). In
their work, the authors explained that the imitation
system can be used for extracting the frequency of
the input signal, learning its waveform, and imitating
the waveform at the extracted or any other frequency.
Similar, but with less properties for trajectory genera-
tion and modulationcan be achievedby using only the
first layer of this system for both frequency extraction
and waveform learning (Righetti et al., 2006). The de-
scribed systems are based on adaptive frequency os-
cillators in a feedback loop. Such an approach can
determine several frequency components of an input
signal. Despite favorable properties of these systems,
there is a considerable drawback in determining the
basic or fundamental frequency of the input signal.
For complex periodic signals with several fre-
quency components, the first layer of the imitation
system, referred to as the canonical dynamical sys-
tem, has to include a high number of oscillators in the
feedback loop. Using this system for movement imi-
tation requires determining the basic frequency. This
is accomplished by a logical algorithm that follows
the feedback loop. With a high number of oscilla-
32
Petri
ˇ
c T., Gams A. and Žlajpah L. (2010).
FREQUENCY EXTRACTION BASED ON ADAPTIVE FOURIER SERIES - Application to Robotic Yoyo.
In Proceedings of the 7th International Conference on Informatics in Control, Automation and Robotics, pages 32-38
DOI: 10.5220/0002933900320038
Copyright
c
SciTePress
tors, and when several of the oscillators tune to the
same frequency, this can become extremely complex.
A high number of oscillators is practically necessary
and cannot be avoided.
The contribution of this paper is the novel design
of the canonical dynamical system for the two-layered
imitation system. The proposed approach does not re-
quire a logic algorithm to determine the fundamental
frequency of the input signal, as in the original ap-
proach (Gams et al., 2009). We use a single adaptive
phase oscillator in a feedback loop. The oscillator is
followed by a complete Fourier series approximation,
with a built-in algorithm to determine the Fourier co-
efficients. The combination of an adaptive phase os-
cillator and the adaptive Fourier series allows us to
extract the fundamental frequency of the input signal
and use it to control rhythmic robotic tasks.
With this approach we essentially implemented a
real-time, adaptive Fourier series analysis. Our sys-
tem is able to calculate the Fourier coefficients of an
unknown periodic signal in real-time and is computa-
tionally inexpensive. The usefulness of this system is
presented on the case of playing the yo-yo. Control-
ling the yo-yo has already been a subject of several
studies (
ˇ
Zlajpah, 2006; Jin et al., 2009), which mostly
rely on complex, specially designed controllers based
on the models of the device. The task of playing
yo-yo is highly non-linear and requires on-line fre-
quency adaptation. The proposed approach simplifies
the synchronization between the upward jerk of the
robot and the movement of the yo-yo by determining
the frequency of the up-down motion from a measur-
able periodic quantity.
The paper is organised as follows. In section 2,
we give a brief description of the original two-layered
imitation system with an emphasis on the first layer -
the canonical dynamical system. In section 3 we de-
scribe the novel approach using the Fourier series in
the feedback loop. In section 4 we evaluate the pro-
posed approach in simulation, and on a real-world ex-
periment of playing the yo-yo. Conclusions and sum-
mary are in section 5.
2 TWO-LAYERED IMITATION
SYSTEM
The two-layered imitation system was presented in
detail in (Gams et al., 2009). In their work the au-
thors explained that the system can be used for ex-
tracting the frequency spectrum of the input signal,
learning the waveform of one period, and imitating
the desired waveform at an arbitrary frequency. The
system structure is presented in Figure 1. The first
layer, i.e. the canonical dynamical system, is used
for frequency extraction. It is based on a set of adap-
tive frequency oscillators in a feedback loop. The sec-
ond layer is called the output dynamical system and is
used for learning and repeating the desired waveform.
The latter is based on dynamic movement primitives
- DMPs, e.g. (Schaal et al., 2007).
Canonic
dynamic
system
Output
dynamic
system
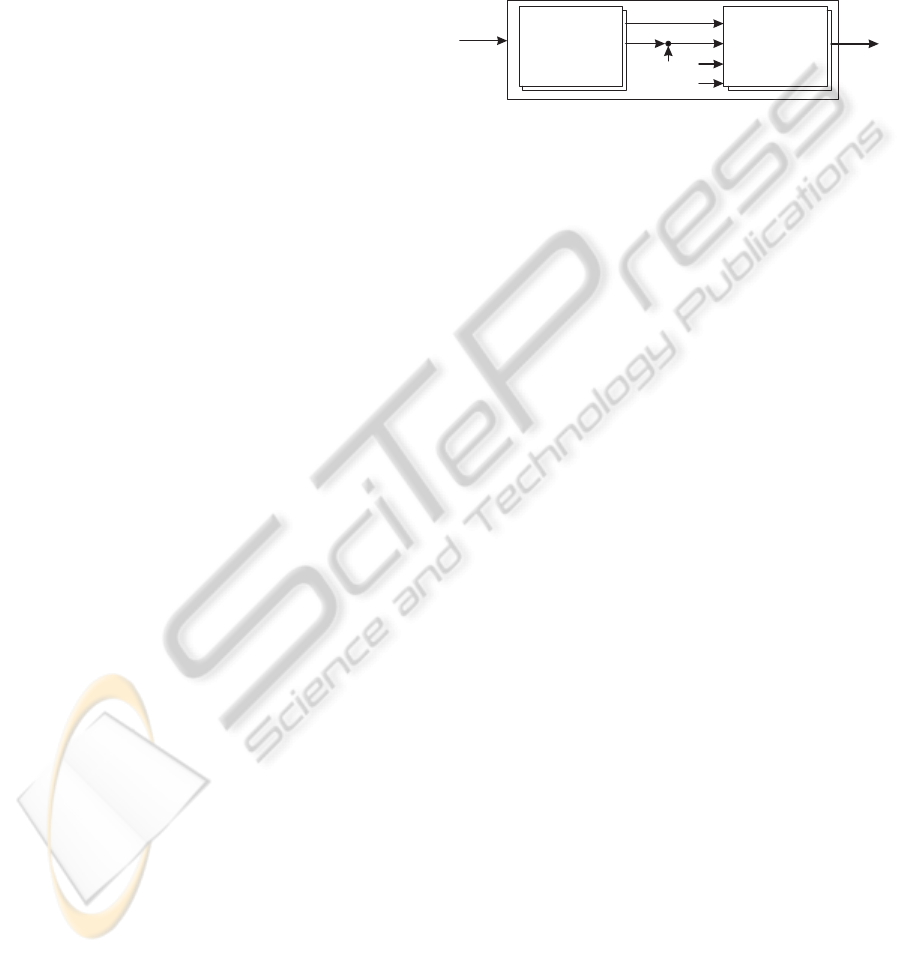
y
in
Ω
φ
x
w
φ
l
r
Figure 1: Two-layered structure of the imitation system.
The input y
in
is a measured quantity and the output is the
desired trajectory x of the robot. The input φ
l
is the ad-
ditional phase lag and r is the amplitude of the trajectory.
The system can work in parallel for an arbitrary number of
dimensions.
The first layer of the system has two major tasks.
It has to extract the fundamental frequency Ω of the
input signal and it has to exhibit stable limit cycle be-
havior in order to provide the phase signal Φ. The
basis of the canonical dynamical system is a set of
adaptive phase oscillator with applied learning rule as
introduced in (Buchli and Ijspeert, 2004). In order
to accurately determine the frequency, it is combined
with a feedback structure (Buchli et al., 2008) (see
Figure 2). The feedback structure of M adaptive fre-
quency oscillators is governed by
˙
φ
i
= ω
i
− K · e· sinφ
i
, (1)
˙
ω
i
= −K · e· sinφ
i
, (2)
e = y
in
− ˆy, (3)
ˆy =
M
∑
i=1
α
i
cosφ
i
, (4)
˙
α
i
= η · e· cosφ
i
, (5)
where K is the coupling strength, φ
i
, i = 1...M is the
phase of separate oscillators, y
in
is the input signal, M
is the number of oscillators, α
i
is the amplitude as-
sociated with the i-th oscillator, and η is the learning
constant.
As shown in Figure 2, each of the oscillators in
the feedback structure receives the same input, i.e. the
difference between the input signal and the weighted
sum of separate frequency components. Such a feed-
back structure preforms a kind of Fourier analysis.
The number of extracted frequencies depends on how
many oscillators are used. As only the fundamental
frequency is of interest, the feedback structure is fol-
lowed by a logic algorithm. Determining the correct
frequency and the phase is crucial, because they are
FREQUENCY EXTRACTION BASED ON ADAPTIVE FOURIER SERIES - Application to Robotic Yoyo
33

+
-
y
in
e
ˆy
Ω, Φ
∑
α
i
cos(φ
i
)
ω
1
, φ
1
ω
2
, φ
2
ω
3
, φ
3
ω
M
, φ
M
Logic
Figure 2: Feedback structure of M nonlinear adaptive fre-
quency oscillators. Note the logic algorithm that follows the
feedback loop.
e
y
in
Ω
φ
ˆy
α
n
,β
n
Figure 3: Feedback structure of nonlinear adaptive fre-
quency oscillator combined with dynamic Fourier series.
Note that no logic algorithm needed.
the basis for the output dynamic system and the de-
sired behavior of the actuated device.
One possible approach is to choose the first non-
zero frequency as was presented in (Gams et al.,
2009). However, it has a drawback that when more
than one oscillators converge to, or oscillate, around
the same frequency, the logic algorithm switches be-
tween the oscillators, and consequently the phase will
not be smooth, leading to oscillations in the output
trajectory.
3 CANONICAL DYNAMICAL
SYSTEM BASED ON FOURIER
SERIES
In this section a novel architecture for canonical dy-
namical system is presented. As the basis of the
canonical dynamical system we use a single nonlin-
ear phase oscillator with applied learning rule (Buchli
and Ijspeert, 2004). This is combined with a feed-
back structure based on an adaptive Fourier series in
order to accurately determine the frequency. A feed-
back structure with an adaptive frequency oscillator
combined with an adaptive Fourier series is shown in
Figure 3. The feedback structure of an adaptive fre-
quency phase oscillator is governed by
˙
φ = Ω − K · e· sinφ, (6)
˙
Ω = −K · e· sinφ, (7)
e = y
in
− ˆy, (8)
where K is the coupling strength, φ is the phase of the
oscillator, e is the input into the oscillator and y
in
is
the input signal. If we compare Eqs. (1, 2) and Eqs.
(6, 7), we can see that the frequency Ω and the phase
φ are now clearly defined. The feedback loop ˆy is now
represented by the Fourier series
ˆy = α
0
+
M
∑
i=1
(α
i
cos(iφ) + β
i
sin(iφ)), (9)
and not by the sum of separate frequency components
as in Eq. 4. M is the size of the Fourier series and α
0
is the amplitude associated with the first segment of
the series, it is governed by
˙
α
0
= η · e, (10)
here η is a learning constant. The amplitudes asso-
ciated with the other terms of the Fourier series are
determined by
˙
α
i
= ηcos(iφ) · e, (11)
˙
β
i
= ηsin(iφ) · e, (12)
where i = 1...M. As shown in Figure 3, the oscillator
of the feedback structure receives the difference be-
tween the input signal and the Fourier series. Since
a negative feedback loop is used, the difference ap-
proaches zero when the Fourier series representation
approaches the input signal. Such a feedback struc-
ture preforms an adaptive Fourier analysis, where the
phase difference between the harmonics can only be
0, π/2, π or 3π/2. This is not the case in the origi-
nal approach (Righetti and Ijspeert, 2006), where the
phase difference can be arbitrary.
The proposed approach has the ability to adapt to
the basic frequencyof the input signal. The number of
harmonic frequency components it can accurately ex-
tract depends on how many terms of the Fourier series
are used. Since in this structure only one oscillator is
used and the harmonics are encoded in the Fourier
series, the basic frequency and phase are clearly de-
fined. This is an important improvement, especially
for the usefulness of the imitation system when per-
forming rhythmic tasks.
The new architecture of the canonical dynamic
system can be used as an imitation system by itself,
as it is able to learn arbitrary periodic signals. Af-
ter convergence, e reaches zero (with an accuracy that
depends on the number of elements of the Fourier se-
ries). Once e is zero, the periodic signal stays encoded
in the Fourier series. The learning process is embed-
ded and is done in real-time. There is no need for any
external optimization process or learning algorithm.
ICINCO 2010 - 7th International Conference on Informatics in Control, Automation and Robotics
34
Adding the output dynamical system enables us to
synchronise the motion of the robot to a measurable
periodic quantity of the task we would like to preform.
The measured signal is now encoded into the Fourier
series and the desired robot trajectory is encoded in
the output dynamic system. Sice adaptation of the
frequency and the learning of the desired trajectory
can be done simultaneously, all of the system time-
delays can be automatically included. Furthermore,
when a predefined motion pattern for the trajectory
is used, the system time-delays can be adjusted with
a phase lag parameter φ
l
. This enables us to either
predefine the desired motion or to teach the robot how
to preform the desired rhythmic task online.
The output dynamical system also ensures greater
robustness against perturbations and smooth modula-
tion. Specially greater robustness to perturbation is
crucial when performing fast, dynamic tasks.
4 EVALUATION
In the following section we evaluate of the proposed
imitation system with the new canonical dynamical
layer. In the Section 4.1 the numerical results from
the original and the novel architectures are presented.
In Section 4.2 a real-world experiment of playing the
yo-yo with the use of the proposed imitation system
is shown.
4.1 Simulation
In this numerical experiment the proposed architec-
ture for the canonical dynamical system learns an ar-
bitrary signal. The populating of the frequency spec-
trum is done without any signal processing, as the
whole process of frequency extraction and adaptation
of the waveform is completely embedded in the dy-
namics of the adaptive frequency oscillator combined
with the adaptive Fourier series. Unless stated other-
wise, we use the following parameters: µ = 2, K = 20,
M = 10.
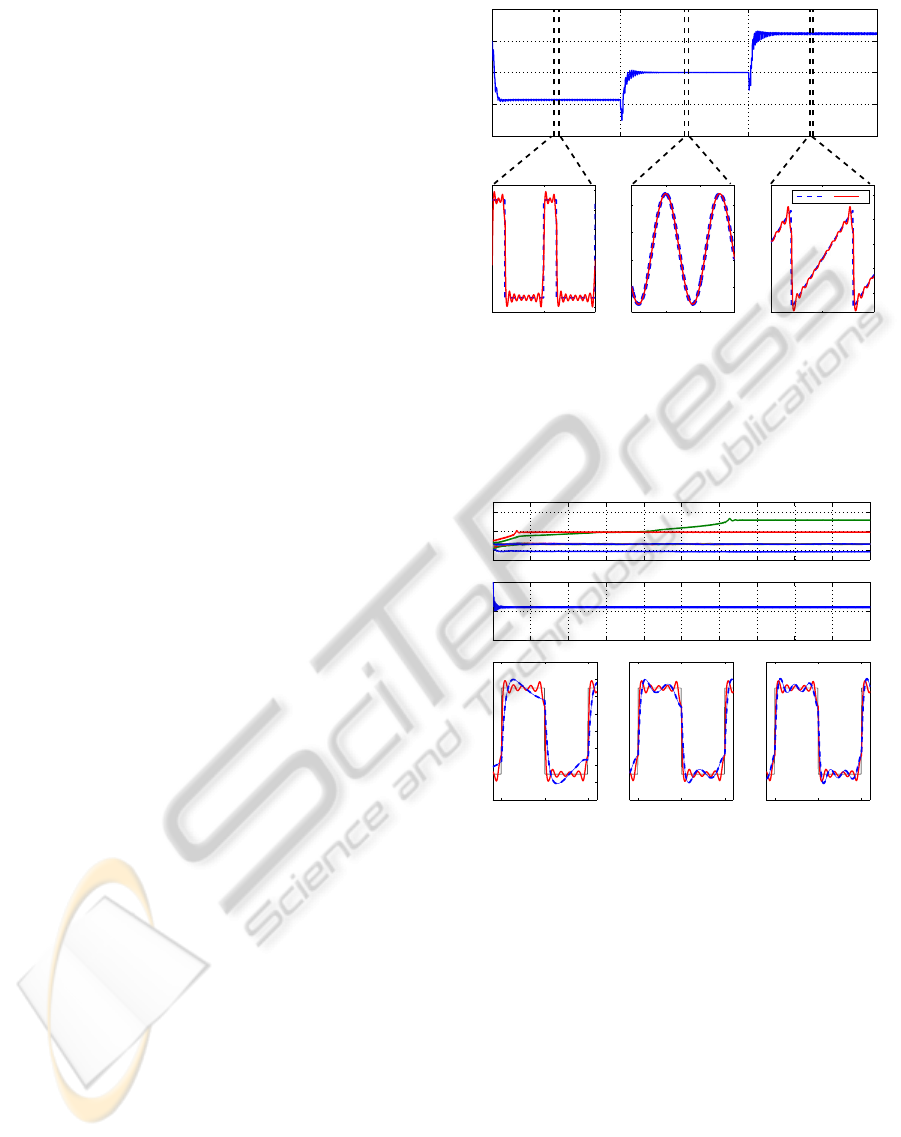
Frequency adaptation results from time- and
shape-varying signals are illustrated in Figure 4. The
input signal itself is of three parts: a periodic pulse
signal, a sinusoid, and a sawtooth wave signal. Tran-
sition between the signal parts is instant for both fre-
quency and waveform. We can see that after the
change of the input signal, the output frequency sta-
bilises very quickly.
A single adaptive frequency oscillator in a feed-
back loop is enough, because the harmonics of the
input signal are encoded with the Fourier series in
the feedback loop. As can be seen from the bottom
0 50 100 150
4
6
8
10
12
24 25 26
−0.4
−0.2
0
0.2
0.4
0.6
0.8
75 75.5 76 76.5
−1
−0.5
0
0.5
124 124.5 125
−1
−0.5
0
0.5
1
y
y
ˆy
Time [s]
Time [s]
Ω rad/s
Figure 4: Typical convergence of an adaptive frequency os-
cillator combined with an adaptive Fourier series, driven by
a periodic signal with different waveforms and frequencies.
Frequency adaptation is presented in the top plot and the
comparison between the input signal y and the approxima-
tion ˆy in the bottom plot.
0 50 100 150 200 250 300 350 400 450 500
0
20
40
0 50 100 150 200 250 300 350 400 450 500
4
6
8
20 20.5 21
−0.8
−0.6
−0.4
−0.2
0
0.2
0.4
0.6
150 150.5 151 350 350.5 351
y
Time [s]
Ω rad/s Ω rad/s
Figure 5: Comparison between the pool of the adaptive os-
cillators and our proposed approach. First plot shows evolu-
tion of frequency distribution using a pool of 10 oscillators.
Middle plot shows the extracted frequency using an adap-
tive frequency oscillator combined with Fourier seres. The
comparison of approximated signals is presented in the bot-
tom plot. The thin solid line presents the input signal, the
solid line presents our new proposed approach and the dot-
ted line presents the pool of adaptive oscillators.
plots in Figure 4, the input signal and the feedback
signal are very well matched. The approximation er-
ror depends only on M. A comparison with the orig-
inal approach as proposed in (Buchli et al., 2008) is
given in Figure 5. In their approach, if there are not
enough oscillators to encode the input signal, the sys-
tem will only learn the frequency components with
more power. Thus, the output signal will only be an
approximation.
FREQUENCY EXTRACTION BASED ON ADAPTIVE FOURIER SERIES - Application to Robotic Yoyo
35
Mitsubishi PA-10
Force sensor
Gripper
Camera
Line
Yo-yo
Figure 6: Experimental setup.
Canonic
dynamic
system
Output
dynamic
system
yoyo
R
y
f
y
v
Ω
φ
x
w
φ
l
r
h
d
˜
h
Figure 7: Proposed two-layered structure of the control sys-
tem for controlling the peak height of the yo-yo. The input
is either the force y
f
or the visual feedback y
v
.
However, if there are more oscillators than the fre-
quency components to learn, either some of them will
not converge to any frequency or the same frequency
components will be coded by several oscillators, as
shown in the top plot in Figure 5, where a pool of ten
oscillators was used. In this particular experiment,
five of the oscillators converge to the basic frequency
of the signal.
Choosing the right oscillator from that pool is a
very difficult task and requires a complex logic algo-
rithm. On the other hand, using our new approach,
where the feedback is encoded with a Fourier series,
the oscillator converges to the basic frequency of the
input signal. Therefore, the basic frequency and the
phase are clearly defined. Furthermore, the approx-
imation and the convergence of the feedback signal
is quicker, as it is shown in the bottom plots in Fig-
ure 5. Even after 350 s, the original architecture from
(Righetti and Ijspeert, 2006) did not produce as good
an approximation as it was after 20 s when using our
new proposed canonical dynamical system.
4.2 Application to Robotic Yo-yo
To illustrate the proposed approach we implemented
it on a real robot playing yo-yo.
Playing yo-yo with a robot can be achieved in dif-
ferent ways, depending on what one can measure. It
can be the length of the unwound string, which can
be effectively measured by a vison system. As de-
scribed in (
ˇ
Zlajpah, 2006), using vison is also one of
the ways humans do it, even though approaches us-
ing only the measured force were described (Jin et al.,
2009). With our proposed system, playing yo-yo can
be accomplished either with force feedback or with
visual feedback. Furthermore, the proposed system is
able to synchronise even if the input signal is changed
from one measurable quantity to another during the
experiment.
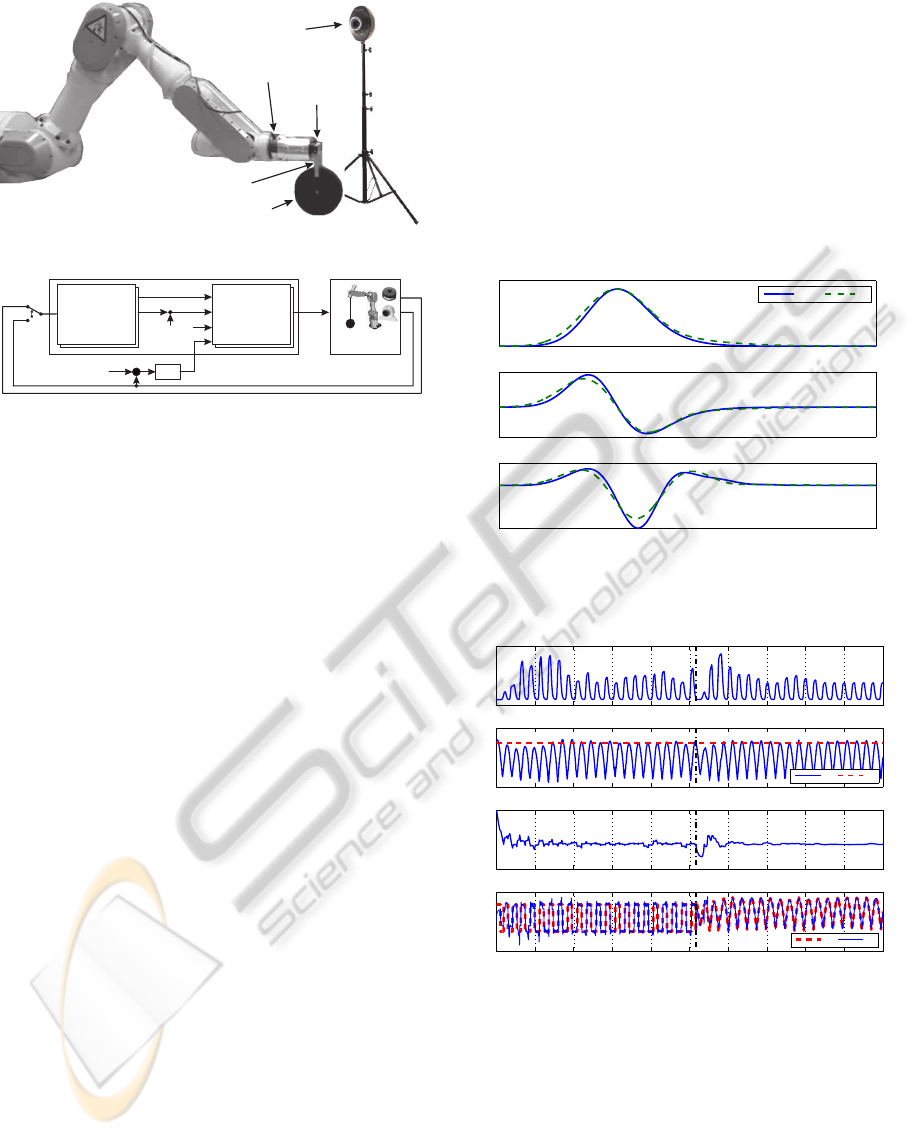
We preformed the experiment on a Mitsubishi PA-
10 robot as presented in Figure 6. A force sensor
(JR3), was attached to the end effector to measure the
impact force of the yoyo, and a USB camera was used
to measure the length of the unwound string.
h
h
ref
h
˙
h
0 1
¨
h
t [s]
Figure 8: Pre-defined hand motion pattern for playing yo-
yo.
0
0.5
−0.8
0.2
0
6
0 10 20 30 40 50 60 70 80 90 100
−0.6
0.6
y
ˆy
y
in
Time [s]
Ω [rad/s]
x [m]
h [m]
h
h
d
Figure 9: Robot trajectory x in the top plot, height h of the
yo-yo in the second plot, extracted frequency in the third
plot and signal adaptation ˆy in the bottom plot. At 52 s
the input signal is switched form force feedback to visual
feedback. Yo-yo parameters in this case are: axle radius
r
a
= 0.01 m and mass m = 0.2564 kg.
The two layered imitation system with the novel
canonical dynamical system was implemented in
Matlab/Simulik. The control scheme is presented
in Figure 7. As we can see, the imitation system,
based on a nonlinear oscillator combined with dy-
ICINCO 2010 - 7th International Conference on Informatics in Control, Automation and Robotics
36

0 s
0.32 s
0.64 s
0.96 s
1.28 s
1.6 s
1.92 s
2.24 s
t
1
s
t
1
+ 0.32 s
t
1
+ 0.64 s
t
1
+ 0.96 s
t
1
+ 1.28 s
t
1
+ 1.6 s
t
1
+ 1.92 s
t
1
+ 2.24 s
t
2
s
t
2
+ 0.32 s
t
2
+ 0.64 s
t
2
+ 0.96 s
t
2
+ 1.28 s
t
2
+ 1.6 s
t
2
+ 1.92 s
t
2
+ 2.24 s
Figure 10: Image sequence of a robotic yoyo. Top sequence present the decent of the yoyo from the gripper. In the middle
sequence a behavior of the system after switching from force feedback to vision feedback is shown (t
1
= 54 s) and in bottom
sequence the behavior in steady state is presented (t
2
= 81 s).
namic Fourier series, provides the desired trajectory
for the robot with a yo-yo attached at the top. The mo-
tion of the robot was constrained to up-down motion
using inverse kinematics. The length of the string or
the force from the top of the robot can be used as the
input into the system. Since a measurable force dif-
ference appears only as a spike, when the yo-yo hits
the end of the string, we modify the signal in a way
that it carries more energy. In our particular case, we
use the measured spike to create a short pulse.
To preform the task, we first determine the wave-
form of the required motion pattern. We chose the
motion pattern described in (
ˇ
Zlajpah, 2006), which
satisfies the required criteria for playing the yo-yo.
The hand motion pattern encoded into the output dy-
namic system (dashed line), and the desired hand mo-
tion pattern (solid line) are presented in Figure8.
The frequency of the task depends on the param-
eters of the yo-yo itself, and on how high the yo-yo
rolls up along the string. The height can be influ-
enced by the amplitude of the hand motion, which can
be easily modified using the amplitude parameter r of
the motion, see Figure 7. PI controller was used to
control the peak height of the yo-yo. The controller is
given by
u(t) = k
p
e(t) + k
i
Z
e(t)dt, (13)
where k
p
= 2, and k
i
= 0.4 were determined empiri-
cally. Figure 9 shows the results of frequency adapta-
tion and yo-yo height during the experiment.
As we can see, the frequency of the imitated mo-
tion quickly adapted to the motion of the yo-yo and
stable motion was achieved. At approximately 52 s
the input into the imitation system was switched from
force feedback to visual feedback. At that point some
oscillation in the frequency and the approximation of
the input signal can be observed because they have to
adapt to the new waveform of the input signal. Fur-
thermore, form the middle sequence in Figure 10 we
can see that the amplitude of hand motion is higher
after switching from the force feedback to the vision
feedback. Despite the change, the imitation system
still manages to extract the correct frequency and the
robot motion returns to steady-state oscillations. Note
that in the bottom sequence in Figure 10 the hand am-
plitude is smaller than immediately after the switch.
FREQUENCY EXTRACTION BASED ON ADAPTIVE FOURIER SERIES - Application to Robotic Yoyo
37

As far as we know, this is the first system which
has the capability of playing the yo-yo by force feed-
back or by vision feedback, without changing the sys-
tem parameters. Furthermore, switching from one to
another measured quantity can even be done during
the experiment. This shows that the proposed system
is adaptable and robust.
5 CONCLUSIONS
We presented a new architecture for the canonical dy-
namical system which is a part of a two layered imi-
tation system, but can be used as an imitation system
by itself. The dynamical system which, is used to ex-
tract the frequency, is composed of a nonlinear phase
oscillator combined with a Fourier series. This sys-
tem essentially implements an adaptive Fourier series
of the input signal. It can extract the frequency, phase
and the Fourier series coefficients of an unknown pe-
riodic signal. This is done in real-time without any
additional processing of the input signal. Integrating
this system into the imitation system based on dy-
namic motion primitives enables simple and compu-
tationally inexpensive control of rhythmic tasks with
at least one measurable periodic quantity.
Furthermore, we presented the use of the imita-
tion system to preform a rhythmic task that requires
synchronization with the controlled device. For play-
ing the yo-yo, we have shown that the information
on how high the yo-yo rolls up along the string, or
the force feedback is enough to achieve stable perfor-
mance. The proposed approach enables to play yo-yo
by measuring either the force or the yo-yo position.
Furthermore we also showed that the system has the
capability of changing the measured quantity in a sin-
gle experiment without loosing the synchronization
between the robot and the yo-yo.
REFERENCES
Buchli, J. and Ijspeert, A. (2004). A simple, adaptive loco-
motion toy-system. In Schaal, S., Ijspeert, A., Billard,
A., Vijayakumar, S., Hallam, J., and Meyer, J., editors,
From Animals to Animats 8. Proceedings of the Eighth
International Conference on the Simulation of Adap-
tive Behavior (SAB’04), pages 153–162. MIT Press.
Buchli, J., Righetti, L., and Ijspeert, A. J. (2008). Frequency
Analysis with coupled nonlinear Oscillators. Physica
D: Nonlinear Phenomena, 237:1705–1718.
Degallier, S., Righetti, L., Natale, L., Nori, F., Metta, G.,
and Ijspeert, A. (2008). A modular bio-inspired ar-
chitecture for movement generation for the infant-
like robot iCub. In Proceedings of the 2nd IEEE
RAS / EMBS International Conference on Biomedical
Robotics and Biomechatronics (BioRob).
Gams, A., Ijspeert, A. J., Schaal, S., and Lenarcic, J.
(2009). On-line learning and modulation of periodic
movements with nonlinear dynamical systems. Auton.
Robots, 27(1):3–23.
Gams, A.,
ˇ
Zlajpah, L., and Lenarˇciˇc, J. (2007). Imitating
human acceleration of a gyroscopic device. Robotica,
25(4):501–509.
Ijspeert, A. J. (2008). Central pattern generators for loco-
motion control in animals and robots: A review. Neu-
ral Networks, 21(4):642–653.
Jin, H.-L., Ye, Q., and Zacksenhouse, M. (2009). Return
maps, parameterization, and cycle-wise planning of
yo-yo playing. Trans. Rob., 25(2):438–445.
Kasuga, T. and Hashimoto, M. (2005). Human-robot hand-
shaking using neural oscillators. In ICRA, pages
3802–3807.
Matsuoka, K., Ohyama, N., Watanabe, A., and Ooshima, M.
(2005). Control of a giant swing robot using a neural
oscillator. In ICNC (2), pages 274–282.
Petriˇc, T., Gams, A., and
ˇ
Zlajpah, L. (2009). Modeling
and control strategy for robotic powerball. In RAAD,
page 7.
Righetti, L., Buchli, J., and Ijspeert, A. J. (2006). Dy-
namic hebbian learning in adaptive frequency oscil-
lators. Physica D, 216(2):269–281.
Righetti, L. and Ijspeert, A. J. (2006). Programmable Cen-
tral Pattern Generators: an application to biped loco-
motion control. In Proceedings of the 2006 IEEE In-
ternational Conference on Robotics and Automation.
Schaal, S. (1999). Is imitation learning the route to hu-
manoid robots? Trends in cognitive sciences, 6:233–
242.
Schaal, S., Mohajerian, P., and Ijspeert, A. (2007). Dy-
namics systems vs. optimal control–a unifying view.
Progress in Brain Research, 165:425–445.
Ude, A., Atkeson, C. G., and Riley, M. (2000). Planning of
joint trajectories for humanoid robots using b-spline
wavelets. In ICRA, pages 2223–2228.
ˇ
Zlajpah, L. (2006). Robotic yo-yo: modelling and control
strategies. Robotica, 24(2):211–220.
ICINCO 2010 - 7th International Conference on Informatics in Control, Automation and Robotics
38
