KNOWLEDGE ORGANIZATION IN CONCEPT MAPS

Teacher Students’ Representations of the Relatedness of Physics Concepts

Maija Nousiainen and Ismo T. Koponen

Department of Physics, University of Helsinki, Helsinki, Finland

Keywords:

Knowledge organization patterns, Concept maps, Directed networks, Learning.

Abstract:

Conceptual understanding of physics is based on understanding what the key concepts are and how they are

related. In learning and teaching connections which tie concepts to each other give direction of progress - there

is a ﬂux of information. We discuss here how such ordering of concepts can be made visible by using concept

maps and how the maps can be used in analysing the students’ views and ideas about the inherent logic of

the relatedness of concepts. The approach discussed here is informed by the recent cognitively oriented ideas

of knowledge organization concentrating on simple knowledge-organization patterns and how they form the

basis of more complex concept networks. The results show that even in well connected maps there can be

abrupt changes in the information ﬂux in the way knowledge is passed from the initial levels to the ﬁnal levels.

This suggests that handling the information content is very demanding and perhaps a very difﬁcult skill for a

teacher student to master.

1 INTRODUCTION

Good conceptual understanding is based on under-

standing what the key concepts are and how these

concepts are connected. This kind of relational struc-

ture of knowledge can be presented as a network

of concepts or as concept maps(Novak, 2002; Ruiz-

Primo and Shavelson, 1996; Liu, 2004; Koponen and

Pehkonen, 2010). Moreover, research on the structure

of the students’ concept maps suggests that a good

cocnceptual understanding is reﬂected as intercon-

nected and web-like structures (Kinchin et al., 2000;

Koponen and Pehkonen, 2010).

We concentrate here on the problem of how the

relational structure of physics concepts can be rep-

resented for purposes of making plans for teaching.

The context of making plans to teach is interesting,

because it is such a context that the relational struc-

ture of concepts must be approached from the view-

point of how to motivate and justify the introduction

of new concepts on the basis of concepts which have

already been learned. Consequently, the connections

which tie concepts to each other quite naturally pro-

vide a comprehensible direction for progress - there is

”ﬂux of information” so that what was learned before

is the basis for learning new ideas. In this study we

discuss how student teachers (in physics) represent

their views about the relatedness of physics concepts

by using specially designed concept maps, which pay

attention to the experiments and models in linking

the concepts (Koponen and Pehkonen, 2010). The

maps discussed here have been used in teacher edu-

cation as tools to represent knowledge and they are

simple enough node-link-node representation to ex-

press the most evident connections between concepts.

There exist richer representational tools, for example

the Concept Graphs (Sowa, 2008) but they would be

too elaborate for purposes of teachers.

The approach discussed here is informed by the

recent cognitively oriented ideas of knowledge or-

ganization around basic knowledge-organization pat-

terns and how they form the basis of more com-

plex concept networks (Kemp et al., 2007; Kemp and

Tenenbaum, 2008; Duong et al., 2009). The analysis

of such concept networks is then very naturally based

on the use of network theory through analysis of the

concept maps made by the students. Here six cases

(ﬁve student maps and one “master map”) are studied

from a general viewpoint (although the context is spe-

ciﬁc, namely electricity and magnetism). The analy-

sis reveals the important features of the inherent con-

nectedness, ordering and the ﬂux of information re-

lated to the progress in introducing the new concepts

in the pre-service teachers’ plans made for physics

teaching. This information is relevant for pre-service

teachers themselves as well as for the instructors.

139

Nousiainen M. and Koponen I..

KNOWLEDGE ORGANIZATION IN CONCEPT MAPS - Teacher Students’ Representations of the Relatedness of Physics Concepts.

DOI: 10.5220/0003615801390144

In Proceedings of the International Conference on Knowledge Engineering and Ontology Development (KEOD-2011), pages 139-144

ISBN: 978-989-8425-80-5

 2011 SCITEPRESS (Science and Technology Publications, Lda.)

2 THEORY

Cognitively oriented studies of knowledge formation

suggest that procedures of knowledge construction

and processing may be simple ones, reducible to ba-

sic patterns, even in those cases where the result-

ing structures are complex. Of particular importance

are different types of hierarchies, cliques, transitive

and cyclical patterns (Kemp et al., 2007; Kemp and

Tenenbaum, 2008; Duong et al., 2009). Apparently,

many aspects of knowledge can then also be repre-

sented in terms of such patterns. On this basis, we

seek here an understanding of the students’ ideas con-

cerning how physics concepts are related and how

they can be introduced in teaching.

In teaching physics the experiments and mod-

elling are two basic procedures used to introduce new

concepts or to show how they apply in different sit-

uations. It is to be expected that the relations be-

tween the concepts are then guided by the inherent

logic of constructing physics experiments and using

models to describe and explain the experimental re-

sults (Safayeni et al., 2005; Koponen and Pehkonen,

2010). The operationalising experiment is frequently

used in advanced-level physics instruction. In this

case the concept is operationalized (i.e. made mea-

surable) through pre-existing concepts. The new con-

cept C is constructed sequentially, starting from the

already existing ones A and B which provide the basis

for an experiment’s design and interpretation. In that

process, it often happens that new connection between

A and B is also established. Due to this interdepen-

dence of concept contained in this procedure, it cre-

ates the basic triangular-like pattern A → C ← B ← A

between the concepts (Safayeni et al., 2005; Koponen

and Pehkonen, 2010). The modelling procedures may

also create similar triangular patterns (Koponen and

Pehkonen, 2010).

In practice, the students use these procedures

when they link concepts and represent the relations

between the concept in form of concept maps. They

draw concept maps representing how they would pro-

ceed in introducingnewconcepts in their teaching and

in what order the concepts are introduced.

The concept maps made by the physics teacher

students represent not only the relatedness of con-

cepts, but they also represent how concepts are intro-

duced in teaching. This means that, in a sense, net-

works also represent the ”ﬂux of information” which

takes place in teaching or, rather, which teacher stu-

dents have planned to take place in their teaching. In

well-planned teaching there should naturally be a reg-

ular ﬂux of new information (in order that new knowl-

edge is learned), but no abrupt changes in that ﬂux

(otherwise there are ﬂuctuations in demandingness),

and no uncontrollable reductions in the ﬂux (which

would give a feeling that learned knowledge is not

needed in further learning). The information ﬂux is

closely related to the possibility of navigating in the

network or going from a givennode to another node in

the network. Therefore, the ordering of nodes, which

comes from the ordering of the procedures, has a cen-

tral role to play in determining the information ﬂux.

3 THE EMPIRICAL DATA

The cases studied here consist of ﬁvestudent maps, all

of which are rather rich in their structure. These maps

are typical to students, who had completed the task

with thought and had taken time to construct the maps

(altogether we have 70 maps and this feature charac-

terises about half of them). The number of the con-

cepts was limited to n=34 most central concepts and

laws of electromagnetism, but students were free to

introduce as many links as they found necessary. One

example of the designed maps is shown in Fig. 1. For

purposes of comparison and reference, we have also

constructed a ”master map”, where all well-motivated

and well-justiﬁed connections that are found in the

student maps are collated into one map.

Figure 1: An example of students’ concept maps (Gs) for

n = 34 concepts in electromagnetism. The map shows con-

cepts (boxes), laws and principles (boxes with thick bor-

ders). Links are either operationalising experiments (E) or

modelling procedures. The nodes are numbered in the order

in which they are introduced through experiments.

In order to visualise the relevant topological fea-

tures of the maps, it is useful to make the visual

appearance of the maps comparable by removing

any ambiguity associated with the graphical layout.

KEOD 2011 - International Conference on Knowledge Engineering and Ontology Development

140

This can be done by redrawing the maps so that the

same rules for ordering the nodes are used in all

cases. In graph theory this is called embedding of the

graph (Kolaczyk, 2009). For the embeddings several

well-deﬁned methods are available. The embedded

maps include the same information as do the origi-

nals (i.e. they are isomorphic representations). We

use here ”spring-embedding”, which serves the pur-

pose of revealing visually how tightly certain con-

cepts are connected, so it is suitable for visual inspec-

tion of the clustering due to triangular patterns. The

spring-embedded visualizations expose much about

the structure of the concept maps, and more is learned

of the structure through the tree embeddings. For ex-

ample, in Fig. 2 (ﬁrst row) tree embeddings are shown

when one node is chosen as a starting point. Then it

is seen just how many hierarchical levels there are in

the ordering, and how nodes in these levels can be

reached. Then again a node in the hierarchical level

is chosen, and yet another ordering is revealed, with a

new set of hierarchical levels. When repeated (shown

as rows 2 and 3 in Fig. 2), the number of nodes which

can be arranged in such a way is reduced as shown in

Fig. 2.

123

1234

1112131415161718192021

2324

123456789

101112131415161718

123456789

101112131415

Figure 2: The ”master map” (Gm, left) and one students’

map (G1, right) in spring-embedded form (ﬁrst row). In

each case, three examples are given of all 34 possible tree-

embedded forms (three rows).

The visualization provides, in principle, all neces-

sary information of the structures. However, on the

basis of visualizations alone it is still difﬁcult to get

any idea of ordering and relatedness of concepts or

how information is passed from the starting level to

the ﬁnal levels. In order to describe such features, we

need to develop suitable quantitative measures to de-

scribe the structure and information ﬂux.

4 METHOD OF ANALYSIS

The concept maps are basically node-link-node net-

works or graphs and can be analyzed as such. In a

map (network) of n nodes, the variables a

indicate

the connections between nodes i and j so that if nodes

are connected, then a

= 1 and if there is no connec-

tion, then a

= 0. These elements are the independent

variables and they form a nxn dimensional matrix, the

so-called adjacency matrix a. All quantities (depen-

dent variables) of interest can now be calculated from

the matrix a, and they are as follows (detailed mathe-

matical deﬁnitions are given in Table 1):

1. The degree k

of the node, which is the number

of the incoming and outgoing links k

and k

out

respectively. The average degree is denoted by D.

The degree describes the connectivity of the map.;

2. The clustering coefﬁcient C

, which is the ratio

of triangles to all the triply connected neighbours

around a given concept.The clustering measures

the probability that the neighbours of the given

concept are also connected i.e. it is a measure for

transitive cliques. ;

3. Flux into the nodes (Flux-I) Φ

, which gives the

total number of links terminating at the given node

k from all levels j < k. This quantity describes

how nodes k < j (i.e. concepts introduced earlier)

support the introduction of node k. In the present

case, it directly describes the ”information” ﬂow-

ing from the previously introduced nodes to ones

introduced later;

4. Flux around the nodes (Flux-A) Ψ

, which gives

the total number of links bypassing the given node

k from all levels j < k. This quantity describes

what fraction of nodes k < j (i.e. concepts intro-

duced earlier) is not used to support the introduc-

tion of node k, but which bypasses the node to

higher levels.

The above-deﬁned quantities have a meaning,

which is closely connected to the topology of the net-

work. They are also discussed extensively within the

literature of network theory, in particular the cluster-

ing C as an important measure to characterize the lo-

cal connectedness of the network (Kolaczyk, 2009).

The ﬂuxes Φ and Ψ, on the other hand, directly de-

scribes the ”information” ﬂowing from the previously

KNOWLEDGE ORGANIZATION IN CONCEPT MAPS - Teacher Students' Representations of the Relatedness of

Physics Concepts

141

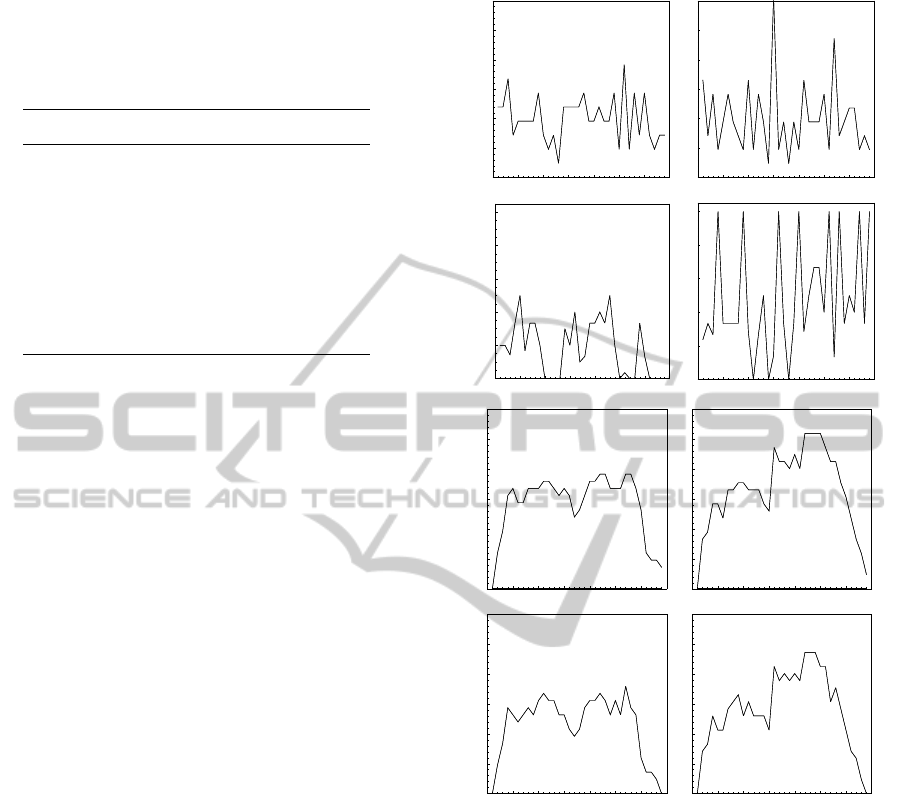
Table 1: Deﬁnitions of the quantities characterizing the

topology of the concept networks. In the deﬁnitions a

the element of the adjacency matrix a. The quantities are

deﬁned for a given node i. The average number of links per

node (average degree) is D.

Quantity Deﬁnition

∑

out

∑

+ k

out

∑

′

> j

j j

′

∑

′

> j

′



∑

i−1

j=1

out

−

∑

i−1

j=1





∑

i−1

j=1

out

−

∑

j=1



introduced nodes to ones introduced later (Karrer and

Newman, 2009) and they are therefore for our present

purposes the most important and interesting quanti-

ties.

5 RESULTS

Five cases of students’ concept maps are discussed in

terms of the structural measures and the information

ﬂuxes. The selected cases are typical, in the sense

that the features found in them can be found also in

all similar, richly connected maps. About half of the

cases appear to be these types of maps (the total sam-

ple of maps collected consists of about 70 in number).

The quantitative analysis of the maps is carried out by

using the quantities deﬁned in Table 1. These quan-

tities were constructed so that they correspond to the

properties of interest: connectivity, relative amount of

transitive triangular cliques and information ﬂuxes.

The average values of the clustering and ﬂuxes are

given in Table 2 for the maps Gm and G1 shown in

Fig. 2 and for other four maps G2-G5. These val-

ues are representative for larger class of maps (about

one half). In general, the student maps, which have

high clustering and connectedness, are all very sim-

ilar in the level of averaged values, detailed differ-

ences becoming apparent only in node-by-node anal-

ysis. On average the clustering attains values around

0.15-0.30, which is common to networks designed

for purposes of passage of information (Kolaczyk,

2009). Large values of clustering indicate that there

are appreciable connections also between concepts

connected to a given concept, i.e. an abundance of

the nearest neighbour connections. In a more tradi-

tional view of concepts maps, this means an apprecia-

ble number of cross-links and thus shortcuts within a

given level of hierarchy (Ruiz-Primo and Shavelson,

Gm, D=4.18

0.0

0.5

1.0

1.5

2.0

2.5

3.0

Number of links kD

èèèè

Gs, D=4.24

0.0

0.5

1.0

1.5

2.0

2.5

3.0

0.0

0.2

0.4

0.6

0.8

1.0

Clustering C

0.0

0.2

0.4

0.6

0.8

1.0

Flux-I  D F

Node

Flux-A  D Y

Node

Figure 3: Node-by-node (nodes 1-34) values of degree D,

clustering C, and ﬂuxes Φ and Ψ. The ﬁrst column shows

the values for master map Gm and the second column for

the student map Gs=G1.

1996; McClure et al., 1999). However, the clustering

must not be too large, because otherwise the naviga-

bility and the passing of information in the maps is

difﬁcult.

The information ﬂuxes are rather large in all stud-

ied cases. The ﬂuxes are given as a total ﬂux per

expected number of links (total ﬂux divided by av-

erage value D of links per node, see Table 1 for def-

inition. The results reveal that typically, per one link

connected to a given node, there are from three to

four links coming from the lower levels. This means

that each node is rather well supported by the many

previous nodes - the meaning content of the concept

KEOD 2011 - International Conference on Knowledge Engineering and Ontology Development

142

(node) is supported or backed up by knowledge con-

tained on the network existing before the introduc-

tion of the new node. This, quite evidently, is one

characteristic of well-planned teaching. In the sample

of 70 maps there are, however, several cases which

are nearly chain-like and very poorly connected with

D < 2 and ﬂuxes of order Ψ ≈ 1 and Φ ≈ 1. It should

be noted that not only in poorly connected maps in-

formation ﬂux is low. In a well-connected network,

though one which is poorly ordered or directed, the

ﬂuxes would be also very small and the passage of

information would be nearly hindered. In terms of

the inherent logic of how concepts are introduced, this

type of situation would indicate either: 1) circular rea-

soning, or 2) constant reference backwards.

In clustering and the ﬂuxes there are large node-

by-node variations. The large variability from node to

node indicates that there is a tendency for certain con-

cepts to gather more links than other concept would

do. A suitable quantity characterizing the relative

variation is the dispersion of the variable deﬁned as

δX = σ

/X, where X is the variable’s average value

and σ

is the standard deviation. Interestingly, the

dispersions for D, C and ﬂuxes Φ and Ψ given in Ta-

ble 2 show that in student maps there is more varia-

tion than in the ”master map”, which means that stu-

dent maps are not equally regular and balanced as the

master map. This of course is related to the fact that in

student maps there are abrupt changes in the informa-

tion ﬂuxes; some concepts become very central and

much effort goes into their introduction. This, on the

other hand, is somewhat awkward for teaching, be-

cause it means that demandingness of learning may

increase in an uncontrollable way. The master map

does not have such abrupt changes; instead, it dis-

plays a rather steady ﬂux of information throughout

the whole concept network. The results suggest that

rich concept maps not only have large values of clus-

tering and ﬂuxes but, in addition, the node-by-node

values do not vary much. This means that all con-

cepts are roughly similar in the degree of importance

for the whole structure. This, of course, is required

from well-planned teaching, where most of the topics

discussed should appear to be of importance for a stu-

dent. Maintaining small node-by-node variability is

relatively demanding, perhaps owing to the fact that it

apparently requires evaluating the functionality of the

structure as a whole instead only of locally.

6 CONCLUSIONS

We have explored physics teacher-student plans for

the teaching of physics (electricity and magnetism),

Table 2: Average degree D, clustering C and ﬂuxes Ψ and

Φ for master map Gm and student maps G1-G5. The dis-

persions δX of the variables X (see text) are also given.

Gm G1 G2 G3 G4 G5

D 4.18 4.24 3.47 3.06 3.18 3.18

δD 0.38 0.60 0.62 0.54 0.66 0.59

C 0.18 0.48 0.25 0.15 0.25 0.16

δC 0.90 0.69 1.28 1.94 1.39 1.91

Φ 2.82 3.31 3.00 2.76 2.97 3.12

δΦ 0.37 0.40 0.48 0.47 0.60 0.46

Ψ 2.32 2.81 2.51 2.26 2.47 2.62

δΨ 0.43 0.46 0.56 0.53 0.70 0.48

carried out in a physics-teacher preparation course.

The plans were represented in the form of concept

maps. The concept maps were made such that each

link between concepts needed to be justiﬁed either

through an experimental or modelling procedure, and

they were explained in a written report coming with

the map. The aspects of interest in these plans are the

relatedness of concepts, and how the ordering of the

concept allows the introductionof new concepts in the

maps. We have introduced here a new method, which

can be used to analyse the students’ plans so that their

inherent logic, ordering and the way to use the infor-

mation in the plans is revealed. These aspects, we

believe, are of importance for understanding and eval-

uating students’ view of how concepts are related and

how they can be introduced in teaching physics.

The structural analysis of the concept maps is

based on the identiﬁcation of the basic knowledge-

ordering patterns. The pattern of most importance is

a triangular pattern, connected to the procedures of

experiments and modelling. However, the analysis of

the plans for teaching show that even in cases where

the maps have a rich set of connections, and when

concepts are well connected, the inherent logic and

the way knowledge is passed from the initial levels

to the ﬁnal levels may be awkward and that there are

often abrupt changes in the information ﬂux. Inter-

estingly, when all valid connections found in the stu-

dents’ maps are combined and reorganized, the result-

ing map shows a very regular and steady information

ﬂux. This suggests that handling the information con-

tent is very demanding and perhaps one of the most

difﬁcult skills for a teacher student to master. This no-

tion has direct implications for teacher education and

it also calls for methods to monitor this kind of devel-

opment. The method of analysis introduced here is a

step in this relatively unexplored direction.

KNOWLEDGE ORGANIZATION IN CONCEPT MAPS - Teacher Students' Representations of the Relatedness of

Physics Concepts

143

ACKNOWLEDGEMENTS

This work has been supported by the Academy of Fin-

land through grant SA133369.

REFERENCES

Duong, T. H., Jo, G. S., Jung, J. J. and Nguyen, N. T.

(2009). Complexity Analysis of Ontology Integra-

tion Methodologies: A Comparative Study. Journal

of Universal Computer Science, 15, 877–897.

Karrer, B. and Newman, M. E. J. (2009). Random graph

models for directed acyclic networks. Physical Review

E, 80, 046110-1-12.

Kemp, C., Perfors, A. and Tenenbaum, J. B. (2007). Learn-

ing Overhypotheses with Hierarchical Bayesian Mod-

els. Developmental Science, 10, 307-321.

Kemp, C. and Tenenbaum, J. B. (2008). The Discovery of

Structural Form. PNAS, 105, 10687–10692.

Kinchin, I. M., Hay, D. B. and Adams, A. (2000). How a

Qualitative Approach to Concept Map Analysis Can

Be Used to Aid Learning by Illustrating Patterns of

Conceptual Development. Educational Research, 42,

43–57.

Kolaczyk, E. D. (2009). Statistical Analysis of Network

Data. New York: Springer.

Koponen, I. T. and Pehkonen M. (2010). Coherent Knowl-

edge Structures of Physics Represented as Concept

Networks in Teacher Education. Science & Educa-

tion, 19, 259–282.

Liu, X. (2004). Using Concept Mapping for Assessing and

Promoting Relational Conceptual Change in Science.

Science Education, 88, 373–396.

McClure, J. R., Sonak, B. and Suen, H. K. (1999) Concept

Map Assesment of Classroom Learning: Reliability,

Validity, and Logistical Practicality. Journal of Re-

search in Science Teaching, 36, 475–492.

Novak, J. (2002). Meaningful Learning: The Essential Fac-

tor for Conceptual Change in Limited or Inappropriate

Propositional Hierarchies Leading to Empowerment

of Learners. Science Education, 86, 548–571.

Ruiz-Primo, M. A. and Shavelson, R. J. (1996). Problems

and Issues in the Use of Concept Maps in Science As-

sessment. Journal of Research in Science Teaching,

33, 569–600.

Safayeni, F., Derbentseva, N. and Ca˜nas A. J. (2005). A

Theoretical Note on Concepts and the Need for Cyclic

Concept Maps. Journal of Research in Science Teach-

ing, 42, 741–766.

Sowa, J. F. (2008). Conceptual Graphs. In van Harmelen,

F., Lifschitz, V. and Porter, B. (Eds.), Handbook of

Knowledge Representation, (pp. 213–237), Amster-

dam:Elsevier.

KEOD 2011 - International Conference on Knowledge Engineering and Ontology Development

144