FUZZIFICATION OF THE RESOURCE-CONSTRAINED

PROJECT SCHEDULING PROBLEM

A Fight against Nature

Anikó Csébfalvi, György Csébfalvi and Sándor Danka

University of Pécs, Pécs, Hungary

Keywords: Project scheduling, Stochastic scheduling, Fuzzy scheduling, Resource-constrained project, Heuristic

algorithm, Simulation.

Abstract: In a recent article (Bhaskar et al., 2011) the authors proposed a heuristic method for the resource-

constrained project scheduling problem (RCPSP) with fuzzy activity times. The apropos of this state-of-the-

art work, we try identify and illuminate a popular misconception about fuzzification of RCPSP. The main

statement of their approach, similarly to the other fuzzy approaches, is simple: the project completion time

can be represented by a "good" fuzzy number. This statement is naturally true: in a practically axiomatic

fuzzy thinking and model building environment, using only fuzzy operators and rules, we get a fuzzy output

from the fuzzy inputs. But the real problem is deeper. The possibilistic (fuzzy) approach, traditionally,

defines itself against the probabilistic approach, so in the "orthodox" fuzzy community everything is

prohibited which is connected to somehow to the probability theory. For example, the Central Limit

Theorem (CLT) is in the taboo list of this community. We have to emphasize, CLT is a humanized

description of a miracle of nature. When we fight against CLT, we fight against nature. The situation in the

"neologist" fuzzy community is not better, because they try to redefine somehow the probability theory

within the fuzzy approach without using "forbidden" statistical terms. In this paper, we will show that the

nature is working totally independently from our "magic" abstractions. According to the robustness of CLT,

the distribution function of the completion time of real-size projects remains nearly normal, which is a

manager friendly, natural and usable result. An abstraction and its "natural" operators are unable to modify

the order of nature. When we want to add a practical scheduling method to the project managers we have to

destroy the borders between the probabilistic and possibilistic approaches and have to define a "unified"

approach to decrease the gap between scientific beliefs and reality. In this paper we present a unified

(probabilistic/possibilistic) model for RCPSP with uncertain activity durations and a concept of a heuristic

approach connected to the theoretical model. It will be shown, that the uncertainty management can be built

into any heuristic algorithm developed to solve RCPSP with deterministic activity durations. The essence

and viability of our unified model will be illustrated by a fuzzy example presented in the recent fuzzy

RCPSP literature.

1 INTRODUCTION

In a recent article (Bhaskar et al., 2011) the authors

proposed a heuristic method for the resource-

constrained project scheduling problem (RCPSP)

with fuzzy activity durations. The apropos of this

state-of-the-art work, we try identify and illuminate

a popular misconception about fuzzification of

RCPSP. The main statement of their approach,

similarly to the other fuzzy approaches, is simple:

the project completion time can be represented by a

"good" fuzzy number. This statement is naturally

true: in a practically axiomatic fuzzy thinking and

model building environment, using only fuzzy

operators and rules, we get a fuzzy output from the

fuzzy inputs. But the real problem is deeper. The

possibilistic (fuzzy) approach, traditionally, defines

itself against the probabilistic approach, so in the

"orthodox" fuzzy community everything is

prohibited which is connected to somehow to the

probability theory. For example, the Central Limit

Theorem (CLT) is in the taboo list of this

community. We have to emphasize, CLT is a

humanized description of a miracle of nature. When

286

Csébfalvi A., Csébfalvi G. and Danka S..

FUZZIFICATION OF THE RESOURCE-CONSTRAINED PROJECT SCHEDULING PROBLEM - A Fight against Nature.

DOI: 10.5220/0003644402860291

In Proceedings of the International Conference on Evolutionary Computation Theory and Applications (ECTA-2011), pages 286-291

ISBN: 978-989-8425-83-6

 2011 SCITEPRESS (Science and Technology Publications, Lda.)

we fight against CLT, we fight against nature. The

situation in the "neologist" fuzzy community is not

better, because they try to redefine somehow the

probability theory within the fuzzy approach without

using "forbidden" statistical terms. In this paper, we

will show that the nature is working totally

independently from our "magic" abstractions.

According to the robustness of CLT, the distribution

function of the completion time of real-size projects

remains nearly normal, which is a manager friendly,

natural and usable result. An abstraction and its

"natural" operators are unable to modify the order of

nature. When we want to add a practical scheduling

method to the project managers we have to destroy

the borders between the probabilistic and

possibilistic approaches and have to define a

"unified" approach to decrease the gap between

scientific beliefs and reality. In this paper we present

a new unified (probabilistic/possibilistic) model and

a conception of a heuristic connected to the unified

model for RCPSP with uncertain activity durations.

In Section 2 we present a unified theoretical model.

In Section 3 we describe the conception of the

uncertainty management according to the theoretical

model. The essence and viability of our unified

model will be illustrated by a fuzzy example in

Section 4. Finally, Section 5 draws conclusions from

this study.

2 THEORETICAL MODEL

In this section we describe the theoretical model for

RCPSP with uncertain activity durations. The

approach produces “robust” schedules which are

immune against uncertainties in the activity

durations. The optimality criterion is defined as a

linear combination (weighted sum) of resource-

feasible makespans connected to the key terms of

the applied uncertainty formulation. Theoretically

the optimal robust schedule searching process is

formulated as a multi-objective mixed integer linear

programming problem (MOMILP) where the

number of objectives corresponds to the number of

key terms (parameters) of uncertainty formulation.

In this paper, we replaced the MOMILP with a

MILP by scalarization. The resulting MILP can be

solved directly in the case of small-scale projects

within reasonable time. The proposed model is

based on the so-called “forbidden set” concept. The

output of the model is the set of the optimal conflict

repairing relations. Obviously, the solution of the

problem depends on the choice of the weights for the

objective functions.

In order to model uncertain activity durations in

projects, we consider the following resource-

constrained project-scheduling problem: A single

project consists of

N real activities

{}

Ni 2 1 ,...,,∈ .

In this paper, without loss of generality, we

assume that each activity duration can be described

by three parameters:

{

}

221 iii

DDD ,, ,

{}

Ni 2 1 ,...,,∈ ,

where triplet

{

}

221 iii

DDD ,, may define a triangular

membership function in the possibilistic approach,

or a density function from beta distribution in the

probabilistic approach. We have to note, that in the

probabilistic approach the triplet is estimated from a

sample using standard statistical tools, assuming that

the future can be described from the past, but in the

possibilistic approach it is only an abstraction which

describe the future according to knowledge of the

project managers.

The fuzzy community, under the spell of the

challenging but manageable nature of the

membership function (it is non-smooth composite of

linear segments) tries to recreate everything from the

beginning. For example, "normalization" is a "coded

message" that the triangle is not a density function,

and the horizontal line corresponding to "α-cut" is a

theoretically questionable replacement of the two

vertical lines, which define the confidence interval

in the probabilistic approach. Changing the position

of α we change our risk-taking habit, but, at the

same time, we omit/add duration segments with

totally different left/right tail probability (Figure 1).

Our opinion about the uncertainty management

in project scheduling is very simple: we have to

replace the triangular membership function with the

equivalent triangular density function, have to let the

CLP to work. Formalisms which in the uncertainty

dimension, try to redefine statistical terms without

statistical terms, are meaningless and misleading.

The activities are interrelated by precedence

constraints: Precedence constraints force an activity

not to be started before all its predecessors are

finished. These are given by network-relations

ji → , where ji → means that activity j cannot

start before activity

i is completed. Furthermore,

activity

(

)

1 0

Nii is defined to be the unique

dummy source (sink). Let

NR be the set of the

network relations.

FUZZIFICATION OF THE RESOURCE-CONSTRAINED PROJECT SCHEDULING PROBLEM - A Fight against

Nature

287

1 i

2 i

3 i

1 i

2 i

3 i

1 3

DD −

Possibilistic Approach

Probabilistic Approach

50.=

01.=

Figure 1: Possibilistic and probabilistic approaches.

Let

denote the number of renewable

resources required for carrying out the project. Each

resource

{}

Rr ,...,1∈ has a constant per period

availability

R . In order to be processed, each real

activity

{}

Ni ...,2, 1, ∈ requires 0≥

i r

R units of

resource

{}

R , ... 1,∈r over its duration.

A schedule is network-feasible if satisfies the

predecessor-successor relations:

jii

SDS ≤+ , for each

NRji ∈→

(1)

Let

ℜ denote the set of network-feasible

schedules. For a network feasible schedule

ℜ

⊂S ,

let

{

}

{}

TDStSiA

iiit

,...,1 t ∈+<≤= , denote the

set of active (working) activities in period

and let

∑

∈

r r

{}

Tt ,...,1∈ ,

{}

Rr ,...,1∈

(2)

be the amount of resource

used in period

, where

T is an upper bound of the resource-feasible

makespan.

A network-feasible schedule

ℜ⊂S is resource-

feasible if satisfies the resource constraints:

RU ≤

{}

Tt ,...,1∈ ,

{}

Rr ,...,1∈

(3)

Let

ℜ⊆ℜ denote the set of resource-feasible

schedules. The presented unified MILP formulation

is based on the forbidden set concept.

In MILP model the total number of zero-one

variables is

RR , and the formulation is based on

well-known "big-M" constraints. The presented

MILP model is a modified and simplified version of

the original forbidden set oriented model developed

by Alvarez-Valdés and Tamarit for the deterministic

case.

A forbidden activity set is identified such that:

(1) all activities in the set may be executed

concurrently, (2) the usage of some resource by

these activities exceeds the resource availability, and

(3) the set does not contain another forbidden set as

a proper subset. A resource conflict can be repaired

explicitly by inserting a network feasible precedence

relation between two forbidden set members, which

will guarantee that not all members of the forbidden

set can be executed concurrently. We note, that an

inserted explicit conflict repairing relation (as its

side effect) may be able to repair one or more other

conflicts implicitly, at the same time.

Let

∑

, which is an “extremely weak”

resource-feasible upper bound and fix the position of

the unique dummy sink in period

. Naturally,

this “weak” upper bound can be replaced by any

“stronger” one.

Let

1ii

{

}

Ni ...,2, 1,

∈

, and let

denote

the number of forbidden sets and let

RR denote the

set of explicit repairing relations for forbidden set

F ,

{

}

Ff ,...,,21

∈

according to the "optimistic"

durations and resource-feasible upper-bound

Let

{}

⎭

⎬

⎫

⎩

⎨

⎧

∈=

∪

FfRRRR ,...,,21

(4)

denote the set of all the possible repairing relations.

In the forbidden set oriented model, a resource-

feasible schedule is represented by the set of the

inserted resource conflict repairing relations

(Alvarez-Valdés and Tamarit, 1993). According to

the implicit resource constraint handling, in this

model the resource-feasibility is not affected by the

feasible activity shifts (movements).

In the time oriented model, a resource-feasible

schedule is represented by the activity starting times.

In this model, according to the explicit resource

constraint handling, an activity movement may be

able to destroy the resource-feasibility.

It is very important to note, that after inserting an

appropriate conflict repairing set, the "immunised"

schedule will invariant to the duration change. In

other words, the schedule will be resource-feasible

on the set of the possible (and allowed) activity

durations because we immunised it according to the

optimistic (shorter) durations:

ECTA 2011 - International Conference on Evolutionary Computation Theory and Applications

288

[]

31 iii

DDD ,= ,

{}

Ni ...,2, 1,

∈

(5)

Let

S denote the starting time of activity i ,

where

{}

1 2 1 0

∈ Ni ,...,,, and

{}

321 ,,∈p . By

definition, in the optimistic, most likely, and

pessimistic schedules the durations are optimistic,

most likely, and pessimistic durations:

pii

DD =

{}

Ni 2 1 ,...,,∈ ,

{}

321 ,,∈p

(6)

Defining the binary decision variables:

RRj i

j i

∈→

⎩

⎨

⎧

→

= ,

otherwise0

inserted if1

(7)

the following MILP model arises:

min→∗

∑

(8)

,≥

∑

∈→

RRji

{}

1 Ff ,...,

∈

(9)

(

)

()

ijip

jpipip

YDSSSDS −∗+−+≤+ 1

RRji ∈→

{}

321 ,,∈p

(10)

jpipip

SDS ≤+ , NRji

∈

→ ,

{}

321 ,,∈p

(11)

The objective function (8) minimizes the linear

combination of the resource-constrained makespans,

where the weights characterize risk-taking habit of

the project manager (for example: "best pessimistic"

may be a good scheduling policy, when the project

manager is a risk-avoider).

Constraint set (9) assures the resource feasibility

(we have to repair each resource conflict explicitly

or implicitly, therefore from each conflict repairing

set we have to choose at least one element).

Constraint sets (10) take into consideration the

precedence relations between activities in the

function of the inserted repairing relations.

Constraint sets (11) take into consideration the

original precedence (network) relations between

activities.

In the "big-M" formulation

(

)

SS define the

earliest (latest) starting time of activity

i , in the

optimistic schedule according to upper-bound

T .

We have to note again, that the optimal solution

is a function of

W ,

{

}

321 ,,∈p weights. According

to the model construction in the optimal schedule

every possible activity movement is resource

feasible, and schedule is “robust” because it is

invariant to the variability of activity durations. In

other words, a non-critical activity movement (a

non-critical delay) or longer (but possible) activity

duration is unable to destroy the resource feasibility

of the schedule.

3 HEURISTIC ALGORITHM

In this section, describe the conception of a heuristic

algorithm connected to the presented theoretical

model, Without loss of generality, we assume that

we have a deterministic list scheduling algorithm

width forward-backward improvement (FBI) to

produce resource-feasible schedules in a arbitrary

metaheuristic frame. According to the essence of the

algorithm, we generate the resource-feasible

schedules by taking the selected activities one by

one in the given activity order and scheduling them

at the earliest (latest) feasible start time using the

optimistic activity durations. After that, using FBI

we try to improve the quality of the generated

schedule.

When the algorithm, in the forward-backward

list scheduling process, inserts a precedence relation

between an already scheduled activity and the

currently scheduled activity whenever they are

connected without lag, than we get a schedule

without "visible" resource-conflicts in which,

according to applied "thumb rule", the number of

"hidden" conflicts is drastically decreased.

The importance of the "thumb rule" may be

explained by the fact, that in this way we are able to

resolve resource conflicts, without explicit forbidden

set computation. After that, the algorithm is able (in

exactly one step) to repair all of the hidden

(invisible) conflicts, inserting always the “best”

conflict repairing relation for each forbidden set.

In this context “best” means a relation between

two forbidden set members for which the lag is

maximal. Naturally, the algorithm memorizes the

best schedule found so far by computing the

durations of the schedule according to the key point

durations. In the search process, according to the

"from optimistic to pessimistic" strategy, the

algorithm resolves the visible (hidden) resource

conflicts using the optimistic durations, after that

replaces the optimistic durations with the most

likely, and pessimistic ones. The algorithm exploits

the fact, that we can not destroy the resource-

feasibility, replacing the optimistic durations in a

conflict free optimistic solution with longer

durations.

After the "best conflict repairing combination"

searching phase, the makespan distribution function

is generated by simulation. In the simulation phase

we have to replace the membership functions with

FUZZIFICATION OF THE RESOURCE-CONSTRAINED PROJECT SCHEDULING PROBLEM - A Fight against

Nature

289

the appropriate density functions (for example: we

replace a triangular membership function with a

triangular density function and use a triangular

random number generator to get duration instances).

4 EXAMPLE

The algorithm of the proposed approach has been

programmed in Compaq Visual Fortran 6.5. To

solve the presented problem to optimality the

callable version of Cplex 12.2 was used. The

computational results were obtained by running the

algorithm on a 1.8 GHz Pentium IV IBM PC under

Microsoft Windows XP

operating system. The

conception of Section 3 was inserted to the "Sounds

of Silence" harmony search metaheuristic frame

(Csébfalvi et al., 2008).

The fuzzy example was borrowed from Bhaskar,

Pal, and Pal. The project is shown in Table 1 and

Figure 2. The project has only one renewable

resource type. The "weak" upper-bound is

397

T .

Table 1: A fuzzy RCPSP.

Activity Duration

Resource

requirement

1 {42, 50, 61} 8

2 {36, 40, 42} 17

3 {35, 50, 79} 12

4 {39, 50, 59} 3

5 {16, 25, 30} 13

6 {43, 51, 57} 17

7 {52, 58, 69} 16

Resource availability 30

According to the presented fuzzy RCPSP

algorithm, which is based on a "distance base

ranking of fuzzy numbers" method, the "good"

schedule obtained by the heuristic is:

{}

278 249 212 ,, .

100

150

176

50 100 150 176

Figure 2: A fuzzy RCPSP with optimistic durations.

Because the project is extremely small, we can

prove by explicit enumeration, that this result is

wrong. According to

397=T setting and using the

optimistic duration estimations, the problem has

only two forbidden sets (see Table 2). The implicit

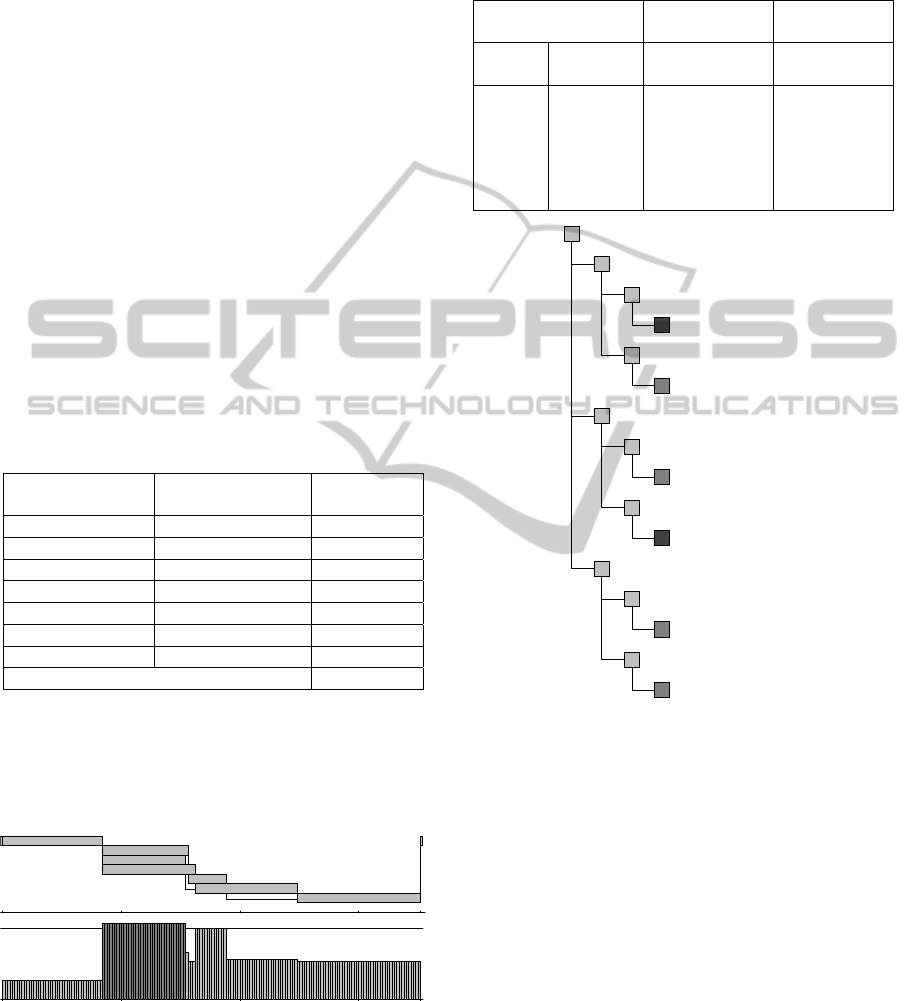
enumeration tree is presented in Figure 3.

Table 2: Forbidden sets and repairing relations.

Forbidden sets

Explicit

repairs

Implicit

repairs

1 {2,6}

→6

→2

→3

→4

2 {2,3,4}

→3

→2

→4

→2

→4

→3

→2

{ , , }

{208, , }

{208, 249, }

{208, 249, 308}

{208, , 308}

{208, 249, 308}

{ , 249, }

{208, 249, }

{208, 249, 308}

{ , 249, 288}

{212, 249, 288}

{ , , 288}

{212, , 288}

{212, 249, 288}

{ , 249, 288}

{212, 249, 288}

Figure 3: Explicit enumeration tree.

The problem has two non-dominated solutions:

{

}

308 249 208 ,, and

{

}

288 249 212 ,, , which illustrate

the fact, that a good optimistic schedule not

necessarily will be a good pessimistic one and vice

versa. The presented "good" solution from (Bhaskar

et al., 2011) is better then a non-dominated solution,

which is impossible.

When we apply the model of Section 2 to the

presented fuzzy problem with unit weights, we get

{

}

288 249 212 ,, as optimal solution within 0.05 sec.

In this case, the optimal resource conflict repairing

relations are:

62 → and 24 → . The optimistic

optimal solution is presented in Figure 4.

ECTA 2011 - International Conference on Evolutionary Computation Theory and Applications

290

100

150

200

212

50 100 150 200 212

Figure 4: Optimal solution with optimistic durations.

The problem is really simple. The applied

harmony search metaheuristic reached the optimal

solution in the random repertoire uploading phase

setting the repertoire size to ten.

After the "best conflict repairing combination"

searching phase, the makespan distribution function

is generated by simulation. In the simulation phase

we replaced the membership functions with density

functions (in this case we replaced the triangular

membership functions with a triangular density

functions and used a triangular random number

generator to get duration instances). We have to

mention it, that simulation is a cheap operation, so

the sample size may be large enough. In the

presented example we set the sample size to ten

thousand. Using the Kolmogorov-Smirnov test, we

can not reject a null hypothesis that the sample

comes from a normal distribution with the following

parameters:

10.0 256.4, 0.158, ===

(12)

where

1580

is the probability of the largest

difference (in absolute value) between the observed

and theoretical distribution functions when the null

hypothesis is true with mean

4256.=

and standard

deviation

010

The histogram in Figure 5 reviles the fact, that

the nature knows nothing about the fuzzification and

does its best according to the CLP.

212 249 288256

Figure 5: Makespan estimation by simulation.

5 CONCLUSIONS

In this paper, a new unified theoretical model and a

concept of the corresponding heuristic approach to

solve RCPSP with uncertain activity durations were

presented. In the proposed heuristic approach, the

uncertainty management is invariant to the applied

heuristic frame; therefore it can be built into any

other heuristic developed to solve RCPSPs. The

essence and viability of our unified approach was

illustrated by a fuzzy example presented in the

recent fuzzy RCPSP literature. A fast and effective

metaheuristic algorithm for large problems is under

development and will be presented in a forthcoming

paper.

REFERENCES

Alvarez-Valdés, R., Tamarit, J. M., 1993. The project

scheduling polyhedron: Dimension, facets and lifting

theorems, Journal of Operational Research, 96, 204-

220.

Bhaskar, T., Pal, M. N., Pal, A. K., 2011. A heuristic

method for RCPSP with fuzzy activity times,

European Journal of Operational Research, 208, 57-

66.

Csébfalvi, G., Csébfalvi, A., Szendrői, E., 2008. A

harmony search metaheuristic for the resource-

constrained project scheduling problem and its multi-

mode version, In Proceedings of the Eleventh

International Workshop on Project Management and

Scheduling, Istanbul, 56–59.

FUZZIFICATION OF THE RESOURCE-CONSTRAINED PROJECT SCHEDULING PROBLEM - A Fight against

Nature

291