A TRACTABLE FORMALISM FOR COMBINING RECTANGULAR

CARDINAL RELATIONS WITH METRIC CONSTRAINTS

Angelo Montanari

, Isabel Navarrete

, Guido Sciavicco

and Alberto Tonon

Department of Mathematics and Computer Science, University of Udine, Udine, Italy

Department of Information Engineering, University of Murcia, Murcia, Spain

Keywords:

Qualitative spatial reasoning, Quantitative spatial reasoning, Cardinal direction relations, Constraint satisfac-

tion problems.

Abstract:

Knowledge representation and reasoning in real-world applications often require to integrate multiple aspects

of space. In this paper, we focus our attention on the so-called Rectangular Cardinal Direction calculus for

qualitative spatial reasoning on cardinal relations between rectangles whose sides are aligned to the axes of the

plane. We ﬁrst show how to extend a tractable fragment of such a calculus with metric constraints preserving

tractability. Then, we illustrate how the resulting formalism makes it possible to represent available knowledge

on directional relations between rectangles and to derive additional information about them, as well as to

deal with metric constraints on the height/width of a rectangle or on the vertical/horizontal distance between

rectangles.

1 INTRODUCTION

Qualitative spatial representation and reasoning play

an important role in various areas of computer sci-

ence such as, for instance, geographic information

systems, spatial databases, document analysis, lay-

out design, and image retrieval. Different aspects of

space, such as direction, topology, size, and distance,

which must be dealt with in a coherent way in many

real-world applications, have been modeled by differ-

ent formal systems (Broxvall, 2002; Condotta, 2000;

Gerevini and Renz, 2002; Liu et al., 2009) (see (Cohn

and Hazarika, 2001) for a survey). For practical rea-

sons, a bidimensional space is commonly assumed,

and spatial entities are represented by points, boxes,

or polygons with a variety of shapes, depending on

the required level of detail.

Information about spatial conﬁgurations is usually

speciﬁed by constraint networks describing the al-

lowed binary relations between pairs of spatial vari-

ables. The central problem in qualitative reasoning is

consistency checking, which is the problem of decid-

ing whether or not a network has a solution, that is,

the problem of establishing whether or not there ex-

ists an assignment of domain values to variables that

satisﬁes all constraints.

Cardinal relations are directional relationships

that allow one to specify how spatial objects are

placed relative to one another either by making use

of a ﬁxed reference system, e.g., to say that an object

is to the “north” or “southwest” of another one in a ge-

ographic space, or, alternatively, by exploiting direc-

tions as “above” or “below and left” in a local space.

Cardinal relations are of particular interest for geo-

graphic information systems, spatial databases, and

image databases (Frank, 1996; Goyal, 2000; Papadias

and Theodoridis, 1997; Skiadopoulos et al., 2005).

The most expressive formalism with cardinal rela-

tions between extended spatial objects is the Cardi-

nal Direction calculus, CD-calculus for short (Goyal

and Egenhofer, 2000; Liu et al., 2010; Skiadopou-

los and Koubarakis, 2005). The consistency problem

for the CD-calculus is NP-complete, and no tractable

fragment of it has been identiﬁed so far, with the

only exceptionof the fragment obtained by forbidding

disjunctive relations (Skiadopoulos and Koubarakis,

2005). Such a restriction is a serious limitation when

we have to deal with incomplete or indeﬁnite infor-

mation in spatial applications.

In (Navarrete and Sciavicco, 2006), the au-

thors introduce a restricted version of the CD-

calculus called Rectangular Cardinal Direction cal-

culus (RCD-calculus), where cardinal relations are

deﬁned only between rectangles whose sides are par-

allel to the axes of the Euclidean plane. Rectangles

of this type (boxes) can be seen as minimum bound-

154

Montanari A., Navarrete I., Sciavicco G. and Tonon A..

A TRACTABLE FORMALISM FOR COMBINING RECTANGULAR CARDINAL RELATIONS WITH METRIC CONSTRAINTS.

DOI: 10.5220/0003747901540163

In Proceedings of the 4th International Conference on Agents and Artiﬁcial Intelligence (ICAART-2012), pages 154-163

ISBN: 978-989-8425-95-9

 2012 SCITEPRESS (Science and Technology Publications, Lda.)

ing rectangles (MBRs) that enclose plane regions (the

actual spatial objects). MBRs have been widely used

in spatial databases (El-Geresy and Abdelmoty, 2001;

Papadias and Theodoridis, 1997), in web-document

analysis (Gatterbauer and Bohunsky, 2006), and in

2D-layout design, e.g., in architecture (Baykan and

Fox, 1997). On the one hand, approximating regions

by rectangles implies a loss of accuracy in the rep-

resentation of the relative direction between regions;

on the other hand, reasoning tasks become more efﬁ-

cient.

The RCD-calculus has a strong connection with the

Rectangle Algebra (RA) (Balbiani et al., 1998), which

can be viewed as a bidimensional extension of Inter-

val Algebra (IA), the well-knowntemporal formalism

for dealing with qualitative binary relations between

time intervals (Allen, 1983). A tractable fragment

of the RCD-calculus, named convex RCD-calculus,

has been identiﬁed by Navarrete et al. in (Navarrete

et al., 2011). It includes all basic relations and a large

number of disjunctive relations, making it possible to

represent and reason about indeﬁnite information ef-

ﬁciently.

This paper aims at adding metric features to for-

malisms for qualitative spatial reasoning. Metric con-

straints between points over a dense linear order have

been dealt with by the Temporal Constraint Satis-

faction Problem formalism (TCSP) (Dechter et al.,

1991). In such a formalism, one can constrain the dis-

tance between a pair of points to belong to a given set

of intervals. If each constraint consists of one inter-

val only, we get a tractable fragment of TCSP, called

Simple Temporal Problem formalism (STP).

In the following, we propose a metric extension to

the convex RCD-calculus that allows one to repre-

sent available knowledge on directional relations be-

tween rectangles and to derive additional informa-

tion about them, as well as to deal with metric con-

straints on the height/width of a rectangle or on the

vertical/horizontal distance between rectangles. We

will show that the resulting formalism is expressive

enough to capture various scenarios of practical inter-

est and still computationally affordable.

The rest of the paper is organized as follows.

In Section 2, we provide background knowledge on

qualitative calculi and we shortly recall Interval Al-

gebra and Rectangle Algebra. In Section 3, we intro-

duce RCD-calculus and its convex fragment. In Sec-

tion 4, we extend the convex RCD-calculus with met-

ric constraints, and we devise a sound and complete

polynomial algorithm for consistency checking. We

conclude the section with a simple application exam-

ple. Conclusions provide an assessment of the work

and outline future research directions.

Figure 1: Basic relations of the Interval Algebra.

2 PRELIMINARIES

In this section, we introduce basic notions and termi-

nology.

Temporal knowledge, as well as spatial knowl-

edge, is commonly represented in a qualitative cal-

culus by means of a qualitative network consisting

of a complete constraint-labeled digraph N = (V,C),

where V = {v

, . . . , v

} is a ﬁnite set of variables, in-

terpreted over an inﬁnite domain D, and the labeled

edges in C specify the constraints describing qual-

itative spatial or temporal conﬁgurations. An edge

from v

to v

labeled with R corresponds to the con-

straint v

, where R denotes a binary relation over

D which restricts the possible values for the pair of

variables (v

, v

). The full set of relations of the cal-

culus is usually taken as the powerset 2

, where B

is a ﬁnite set of binary basic relations that forms a

partition of D × D. Thus, a relation R

∈ 2

is of

the form R = {r

, . . . , r

}, where each r

is a basic

relation, and R represents the union of the basic rela-

tions it contains. If m = 1, we call R a basic relation;

otherwise, we call it a disjunctive relation. A special

case of disjunctive relation is the universal relation,

denoted by ‘?’, which contains all the basic relations.

A basic constraint v

{r}v

expresses deﬁnite knowl-

edge about the values that the two variables v

, v

can

take, while a disjunctive constraint v

, . . . , r

expresses indeﬁnite or imprecise knowledge about

these values. In particular, the universal constraint

states that the relation between v

an v

is to-

tally unknown. From a logical point of view, a dis-

junctive constraint v

, . . . , r

can be viewed as

the logical disjunction v

} v

∨ ·· · ∨ v

} v

An instantiation (or interpretation) of the con-

straints of a qualitative network N is a mapping ι rep-

resenting an assignment of domain values to the vari-

ables of N. A constraint v

is said to be satisﬁed

by an instantiation ι if the pair (ι(v

), ι(v

)) belongs

to the binary relation represented by R. A consistent

A TRACTABLE FORMALISM FOR COMBINING RECTANGULAR CARDINAL RELATIONS WITH METRIC

CONSTRAINTS

155

instantiation, or solution, of a network is an assign-

ment of domain values to variables satisfying all the

constraints. If such a solution exists, then the network

is consistent, otherwise it is inconsistent.

The main reasoning task in qualitative reasoning

is consistency checking, which amounts to deciding if

a network is consistent. If all relations are considered,

consistency checking is usually NP-hard. Hence,

ﬁnding subsets of 2

for which consistency check-

ing turns out to be polynomial (tractable subsets) is

an important issue to address. Another common task

in qualitative reasoning is computing the unique mini-

mal network equivalentto a given one by determining,

for each pair of variables, the strongest relation (min-

imal relation) entailed by the constraints of the net-

work. It can be easily shown that each basic relation

in a minimal network is feasible, i.e., it participates in

some solution of the network.

To deal with these tasks, constraint propagation

techniques are usually exploited. The most promi-

nent method for constraint propagation is the path-

consistency algorithm, PC-algorithm for short (Mack-

worth, 1977). Such an algorithm reﬁnes relations

by successively applying the operation R

← R

∩

◦ R

) for every triple of variables (v

, v

), un-

til a stable network is reached, where R

, R

are the relations constraining the pair of variables

, v

), (v

, v

), (v

, v

), respectively (◦ stands for the

composition of relations). If the empty relation is ob-

tained during the process, then the input network is in-

consistent; otherwise, we can conclude that the output

network is path consistent, which does not necessarily

imply that it is consistent. In some special cases, the

PC-algorithm can be used to decide the consistency

of a qualitative network and to get the minimal one.

2.1 Interval Algebra and Point Algebra

Allen’s Interval Algebra (IA) allows one to model

the relative position of two temporal intervals (Allen,

1983). An interval I is usually interpreted as a closed

interval over the rational numbers [I

−

, I

], whose

endpoints I

−

and I

satisfy the relation I

−

< I

. Let

be the set of the thirteen basic interval relations

capturing all possible ways to order the four end-

points of two intervals, usually denoted by the sym-

bols b, o, d, m, s, f, e,bi, oi, di,mi, si, and fi. The se-

mantics of basic IA-relations is deﬁned in terms of

ordering relations between the endpoints of the inter-

vals, as shown in Figure 1. Notice that, given a basic

relation r between two intervals I and J, the inverse

relation ri is deﬁned by simply exchanging the roles

of I and J (see Figure 1). IA can be viewed as a con-

straint algebra deﬁned by the power set 2

and the

operations of intersection, inverse (

−1

), and composi-

tion (◦) of relations.

IA subsumes Point Algebra, PA for short (Vi-

lain and Kautz, 1986), a simpler qualitative calculus

whose binary relations specify the relative position of

pairs of time points. PA binary relations are <, >, =

(basic) and ≤, ≥, 6=, ? (disjunctive), plus the empty re-

lation. The endpoint relations deﬁning an IA-relation

(Figure 1) are basic relations of PA.

2.2 Rectangle Algebra

Rectangle Algebra (RA), proposed by Balbiani et

al. (1998), is an extension of IA to a bidimensional

space

. We assume here the domain of RA to consist

of the set of rational rectangles whose sides are paral-

lel to the axes of the Euclidean plane. To avoid a no-

tational overload, with an abuse of notation, hereafter

we will denote by a, b both rectangles in the domain

of RA and constraint (rectangle) variables. A rectan-

gle a is completely characterized by a pair of intervals

, a

), where a

and a

are the projections of a onto

the x- and y-axis, respectively. We call B

the set of

basic relations of RA, which is obtained by consid-

ering all possible pairs of basic IA-relations. Hence,

a basic RA-relation r is denoted by a pair r = (t, t

′

)

of basic IA-relations, representing the set of pairs of

rectangles (a, b) such that a(t,t

′

)b holds if and only

if, by deﬁnition, a

t b

and a

′

hold. Given a basic

RA-relation r = (t, t

′

), let t = π

(r) and t

′

= π

(r) be

the x- and y-projection of r, respectively.

Example 1. Figure 2 shows a spatial realization of

the basic RA-constraint a{(o, bi)}b. We have that

(o, bi) = o, π

(o, bi) = bi, a

overlaps b

, and a

after b

. The left endpoints of the intervals assigned

to a

and a

(1 and 5.9, respectively) and their right

endpoints (4.6 and 8, respectively) are the coordinates

of the lower-left and upper-right vertices of the given

instantiation of a, respectively. The same for b. Thus,

the values assigned to the endpoints of the projections

of a and b represent an assignment for a and b that

satisﬁes the constraint a{(o, bi)}b.

In the case of an arbitrary RA-relation R ∈ 2

the projections of R are deﬁned as follows:

(R) = {π

(r) | r ∈ R} π

(R) = {π

(r) | r ∈ R}.

Notice that, in general, π

(R) × π

(R) may be differ-

ent from R or, equivalently, we may have π

) =

) and π

) = π

) for some R

6= R

The mappings π

and π

can be generalized to

RA-networks. We deﬁne the projections π

and π

An extension of RA to n-dimensional spaces can be

found in (Balbiani et al., 2002).

ICAART 2012 - International Conference on Agents and Artificial Intelligence

156

4.6

6.7

1.5

5.9

Figure 2: An instantiation of the RA-constraint a{(o, bi)}b.

The corresponding RCD-relation is a{NW:N}b

of an RA-network N = (V,C) as the two IA-networks

(N) = (V

) and π

(N) = (V

), where V

are the sets of interval variables corresponding to the

rectangle variables in V and the set of IA-constrains

(resp., C

) is obtained by replacing each relation

in C by π

) (resp., by π

)).

2.3 Convex Subalgebras

The consistency problem for both IA and RA is

known to be NP-complete. Several tractable frag-

ments of both calculi have been identiﬁed in the liter-

ature. In this paper, we focus our attention on convex

tractable subsets of IA (van Beek and Cohen, 1990)

and RA (Balbiani et al., 1998), which consist of the

set of convex IA-relations and convex RA-relations,

respectively. Convex relations are those relations that

can be equivalently expressed as a set of convex PA-

constraints (all PA-relations except 6= are allowed)

between the endpoints of interval variables (convex

IA-relations) or between the endpoints of the projec-

tions of rectangle variables (convex RA-relations) It is

worth to mention that a convex RA-relation is equiv-

alently characterized as a RA-relation which can be

obtained as the Cartesian product of two convex IA-

relations. A PC-algorithm can be used to solve both

the consistency and the minimality problems in the

convex fragments of PA, IA, and RA in O(n

), where

n in the number of variables of the input network.

3 RECTANGULAR CARDINAL

DIRECTION CALCULUS

The Rectangular Cardinal Direction calculus (RCD-

calculus, for short) (Navarrete and Sciavicco, 2006;

Navarrete et al., 2011) deals with cardinal direction

relations between rectangles. Hence, its domain is

the same as that of RA. Let b be a reference rect-

angle. We denote by b

−

and b

(resp., b

−

and b

)

the left and the right endpoint of the projection of

b onto the x-axis (resp., y-axis), respectively. The

N W (b)

N (b)

N E(b)

W (b)

B(b)

E(b)

SE(b)

S(b)

SW (b)

−

M BR(b)

M BR(a)

(a) (b)

Figure 3: (a) Cardinal tiles with respect to rectangle b. (b) A

possible instantiation of the RCD-constraint aB:N:NE:E b.

straight lines x = b

−

, x = b

, y = b

−

, y = b

di-

vide the plane into nine tiles τ

(b), with 1 ≤ i ≤ 9, as

shown in Figure 3-(a), where τ

is a tile symbol from

the set TS = {B, S, SW,W, NW,N, NE, E, SE}, denot-

ing the cardinal directions in the Bounds of, to the

South of, to the SouthWest of, to the West of, to the

NorthWest of, to the North of, to the NorthEast of, to

the East of, and to the SouthEast of, respectively.

Deﬁnition 1. A basic rectangular cardinal relation

(basic RCD-relation) is denoted by a tile string

:τ

:. . . :τ

, where τ

∈ TS, for 1 ≤ i ≤ k, such that

aτ

:τ

:. . . :τ

b holds iff for all τ

∈ {τ

, τ

, . . . , τ

◦

∩ τ

(b) 6= ∅, and for all τ

∈ TS \ {τ

, τ

, . . . , τ

◦

∩ τ

(b) = ∅, where a

◦

is the interior of a. A rectan-

gular cardinal relation (RCD-relation) is represented

by a set R = {r

, . . . , r

}, where each r

is a basic

RCD-relation.

As usual, if R is a singleton, then it is a basic RCD-

relation; otherwise, it is a disjunctive one.

The set B

rcd

of basic RCD-relations consists of 36

elements (see Figure 4). Qualitative networks with

labels in 2

rcd

, as well as the consistency problem for

such networks, are deﬁned in the standard way.

The RCD-calculus can be viewed as a restricted

version of the CD-calculus over the domain of reg-

ular regions (Goyal and Egenhofer, 2000; Liu et al.,

2010; Skiadopoulos and Koubarakis, 2005), which

includes all rectangles aligned to the axes. Let a, b

denote regions. A cardinal relation is deﬁned by con-

sidering the exact shape of a primary region a and

the minimum bounding rectangle (MBR) of the refer-

ence region b, where MBR(b) is the smallest rectangle

aligned to the axes of the plane that encloses b. There

are 218 CD-relations over connected regions, that be-

come 512 if we allow disconnected regions. Cardinal

relationships between regions may be approximated

by RCD-relations between their MBRs, with a possi-

ble loss of accuracy when the regions are non-convex

or diagonal. The advantage of the RCD-calculus over

the CD-calculus is its simplicity (only 36 basic rela-

A TRACTABLE FORMALISM FOR COMBINING RECTANGULAR CARDINAL RELATIONS WITH METRIC

CONSTRAINTS

157

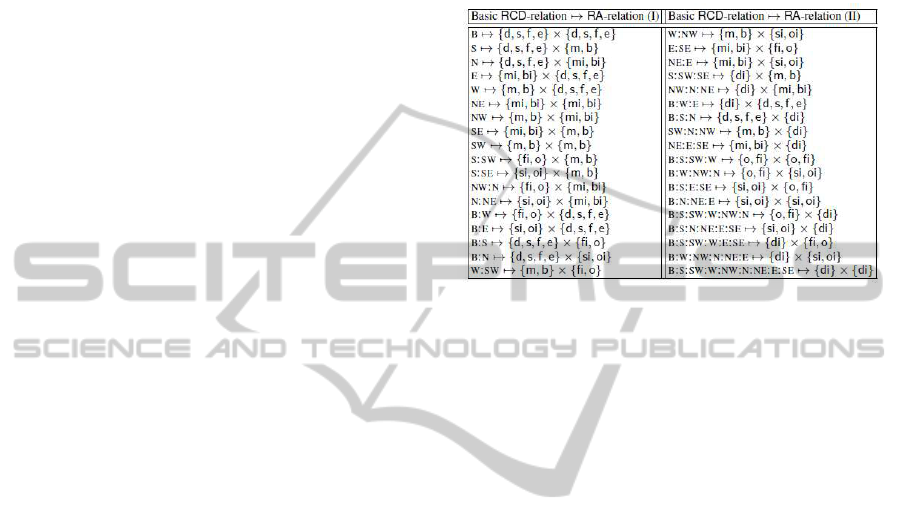
tions), which leads to a better computationalbehavior,

also when disjunctive relations are considered.

Example 2. Figure 3-(b) shows a possible instan-

tiation of the CD-constraint aB:N:E b. We in-

deed have that a lies partly in the bounds, partly

to the north, and partly to the east of MBR(b).

Alternatively, the pair (MBR(a), MBR(b)) in Fig-

ure 3-(b) can be viewed as an instantiation of

the RCD-constraint aB:N:NE:E, b, as it holds that

MBR(a) B:N:NE:E MBR(a). Notice that while the

CD-constraint exactly speciﬁes the direction of re-

gion a with respect to the minimum bounding rectan-

gle of region b, the direction expressed by the RCD-

constraint is just approximated, since a does not in-

tersect the tile NE(b) (= NE(MBR(b))), that is, a

does not lie partly to the northeast of MBR(b). No-

tice also that, in general, a basic CD-constraint aRb

alone does not provide deﬁnite information about the

relative direction of pairs of regions. For that pur-

pose, both aRb and bR

′

a must be speciﬁed.

3.1 RCD and RA

The relationships between RCD and RA have

been systematically investigated in (Navarrete et al.,

2011). For instance, consider the RCD-constraint

a{NW:N}b. A possible instantiation of such a con-

straint is depicted in Figure 2. The very same

pair of rectangles can be viewed as an instance

of the RA-constraint a{(o, bi)}b as well. How-

ever, there exists another possible instantiation of

the constraint a{NW:N}b that satisﬁes the RA-

constraint a{(o, mi)}b. In general, for a given RCD-

constraint there exist more than one corresponding

RA-constraints, while for a given RA-constraint there

exists exactly one corresponding RCD-constraint.

This is due to the coarseness of RCD-relations with

respect to RA-relations. As an example, RCD does

not allow one to precisely state that two given rectan-

gles are externally connected or strictly disconnected,

or to constrain their sides to be (or to be not) ver-

tically (resp., horizontally) aligned. As a general

rule, given an RCD-relation, we can always deter-

mine the strongest RA-relation it implies. As an ex-

ample, the strongest RA-relation implied by NW:N is

{ fi, o} × {mi, bi}. Notice that such an RA-relation,

which is entailed by a basic RCD-relation, is not a

basic RA-relation.

The weaker expressivepowerof RCD with respect

RA is not necessarily a problem. As an example, if

an application is interested in pure cardinal informa-

tion only, the expressiveness of RCD-relations suf-

ﬁces. Moreover, the constraint language of the RCD-

calculus is closer to the natural language than the one

of the RA. For example, stating that “rectangle a lies

partly to the northwest and partly to the north of b”

(a{NW:N}b) is much more natural than stating that

“the x-projection of a is overlapping or ﬁnished by

the x-projection of b, and the y-projection of b is ...”

(a{ fi, o} × {mi, bi}b).

Figure 4: Translation from basic RCD-relations to RA-

relations via toRA mapping.

Figure 4 describes a translation function, called

toRA, to map a basic RCD-relation into the strongest

entailed RA-relation. This mapping can be extended

to translate arbitrary relations, constraints, and net-

works of RCD-calculus to their counterparts in RA,

preserving consistency. More precisely, given a dis-

junctive relation R, toRA(R) is obtained as the union

of the translation of the basic relations in R, while,

given an RCD-network N = (V,C), the corresponding

RA-network toRA(N) is obtained by replacing each

relation R

in C by toRA(R

). As the following the-

orem states, to decide the consistency of an RCD-

network N, one can compute the corresponding RA-

network toRA(N) and then apply any algorithm for

deciding the consistency of RA-networks (Navarrete

et al., 2011).

Theorem 1. An RCD-network N is consistent if and

only if the RA-network toRA(N) is consistent.

3.2 The Convex Fragment of RCD

In (Navarrete and Sciavicco, 2006), the authors prove

that the consistency problem for the RCD-calculus is

NP-complete, and they identify a tractable subset of

RCD-relations. A larger tractable fragment of RCD-

calculus, called convex RCD-calculus, has been iden-

tiﬁed in (Navarrete et al., 2011). Such a fragment

consists of all and only the RCD-relations R whose

translation toRA(R) is a convex RA-relation (convex

RCD-relations). It is possible to show that there exist

400 such relations.

As we already pointed out, the convex subclasses

ICAART 2012 - International Conference on Agents and Artificial Intelligence

158

Algorithm 3.1: the algorithm con-cRCD.

Require: a convex RCD-network N

1: N

← toRA(N);

2: N

← π

); N

← π

);

3: N

← toPA(N

); N

← toPA(N

);

4: If CSPAN(N

) or CSPAN(N

) returns an empty

network, then return ‘inconsistent’; otherwise, re-

turn ‘consistent’.

of IA, PA, and RA are tractable and PC-algorithms

can be used to decide their consistency. In particu-

lar, the following result holds for RA (Balbiani et al.,

1998):

Theorem 2. Let N be a convex RA-network. N is

path-consistent (resp., consistent) iff its projections

(N) and π

(N) are path-consistent (resp., consis-

tent). Moreover, if N is path consistent, then it is con-

sistent.

Making use of the above results, polynomial-time

algorithms to solve the consistency and the minimal-

ity problems for convex RCD-networks have been

proposed in (Navarrete et al., 2011). In the follow-

ing, we will exploit one of these algorithms, called

con-cRCD, that solves the two PA-networks corre-

sponding to a convex RCD-network. Such an algo-

rithm can be summarized as follows. Let N be a con-

vex RCD-network. First, it applies the mapping toRA

to get the convex RA-network N

corresponding to N.

Then, it computes the projections N

and N

of N

Next, it applies the mapping toPA to translate the con-

vex IA-networks N

and N

into two equivalent PA-

networks N

and N

with convex relations between

intervals endpoints. Such a mapping is based on the

list of the convex IA-relations and of their transla-

tions to PA given in (van Beek and Cohen, 1990).

Finally, the algorithm CSPAN (van Beek, 1992) is

applied to decide the consistency of the two convex

PA-networks in O(n

) (we assume that this algorithm

returns an empty network in case the input network

is inconsistent). It can be easily shown that such an

algorithm runs in O(n

). Algorithm 3.1 provides a

pseudocode encoding of con-cRCD.

4 CONVEX-METRIC RCD

In this section, we propose a tractable metric ex-

tension of the convex RCD-calculus, called convex-

metric RCD, to represent and to reason with both

qualitative cardinal constraints between rectangles

and metric constraints on the distance between the

endpoints of their projections.

4.1 STP

The main tool we use to deal with metric information

in convex-metric RCD is the STP formalism, which

was introduced in (Dechter et al., 1991) to process

metric information about time points. More precisely,

we use STP to elaborate information on the endpoints

of MBR projections onto the Cartesian axes.

Formally, an STP is speciﬁed by a constraint net-

work S = (P, M), where P is a set of point variables,

whose values range over a dense domain (we as-

sume it to be Q), and M is a set of binary metric

constraints over P. A metric constraint M

= [q, q

′

]

(open and semi-open intervals can be used), with

q, q

′

∈ Q, on the distance between (the values of)

, p

∈ P states that p

− p

∈ [q, q

′

], or, equivalently,

that q ≤ p

− p

≤ q

′

. Hence, the constraint M

de-

ﬁnes the set of possible values for the distance p

− p

In the constraint graph associated to S, M

= [q, q

′

]

is represented by an edge from p

to p

labeled by

the rational interval [q, q

′

]. Unary metric constraints

restricting the domain of a point variable p

can be

encoded as binary constraints between p

and a spe-

cial starting-point variable with a ﬁxed value, e.g., 0.

The universal constraint is ] − ∞, +∞[. The opera-

tions of composition (◦) and inverse (

−1

) of metric

constraints are computed by means of interval arith-

metic, that is, [q

, q

]◦ [q

, q

] = [q

+ q

, q

+ q

] and

, q

]

−1

= [−q

, −q

]. Intersection of constraints

(intervals) is deﬁned as usual.

Assuming such an interpretation of the operations

of composition, inverse, and intersection, Dechter et

al. (1991) showed that any PC-algorithm can be ex-

ploited to compute the minimal STP equivalent to

a given one, if any (if an inconsistency is detected,

the algorithm returns an empty network). In the fol-

lowing, we will denote such an algorithm by PC

stp

Making use of such a result, Meiri (1996) proposed

a formalism to combine qualitative constraints be-

tween points and intervals with (possibly disjunc-

tive) metric constraints between points (as in TCSP).

An easy special case arises when only convex PA-

constraints and STP-constraints are considered. Con-

vex PA-constraints can be encoded as STP-constraints

by means of the toSTP translation function described

in Table 1. The following result can be found in

Meiri (1996):

Theorem 3. Let N be a network with convex PA-

constraints and STP-constraints. If N is path-

consistent, then N is also consistent and its metric

constraints are minimal.

stp

can thus be used to decide the consistency

of a network N satisfying the conditions of the above

A TRACTABLE FORMALISM FOR COMBINING RECTANGULAR CARDINAL RELATIONS WITH METRIC

CONSTRAINTS

159

theorem. To this end, it sufﬁces to encode PA-

constraints into equivalent STP-constrains.

Table 1: Translation of convex PA-constraints to STP-

constraints via the toSTP mapping.

Convex PA relation STP constraint

< p

− p

∈ ]0, +∞[

≤ p

− p

∈ [0, +∞[

= p

− p

∈ [0, 0]

> p

− p

∈ ]−∞, 0[

≥ p

− p

∈ ]−∞, 0]

? p

− p

∈ ]−∞, +∞[

4.2 Integrating Convex RCD with STP

Combining RCD with STP makes it possible to ex-

press both directional constraints and metric con-

straints in a uniform framework. As an example, the

resulting formalism allows one to constrain the posi-

tion of a rectangle in the plane and to impose mini-

mum and/or maximum values to the width/height of

a given rectangle, or on the vertical/horizontal dis-

tances between the sides of two rectangles. Obvi-

ously, RCD-constraints and STP-constraints are not

totally independent, that is, RCD-constraints entail

some metric constraints and vice versa.

Example 3. Let a and b be two rectangle. We can

use the metric constraint 0 < a

− a

−

≤ 7 to state

that the maximum width of a is 7 and, similarly, we

can exploit the metric constraint 2 ≤ a

− a

−

to state

that the minimum height of a is 2 (leaving the max-

imum height unbounded). We can also express dis-

tance constraints between the boundaries of a and b.

We can constrain the horizontal distance between the

right side of a and the left side of b to be at least 3

by means of the constraint 3 ≤ b

−

− a

, and the ver-

tical distance between the upper side of a and the

bottom side of b to be greater than or equal to 0

by means of the constraint 0 ≤ b

−

− a

. The two

constraints together entail the basic RCD constraint

a{SW}b. Finally, some metric constraints can be

entailed by RCD ones. For instance, the convex re-

lation a{NW, N, NE, NW:N, NW:N:NE, N:NE}b im-

plies that 0 ≤ a

−

− b

If we allow one to combine arbitrary RCD-

constraints with metric constrains, then checking the

consistency of the resulting set of constraints turns out

to be an NP-complete problem (the consistency prob-

lem for RCD-networks is already NP-complete). To

preserve tractability, we restrict our attention to the

combination of convex RCD-constraints with STP-

constraints to establish the convex-metric RCD for-

malism.

Given a convex RCD-network N

= (V,C), we de-

note the sets of interval variables belonging to the pro-

jections π

(toRA(N

)) and π

(toRA(N

)) by V

and

, respectively. Moreover, we denote by P(V

) and

P(V

) the sets of point variables representing the end-

points of the interval variables in V

and V

, respec-

tively. A convex-metric RCD-network is formally de-

ﬁned as follows.

Deﬁnition 2. A convex-metric RCD-network

(cmRCD-network) is an integrated qualitative and

metric constraint network N consisting of three

sub-networks (N

, S

), where N

= (V,C) is a

convex RCD-network, and S



P(V

), M



and



P(V

), M



are two STPs.

The convex-metric RCD formalism we propose sub-

sumes the STP formalism and the convex RCD-

calculus. Moreover, it also generalizes the convex

fragment of the RA, since convex RA-relations are

expressible as convexPA-relations and these relations

can be, in turn, encoded into an STP.

Now, we provide an algorithm to solve the consis-

tency problem for cmRCD that runs in O(n

)

. First,

we extend the translation mapping toSTP of Table 1

to encode a convex PA-network N

into an STP S

by replacing each relation R

in the network N

toSTP(R

). By exploiting such a function, we can

generalize the algorithm con-cRCD of Section 3.2

to deal with both RCD- and STP-constrains (Algo-

rithm con-cmRCD). First, con-cmRCD computes

the PA-networks N

and N

, and then, making use

of information about convex RCD-relations encoded

as PA-relations, it looks for possible inconsistencies

between these constraints and the STP-constrains on

the same variables given in S

and S

that can be de-

tected at this stage. To this end, it translates the PA-

network N

(resp., N

) into an STP-network by ap-

plying the function toSTP, and then it uses the func-

tion intersect to compute the “intersection” between

toSTP(N

) and S

(resp., toSTP(N

) and S

). This

function simply intersects the intervals / metric con-

strains associated with the same pairs of variables in

the two STPs. If an interval intersection produces an

empty interval, then intersect returns an empty net-

work, and we can conclude that N is inconsistent.

Otherwise, we apply the path-consistency algorithm

to the two STPs computed at lines 4 and 5 independ-

A similar combination of qualitative and quantitative

networks is given by preconvex-augmented rectangle net-

works by Condotta (2000), that subsume cmRCD-networks.

An O(n

) algorithm for checking the consistency of these

networks has been devised by Condotta (Condotta, 2000).

We exploit the trade-off between expressiveness and com-

plexity to obtain a more efﬁcient consistency checking al-

gorithm.

ICAART 2012 - International Conference on Agents and Artificial Intelligence

160

Algorithm 4.1: The algorithm con-cmRCD.

Require: a cmRCD-network N = (N

, S

)

1: N

← toRA(N

);

2: N

← π

), N

← π

);

3: N

← toPA(N

), N

← toPA(N

);

4: xSTP ← intersect(toSTP(N

), S

);

5: ySTP ← intersect(toSTP(N

), S

);

6: if xSTP or ySTP is empty, then return ‘inconsis-

tent’;

7: xSTP

min

← PC

stp

(xSTP);

8: ySTP

min

← PC

stp

(ySTP);

9: If xSTP

min

or ySTP

min

is empty, then return ‘in-

consistent’; otherwise, return ‘consistent’.

ently. The following theorem proves that

con-cmRCD is sound and complete.

Theorem 4. Given a cmRCD-network N = (N

, S

), the algorithm con-cmRCD returns ‘consistent’

if and only if N is consistent.

Proof. We basically follow the steps of the algo-

rithm. By Theorem 1, N

is consistent if and only

if N

is consistent, and, by Theorem 2, N

is con-

sistent if and only if N

and N

are consistent (they

can be checked independently). Next, N

and N

are consistent if and only if N

and N

are consis-

tent, since there is no loss in information in the trans-

lations (van Beek and Cohen, 1990). The consis-

tency of N

and N

could be checked by comput-

ing the corresponding STPs and by applying PC

stp

However, we cannot apply PC

stp

directly to the STPs

toSTP(N

) and toSTP(N

) since the metric con-

straints of S

and S

must be taken into account.

Hence, we compute intersect(toSTP(N

), S

) and

intersect(toSTP(N

), S

). If one of them returns an

empty network, then N is inconsistent. Otherwise, we

independently apply PC

stp

to xSTP and ySTP. By

Theorem 3, if one of the two applications of PC

stp

re-

turns an empty network, then N is inconsistent; other-

wise, the path-consistent STPs xSTP

min

and ySTP

min

are consistent (and minimal), and thus N is consis-

tent.

Theorem 5. The complexity of the algorithm

con-cmRCD is O(Rn

), where n is the number of

variables and R is the maximum range of the network.

Proof. The translation via toRA, the generation of a

projection of a network, the transformation of a IA-

network into a RA-network via toPA and the last two

encodings via toSTP require O(n

) steps, since there

are O(n

) constraints and each constraint can be trans-

lated in constant time. The function toPA introduces

two variables for each interval variable, so xSTP and

ySTP have O(n) variables each. Finally, PC

stp

runs

in O(Rn

) time, so the overall complexity is O(Rn

)

time, where R is the maximum range of the network

(for more details about the complexity of achieving

path-consistency for combined networks see (Meiri,

1996)).

Once we have computed the path-consistent STPs

xSTP

min

and ySTP

min

with algorithm con-cmRCD,

we can build a solution to the convex-metric RCD-

network N by computing a solution for the points

in xSTP and ySTP, since the assignment for point

variables deﬁnes a consistent assignment for rectan-

gle variables (see Example 1). To this end, the al-

gorithm STP-SOLUTION by Gerevini and Cristani

(1997) (Gerevini and Cristani, 1997) can be used.

To illustrate the expressive power of the convex-

metric RCD-calculus and its potential applications,

we show an example regarding the design of 2D-

layouts.

Example 4. Uncle Scrooge wants to buy a plot of

land (p) to build a new money bin (m), an ofﬁce (o), a

house (h) and a swimming pool (s) for Huey, Dewey,

and Louie. The surfaces of the buildings are supposed

to be rectangular, with sides aligned to the sides of

the plot, which also has a rectangular shape. Dur-

ing the feasibility study of the project, the following

requirements arose: i) the vertical and horizontal dis-

tance between the boundaries of p and any building

it contains must be at least 100m for reasons of pri-

vacy; ii) the surface area of m is 70m×70m; iii) m

must lie somewhere between the northwest zone and

the northeast zone of h, and the same w.r.t. o; iv) the

vertical distance between m and h (resp., o) must be

at least 100m because Uncle Scrooge does not want

to be disturbed too much by his employees; v) the sur-

face area of h is 100m×50m, while the surface area

of o is 30m×70m; vi) o must lie between the northeast

zone and east zone of h; vii) the horizontal distance

between o and h must be at least 60m and at most

80m so that Huey, Dewey, and Louie can play without

disturbing their uncle’s workers; viii) s is an olympic-

size swimming pool so its surface area has to be at

least 50m×25m and at most 100m×50m; ix) s must

be situated between the southwest zone and southeast

zone of h, and the same w.r.t. o; x) the vertical dis-

tance between s and h and between s and o must be at

least 50m.

Let us see howto represent the requirements of the

above example with a cmRCD-network. The qualita-

tive part of the network contains the following convex

RCD-contraints between variables p, m, h, o, and s

representing the plot and the buildings:

A TRACTABLE FORMALISM FOR COMBINING RECTANGULAR CARDINAL RELATIONS WITH METRIC

CONSTRAINTS

161

−

]-∞, 100]

[100, +∞[

]-∞, 0[

]0, +∞[

[100, 100]

[30, 30]

[70, 70]

[50, 100]

]-∞, 0[

[60, 80]

]-∞, 100]

]0, +∞[

−

[100, +∞[

[50, 50]

[70, 70]

[25, 50]

[50, +∞[

[100, +∞[

]-∞, 0[

]-∞, 0]

]-∞, 0[

]0, +∞[

[70, 70]

Figure 5: Graph representation of part of xSTP and part of ySTP of Example 4. For clarity, constraints involving p in ySTP

are omitted, as well as the universal constraint.

Implicit: “buildings must be inside the plot”:

oB p, hB p, mB p, sB p;

iii) m{NW, N, NW:N, NW:N:NE, N:NE, NE}h,

m{NW, N, NW:N, NW:N:NE, N:NE,NE}o;

vi) o{NE, NE:E, E}h;

ix) s{SW, S, SW:S, SW:S:SE, S:SE, SE} h,

s{SW, S, SW:S, SW:S:SE, S:SE, SE} o;

The quantitative part of the network contains the fol-

lowing metric constraints forming two STPs:

i) for all buildings b:

−

− p

−

≥ 100, p

− b

≥ 100,

−

− p

−

≥ 100, p

− b

≥ 100

ii) m

− m

−

= 70, m

− m

−

= 70;

iv) m

−

− h

≥ 100, m

−

− o

≥ 100;

v) h

− h

−

= 100, h

− h

−

= 50,

− o

−

= 30, o

− o

−

= 70;

vii) 60 ≤ o

−

− h

≤ 80;

viii) 50 ≤ s

− s

−

≤ 100, 25 ≤ s

− s

−

≤ 50

x) h

−

− s

≥ 50, o

−

− s

≥ 50.

By applying our consistency algorithm we can verify

that it is possible to realize the building project of the

example (the corresponding cmRCD-network is con-

sistent). We can also determine the minimum area

that the plot should have by using the minimal net-

works xSTP

min

and ySTP

min

: in our example the min-

imum area of p is 390m×515m while the maximum

area is unbounded. The STPs xSTP and ySTP, com-

puted by steps 4 and 5 of our algorithm, are sketched

in Figure 5, while a solution of the problem is illus-

trated by Figure 6, showing the minimum feasible val-

ues for the point variables. To simplify, we suppose

that the origin of the reference system is the lower-left

vertex of the plot, since the plot encloses all the build-

335

415

245

175

125

100

225

180

250 260 290

100

200

165

0 390

515

100

Figure 6: A solution to the cmRCD-network corresponding

to Example 4.

ing and there is no constraint between the plot and the

space around it.

5 CONCLUSIONS

In this paper, we have proposed a quite expressive,

but tractable, metric extension of RCD (cmRCD),

that integrates STP-constraints with convex RCD-

constraints. cmRCD allows one to constrain the posi-

tion of a rectangle in the plane, its width/height, and

the vertical/horizontal distance between the sides of

two rectangles, as well as to represent cardinal rela-

tions between rectangles. We have devised an O(n

)

consistency-checking algorithm, and we have showed

how a spatial realization of a network can be built.

As for future work, we plan to extend cmRCD

with topological relations to improve its expressive-

ICAART 2012 - International Conference on Agents and Artificial Intelligence

162

ness (similar results can be found in (Gerevini and

Renz, 2002; Liu et al., 2009)). The problem of identi-

fying maximal tractable subsets of RCD is still open.

It would be interesting to search for tractable classes

(strictly) including the convex fragment.

ACKNOWLEDGEMENTS

This work has been partially supported by the Span-

ish Ministry of Science and Innovation, the European

Regional Development Fund of the European Com-

mission under grant TIN2009-14372-C03-01, and

the Spanish MEC through the project 15277/PI/10,

funded by Seneca Agency of Science and Technol-

ogy of the Region of Murcia within the II PCTRM

2007-2010. Finally, Guido Sciavicco and Angelo

Montanari were also partially founded by the Span-

ish fellowship ‘Ramon y Cajal’ RYC-2011-07821and

by the Italian PRIN project Innovative and multi-

disciplinary approaches for constraint and preference

reasoning, respectively.

REFERENCES

Allen, J. (1983). Maintaining knowledge about temporal

intervals. Communications of the ACM, 26(11):832–

843.

Balbiani, P., Condotta, J., and del Cerro, L. (1998). A model

for reasoning about bidimensional temporal relations.

In Proceedings of KR-98, pages 124–130.

Balbiani, P., Condotta, J., and del Cerro, L. (2002).

Tractability results in the block algebra. Journal of

Logic and Computation, 12(5):885–909.

Baykan, C. and Fox, M. (1997). Spatial synthesis by dis-

junctive constraint satisfaction. Artiﬁcial Intelligence

for Engineering Design, Analysis and Manufacturing,

11(4):245–262.

Broxvall, M. (2002). A method for metric temporal reason-

ing. In Proceedings of AAAI-02, pages 513–518.

Cohn, A. and Hazarika, S. (2001). Qualitative spatial rep-

resentation and reasoning: An overview. Fundamenta

Informaticae, 46(1-2):1–29.

Condotta, J. F. (2000). The augmented interval and rectan-

gle networks. In Proceedings of KR-00, pages 571–

579.

Dechter, R., Meiri, I., and Pearl, J. (1991). Temporal con-

straint networks. Artiﬁcial Intelligence, 49(1-3):61–

95.

El-Geresy, B. and Abdelmoty, A. (2001). Qualitative rep-

resentations in large spatial databases. Proceedings of

IDEAS-01, pages 68–75.

Frank, A. (1996). Qualitative spatial reasoning: Cardinal

directions as an example. International Journal of Ge-

ographical Information Science, 10(3):269–290.

Gatterbauer, W. and Bohunsky, P. (2006). Table extraction

using spatial reasoning on the CSS2 visual box model.

In Proceedings of AAAI-06, volume 2.

Gerevini, A. and Cristani, M. (1997) On ﬁnding a solution

in temporal constraint satisfaction problems. In Pro-

ceedings of IJCAI-97, pages 1460–1465.

Gerevini, A. and Renz, J. (2002). Combining topological

and size information for spatial reasoning. Artiﬁcial

Intelligence, 137(1-2):1–42.

Goyal, R. (2000). Similarity assessment for cardinal di-

rections between extended spatial objects. PhD the-

sis, University of Maine, Dept. of Spatial Information

Science and Engineering.

Goyal, R. and Egenhofer, M. (2000). Consistent queries

over cardinal directions across different levels of de-

tail. In Proceedings of DEXA-00, pages 876–880.

Liu, W., Li, S., and Renz, J. (2009). Combining RCC-8 with

qualitative direction calculi: Algorithms and complex-

ity. In Proceedings of IJCAI-09, pages 854–859.

Liu, W., Zhang, X., Li, S., and Ying, M. (2010). Reasoning

about cardinal directions between extended objects.

Artiﬁcial Intelligence, 174:951–983.

Mackworth, A. (1977). Consistency in networks of rela-

tions. Artiﬁcial Intelligence, 8(1):99–118.

Meiri, I. (1996). Combining qualitative and quantitative

constraints in temporal reasoning. Artiﬁcial Intelli-

gence, 87(1-2):343–385.

Navarrete, I., Morales, A., Sciavicco, G., and Cardenas-

Viedma, M. (2011). Spatial reasoning with rect-

angular cardinal relations. Technical Report TR-

DIIC 2/11, Departamento de Ingenieria de la Informa-

cion y las Comunicaciones. Universidad de Murcia.

http://sites.google.com/site/aikespatial/RCDC.

Navarrete, I. and Sciavicco, G. (2006). Spatial reason-

ing with rectangular cardinal direction relations. In

Proceedings of the ECAI-06 Workshop on Spatial and

Temporal Reasoning, pages 1–10.

Papadias, D. and Theodoridis, Y. (1997). Spatial relations,

minimum bounding rectangles, and spatial data struc-

tures. International Journal of Geographical Informa-

tion Science, 11(2):111–138.

Skiadopoulos, S., Giannoukos, C., Sarkas, N., Vassiliadis,

P., Sellis, T., and Koubarakis, M. (2005). Comput-

ing and managing cardinal direction relations. IEEE

Transactions on Knowledge and Data Engineering,

17(12):1610–1623.

Skiadopoulos, S. and Koubarakis, M. (2005). On the con-

sistency of cardinal directions constraints. Artiﬁcial

Intelligence, 163(1):91–135.

van Beek, P. (1992). Reasoning about qualitative temporal

information. Artiﬁcial Intelligence, 58(1-3):297–326.

van Beek, P. and Cohen, R. (1990). Exact and approximate

reasoning about temporal relations. Computation In-

telligence, 6(3):132–147.

Vilain, M. and Kautz, H. (1986). Constraint propagation

algorithms for temporal reasoning. In Proceedings of

AAAI-86, pages 377–382.

A TRACTABLE FORMALISM FOR COMBINING RECTANGULAR CARDINAL RELATIONS WITH METRIC

CONSTRAINTS

163