EFFICIENCY EVALUATION IN ACADEMIC UNITS APPLYING

DATA ENVELOPMENT ANALYSIS

Initial State of Project

Horacio Rojo, Silvia Adriana Ramos, Pedro Tolón Estarelles

Claus Stegmann, Leandro J. Raspa and Diego Castro

Grupo de Investigación Modelos de Gestión, Departamento de Gestión Industrial

Facultad de Ingeniería, Universidad de Buenos Aires, Avenida Las Heras 2214

Ciudad Autónoma de Buenos Aires, Argentina

Keywords: DEA, Data Envelopment Analysis, Efficiency Analysis, Resource Administration, Multicriteria Decision.

Abstract: The aim of this paper is to present a procedure to look into the relative efficiency of university departments

in order to make a good allocation of resources. This procedure uses a model based on Data Envelopment

Analysis (DEA). DEA measures relative efficiency of a set of alternatives (decision making units – DMUs)

that consume multiple inputs and produce multiple outputs. Results of the model will help to plan

development of university departments in Facultad de Ingeniería of Universidad de Buenos Aires.

1 INTRODUCTION

In recent years, Facultad de Ingeniería (Engineering

School) of University of Buenos Aires (FIUBA) has

established a policy in order to encourage research

activities mostly on new areas. As a result of this

policy, several professors with full dedication were

designated by open contest in some departments

such as Industrial Engeneering.

In the case of Industrial Management

Department (Departamento de Gestión Industrial),

incorporation of professors has led to the formation

of teacher-investigators groups that, even being still

small, are allowing to start an interesting series of

investigating activities. One of the mentioned cores

is the Management Models Group (Grupo de

Modelos de Gestión) which leads the working line

presented on this paper.

The aim of this Group is to provide tools for

decision making in order to analize, evaluate and

solve real problems in operations, processes and

management. The objective of this first project is the

asessment of FIUBA's teaching departments to

provide information for better allocation of

resources. This information will be based on

comparison of the teaching and research

performance.

Nowadays, resource allocation in FIUBA has

been implemented with intuitive, subjective

procedures, not properly focused within a problem

structure. Usually, not enough criteria were taken

into account.

Therefore, changing the indexes used in

productivity often leads to apparently inconsistent

results. That is, according to an index, performance

is well, but other index does not rate as well.

Sometimes, results among different indexes can be

complete opposites.

The idea of this paper consists in developing a

mathematical model in which different indexes

weights are the variables to calculate. In that way

objectivity can be achieved because it would be

possible to demonstrate that some of the evaluated

units would not be able to achieve the best results

even adopting the most favourable weights for them.

The starting point is the classical work of

Charnes, Cooper and Rhodes (Charnes et al, 1978)

in wich a new method for evaluating decision

making units named Data Envelopment Analysis is

proposed. Also the work of Banker, Charnes and

Cooper (Banker et al, 1984) broadens the field of the

first work.

In the present paper, we study factibility of

application of those approaches plus other posterior

works (Cooper et al, 2000), (Thanassoulis, 2001),

473

Rojo H., Adriana Ramos S., Tolón Estarelles P., Stegmann C., J. Raspa L. and Castro D..

EFFICIENCY EVALUATION IN ACADEMIC UNITS APPLYING DATA ENVELOPMENT ANALYSIS - Initial State of Project.

DOI: 10.5220/0003748904730475

In Proceedings of the 1st International Conference on Operations Research and Enterprise Systems (ICORES-2012), pages 473-475

ISBN: 978-989-8425-97-3

 2012 SCITEPRESS (Science and Technology Publications, Lda.)

(Ray, 2004) to academic departments of FIUBA,

taking those departments as operative units and

asessing, analyzing and evaluating their possible

inputs and outputs.

This project was accredited by University of

Buenos Aires with code 2002 0090 2006 29 in the

UBACyT 2010-2012 period.

2 METHOD

2.1 Hypothesis

In order to achieve that, validity of a quantitative

approach like DEA methodology will be

experimented. This methodology bases in this

essential hipothesis:

 Input and output measures for each university

unit are known;

 Efficiency for each unit is presented as a

weighted sum of the outputs defined divided

by the weighted sum of the inputs defined;

 All efficiencies will be restricted to the (0, 1)

range;

 Mathematical model proposed will ougth to

determine efficiency value for each unit by

maximizing its efficiency and determining

weights for inputs and outputs.

2.2 Information Used

The first plan is to use information that belongs to

last years of management in FIUBA. In subsequent

stages, when methodology would be validated,

extension to other internal academic units and

external units is planned.

One of the core aspects of the process will be the

selection of units to be evaluated. We have used

academic units as FIUBA Departments, Institutes,

Schools, trying to be relatively similar in dimension

and characteristics.

Once the units to be evaluated were identified

we defined inputs and output, according to available

information and apply some appropriate method for

selecting variables, restricting its quantity in order to

obtain a reasonable degree in discriminating

efficiencies and taking into account (Cooper et al,

2000, 2007) refering compliance of >= max((m .

s);3(m + s)) being m inputs and s outputs.

Other aspect that should be matter of analysis is

DEA's classical assumption that efficiencies of units

for every activity are equal. This assumption could

not be true in case of academic units, where

activities as teaching, research, extension may have

different efficiencies. This situation would force

rethinking the model to consider that fact.

2.3 Method

As mentioned early, a quantitative method based on

DEA approach is used. Within this central idea,

DEA method has a group of alternative models

available, from which the basic are CCR models,

owing their name to their authors: A. Charnes, W.

W. Cooper and E. Rhodes (Charnes et al, 1978),

who initially proposed them with a non linear

optimization structure.

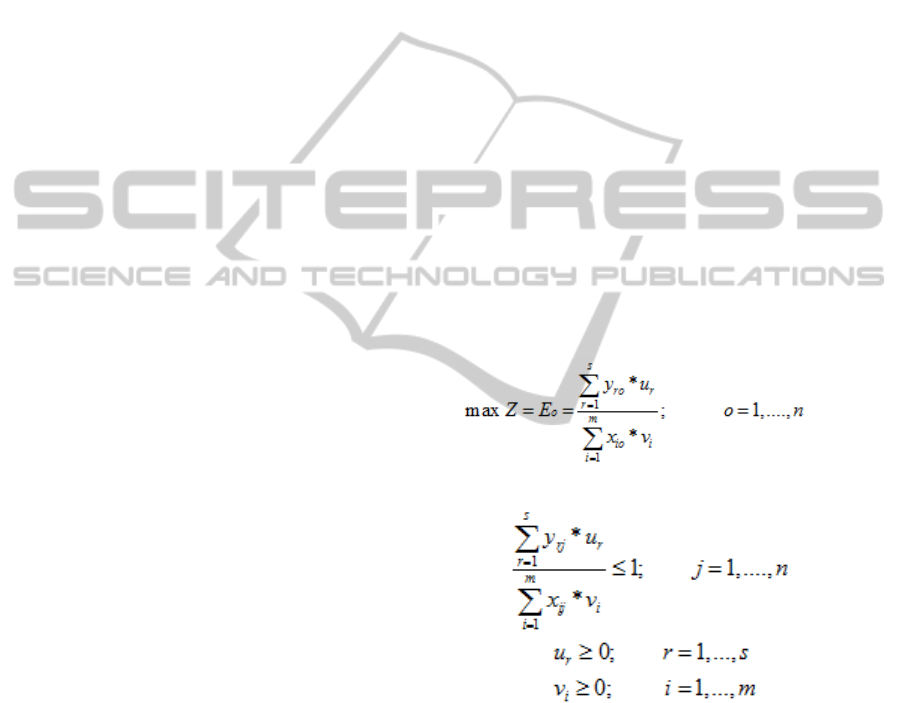
Non-linear CCR DEA model has an objective

function that maximizes efficiency E

, where j = o

means the generic S

system subscript. In this paper,

j is an academic or management unit for which

weights u

and v

are to be determined. This weights

and v

are applied in every S

efficiency expression

within ≤ 1 inequalities, trying to normalize its

values, therefore forming the model restrictions,

which are complemented with non-negativity

conditions for the weights u

and v

. The model

obtained has a non-linear structure.

(1)

Subject to:

(2)

The process is repeated for each of the n S

systems, leading to a series of n groups of weights u

and v

To determine efficiency of each functional unit,

it is needed to solve n models, one for each unit. The

basic model is a non-linear model, which can be

solved with non-linear optimizers. However, this

model can be taken to an equivalent linear form,

with the inherent advantages of linear models. This

is achieved fixing the objective

function

denominator as 1. In that way, the numerator itself

will measure efficiency, passing denominators of the

n restrictions to the second member and, then, the

ICORES 2012 - 1st International Conference on Operations Research and Enterprise Systems

474

whole second member to the first

member. The

model obtained has a linear programming structure:

(3)

Subject to:

(4)

As in the non-linear model, the process repeats

in the same way for each of the n S

systems, thus

obtaining a series of n groups of weights u

and v

2.4 Preliminary Results

In Figure 1 is shown the preliminary results of our

project:

DMU Score Rank

1 Matemática 0,61 8

2 Física 0,55 10

3 Química 0,68 6

4 Estabilidad 0,61 7

5 Electrotecnia 0,44 15

6 Electrónica 0,43 16

7 Mecánica 0,55 9

8 Transporte 0,25 18

9 Hidráulica 0,46 13

10 Agrimensura 0,45 14

11 Economía 0,89 4

12 Industrias 0,87 5

13 Ingeniería Naval 0,38 17

14 Construcciones 0,52 11

15 Computación 1,00 1

16 Ing. Química 0,52 12

17 Ambiente y Trabajo 1,00 1

18 Idiomas 1,00 1

Figure 1: Preliminary Results

Further research might explore other DEA

models, like BCC (Banker et al, 1984), AR (Cooper

et al, 2000, 2007) and other models that may emerge

from investigation.

Another aspect to define will be the software to

use. This selection will depend on the dimension of

the Linear Programming formulated model. Some of

the specific decision analysis software are DEA

Solver, Frontier Analysis, Warwick DEA.

3 CONCLUSIONS

The approach proposed is not the only tool being

taken into account for decision making within

planning and management of FIUBA's academic

units, but is considered as a new contribution to be

integrated to the collection of other proceedings and

tools in use.

DEA methodology seems to be a suitable tool

for efficiency analysis in education.

As a not less important sub-product, the

investigation work already developed by the

Management Models Group during this first stage

and the work to develop during the project will

allow gain experience in research activities that will

not only benefit its members but also motivate other

teachers and students to initiate them in similar

activities, therefore responding to the policy

promoted by the authorities of FIUBA.

REFERENCES

Banker, R. D., Charnes, A. I., Cooper, W. W. 1984. Some

Models for estimating technical and scale

inefficiencies in Data Envelopment Analysis. In

Management Science, vol 30 (9). Pgs 1078 to 1092.

Charnes, A. I., Cooper, W. W., Rhodes, S. 1978.

Measuring the efficiency of Decision Making Units. In

European Journal of Operational Research, vol 2, Pgs

429 to 444.

Cooper, W. W., Seiford, L. M., Tone, K. 2000, 2007. Data

Envelopment Analysis: A comprehensive text with

models, applications, references and DEA-Solver

software. Kluwer Academic Publishers, Norwell

Massachusetts.

Thanassoulis, E. 2001. Introduction to the theory and

application of Data Envelopment Analysis. Kluwer

Academic Publishers, Norwell Massachusetts.

Ray, S. C., 2004. Data Envelopment Analysis. Theory and

techniques for economics and Operations Research,

Cambridge University Press.

EFFICIENCY EVALUATION IN ACADEMIC UNITS APPLYING DATA ENVELOPMENT ANALYSIS - Initial State

of Project

475