SPACE-TIME VISUALIZATION OF DYNAMICS IN LAGRANGIAN

COHERENT STRUCTURES OF TIME-DEPENDENT 2D VECTOR

FIELDS

Sven Bachthaler

, Filip Sadlo

, Carsten Dachsbacher

and Daniel Weiskopf

VISUS, University of Stuttgart, Stuttgart, Germany

Karlsruhe Institute of Technology, Karlsruhe, Germany

Keywords:

Flow Visualization, Lagrangian Coherent Structures, Hyperbolic Trajectories.

Abstract:

Lagrangian coherent structures (LCS), apparent as ridges in the ﬁnite-time Lyapunov exponent (FTLE) ﬁeld,

represent a time-dependent alternative to the concept of separatrices in vector ﬁeld topology. Traditionally,

LCS are analyzed and visualized in terms of their geometric shape only, neglecting stretching and compression

in tangent directions. These effects are, however, of particular interest in mixing phenomena and turbulence.

Hyperbolicity plays an important role in these processes and gives rise to hyperbolic trajectories originating at

the intersections of forward and reverse LCS. Since integration of hyperbolic trajectories is difﬁcult, we pro-

pose to visualize the corresponding space-time intersection curves of LCS instead. By stacking the traditional

2D FTLE video frames of time-dependent vector ﬁelds, a space-time FTLE ﬁeld is obtained. In this ﬁeld, ridge

lines turn into ridge surfaces representing LCS, and their intersection forms curves that are a robust alterna-

tive to hyperbolic trajectories. Additionally, we use a space-time representation of the time-dependent vector

ﬁeld, leading to a steady 3D space-time vector ﬁeld. In this ﬁeld, the LCS become stream surfaces given that

their advection property is sufﬁciently met. This makes visualization of the dynamics within LCS amenable

to line integral convolution (LIC), conveying in particular the dynamics around hyperbolic trajectories. To

avoid occlusion, the LCS can be constrained to space-time bands around the intersection curves, resembling

visualization by saddle connectors. We evaluate our approach using synthetic, simulated, and measured vector

ﬁelds.

1 INTRODUCTION

As science and engineering methods evolve, model-

ing of phenomena is shifting from stationary to time-

dependent domains. 2D computational ﬂuid dynam-

ics (CFD) simulations are of major importance in sev-

eral domains, such as the analysis of ﬂow in ﬁlms and

on free-slip boundaries. To examine and understand

such data, efﬁcient tools for analysis and visualization

are required. Feature extraction techniques, provid-

ing a condensed representation of the essential infor-

mation, are often applied to the visualization of vec-

tor ﬁelds. A prominent concept revealing the overall

structure is vector ﬁeld topology (Helman and Hes-

selink, 1989). Whereas vector ﬁeld topology is di-

rectly applicable only to steady or quasi-stationary

vector ﬁelds, Lagrangian coherent structures (LCS)

(Haller, 2001) are popular for the analysis of time-

dependent vector ﬁelds. LCS are a time-dependent

counterpart to separatrices, which are streamlines st-

arted from separating regions of different behavior.

LCS have been increasingly subject to research in

the last decade and can be obtained as maximizing

curves (ridges) in the ﬁnite-time Lyapunov exponent

(FTLE), a scalar ﬁeld measuring the separation of tra-

jectories (Haller, 2001). FTLE computation is, how-

ever, an expensive task because at least one trajectory

needs to be computed per sample point. LCS behave

as material lines under the action of time-dependent

ﬂow, i.e., they are advected and exhibit negligible

cross-ﬂow for sufﬁciently long advection time inter-

vals, as reported by Haller (Haller, 2001), Lekien et

al. (Lekien et al., 2005), and Sadlo et al. (Sadlo et al.,

2012). This property gives rise, e.g., to the acceler-

ation technique by Sadlo et al. (Sadlo et al., 2011)

based on grid advection.

Our new method adopts the concept of hyperbolic

trajectories and space-time streak manifolds, which

we therefore discuss in the following. Previous work

by Sadlo and Weiskopf (Sadlo and Weiskopf, 2010)

573

Bachthaler S., Sadlo F., Dachsbacher C. and Weiskopf D..

SPACE-TIME VISUALIZATION OF DYNAMICS IN LAGRANGIAN COHERENT STRUCTURES OF TIME-DEPENDENT 2D VECTOR FIELDS.

DOI: 10.5220/0003810905730583

In Proceedings of the International Conference on Computer Graphics Theory and Applications (IVAPP-2012), pages 573-583

ISBN: 978-989-8565-02-0

 2012 SCITEPRESS (Science and Technology Publications, Lda.)

Figure 1: Left: traditional visualization of a time-dependent vector ﬁeld by time series of the ﬁnite-time Lyapunov exponent

is difﬁcult to analyze and does not convey the dynamics inside its ridges (Lagrangian coherent structures). Right: our space-

time representation reveals the overall structure and makes the dynamics inside the Lagrangian coherent structures visible by

line texture patterns. Close-ups: in contrast to the traditional 2D visualization, different dynamics along intersection curves

(almost parallel ﬂow on the left vs. strongly hyperbolic ﬂow on the right) is apparent.

generalized vector ﬁeld topology to time-dependent

vector ﬁelds by replacing the role of streamlines by

generalized streak lines (Wiebel et al., 2007). This

way, critical points turn into degenerate streak lines

and separatrices into streak lines (space-time streak

manifolds) converging toward these degenerate streak

lines in forward or reverse time. It was found that

these need to be distinguished degenerate streak lines

identical to the previously discovered hyperbolic tra-

jectories (Haller, 2000).

Hyperbolic trajectories can be seen as constituent

structures in time-dependent 2D vector ﬁeld topol-

ogy. As mentioned, space-time streak manifolds—

the time-dependent counterpart to separatrices—can

be constructed alone from hyperbolic trajectories—

no dense sampling is required in contrast to the FTLE

approach. However, a major limitation with hyper-

bolic trajectories is the difﬁculty of their integration.

Although the integration error tends to grow exponen-

tially in linear vector ﬁelds, it is usually negligible due

to comparably short advection times and low separa-

tion rates along common trajectories. Unfortunately,

this is not the case in typical hyperbolic conﬁgura-

tions due to large separation rates and the fact that

both forward and reverse integration are subject to re-

pulsion from one of the LCS (see Fig. 2). Hyperbolic

trajectories coincide with the intersection of forward

and reverse LCS; since ridges in forward FTLE repre-

sent repelling LCS whereas those in reverse FTLE are

attracting, the trajectory is repelled from the former

forward and from the latter in backward direction.

Our method has therefore a twofold objective:

(1) avoiding the integration of hyperbolic trajecto-

ries by replacing them with intersections of LCS, and

(2) revealing tangential dynamics in LCS, accom-

plished by line integral convolution (LIC). By treat-

ing time as an additional dimension, we obtain a sta-

tionary visualization that conveys the overall struc-

ture in space-time. Several approaches for obtaining

seeds for hyperbolic trajectories exist: by intersecting

ridges in hyperbolicity time (Haller, 2000), ridges in

FTLE (Sadlo and Weiskopf, 2010), and constructing

streak manifolds from them, or by building a time-

dependent linear model from critical points (Ide et al.,

2002). This kind of visualization of hyperbolic tra-

jectories is, however, restricted to LCS geometry, i.e.,

the dynamics in the vicinity of the hyperbolic trajecto-

ries is not conveyed. Furthermore, the hyperbolicity

of the vector ﬁeld is typically analyzed by requiring

negative determinant of the velocity gradient. This

approach fails in providing insight into the role and

importance of hyperbolic trajectories. In contrast, our

LIC-based visualization captures the conﬁguration of

the ﬂow in the neighborhood of hyperbolic trajecto-

ries and also in general along LCS. One example is

the discrimination of almost parallel ﬂow conﬁgura-

tions from strongly hyperbolic ones (Fig. 1, (right)).

This provides increased insight in the overall dynam-

ics, interplay, and importance of LCS. For example,

this allows for a qualitative analysis of mixing phe-

nomena. We refer the reader to ﬂuid mechanics litera-

ture, e.g., (Mathur et al., 2007) for the underlying con-

cepts, e.g., for details on mixing and the Lagrangian

skeleton of turbulence.

IVAPP 2012 - International Conference on Information Visualization Theory and Applications

574

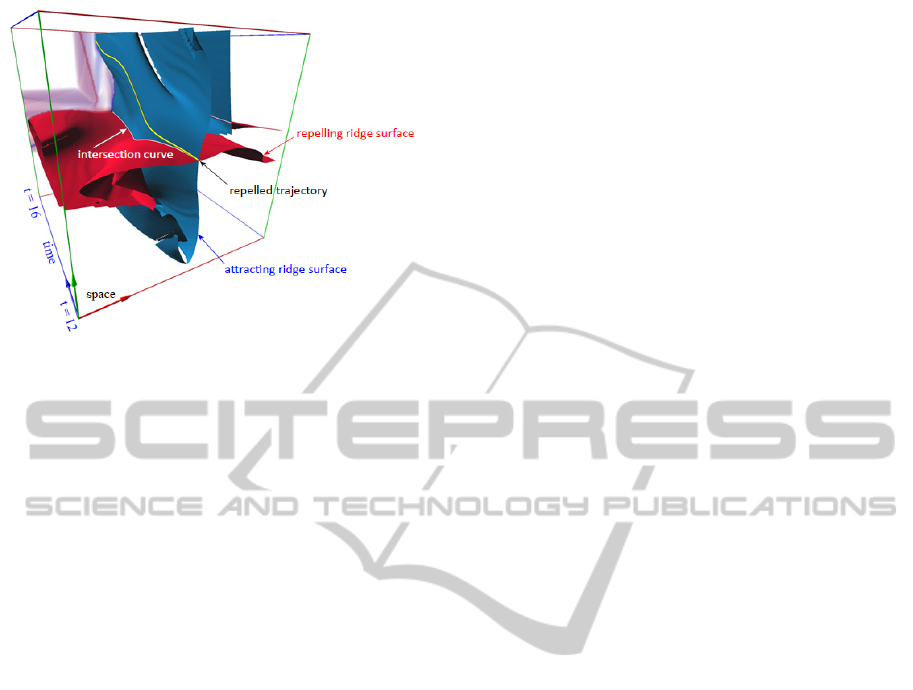
Figure 2: Space-time ridge surfaces in forward (red) and

reverse (blue) ﬁnite-time Lyapunov exponent together with

cross section at ﬁnal time step (colored). The space-time

intersection curve in the center (white) represents a hyper-

bolic trajectory. Traditional integration of the hyperbolic

trajectory from the initial intersection is difﬁcult due to ex-

ponential growth of error (yellow curve).

2 RELATED WORK

Work that is closely related to our objectives was dis-

cussed in the previous section, here we give a short

overview of work that is in weaker context.

Many applications of vector ﬁeld topology in ﬂuid

mechanics have been presented by Perry et al. (Perry

and Chong, 1987). It was later introduced in visual-

ization by Helman and Hesselink (Helman and Hes-

selink, 1989), (Helman and Hesselink, 1991) again in

the context of ﬂow ﬁelds. For details, we refer the

reader to the work by Globus et al. (Globus et al.,

1991), Asimov (Asimov, 1993), and Abraham and

Shaw (Abraham and Shaw, 1992).

Vector ﬁeld topology did also give rise to derived

concepts and techniques. Examples are saddle con-

nectors (Theisel et al., 2003) and boundary switch

curves (Weinkauf et al., 2004a), both augmenting vec-

tor ﬁeld topology by line-type features. Several ap-

proaches have been proposed for adopting the con-

cepts to time-dependent vector ﬁelds, including the

path line oriented topologies for 2D vector ﬁelds by

Theisel et al. (Theisel et al., 2004) and Shi et al. (Shi

et al., 2006), Galilean-invariant alternatives for criti-

cal points (Kasten et al., 2010), and basics for a La-

grangian vector ﬁeld topology (Fuchs et al., 2010).

Separatrices and LCS are able to convey the struc-

tural and temporal organization of vector ﬁelds, how-

ever, at the same time, they suffer from this prop-

erty; vector direction and magnitude are not repre-

sented, neither along these constructs, nor, and more

importantly, in their vicinity. There are different

approaches to overcome these drawbacks. Critical

points are often visualized with glyphs representing

the linearized ﬂow. Another approach is to augment

separatrices with arrows as presented by Weinkauf et

al. (Weinkauf et al., 2004b). However, the most com-

mon approach in 2D and 3D vector ﬁeld topology is

to combine the separatrices with line integral convolu-

tion (LIC), e.g., see Weiskopf and Ertl (Weiskopf and

Ertl, 2004). This vector ﬁeld visualization with LIC

can be either drawn between separatrices in the case

of 2D vector ﬁelds, or on the separatrices themselves

in the case of 3D vector ﬁelds. For time-dependent

vector ﬁelds, this approach is not applicable because

LIC visualizes transport along streamlines, i.e., in-

stantaneous ﬁeld lines, whereas LCS are usually ob-

tained using true trajectories, i.e., from path lines.

Therefore, other methods are required for visualiz-

ing the advection in the context of LCS, e.g., Shad-

den et al. (Shadden et al., 2005) advect particles. We

exploit the fact that 2D time-dependent vector ﬁelds

can be turned into 3D stationary vector ﬁelds by di-

mension lifting. Since the resulting domain is steady,

streamlines are then equal to path lines, which allows

us to use LIC. Texture-based methods (like LIC) have

the advantage of avoiding the seed point positioning

problem by conveying the ﬁeld structure in a dense

fashion. Since there is no intrinsic, predeﬁned sur-

face parametrization available, image-space oriented

methods like (Laramee et al., 2003), (Wijk, 2003),

and (Weiskopf and Ertl, 2004) are predestined for our

task (we build on the latter one). More background in-

formation on texture-based vector ﬁeld visualization

can be found in the survey of (Laramee et al., 2004).

3 SPACE-TIME LCS

VISUALIZATION

Our new visualization technique builds on the fact

that time-dependent vector ﬁelds can be turned into

stationary ones by treating time as additional dimen-

sion. This approach is common in the ﬁeld of dif-

ferential equations, where non-autonomous systems

are made autonomous. Hence, 2D time-dependent

vector ﬁelds (u(x,y,t),v(x,y,t))

are converted into

steady 3D vector ﬁelds (u(x,y,t),v(x,y,t),1)

, which

we denote as space-time vector ﬁelds. All fol-

lowing steps of our algorithm (see Fig. 4) take

place in this space-time domain. Since 2D path

lines represent streamlines in space-time, we use

3D streamline integration over advection time T in-

side the space-time vector ﬁeld to generate a ﬂow

map φ(x,y,t) 7→ (x

,t + T )

. Then, for each time

SPACE-TIME VISUALIZATION OF DYNAMICS IN LAGRANGIAN COHERENT STRUCTURES OF

TIME-DEPENDENT 2D VECTOR FIELDS

575

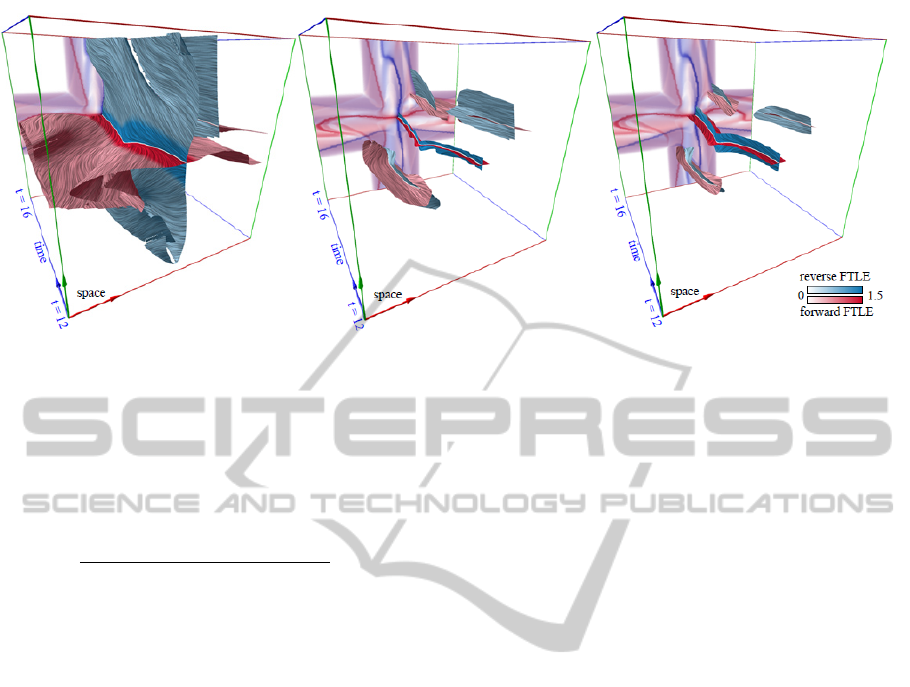
(a) (b) (c)

Figure 3: Building blocks for space-time LCS visualization, shown with the example from Sec. 5.1. Advection time for

forward and reverse FTLE is T = 4s. (a) Space-time LIC qualitatively visualizes LCS dynamics: hyperbolic behavior is

apparent. In addition, hyperbolicity is encoded by color saturation. A minimum FTLE value of 0.5 is used. (b) Intersection

bands by clipping with complementary FTLE reduce occlusion but still provide context and convey structure of reverse

FTLE. The minimum complementary FTLE value is 0.41. (c) Intersection bands by clipping with distance to intersection

curves further reduces occlusion and provides the topological skeleton.

slice

t of the space-time stack we compute the tradi-

tional 2D FTLE according to Haller (Haller, 2001) as

1/|T | ln

max

[(∇

φ(x,y,

t))

∇

φ(x,y,

t)] with ∇

(∂/∂x,∂/∂y,0)

and major eigenvalue λ

max

(·). LCS

are then extracted from the resulting stack of tradi-

tional 2D FTLE ﬁelds by ridge surface extraction, dis-

cussed in Section 3.1.

Due to the discussed material advection property

of LCS, these surfaces represent stream surfaces in

the space-time vector ﬁeld, i.e., they are tangent to the

space-time ﬂow. This allows a direct application of

LIC techniques, which we describe in Section 3.3. By

this, LIC visualizes the dynamics of path lines along

which the LCS are advected, and hence the dynamics

within the LCS. As intersections of stream surfaces

are streamlines, the space-time intersection of these

LCS surfaces from forward and reverse FTLE repre-

sents a counterpart to hyperbolic trajectories. In Sec-

tion 3.2, we address the investigation of these inter-

section curves in terms of hyperbolicity, again based

on LCS. Restricting the LIC visualization to bands

around the intersection curves comprises our second

major contribution, detailed in Section 3.4.

3.1 Ridge Surface Extraction

We extract the ridge surfaces from the stack of 2D

FTLE ﬁelds as height ridges (Eberly, 1996) of codi-

mension one from the 3D space-time FTLE ﬁeld,

according to Sadlo and Peikert (Sadlo and Peikert,

2009). We follow this approach to avoid the prob-

lems that would arise from stitching of the individual

ridge curves from the 2D FTLE ﬁelds. Furthermore,

ridges are typically non-manifold, which would cause

further issues. Since Eberly’s formulation (Eberly,

1996) is local and relies on higher-order derivatives, it

is subject to erroneous solutions. It is therefore com-

mon practice to apply ﬁltering and we follow the ﬁl-

tering process described by Sadlo and Peikert (Sadlo

and Peikert, 2007): since only sufﬁciently “sharp”

FTLE ridges represent LCS, ridge regions where the

modulus of the eigenvalue of the Hessian is too low

are suppressed. Further, we require a minimum FTLE

value, hence requiring a minimum separation strength

of the LCS. Finally, to suppress small ridges, we ﬁl-

ter the ridge surfaces by area. As described in (Sadlo

and Peikert, 2007), we also use a least-squares ap-

proach to prevent noise ampliﬁcation during estima-

tion of the gradient and Hessian. Figure 2 shows ex-

amples of ridges extracted from a stack of forward

and reverse-time FTLE: repelling LCS (ridges in for-

ward FTLE) colored red and attracting ones (ridges

in reverse FTLE) blue. The space-time structure of

the ﬁeld is revealed including the intersection curves.

However, this does not convey hyperbolicity aspects,

e.g., it does not disambiguate intersection curves rep-

resenting strong hyperbolic trajectories from weak

hyperbolic ones. This motivates the visualization of

hyperbolicity on LCS.

3.2 Visualizing Hyperbolicity

To help the user in the investigation of hyper-

bolic effects, and hyperbolic trajectories in partic-

IVAPP 2012 - International Conference on Information Visualization Theory and Applications

576

ular, we map hyperbolicity to saturation, shown in

Fig. 3a. We have chosen the hyperbolicity deﬁnition

by Haller (Haller, 2000), i.e., the sign of the determi-

nant of the velocity gradient of the original 2D vec-

tor ﬁeld at the respective space-time location. One

can see how this technique not only reveals the pres-

ence of hyperbolicity but also allows for the interpre-

tation of the hyperbolic regions around the intersec-

tion curves. To examine hyperbolicity more precisely,

we introduce a novel technique to visualize LCS dy-

namics in the next section.

3.3 Visualizing LCS Dynamics

The LCS in our space-time FTLE ﬁeld are present as

ridge surfaces and to fully capture the spatial varia-

tion of their dynamics they lend themselves to dense

texture-based visualization such as LIC. Since LCS

lack intrinsic surface parametrization and need to be

analyzed in overview scales as well as in local detail,

image-space oriented approaches are predestined to

visualize the space-time structure. We use the hybrid

physical/device-space LIC approach by Weiskopf and

Ertl (Weiskopf and Ertl, 2004), which relies on par-

ticle tracing computed in the physical space of the

object and in the device space of the image plane at

the same time. The LIC pattern is based on the tan-

gential part of the vectors attached to our surfaces.

This dual-space approach combines the advantages of

image-space methods with frame-to-frame coherence

and avoids inﬂow issues at silhouette lines. For a de-

tailed description of this visualization technique, we

refer to the original paper.

In the context of our visualization of LCS dynam-

ics, the goal is to visualize the space-time direction

of the vector ﬁeld. Hence, we normalize the space-

time vectors during LIC computation to obtain LIC

line patterns of uniform length for optimal perception.

In contrast to traditional spatial LIC, we retain the vi-

sual encoding of velocity magnitude in the form of

surface orientation in space-time. For example, small

angles between surface normal and the time axis indi-

cate high speed.

Figure 3a exempliﬁes the method again on the

same data set. It is apparent how this technique con-

veys the time-dependent dynamics within LCS. Com-

bining it with the saturation-based visualization of hy-

perbolicity (Section 3.2) supports the identiﬁcation of

hyperbolic intersection curves and still provides the

LCS dynamics context. Since LCS are often convo-

luted, they typically exhibit many intersections that

are, however, often occluded. We address this prob-

lem by the building block described next: the restric-

tion of the technique to regions around space-time

LCS intersection curves. At the same time, this ap-

proach explicitly addresses the analysis of the inter-

section curves.

3.4 LCS Intersection Bands

Even in the simple example shown so far, it is obvi-

ous that occlusion tends to be a problem in space-time

visualization of LCS. To address this and to provide

a method for analyzing intersection curves of LCS

at the same time, we introduce two complementary

approaches that have proven valuable in our experi-

ments, both restricting the presented visualization to

bands around the LCS intersection curves.

As discussed in Section 3.1, a common approach

is to ﬁlter FTLE ridges by prescribing a minimum

FTLE value. This way, the visualization is restricted

to important LCS, i.e., those representing strong sep-

aration. This ﬁlter is applied to ridges in both forward

and reverse FTLE ﬁelds. If we additionally prescribe

a minimum value for the complementary FTLE, i.e.,

the reverse in case of forward FTLE ridges and the

forward in case of reverse FTLE ridges, one typically

restricts the visualization to bands around the inter-

section curves, shown in Fig. 3b. This technique has

the advantage that the proﬁle of the complementary

FTLE ﬁeld is conveyed, allowing qualitative interpre-

tation of the interplay of LCS. Furthermore, it often

features additional bands that do not exhibit LCS in-

tersections. They are generated if FTLE ridges are lo-

cated in regions of high complementary FTLE. These

additional bands are still of interest: the respective

regions exhibit both high forward and reverse-time

FTLE. Additionally, these bands may connect to other

bands that feature intersection curves and hence con-

vey the overall organization of the LCS. A drawback

of this approach, however, is that the bands may get

too narrow for appropriate LIC visualization or too

wide to sufﬁciently reduce occlusion.

Therefore, we propose, as an alternative, to re-

strict the LCS to the neighborhood of their intersec-

tion curves. To avoid numerical issues, we ﬁrst omit

regions where the LCS intersect at small angle.

Furthermore, a minimum length of the intersec-

tion curves is required to obtain signiﬁcant visual-

izations. The remaining intersection curves are then

used for distance computation, leading to a distance

ﬁeld on the LCS that is then used for clipping. Fig-

ure 3c shows an example: the dynamics of the LCS is

well depicted by LIC and at the same time occlusion

is substantially reduced, allowing for the analysis of

the intersection curves with respect to LCS dynamics

and hyperbolicity. Since the resulting bands can still

be too narrow due to perspective foreshortening, we

SPACE-TIME VISUALIZATION OF DYNAMICS IN LAGRANGIAN COHERENT STRUCTURES OF

TIME-DEPENDENT 2D VECTOR FIELDS

577

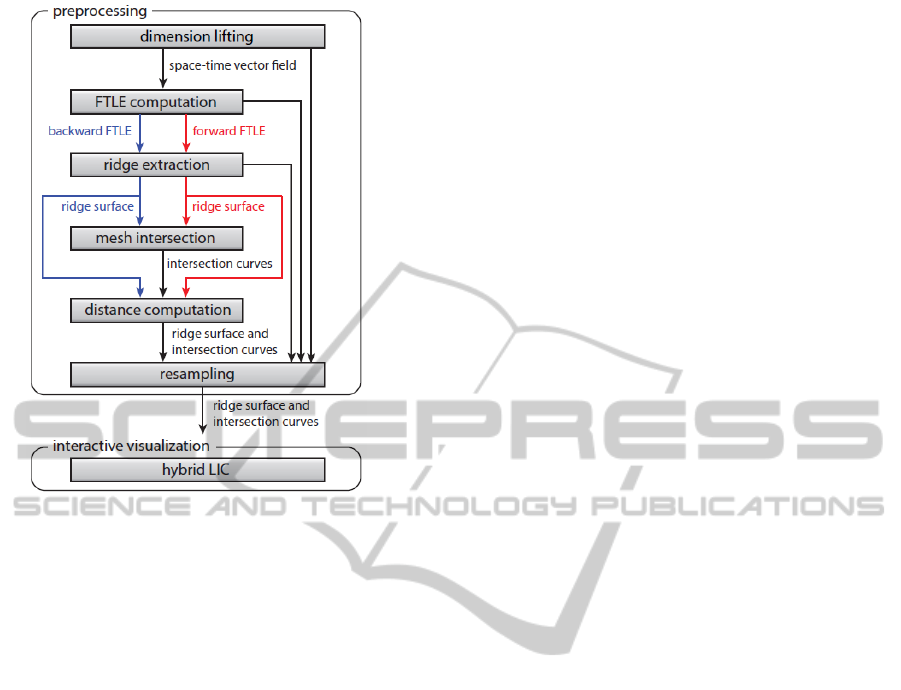
Figure 4: Overview of our technique, accompanied by the

data that are passed between the stages of the pipeline.

also support depth-corrected width of the bands de-

scribed in Section 4.2.

To sum up, these clipping approaches result in vi-

sualizations that can be seen as an extended topologi-

cal skeleton of time-dependent ﬂow. Note that we use

equal thresholds for forward and reverse-time FTLE

ridge ﬁltering as well as for complementary FTLE

band clipping, for ensuring consistent visualization.

Finally, we would like to point out the similarity to

saddle connectors (Theisel et al., 2003), although our

approach resides in space-time, whereas saddle con-

nectors visualize 3D steady vector ﬁelds.

3.5 Combined Visualization

Our system allows the user to interactively switch on

and off the clipping for intersection bands. When

clipping is enabled, the remaining choice is between

complementary FTLE and distance-based intersec-

tion bands. Depth-correction of intersection band

width is always enabled. In summary, only three dif-

ferent modes (no clipping, complementary clipping,

distance-based clipping) are required to cover the vi-

sualization needs.

4 IMPLEMENTATION

This section details the implementation of the differ-

ent building blocks of our technique as well as modiﬁ-

cations to existing approaches. The pipeline shown in

Fig. 4 gives an overview of the steps and provides in-

formation about the data that are exchanged between

different stages of the pipeline.

4.1 Preprocessing

Several steps in our technique are performed in a pre-

processing phase, once per data set.

The original data set is given as a series of time

steps of a 2D vector ﬁeld. To create the stationary

space-time 3D vector ﬁeld, we apply dimension lift-

ing, i.e., the time series of the 2D vector ﬁeld are

stacked and the time dimension is treated as addi-

tional third dimension. This space-time vector ﬁeld

is used to compute the 3D space-time FTLE ﬁeld for

forward and reverse time direction. Using this FTLE

ﬁeld, ridge surfaces are extracted. A detailed descrip-

tion of the ridge extraction method is given by Sadlo

and Peikert (Sadlo and Peikert, 2007).

The ridge surface meshes from forward and

reverse–time FTLE are intersected to obtain the in-

tersection curves. Once the geometry of all intersec-

tion curves is obtained, a distance ﬁeld is computed

that holds the distance of ridge surface vertices to the

nearest intersection curve. Next, we compute vertex-

based normals, which are used for shading in the in-

teractive visualization. During this process, normals

are reoriented if necessary; however, since ridge sur-

faces are not necessarily orientable, we may not suc-

ceed for all normals. Remaining inconsistencies for

the normals are treated during interactive visualiza-

tion using a shader. Finally, the space-time ﬂow vec-

tors are sampled at the vertex locations of the ridge

surface mesh. This resampling is independent of the

FTLE sampling grid, allowing for acceleration meth-

ods (Garth et al., 2007), (Sadlo and Peikert, 2007),

and (Hlawatsch et al., 2011). Distance values, nor-

mals, resampled ﬂow vectors, and additional scalars

like FTLE values, hyperbolicity, and the minor eigen-

value of the Hessian (see Section 3.1) are attached to

the ridge surface mesh that is then passed to the inter-

active visualization stage.

4.2 Interactive Visualization

The core of our interactive visualization is based on

hybrid physical/device-space LIC (Weiskopf and Ertl,

2004) to create line-like texture on the ridge surfaces.

During rendering of the space-time ridge surfaces,

we apply Phong illumination to enhance visibility and

perception of the geometry. Since the ridge surfaces

may be non-orientable, we have to ensure that local

IVAPP 2012 - International Conference on Information Visualization Theory and Applications

578

normal vectors are consistently oriented in order to

avoid shading artifacts. Therefore, we make normal

orientation consistent during fragment processing us-

ing the dot product between normal and view vec-

tor. This prevents inconsistent shading due to nor-

mal interpolation; however, ridge surfaces may still

appear rippled. This happens because of FTLE alias-

ing effects at strong and sharp ridges, where very

high FTLE gradients are present. To compensate for

this, we correct the normals to be perpendicular to the

space-time vector ﬁeld and hence to its LCS during

fragment processing.

We handle occlusion by attaching additional data

(regular FTLE, complementary FTLE, distance to

nearest intersection curve) obtained during the pre-

processing stage (see Section 4.1) to each vertex of

the ridge surface mesh and upload this data as ad-

ditional texture coordinates to the GPU. Fragments

that do not meet the ﬁltering criteria are discarded.

All thresholds used in this process are adjustable in

real-time by the user. In addition to user controlled

clipping, we adjust the width of our LCS intersection

bands if they are clipped by the distance to the nearest

intersection curve. We adjust the clipping threshold

based on distance to the camera position. This re-

sults in intersection bands with constant image-space

width, which reduces occlusion of intersection bands

that are close to the camera. At the same time, in-

tersection bands that are farther away are enlarged,

which improves visibility of the LIC pattern.

5 RESULTS

We apply the presented methods to different data sets.

The ﬁrst two data sets are synthetic, whereas the third

is created by CFD simulation, and the fourth is ob-

tained by remote sensing of ocean currents.

Our implementation was tested on a PC with an

Intel Core Quad CPU (2.4 GHz), 4 GB of RAM and

an NVIDIA GeForce 275 GPU with 896 MB of ded-

icated graphics memory. Each of the presented data

sets is visualized at interactive rates. Since our im-

plementation is based on the approach presented by

Weiskopf and Ertl (Weiskopf and Ertl, 2004), it shows

the same performance behavior—we refer to their pa-

per for a detailed performance analysis.

A bounding box of the domain helps the user to

navigate and orientate in space-time. This bounding

box is color coded—the time dimension is indicated

by a blue axis while the two spatial dimensions have a

red and green axis, respectively. The last time step of

the space-time region of interest is located at the back

end of the bounding box which shows the FTLE ﬁeld

(a) (b)

Figure 5: (a) Gyre Saddle example at t = 0. (b) Quad Gyre

example at t = 0.

as a color–coded texture. In this texture, FTLE values

are mapped to saturation, with full saturation mapping

to the highest FTLE value. There, we use the same

color-coding as for the space-time ridge surfaces.

5.1 Oscillating Gyre Saddle

The synthetic vector ﬁeld that we use as an example

in this section is due to Sadlo and Weiskopf (Sadlo

and Weiskopf, 2010). It exhibits a non-linear sad-

dle (Fig. 5a) that oscillates between the locations

(0.25,0.25) and (−0.25, −0.25) at a period of τ = 4.

Please refer to Fig. 1, Fig. 2, and Fig. 3 for resulting

visualizations. To sum up, it exhibits a strongly hy-

perbolic intersection curve visualized in Fig. 1 (right)

and several non-hyperbolic ones. This is consistent

with the Eulerian picture (Fig. 5a) showing distin-

guished saddle behavior at its center. As mentioned

by Sadlo and Weiskopf (Sadlo and Weiskopf, 2010),

there are other ridges due to shear processes. These

are of inferior importance for mixing and cannot give

rise to hyperbolic trajectories, i.e., their LIC patterns

do not show hyperbolic behavior. Note that we ﬁlter

FTLE ridges to show the strongest and largest LCS.

Supressing weaker ridges simpliﬁes the resulting vi-

sualization which we use for depicting purposes.

(a) (b) (c)

Figure 6: Three time steps of the buoyant plume exam-

ple, color indicates temperature (red maps to high temper-

ature, blue to low temperature). (a) Two plumes build up

and travel toward each other in vertical direction. (b) After

collision, two new plumes are created that travel toward the

walls. (c) After collision with the side walls.

SPACE-TIME VISUALIZATION OF DYNAMICS IN LAGRANGIAN COHERENT STRUCTURES OF

TIME-DEPENDENT 2D VECTOR FIELDS

579

(a) (b) (c)

Figure 7: Quad-Gyre example. The advection time for forward and reverse FTLE is T = 7.5s. (a) Full visualization of

forward and reverse LCS. A lower FTLE threshold of 0.4 is used. (b) Visualization restricted to complementary FTLE bands.

Minor artifacts appear due to aliasing effects of forward and reverse FTLE. The minimum complementary FTLE value is

0.19. (c) Restriction to distance-based LCS intersection bands reveals the topological space-time skeleton.

(a) (b)

Figure 8: Buoyant plumes example. The advection time for forward and reverse FTLE is T = 1.5s. (a) Full visualization of

forward and reverse LCS. The dynamics of the two plumes is apparent in the ﬁrst part of the examined time interval. A lower

FTLE threshold of 0.87 is used. (b) LCS intersection bands clipped by distance, revealing the skeleton.

5.2 Quad Gyre

The double gyre example was introduced by Shad-

den et al. (Shadden et al., 2005) to examine FTLE

and LCS, and to compare them to vector ﬁeld topol-

ogy. It consists of two vortical regions separated by a

straight separatrix that connects two saddle-type crit-

ical points: one oscillating horizontally at the upper

edge and the other synchronously oscillating horizon-

tally along the lower edge. This is a prominent ex-

ample where the vector ﬁeld topology result substan-

tially differs from LCS. This data set is temporally

periodic. To avoid boundary issues, we use a larger

range of ﬁeld, resulting in four gyres. As proposed

by Shadden et al., we use the conﬁguration ε = 1/4,

ω = π/5, and A = 1/10. Figure 5b shows a plot at

t = 0 for these parameters.

Rendering the quad gyre without clipping (Fig. 7a)

results in space-time ridges that heavily occlude each

other. Please note that the y = 0 plane represents an

LCS in both forward and reverse direction, which re-

sults in z-ﬁghting. Nevertheless, the LIC line pattern

is consistent in that region due to the image-based

LIC technique. Reducing occlusion by clipping with

the complementary FTLE (Fig. 7b) removes parts of

the ridge surfaces, while preserving the context of the

bands. Note, for example, that the red bands are con-

nected at the upper edge of the domain and hence

are part of the same LCS. If we clip the space-time

ridge surfaces by distance to their intersection curves

(Fig. 7c), occlusion is even more reduced, but less

context is conveyed. However, this technique espe-

cially pays off in data sets with complex space-time

dynamics, since the topological skeleton is well visi-

IVAPP 2012 - International Conference on Information Visualization Theory and Applications

580

(a) (b)

Figure 9: OSCAR example. The advection time for forward and reverse FTLE is T = 25 days. The small map on the left

hand side shows the atlantic ocean and the east coast of North America. It gives a frame of reference for our visualization

results and exempliﬁes the prevalent mixing due to the gulf stream. Please note that this map does not show ﬂow but rather

water temperature mapped to colors and that it was generated outside of the investigated time interval. (a) Full visualization

of forward and reverse LCS. A lower FTLE threshold of 9 × 10

−7

is used. Flow around several intersection curves shows

strong hyperbolic behavior. (b) LCS intersection bands clipped by distance.

ble from most views. In all images, hyperbolicity is

visualized by mapping it to the saturation of the ridge

surface color. It can be seen that it readily guides at-

tention to hyperbolic LIC patterns. As in the results

by Sadlo and Weiskopf (Sadlo and Weiskopf, 2010),

we identify a hyperbolic trajectory at the center of the

data set.

5.3 Buoyant Plumes

This data was obtained by a CFD simulation of buoy-

ant 2D ﬂow. A square container was modeled with

a small heated region at its bottom wall and a small

cooled region at its top wall. Figure 6 illustrates the

ﬂow. Two plumes are developed, a hot one rising to

the top and a cold one moving in reverse direction to

the bottom. They then collide at the center and give

rise to two new plumes traveling horizontally toward

the side walls. As they approach the walls, they both

split and produce plumes traveling in vertical direc-

tion. From that point on, the regular behavior is re-

placed by increasingly turbulent ﬂow behavior.

Figure 8a shows the visualization of both forward-

and reverse-time FTLE ridges. There is no clipping

applied for this image but saturation already guides

to the hyperbolic regions, however, many of them are

occluded. In Fig. 8b, the distance-based LCS inter-

section bands nicely visualize the hyperbolic mech-

anisms. One can see how the two plumes approach

each other and merge, then divide and later give rise

to turbulent ﬂow. We ﬁnally identify several strong

hyperbolic regions toward the end of the examined

time interval. The multitude of hyperbolic regions ap-

proves the observation of strong buoyant mixing. The

high intricacy and topological complexity of turbulent

buoyant ﬂow reﬂects in our visualization.

5.4 OSCAR

Ocean Surface Currents Analyses Real-time (OS-

CAR) (Bonjean and Lagerloef, 2002) is a project to

calculate ocean surface velocities from satellite data.

The OSCAR product is a direct computation of global

surface currents using satellite sea surface height,

wind, and temperature. The OSCAR analyses have

been used extensively in climate studies, such as for

ocean heat storage and phytoplankton blooms.

We applied our technique to the gulf stream at

the east coast of North America. We thereby focused

on a strong hyperbolic LCS system involved in mix-

ing (Fig. 9a). As expected, our technique revealed a

complex Lagrangian skeleton of turbulence (Mathur

et al., 2007), shown in Fig. 9a. Our LIC patterns

allow a direct and qualitative inspection of the LCS

with respect to hyperbolic mechanisms and mixing.

Whereas many regions in the OSCAR data set ex-

hibited inferior hyperbolic behavior, it is prominent

in the selected region. Again, the LCS intersection

SPACE-TIME VISUALIZATION OF DYNAMICS IN LAGRANGIAN COHERENT STRUCTURES OF

TIME-DEPENDENT 2D VECTOR FIELDS

581

bands dramatically reduce occlusion while still con-

veying topological structure and hyperbolic dynam-

ics, see Fig. 9b. Following the LIC line patterns along

the temporal axis directly conveys the action of the

ﬂow in terms of mixing, i.e., thinning and folding.

6 CONCLUSIONS

We have presented an approach for the visualiza-

tion and analysis of the dynamics in LCS of time-

dependent 2D vector ﬁelds. Compared to traditional

approaches, we do not restrict the investigation of

LCS to their geometric shape. We extend the visu-

alization by allowing the user to analyze the intrin-

sic dynamics of LCS in terms of stretching and com-

pression, in particular along hyperbolic trajectories.

These dynamics are visualized by space-time LIC on

space-time ridge surfaces of the 2D FTLE.

Occlusion problems due to convoluted and heav-

ily intersecting LCS are reduced by clipping of the

LCS, providing LCS intersection bands. Clipping can

be based on the distance to the hyperbolic trajecto-

ries and on forward and reverse FTLE to suppress less

important regions. A major numerical aspect of our

method is the avoidance of the difﬁcult direct inte-

gration of hyperbolic trajectories, we intersect FTLE

ridge space-time surfaces instead. Still, the growth

of the respective space-time streak manifolds is con-

veyed by the LIC.

Finally, we have demonstrated the applicability of

our method with several synthetic and real-world data

sets, also in the context of turbulent ﬂow analysis, a

topic of ongoing research. In future work, we plan

to extend our technique to 3D time-dependent vector

ﬁelds, i.e., investigate intersection curves of LCS and

the surfaces they span over time.

ACKNOWLEDGEMENTS

The ﬁrst author and fourth author thank the Ger-

man Research Foundation (DFG) for ﬁnancial sup-

port within SFB 716 / D.5 at University of Stuttgart.

The second author thanks DFG for ﬁnancial support

within the Cluster of Excellence in Simulation Tech-

nology (EXC 310/1), and SFB-TRR 75 at University

of Stuttgart.

REFERENCES

Abraham, R. H. and Shaw, C. D. (1992). Dynamics, the

Geometry of Behavior. 2nd ed. Addison-Wesley.

Asimov, D. (1993). Notes on the topology of vector ﬁelds

and ﬂows. Technical Report RNR-93-003, NASA

Ames Research Center.

Bonjean, F. and Lagerloef, G. (2002). Diagnostic model

and analysis of the surface currents in the tropical

paciﬁc ocean. Journal of Physical Oceanography,

32(10):2938–2954.

Eberly, D. (1996). Ridges in Image and Data Analysis.

Computational Imaging and Vision. Kluwer Aca-

demic Publishers.

Fuchs, R., Kemmler, J., Schindler, B., Sadlo, F., Hauser,

H., and Peikert, R. (2010). Toward a Lagrangian

vector ﬁeld topology. Computer Graphics Forum,

29(3):1163–1172.

Garth, C., Gerhardt, F., Tricoche, X., and Hagen, H.

(2007). Efﬁcient computation and visualization of

coherent structures in ﬂuid ﬂow applications. IEEE

Transactions on Visualization and Computer Graph-

ics, 13(6):1464–1471.

Globus, A., Levit, C., and Lasinski, T. (1991). A tool for

visualizing the topology of three-dimensional vector

ﬁelds. In Proc. of IEEE Visualization, pages 33–41.

Haller, G. (2000). Finding ﬁnite-time invariant manifolds

in two-dimensional velocity ﬁelds. Chaos, 10(1):99–

108.

Haller, G. (2001). Distinguished material surfaces and

coherent structures in three-dimensional ﬂuid ﬂows.

Physica D Nonlinear Phenomena, 149(4):248–277.

Helman, J. and Hesselink, L. (1989). Representation and

display of vector ﬁeld topology in ﬂuid ﬂow data sets.

IEEE Computer, 22(8):27–36.

Helman, J. and Hesselink, L. (1991). Visualizing vector

ﬁeld topology in ﬂuid ﬂows. IEEE Computer Graph-

ics and Applications, 11(3):36–46.

Hlawatsch, M., Sadlo, F., and Weiskopf, D. (2011). Hierar-

chical line integration. IEEE Transactions on Visual-

ization and Computer Graphics, 17(8):1148 –1163.

Ide, K., Small, D., and Wiggins, S. (2002). Distinguished

hyperbolic trajectories in time-dependent ﬂuid ﬂows:

Analytical and computational approach for velocity

ﬁelds deﬁned as data sets. Nonlinear Processes in

Geophysics, 9(3/4):237–263.

Kasten, J., Hotz, I., Noack, B., and Hege, H.-C. (2010).

On the extraction of long-living features in unsteady

ﬂuid ﬂows. In Pascucci, V., Tricoche, X., and Terny,

J., editors, Topological Methods in Data Analysis

and Visualization. Theory, Algorithms, and Applica-

tions., Mathematics and Visualization, pages 115–

126. Springer.

Laramee, R. S., Hauser, H., Doleisch, H., Vrolijk, B., Post,

F. H., and Weiskopf, D. (2004). The state of the art

in ﬂow visualization: Dense and texture–based tech-

niques. Computer Graphics Forum, 23(2):203–221.

Laramee, R. S., Jobard, B., and Hauser, H. (2003). Im-

age space based visualization of unsteady ﬂow on sur-

faces. In Proc. IEEE Visualization, pages 131–138.

Lekien, F., Coulliette, C., Mariano, A. J., Ryan, E. H., Shay,

L. K., Haller, G., and Marsden, J. E. (2005). Pollution

release tied to invariant manifolds: A case study for

IVAPP 2012 - International Conference on Information Visualization Theory and Applications

582

the coast of Florida. Physica D Nonlinear Phenom-

ena, 210(1):1–20.

Mathur, M., Haller, G., Peacock, T., Ruppert-Felsot, J. E.,

and Swinney, H. L. (2007). Uncovering the La-

grangian skeleton of turbulence. Physical Review Let-

ters, 98(14):144502.

Perry, A. E. and Chong, M. S. (1987). A description of

eddying motions and ﬂow patterns using critical-point

concepts. Annual Review of Fluid Mechanics, 19:125–

155.

Sadlo, F. and Peikert, R. (2007). Efﬁcient visualization of

Lagrangian coherent structures by ﬁltered AMR ridge

extraction. IEEE Transactions on Visualization and

Computer Graphics, 13(5):1456–1463.

Sadlo, F. and Peikert, R. (2009). Visualizing Lagrangian co-

herent structures and comparison to vector ﬁeld topol-

ogy. In Topology-Based Methods in Visualization II,

pages 15–30.

Sadlo, F., Rigazzi, A., and Peikert, R. (2011). Time–

dependent visualization of Lagrangian coherent struc-

tures by grid advection. In Topological Methods

in Data Analysis and Visualizationc (Proceedings of

TopoInVis 2009), pages 151–165. Springer.

Sadlo, F.,

Ufﬁnger, M., Ertl, T., and Weiskopf, D. (2012).

On the ﬁnite–time scope for computing Lagrangian

coherent structures from Lyapunov exponents. In

Topological Methods in Data Analysis and Visualiza-

tion II (Proceedings of TopoInVis 2011), pages 269–

281. Springer.

Sadlo, F. and Weiskopf, D. (2010). Time–dependent 2D

vector ﬁeld topology: An approach inspired by La-

grangian coherent structures. Computer Graphics Fo-

rum, 29(1):88–100.

Shadden, S., Lekien, F., and Marsden, J. (2005). Deﬁnition

and properties of Lagrangian coherent structures from

ﬁnite-time Lyapunov exponents in two-dimensional

aperiodic ﬂows. Physica D Nonlinear Phenomena,

212(3):271–304.

Shi, K., Theisel, H., Weinkauf, T., Hauser, H., Hege, H.-C.,

and Seidel, H.-P. (2006). Path line oriented topology

for periodic 2D time–dependent vector ﬁelds. In Proc.

Eurographics / IEEE VGTC Symposium on Visualiza-

tion, pages 139–146.

Theisel, H., Weinkauf, T., Hege, H.-C., and Seidel, H.-P.

(2003). Saddle connectors - An approach to visual-

izing the topological skeleton of complex 3D vector

ﬁelds. In Proc. IEEE Visualization, pages 225–232.

Theisel, H., Weinkauf, T., Hege, H.-C., and Seidel, H.-P.

(2004). Stream line and path line oriented topology

for 2D time–dependent vector ﬁelds. In Proc. IEEE

Visualization, pages 321–328.

Weinkauf, T., Theisel, H., Hege, H.-C., and Seidel, H.-P.

(2004a). Boundary switch connectors for topological

visualization of complex 3D vector ﬁelds. In Proc.

Joint Eurographics - IEEE TCVG Symposium on Vi-

sualization (VisSym ’04), pages 183–192.

Weinkauf, T., Theisel, H., Hege, H.-C., and Seidel, H.-P.

(2004b). Topological construction and visualization

of higher order 3D vector ﬁelds. In Computer Graph-

ics Forum, pages 469–478.

Weiskopf, D. and Ertl, T. (2004). A hybrid physical/device-

space approach for spatio-temporally coherent inter-

active texture advection on curved surfaces. In Proc.

Graphics Interface, pages 263–270.

Wiebel, A., Tricoche, X., Schneider, D., Jaenicke, H., and

Scheuermann, G. (2007). Generalized streak lines:

Analysis and visualization of boundary induced vor-

tices. IEEE Transactions on Visualization and Com-

puter Graphics, 13(6):1735–1742.

Wijk, J. J. V. (2003). Image based ﬂow visualization for

curved surfaces. In Proc. IEEE Visualization, pages

123–130.

SPACE-TIME VISUALIZATION OF DYNAMICS IN LAGRANGIAN COHERENT STRUCTURES OF

TIME-DEPENDENT 2D VECTOR FIELDS

583