
Measuring Musical Rhythm Similarity
Statistical Features versus Transformation Methods
J. F. Beltran, X. Liu, N. Mohanchandra and G. T. Toussaint
Faculty of Science, New York University Abu Dhabi, Abu Dhabi, U.A.E.
Keywords: Musical Rhythm, Similarity Measures, Transformations, Inter-Onset Interval Histograms, Mallows
Distance, Edit Distance, Statistical Features, Pattern Recognition, Music Information Retrieval, Mantel Test.
Abstract: Two approaches to measuring the similarity between symbolically notated musical rhythms are compared
with human judgments of perceived similarity. The first is the edit-distance, a popular transformation
method, applied to the rhythm sequences. The second works on the histograms of the inter-onset-intervals
(IOIs) of these rhythm sequences. Furthermore, two methods of dealing with the histograms are also
compared: the Mallows distance, and the employment of a group of standard statistical features. The results
provide further evidence from the aural domain, that transformation methods are superior to feature-based
methods for predicting human judgments of similarity. Furthermore, the results also support the hypothesis
that statistical features applied to the histograms of the rhythms are better than music-theoretical structural
features applied to the rhythms themselves.
1 INTRODUCTION
A fundamental problem in many scientific domains
is the measurement of similarity between a pair of
objects (Toussaint, 2013). There are two general
approaches to tackling this problem that have
received much attention in the literature: feature-
based methods and transformation-based
procedures. In feature-based methods a collection of
d features (measurements) is first calculated for each
object. Then the dissimilarity between two objects is
defined as the distance between their corresponding
feature vectors (Duda et al., 2000). The
transformation-based techniques on the other hand
measure similarity between two objects by the
minimum amount of work (suitably defined) that is
required to transform one object into the other.
Experimental evidence and consensus has been
accumulating, for at least a decade, which suggests
that in the visual domain, the transformation
methods appear to be superior to the feature-based
methods (Hahn et al., 2003). One such method
utilized in many pattern recognition applications is
the edit distance (also known as the Levenshtein
distance). Experiments have suggested that the edit
distance is a good predictor of human perceptual
judgments of rhythm similarity (Toussaint et al.,
2011); (Post and Toussaint, 2011). Furthermore, a
recent comparison of the edit distance with a
feature-based measure that employed a collection of
structural features common in music theory and
ethnomusicology for the purpose of classification,
has highlighted the superiority of the edit distance,
and has thus added support from the auditory
domain to the growing consensus of the advantages
of transformation methods observed in the visual
domain (Toussaint et al., 2012).
Here two approaches to measuring the similarity
between symbolically notated musical rhythms are
compared with each other and with human
judgments of perceived similarity: the feature-based
approach and the transformation method. In the
feature-based approach, unlike the music-theoretical
structural features used by Toussaint et al., (2012),
the features employed here are statistical features
computed from the Inter-Onset-Interval (IOI)
histograms of the rhythms, as was done with
acoustic input by Gouyon et al., (2004). For the
transformation approach we used two methods. The
first calculates the edit-distance, used frequently in
previous studies, directly on the rhythm sequences,
and the second calculates the Mallows distance from
the IOI-histograms (Levina and Bickel, 2001).
595
Beltran J., Liu X., Mohanchandra N. and Toussaint G. (2013).
Measuring Musical Rhythm Similarity - Statistical Features versus Transformation Methods.
In Proceedings of the 2nd International Conference on Pattern Recognition Applications and Methods, pages 595-598
DOI: 10.5220/0004325505950598
Copyright
c
SciTePress

2 TRANSFORMATION
METHODS
The Mallows distance (Levina and Bickel, 2001) is
closely related to the earth-mover's and
transportation distances and is quite complicated in a
general setting. In this study we use it in the context
of binary rhythm sequences all of which have the
same numbers of pulses and onsets. This implies that
the histograms of the IOIs have the same number of
bins. In this case the Mallows distance is simple and
may be computed efficiently in O(d) time, where d
is the cardinality of the two histograms being
compared.
The edit distance measures the least amount of
work needed to convert one rhythm to another by the
minimum number of basic operations (insertions,
deletions, and substitutions of symbols) that are
necessary to accomplish the task. An insertion of a
symbol into a sequence lengthens the sequence by
one symbol, a deletion shortens the sequence
accordingly, and a substitution exchanges one
symbol for another without altering its length.
3 STRUCTURAL FEATURES
Toussaint et al., (2012) compared the edit distance to
a feature-based method that used a group of 14
structural features that are frequently employed for
rhythm analysis and classification in music theory
and ethnomusicology. The data they used consisted
of the nine 8-pulse rhythms shown in Table 1. Thus
each rhythm was converted into a 14-dimensional
feature vector, and the dissimilarity between two
rhythms was measured by the 1st-order Minkowski
metric between their feature vectors. The listening
tests they performed with human subjects
demonstrated that the edit distance was successful at
predicting human judgments, whereas the feature-
based method fared quite poorly.
4 STATISTICAL FEATURES
The statistical features used here included the eight
features calculated from the IOI histograms that
were previously investigated by Gouyon et al.,
(2004) in the acoustic domain, and five additional
features calculated from the IOI values themselves.
The latter five features consisted of the shortest, the
longest, the range, the standard deviation, and the
Normalized Pairwise Variability index (nPVI). The
nPVI measures the directional change of the IOIs as
they occur in a sequence. Unlike the standard
deviation, which treats IOIs in isolation, the nPVI
measures the deviations between adjacent IOIs. It
was originally proposed for the analysis of
variability in speech rhythms using vocalic lengths.
More recently it has been explored as a general tool
in musical rhythm analysis (Toussaint, 2012).
5 THE RHYTHM DATA SETS
The experiments were done with two data sets that
had been used in previous studies. The first data set
consisted of six 16-pulse, 5-onset distinguished
Afro-Cuban timelines shown in box notation in
Table 1.
Table 1: The six 5-onset, 16-pulse rhythms used.
Rhythm Name Binary Box Notation
Shiko
× − − − × − × − − − × − × − − −
Son
× − − × − − × − − − × − × − − −
Soukous
× − − × − − × − − − × × − − − −
Rumba
× − − × − − − × − − × − × − − −
Bossa-Nova
× − − × − − × − − − × − − × − −
Gahu
× − − × − − × − − − × − − − × −
Table 2: The nine 8-pulse rhythms used.
Rhythm Name Binary Box Notation
2-3-3
× − × − − × − −
3-2-3
× − − × − × − −
Cinquillo-Variant
× − × × − × × ×
Cinquillo
× − × × − × × −
Conga
× − − × − − − −
Contradanza
× − × × × × × −
Habanera
× − − × × − × −
Tango-Congo
× − − × × − − −
Tresillo
× − − × − − × −
In this notation the symbols "×" and "−" stand for a
sounded unit-time pulse and a silent unit-time pulse,
respectively. These six rhythms had been used
previously to compare human judgments with the
edit distance (Toussaint et al., 2011). The second
data set consisted of nine Afro-Cuban rhythms of
eight pulses each, with onsets varying between two
and six, shown in box notation in Table 2, that had
been used in a former study to compare the edit
distance with the group of distinguished structural
features taken from music theory (Toussaint et al.,
2012).
ICPRAM2013-InternationalConferenceonPatternRecognitionApplicationsandMethods
596
6 RESULTS
To compare the various similarity measures with
each other and with human judgments, the
dissimilarity between every pair of rhythms in the
data was first calculated, obtaining a distance matrix.
Then a statistical procedure called the Mantel test
was used for calculating the correlation coefficients
between pairs of these distance matrices. The results
of the Mantel tests for the 16-pulse and 8-pulse
rhythms are listed in Tables 3 and 4, respectively,
where the statistically significant results are shown
in boldface type, and the asterisk indicates results
that were obtained in previous studies. Space
limitations do not permit the duplication here of the
description of the human listening tests performed
(for this see Toussaint et al., 2011).
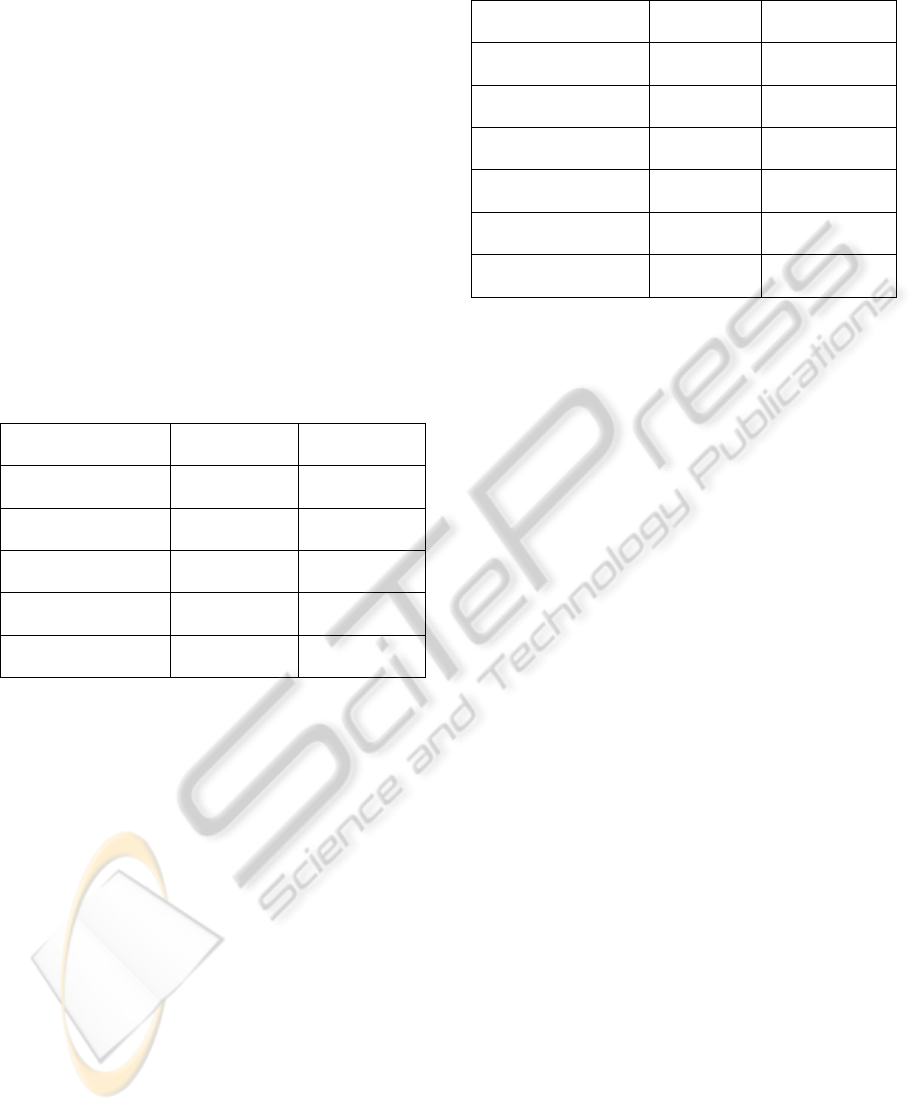
Table 3: Mantel test results for the 16-pulse rhythms.
Human
Judgment
Edit Distance
Statistical Features
r = -0.07
p = 0.47
r = -0.14
p = 0.28
Stat. Features and
nPVI
r = 0.02
p = 0.44
r = -0.09
p = 0.42
nPVI only
r = 0.24
p = 0.21
r = 0.07
p = 0.43
Normalized
Mallows Distance
r = 0.70
p = 0.02
r = 0.35
p = 0.2
Edit Distance*
r = 0.76
p = 0.02
−
Of all the experiments performed with the 16-pulse
rhythms, only the Mallows distance gave statistically
significant results, correlating highly with human
judgments (r = 0.70, p = 0.02). This is almost as
high as the previous result obtained with the edit
distance (r = 0.76, p = 0.02) calculated directly on
the rhythms themselves (Toussaint et al., 2011).
Note that in this corpus all the rhythms have the
same number of onsets, and therefore corpus
normalization is equivalent to pairwise
normalization.
By contrast with the 16-pulse rhythms, all the
experiments with the 8-pulse rhythms, yielded mild
but statistically significant correlations with human
judgments. The nPVI, a successful measure of
rhythm complexity (Toussaint, 2012), gave the
lowest correlation (r = 0.25, p = 0.04) when used in
isolation, and all the other models yielded
correlation coefficients ranging between 0.48 and
0.43. This represents a significant drop from the
previously obtained result with the edit distance (r =
0.59, p = 0.0002) when calculated directly on the
Table 4: Mantel test results for the 8-pulse rhythms.
Human
Judgment
Edit Distance
Statistical Features
r = 0.43
p = 0.006
r = 0.57
p = 0.003
Stat. Features and
nPVI
r = 0.46
p = 0.003
r = 0.57
p = 0.003
nPVI only
r = 0.25
p = 0.04
r = -0.05
p = 0.44
Corpus-Normalized
Gen. Mallows Dist.
r = 0.45
p = 0.003
r = 0.41
p = 0.03
Pairwise-Normalized
Gen. Mallows Dist.
r = 0.48
p = 0.001
r = 0.21
p = 0.1
Edit Distance*
r = 0.59
p = 0.0002
−
rhythm sequences (Toussaint et al., 2012). In this
corpus the number of onsets in the rhythms varies
considerably, and therefore the results with the
corpus and pairwise normalizations differ a little.
Surprisingly, the statistical features calculated from
the IOI histograms correlate quite highly with the
edit distance.
7 CONCLUSIONS
One of the main conclusions we can draw from this
study is that the statistical features calculated from
the inter-onset interval histograms, used by Gouyon
et al. (2004) in the context of music information
retrieval, are much better than the music-theoretical
structural features investigated previously by
Toussaint et al., 2012), for predicting human
judgments of rhythm similarity. The Mallows
distance computed from the IOI histograms gave the
best results obtained here, providing further
evidence to support the hypothesis that
transformation methods are superior to feature-based
methods as tools for predicting human judgments of
similarity.
REFERENCES
Duda, R. O., Hart, P. E., & Stork, D. G., 2000. Pattern
Classification, Wiley-Interscience, 2
nd
Edition.
Gouyon, F., Dixon, S., Pampalk, E., & Widmer, G., 2004.
Evaluating rhythmic descriptors for musical genre
classification. Proc. 25th Int. AES Conference.
Hahn, U., Chater, N., & L. B. Richardson, L. B., 2003.
Similarity as transformation. Cognition, 87, 1-32.
Levina, E. & Bickel, P. (2001). The earth mover’s distance
is the Mallows distance: Some insights from statistics.
Proceedings Eighth IEEE International Conference on
MeasuringMusicalRhythmSimilarity-StatisticalFeaturesversusTransformationMethods
597

Computer Vision, Vancouver, Canada, 2, 251-256.
Post, O. & Toussaint, G. T. 2011. The edit distance as a
measure of perceived rhythmic similarity. Empirical
Musicology Review, 6, 3, 164-179.
Toussaint, G. T., 2013. The Geometry of Musical Rhythm.
Chapman-Hall/CRC Press.
Toussaint, G. T., 2012. The pairwise variability index as a
tool in musical rhythm analysis. Proc. 12th Int. Conf.
Music Perception and Cognition (ICMPC),
Thessaloniki, Greece, July 23-28, 1001-1008.
Toussaint, G. T., Mathews, L., Campbell, M., & Brown,
N., 2012. Measuring musical rhythm similarity:
Transformation versus feature-based methods, (in
preparation).
Toussaint, G. T., Campbell, M., & Brown, N., 2011.
Computational models of symbolic rhythm similarity:
Correlation with human judgments. Analytical
Approaches to World Music, 1, 2.
ICPRAM2013-InternationalConferenceonPatternRecognitionApplicationsandMethods
598
