Numerical Model for the Prediction of Final Mechanical Properties

of EPDM Vulcanized with Peroxides

Part I: Basis of the Numerical Model and Experimental Campaign

Gabriele Milani

and Federico Milani

Politecnico di Milano, Piazza Leonardo da Vinci 32, 20133 Milan, Italy

Chem. Co Consultant, Via J.F. Kennedy 2, 45030 Occhiobello (RO), Italy

Keywords: Kinetic Numerical Model, EPDM Rubber, Peroxides, Comparison with Experimental Data.

Abstract: In the paper, a simple but effective mathematical model having kinetic base, to predict the vulcanization

degree of rubber vulcanized with peroxides, is presented. The approach takes contemporarily into

consideration, albeit within a simplified scheme, the actual reactions occurring during peroxidic curing,

namely initiation, H-abstraction, combination and addition, and supersedes the simplified approach used in

practice, which assumes for peroxidic curing a single first order reaction. After a suitable re-arrangement of

the first order system of differential equations obtained from the actual kinetic system adopted, a single

second order non-linear differential equation is obtained and numerically solved by means of a Runge-Kutta

approach. Kinetic parameters to set are evaluated by means of a standard least squares procedure where

target data are represented by experimental values available, i.e. normalized rheometer curves. In order to

assess numerical results, a wide experimental campaign is conducted, varying curing agents, vulcanization

temperatures and concentration of the peroxides. Both rheometer curves to compare with those provided by

the numerical model and stretch-strain curves of the vulcanized samples are obtained, to have a precise

insight into the most suitable parameters to use during curing.

1 INTRODUCTION

The curing process of medium and high voltage

electric cables with EPM/EPDM rubber having low

molecular weight (Mooney viscosity ML), ethylene

high content and low-medium amount of ter-

monomer (generally 1,4-Hesadiene, ENB, DCPD

and VNB) is made by peroxides.

In this paper, an industrial formulation with a ter-

polymer and VNB as third monomer (Vistalon,

1703) exhibiting a large molecular weights

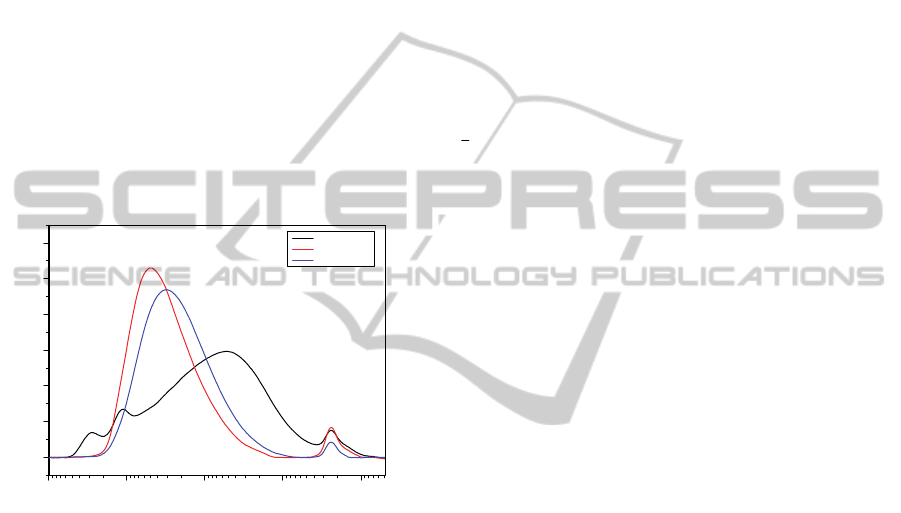
distribution, as shown in Figure 1, good

processability suitable for the extrusion of medium-

high voltage electric cables is considered. The

peculiar characteristic of the industrial product

analyzed is that unvulcanized rubber is produced in

pellettes, allowing absorption of the peroxide on the

surface of each single element, satisfactory stocking

stability and the possibility to vulcanize directly the

compound by other factories.

On the base of such considerations and with the

aim to determine the optimal reticulation conditions

of real thick items, it appears therefore interesting to

propose a comprehensive numerical model based on

the actual decomposition reactions occurring in

reality, as close as possible to that observed

experimentally, to predict with simple computer

simulations the optimal curing time and temperature,

Milani and Milani (2008, 2012a, 2013) and the most

suitable peroxide to utilize in order to maximize the

final crosslinking density of an item with given

geometry, Milani and Milani (2010).

This work is part of a wider

experimental/numerical program conducted by the

authors on the same rubber recipe constituted by an

EPDM elastomer, changing both peroxides used and

controlled vulcanization temperature. In particular,

test were conducted in a range temperature varying

between 160 and 200°C, with a 20°C step, using

different peroxides, changing their concentration and

considering also a mixture of three of them. A huge

amount of rheometric cure curves were obtained

(one for each temperature, peroxide used and

concentration) and quantitatively compared one each

other, to have an insight into the most effective

temperature and peroxide to use during such kind of

Milani G. and Milani F..

Numerical Model for the Prediction of Final Mechanical Properties of EPDM Vulcanized with Peroxides - Part I: Basis of the Numerical Model and

Experimental Campaign.

DOI: 10.5220/0004423700580065

In Proceedings of the 3rd International Conference on Simulation and Modeling Methodologies, Technologies and Applications (SIMULTECH-2013),

pages 58-65

ISBN: 978-989-8565-69-3

 2013 SCITEPRESS (Science and Technology Publications, Lda.)

vulcanization, in terms of both vulcanization

velocity and maximum torque reached.

In order to interpret theoretically experimental

results obtained, a peroxide decomposition kinetic

model is presented, considering the complex set of

reactions that characterize peroxide crosslinking,

namely homolytic cleavage of peroxide, hydrogen

abstraction, polymer radicals formation, polymer

radicals coupling, cross-link formation and polymer

scission.

Starting from the above -most probable- kinetic

scheme, a system of non-linear differential equations

with few variables is obtained and solved

numerically, after suitable variable substitutions, by

means of a Runge-Kutta algorithm. Crosslinking

density is thus evaluated numerically and compared

to rheometer experimental curve, which may provide

a reliable indication of the average cured rubber

crosslinking, once suitably scaled to unitary values.

Figure 1: Molecular weight distribution of Vistalon 1703

compared with other two distributions of common

industrial products.

By means of the numerical approach proposed,

an estimation of the kinetic constants per single

reaction, once known kinetic decomposition laws,

may be evaluated and hence the activity of each

peroxide may be estimated using a few experimental

data at only two different temperatures.

By means of the additional experimental and

numerical evaluation of the stress-strain curves of

the vulcanized samples, it is also possible to

establish a correlation between tensile strength and

maximum torque obtained with the rheometer test,

again useful for an optimization of the production

processes.

It has to be finally considered that, for thick

items exceeding 5 mm, a numerical algorithm is

already at disposal to determine the most suitable

temperatures and exposition times to be used in the

industrial production, in order to guarantee that the

core results not under-vulcanized and the skin not

over-vulcanized.

2 RETICULATION KINETIC OF

EPDM VULCANIZED WITH

PEROXIDES

The basic chemistry in the generally accepted

mechanism of peroxide cure of EPDM have been

reviewed by van Duin and co-workers (Dees and

van Duin, 2008); (van Duin, 2002), and may be

summarized by means of the following partial

reactions occurring in series and parallel:



(addition)

HEP(D)MEP(D)MEP(D)MEP(D)MEP(D)MEP(D)M

on)(combinati

EP(D)M-EP(D)M2EP(D)M

nabstractio-H

HROH/R'EP(D)M/R'ROH-EP(D)M

initiation

R'ROROOR









(1)

The chain of free-radical reactions is initiated by

thermal decomposition of the peroxide, yielding

primary alkoxy (RO) or secondary alkyl radicals

(R). Subsequent abstraction of H-atoms from the

EPDM polymer results in the formation of EPDM

macro-radicals (EPDM). Calculations based on

kinetic data for H-abstraction indicate that H-

abstraction mainly occurs along the saturated EPM

polymer backbone, Van Duin (2002), whereas

several electron paramagnetic resonance (EPR)

spectroscopy studies have shown the selective

formation of allyl radicals derived from the diene

monomer.

Considering the energy required for the

abstraction of the H-atoms, see Figure 2, within the

formation of the back-bone, the allyl radicals are

more probable than the others, because of the lowest

energy required by the abstraction of the H-atoms ,

Knox and Palmer (1961).

The actual cross-linking proceeds via two

pathways, which have been shown to be additive.

Two EPDM macro-radicals either combine or,

alternatively, a macro-radical adds to an EPDM

unsaturation. Visible spectroscopy has confirmed the

conversion of the EPDM unsaturation upon peroxide

cure, Dickland and van Duin (2002). It is noted that

in practical EPDM/peroxide compounds usually co-

agents, such as triallyl (iso)cyanurate,

trimethylolpropane trimethacrylate or m-

phenylenebis(maleimide), are included to increase

the peroxide curing efficiency, Hofmann (1989),

which obviously affects the mechanism of peroxide

1E7 1000000 100000 10000 1000

0,0

0,2

0,4

0,6

0,8

1,0

1,2

a.u

PM (g/mol)

V1703 norm

CO-058 norm

V785 norm

NumericalModelforthePredictionofFinalMechanicalPropertiesofEPDMVulcanizedwithPeroxides-PartI:Basisof

theNumericalModelandExperimentalCampaign

cure.

Figure 2: Energy required in order to extract hydrogen

atom from the backbone of the macromolecules at 0 K. At

25°C add 1 Kcal.

In the paper, a simple but effective mathematical

model having kinetic base, to predict the

vulcanization degree of rubber vulcanized with

peroxides is presented.

The approach takes contemporarily into

consideration a large set of reactions occurring

during peroxidic curing, i.e. initiation, H-abstraction,

combination and addition, overcoming the

simplified approach which assumes for peroxidic

curing a single first order reaction. After a suitable

re-arrangement of the first order system of

differential equations obtained from the actual

kinetic system adopted, a single second order non-

linear differential equation is obtained and

numerically solved by means of a Runge-Kutta

approach. Kinetic parameters to set are evaluated by

means of a standard least square procedure where

target data are represented by experimental values

available, i.e. normalized rheometer curves or

percentage crosslink density experimentally

evaluated by means of more sophisticated

procedures.

Two cases of technical interest are critically

discussed, the first relying into an EPDM

crosslinked with di-cumyl peroxide, the second into

a compound with high level of unsaturation

exhibiting reversion at relatively high temperatures

(175°C) near the end of the vulcanization process. A

quite promising agreement is experienced, even in

presence of reversion.

3 THE KINETIC

MATHEMATICAL MODEL

PROPOSED

The mechanism of EPM/EPDM peroxide curing and

the structures formed are still lacking, mainly due to

the complexity of the system (large number of

structures formed at low concentrations) combined

with the relatively difficult in term of analytical

accessibility to determine the cross-linked polymer

networks.

The complex set of the reactions that

characterize peroxide cross-linking involve

homolytic cleavage of peroxide, oxy and alkyl

radicals, hydrogen abstraction, radical coupling

(cross-link formation), polymer scission and radical

transfer. In the case of EPDM, the amount and type

of the third-monomer, could be considered, but in

first approximation it is not considered for the types

of EPDM with lower concentrations of the third-

monomer. Therefore, our cross-link scheme the

different activity from alkyl and oxy radicals in the

homolytic cleavage of peroxide and the secondary

reactions as oxygenation and acid-catalyzed

decomposition of peroxide. From these

considerations, we have adopted for EPM/EPDM the

kinetic scheme for the peroxide cross-link reactions

summarized in Equation (1).

To circumvent limitations of such models in the

application of EPDM rubber, the mechanisms at the

base of vulcanization for such blend should be

properly considered, Milani & Milani (2011, 2012b).

In this framework, focusing exclusively on EPDM

rubber, one possible polymerization scheme may be

the following:



PPR



PP 

(2)

In Equation (2),

is the peroxide,

the primary

alkoxy (RO) or secondary alkyl radicals (R),

the uncured polymer,

is the EPDM macro-

radical,

and

the matured cross-linked

polymers, and

4,...,1

are kinetic reaction constants..

Here it is worth emphasizing that

4,...,1

are

temperature dependent quantities, hence they

rigorously should be indicated as

)(

4,...,1

, where

is the absolute temperature. In what follows, for

the sake of simplicity, the temperature dependence

will be left out.

By means of the so called xyz method,

independent variables may be established. From

stoichiometry of the reaction, it can be argued that:

H-vinilyc

H-allilyc

H-3th

H-2nd

H-1st

CCCCCC

431.0 kJ/mol

355.6 kJ/mol

380.7 kJ/mol

395.4 kJ/mol

410.0 kJ/mol

E: energy

required to extract H

SIMULTECH2013-3rdInternationalConferenceonSimulationandModelingMethodologies,Technologiesand

Applications

xII 

yxR  2

yPP 

qzyP  2



(3)

Obviously, from (3) it can be argued that

independent variables are:











Indeed:

IIx





RIIy  )(2



2 IIRPP 





2)(2

PPRIIP 

Pz 

Pq 

(4)

The aim is to provide an analytical expression for

vulcanized rubber, i.e. concentration of

with

respect to time.

From (2) and (3), the following set of differential

equations is deduced:



RPKIK

2 

RPK





PKPKRPK







(5)

(a), (b) and (c) form a system of differential

equations in three variables which may be solved as

follows. (a) may be solved immediately by

separation of variables:



ttK

eII





(6)

(b)-(c) is:



011

ttK

eIKIK





(7)

Differentiation of (c) results into:



(8)

From (7), remembering from (5) that



, the second order differential equation (8) may be

re-written exclusively in terms of P as follows:



021





















ttK

PeIKK

(9)

The non linear differential equation (9) may be

solved numerically with a standard Runge-Kutta

algorithm, Matlab (2011), to find concentration P(t).

The knowledge of P(t) allows to determine R(t) and,

from equation (5)(d),

P :



)()( PKPKtPtRK



(10)

Equation (10) is again solved using a Runge-Kutta

numerical approach.

4 COMPARISON WITH

EXPERIMENTAL DATA

A wide experimental campaign has been conducted

by the authors in cooperation with Mixer Spa,

Milani et al., (2013), on a same EPDM blend but

varying a number of different parameters, as for

instance curing agent, peroxide concentrations and

vulcanization temperature.

Table 1: Experimental data set analyzed, EPDM

composition in phr.

Polymer













(Exxon)er Manufactur

35.3 100°C 4)+(1 MLMooney

0.9 % in wt. rnenevinylnorbo-VNB

76.2 % in wt. Ethylene

1703P VISTALON

Silane treated calcinated kaolin 30

Antioxidants 8

LDPE Riblene MR 10 MFI= 18.7

(ASTM D1238) 6

PE WAX 1

Peroxide 1

A huge amount of experimental data have been

collected, including rheometer curves at different

temperatures and stress strain curves of the

vulcanized items.

The blend under consideration has the

composition schematically reported in Table 1.

As it is possible to notice from the molecular

NumericalModelforthePredictionofFinalMechanicalPropertiesofEPDMVulcanizedwithPeroxides-PartI:Basisof

theNumericalModelandExperimentalCampaign

weight distribution of Vistalon 1703 reported in

Figure 1, it is quite wider with respect to other

common products available in the market stock.

The experimentation was conducted varying the

following parameters:

Curing agent. Three different peroxides or a

mixture of peroxides have been tested. The first,

hereafter called M2 for the sake of clearness, is a

mixture of three different peroxides utilized by

the factory, AkzoNobel (2012), to industrially

produce cured rubber. The three peroxides

composing the mixture are the following:

Trigonox T, Perkadox BC-FF and Perkadox 14S

all in the same proportion. The sum of

concentrations of the three peroxides used

industrially are equal to around 1 phr and

hereafter is labeled as +/- 0% concentration. The

second and third peroxides investigated are

commercial products provided by AkzoNobel,

having commercial names as Perkadox BC-FF

and Perkadox 14S-FL. They will be indicated in

the following comparisons as BC-FF and 14S for

the sake of conciseness. Perkadox BC-FF is

dycumil peroxide whereas Perkadox 14S-FL is a

di(tert-butylperoxyisopropyl) benzene. They

exhibit a half time equal to 1 minute at 138°C

and 146°C, respectively. The peroxides are

therefore quite similar; nonetheless, some

perceivable differences in the experimental

rheometer curves of the EPDM under

consideration were observed.

Concentration of the curing agent: five different

concentrations for the different curing agents

investigated were considered, hereafter labeled

as -50%, +/- 0%, 50%, 100 % and 150% for the

sake of clearness. Labels indicated the molar

concentration of the curing agent with respect to

the standard concentration (indicated as +/- 0%)

utilized by Mixer within their production

process.



3) Vulcanization temperature. Rheometer data were

obtained in an oscillating disc curometer at three

different temperatures, respectively equal to

160°C, 180°C and 200°C. A wider range of

temperatures was experimentally investigated by

the authors, namely from 120°C up to 220°C, but

the activity of the curing agents resulted

suboptimal out of the range 160°-200°C.

In figures from Figure 3 to Figure 7, detailed

comparisons among the performances during

vulcanization (rheometer curves) exhibited by the

three different curing agents at different

concentrations are shown. Such comparisons are

useful to have an insight into the most suited curing

agent to be used at different temperatures. It is quite

clear that the optimal behavior of all curing agents is

obtained at 150% concentrations.

-a

-b

-c

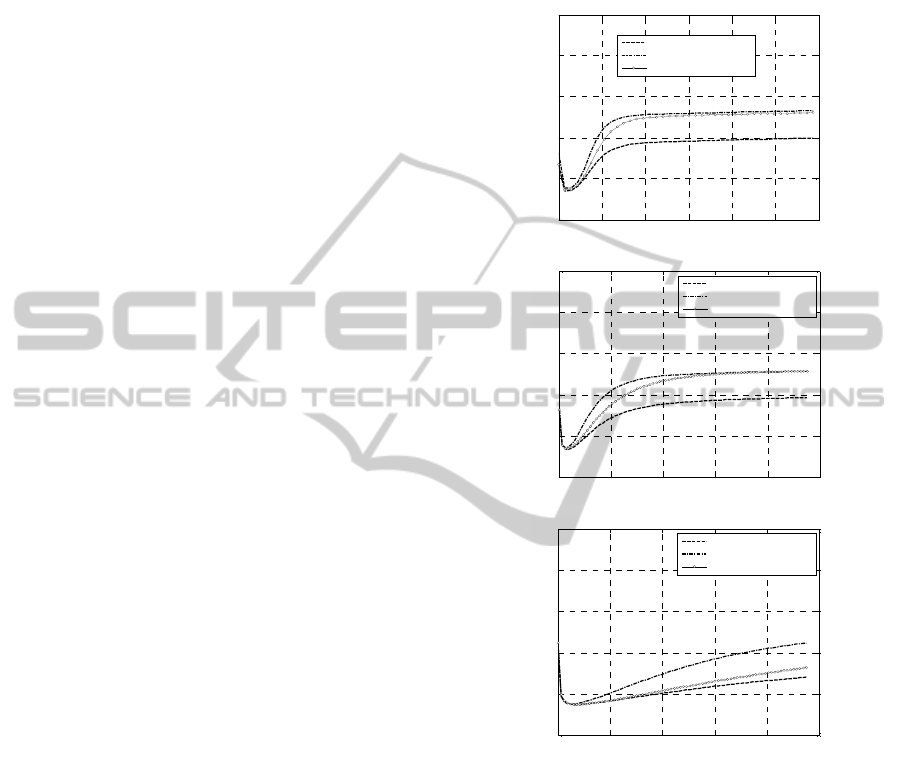
Figure 3: -50% Concentrations. Experimental rheometer

curves at different peroxide concentrations. –a: 160 °C. –

b: 180 °C. –c: 200 °C.

For such concentrations and for a curing

temperature equal to 200°C, it can be observed that

the maximum torque provided by all curing agents

(M2, BC-FF and 14-S) is roughly the same (around

55 dNm, see Figure 7). Furthermore, the times

needed to obtain 90% of the maximum torque (t90

values) seem very similar, meaning that also the

kinetic constants at 200°C to be expected by the

numerical model proposed should be very similar.

Two replicates for each vulcanization condition

were considered within the experimental campaign,

in order to reduce possible inaccuracies of the

0 2 4 6 8 10 12

200°C -50%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

0 5 10 15 20 25

180°C -50%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

0 5 10 15 20 25

160°C -50%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

SIMULTECH2013-3rdInternationalConferenceonSimulationandModelingMethodologies,Technologiesand

Applications

experimentation. In this manner, a total amount of

90 different rheometer curves were experimentally

determined.

Table 2: half-life temperatures of the peroxides analysed at

0.1, 1 and 10 hours.

Peroxide

typology

1/2

hours

0.1 1 10

TRIGONOX T 169 146 117

PERKADOX

BC-FF

162 138 112

PERKADOX

14 S-FL

169 146 117

TRIGONOX T: ter-butylcumylperoxide

PERKADOX BC-FF: dicumyl peroxide

PERKADOX 14S-FF: di(tert-butylperoxyisopropyl) benzene

Curing agent M2 is a mixture of BC-FF, 14S-FF and Trigonox

-a

-b

-c

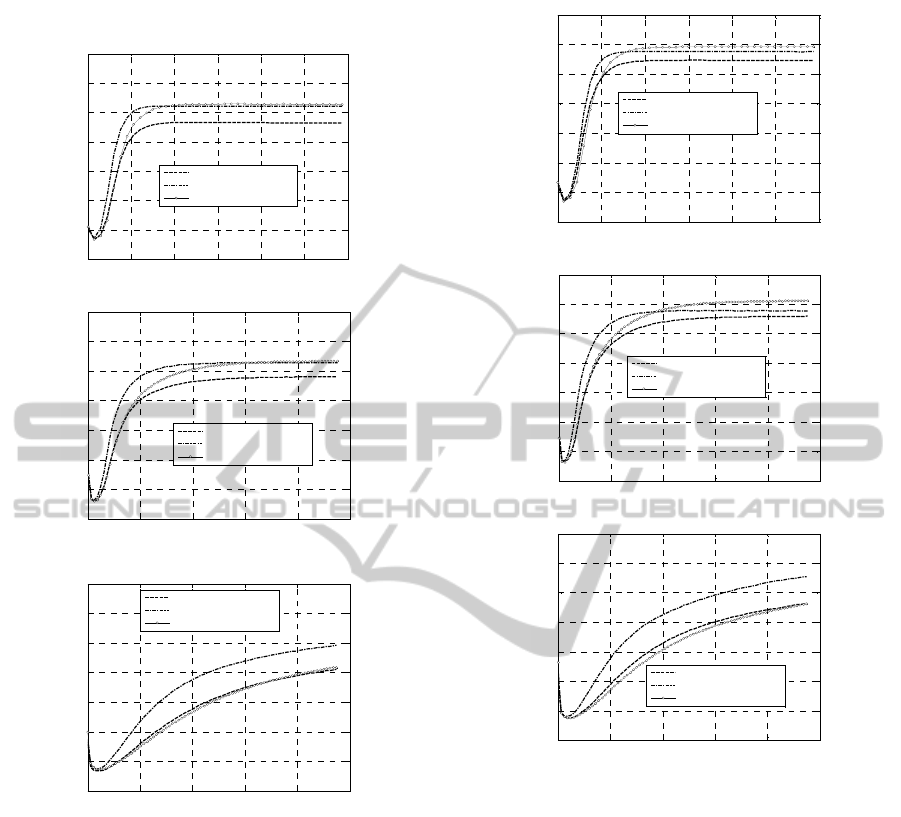
Figure 4: +/-0% Concentrations. Experimental rheometer

curves at different peroxide concentrations. –a: 160 °C. –

b: 180 °C. –c: 200 °C.

As expected, in all cases, experimental rheometer

curves do not exhibit perceivable reversion, due to

the low amount of unsaturations present in the

EPDM utilized.

-a

-b

-c

Figure 5: +50% Concentrations. Experimental rheometer

curves at different peroxide concentrations. –a: 160 °C. –

b: 180 °C. –c: 200 °C.

5 CONCLUSIONS

A combined numerical model and experimental

campaign have been conducted with the aim of

tuning and validating a complex numerical model

with kinetic base, useful to predict the final crosslink

density of rubber vulcanized with single peroxides

or mixtures of peroxides. In the present Part I, the

experimental results are presented and discussed. In

addition, the theoretical base of the numerical model

0 2 4 6 8 10 12

200°C +/-0%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

0 5 10 15 20 25

180°C +/-0%

Time t [min]

Torque [

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

0 5 10 15 20 25

160°C +/-0%

Time t [mi n]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

0 2 4 6 8 10 12

200°C +50%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

0 5 10 15 20 25

180°C +50%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

0 5 10 15 20 25

160°C +50%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

NumericalModelforthePredictionofFinalMechanicalPropertiesofEPDMVulcanizedwithPeroxides-PartI:Basisof

theNumericalModelandExperimentalCampaign

is presented.

-a

-b

-c

Figure 6: +100% Concentrations. Experimental rheometer

curves at different peroxide concentrations. –a: 160 °C. –

b: 180 °C. –c: 200 °C.

The experimental part is wide, since a number of

different cases occurring in practice has been

investigated in detail, varying curing agent typology,

vulcanization temperatures and concentration of the

peroxides. Both rheometer curves to compare with

those provided by the numerical model and stretch-

strain curves of the vulcanized samples have been

collected, to have a precise insight into the most

suitable parameters to use during curing.

-a

-b

-c

Figure 7: +150% Concentrations. Experimental rheometer

curves at different peroxide concentrations. –a: 160 °C. –

b: 180 °C. –c: 200 °C.

REFERENCES

AkzoNobel (2012). Brochure of organic peroxides,

available at http://www.akzonobel.com/polymer/

Dees, M., van Duin, M., 2008. Rubber World, Aug./Sept.

Dikland, H.G., van Duin, M., 2002. Crosslinking of

EPDM and Polydiene Rubbers Studied by Optical

Spectroscopy. In: Spectroscopy of Rubbers and

Rubbery Materials, Rapra Technology Ltd., Litvinov

VM, De PP (Eds), Shawbury, Shrewsbury, Shropshire,

207.

Hofmann, W., 1989. Rubber Technology Handbook,

Hanser Publishers, Munich.

Knox, B.E., Palmer, B., 1961. Chem. Rev., 61: 247.

Matlab User’s Guide, 2007. http://www.mathworks.com/

products/matlab/

0 2 4 6 8 10 12

200°C +100%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

0 5 10 15 20 25

180°C +100%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

0 5 10 15 20 25

160°C +100%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

0 2 4 6 8 10 12

200°C +150%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

0 5 10 15 20 25

180°C +150%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

0 5 10 15 20 25

160°C +150%

Time t [min]

Torque [dN*m]

M2 Retic M3 Laboratory

Perkadox BC - FF

Perkadox 14 S - FL

SIMULTECH2013-3rdInternationalConferenceonSimulationandModelingMethodologies,Technologiesand

Applications

Milani, G., Galanti, A., Cardelli, C., Milani, F., 2013.

Peroxide cross-linking of EPDM for medium voltage

cable applications: experimental insight. In: Proc.

183rd Technical Meeting Akron, Ohio Monday, April

22, 2013 - Wednesday, April 24, 2013

Milani, G., Milani, F., 2008. Computers & Chemical

Engineering, 32: 3198-3212.

Milani, G., Milani, F., 2010. Journal of Mathematical

Chemistry 47(1): 229-267.

Milani, G., Milani, F., 2011. Journal of Mathematical

Chemistry, 48: 530–557.

Milani, G., Milani, F., 2012a. Computers & Chemical

Engineering, 43: 173–190.

Milani, G., Milani, F., 2012b. Journal of Applied Polymer

Science, 124(1): 311–324.

Milani, G., Milani, F., 2013. Kinetic FE model to optimize

sulphur vulcanization: application to extruded EPDM

weather-strips. Polymer Engineering and Science, in

press.

van Duin, M., 2002. Kautsch. Gummi Kunstst, 55: 150.

NumericalModelforthePredictionofFinalMechanicalPropertiesofEPDMVulcanizedwithPeroxides-PartI:Basisof

theNumericalModelandExperimentalCampaign