
LUC - Land Uptake Control
A GIS-based Approach
Elena De Santis
1
and Bernardino Romano
2
1
Department of Information Engineering, Computer Science and Mathematics (DISIM), Center of Excellence DEWS,
University of L’Aquila, 67100 L’Aquila, Italy
2
Department of Civil and Environmental Engineering (DICEAA), University of L’Aquila, 67100 L’Aquila, Italy
Keywords:
Land Uptake Control, Geographical Information System.
Abstract:
Land use caused by urbanisation is one of the main causes of political and social conflicts and altered envi-
ronmental quality of land. It is a widespread feeling that the zero-balance objective (i.e. new areas can be
urbanized only if already urbanized areas are restored) is feasible, at least in developed country, where the
population is stabilizing around constant values. But it is very important to control the transient, between the
current almost unrestricted situation and the zero-balance regime. Therefore, this paper proposes land uptake
control procedures, based on geographical information systems and remote sensing.
1 INTRODUCTION
In the broader framework of sustainability challenges,
land uptake surely plays an important role. Advanced
technologies influence urban developments and, at the
same time, they can support required cultural, social,
economic and politic adjustments and can make ef-
fective control procedures, needed to mitigate the im-
pact of human activities on the environment (see e.g.
(Lynette I. Millett and Deborah L. Estrin, 2012)).
According to consolidated scientific opinions,
land use caused by urbanization is one of the main
causes of political and social conflicts (Plotkin,
1987)and altered environmental quality of land (Ellis
and Ramankutty, 2008), (Sala et al., 2000).
The aspects involved, either directly or indirectly,
in urban conversion of land include the following:
Economic and energetic field:
• diseconomies in transport.
• waste of energy;
• reduced agricultural produce.
Hydro-geo-pedologic field:
• geological destabilization;
• irreversible use of land;
• alteration of underground and surface water-
courses.
Physico-climatic field;
• increased thermal reflection and climate change;
• reduced emission absorption capacity;
• effects on carbon sequestration;
• spatial propagation of physico-chemical distur-
bances.
Eco-biological field
• physical erosion and destruction of habitats;
• ecosystem fragmentation;
• dystrophy of ecological and biological pro-
cesses;
• penalization of the ecosystem services of the en-
vironment;
• reduction in overall ecological “resilience”.
The awareness of the need of land uptake con-
trol is shared in the scientific and social community
(EEA, 2006). To cite an example, in (RNE, 2008) it
is stated that the German Federal Government’s goal
is to reduce the land being claimed for settlement and
transport use to 30 hectares per day by 2020. When
last assessed, the new use of land for settlement and
transport stood at 113 hectares per day (trend 2003—
2006). The achievement of the 30-hectare goal in the
practice of urban development is in fact primarily a
matter for the L
¨
ander and the municipalities. The
Federal Government is, however, active in providing
support through the programmes for funding urban
development which are geared towards the inner de-
velopment of towns and municipalities, through re-
search programmes and last but not least through leg-
islative measures ((RNE, 2008), (Henger and Bizer,
2010), (Siedentop and Fina, 2010)).
It is a widespread feeling that the zero-balance ob-
jective (i.e. new areas can be urbanized only if already
450
De Santis E. and Romano B..
LUC - Land Uptake Control - A GIS-based Approach.
DOI: 10.5220/0004487004500456
In Proceedings of the 10th International Conference on Informatics in Control, Automation and Robotics (ICINCO-2013), pages 450-456
ISBN: 978-989-8565-70-9
Copyright
c
2013 SCITEPRESS (Science and Technology Publications, Lda.)

urbanized areas are restored) is feasible, at least in de-
veloped country, where the population is stabilizing
around constant values. But it is very important to
control both the transient evolution, between the cur-
rent almost unrestricted situation and the zero-balance
regime, and the transient duration, to allow the adap-
tation to the new paradigm for the current social and
economic model, which is instead strongly dependent
on the land transformability.
In this paper we propose a land uptake control pro-
cedure, that involves the main actors (municipalities,
regional authorities), at different level of responsibil-
ity. This control procedure could be implemented by
defining appropriate normative tools, by establishing
technical staffs with the task of system monitoring
and of data base maintaining, and by establishing an
efficient information management infrastructure.
The main idea is that of introducing the output-
feedback paradigm in the environmental planning
process. While closed loop control is a standard tool
in most technological disciplines, it is not so in social
management systems, in general. This is mainly due
to the complexity of the systems, whose behavior is
in most case unpredictable with sufficient accuracy,
to the difficult in obtaining data and sometimes to the
cultural inadequacy of the system managers (politi-
cians, administrative and technical staff). In fact the
seminal book (McLoughlin, 1969) remains an im-
portant reference for a system approach to the urban
and environmental planning, but, at the best of our
knowledge, this conceptual formulation of the prob-
lem has not so far produced an effective information
system, for a dynamic plan management. Nowadays
the things are dramatically changing: the availability
and penetration in the public administration of Ge-
ographical Information Systems (GIS), the availabil-
ity of remote sensing techniques and powerful hard-
ware and communication infrastructures (WEB), to-
gether with advances in systems and control theory
make innovative procedures feasible ((Sharma et al.,
2012),(Thompson and Prokopy, 2009)). Anyway the
complexity of the systems remains unchanged, and
hence a possible approach is that of splitting the over-
all system into different interacting sub-systems, each
characterized by simple models. The level of abstrac-
tion of each model has to be obviously appropriate for
the problem to be solved.
In the system we are considering humans are in
loop, and the social behavior has not been modeled.
Therefore the resulting control system is not auto-
matic, but it can offer an important formal frame-
work to support the decision process. This control
scheme could be integrated in an environmental De-
cision Support System (eDSS) together with all the
monitoring, management and communication tools.
The goal of the procedure we propose is twofold:
minimization of the total amount of land uptake,
while assuring the per capita standard of urbanized
areas, and maximization of the aggregation of these
areas. In fact the urban sprawl is another phenomenon
to be controlled, in order to mitigate the above defined
aspects, with a particular attention to diseconomies in
transport and waste of energy. See (Glaeser, 2011),
for a vibrant discussion on the advantage of a dense
city, with respect to energy consumptions, sharing of
amenities and so on, even at popular level.
Instead of giving hard constraints on the land
uptake amount, our proposal is based on a reward-
penalty approach, by giving a monetary value to the
untransformed land. In this way each municipality
has flexibility in planning the environmental trans-
formations, but has also the awareness that the deci-
sions have to be the result of an optimization prob-
lem, where the land uptake has its cost. We think that
the flexibility and the graduality are the keys to allow
the practical implementation of environmental control
policies, and to reduce possible social conflicts.
The idea of planning the future developments, step
by step, on the base of actual measured data, but in the
framework of given objectives, could cause a change
of perspective in urban planning itself, as it is tradi-
tionally considered, at least in those countries (e.g.
Italy) where a process control on the current plan is
not actuated, after the approval procedures. We do
not dwell here on this question, but a parallel analysis
is in progress.
The paper is organized as follows: the land uptake
problem is defined in Section 2. In Section 3, three
possible control schemes are introduced. Section 4
considers the sprawl control problem. In Section 5 a
discussion is offered, with possible extensions of the
approach.
2 LAND UPTAKE
The issue of excessive urban conversion of natural
land has been raised at European level only in recent
years, mainly through the initiatives undertaken by
the European Environmental Agency (EEA, 2006) to
analyze this phenomenon and its consequences. See
Fig.1 and Fig 2, for some revealing data. See also (Pi-
leri and Maggi, 2010) and (Romano and Zullo, 2013a)
for analysis of urbanization in Italy.
Furthermore, it is worth recalling that the Euro-
pean Commission, based on a need to tackle soil
productivity, risks to human health and the environ-
ment, and to provide opportunities for climate mit-
LUC-LandUptakeControl-AGIS-basedApproach
451
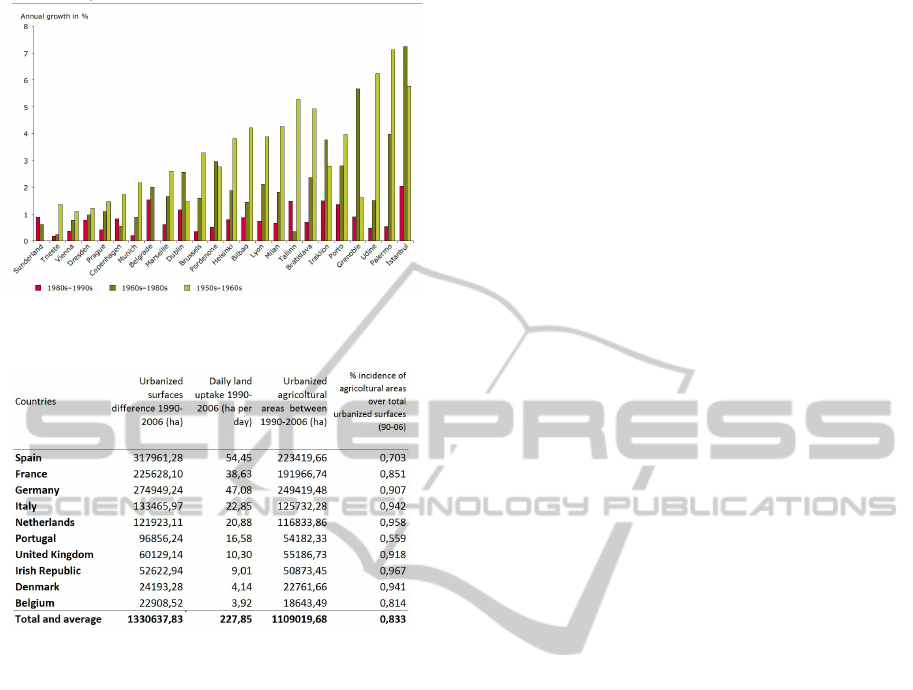
Figure 1: Annual grow of land uptake in some municipali-
ties (EEA, 2006).
Figure 2: Data on urbanisation in Western Europe (Romano
and Zullo, 2013b).
igation and adaptation as well as stimulating busi-
ness opportunities for soil remediation, proposed a
Soil Framework Directive in 2006, which amends di-
rective 2004/35/EC (on environmental liability with
regard to the prevention and remedying of environ-
mental damage) and reaffirms the status of “non-
renewable resource” for this particular territorial com-
ponent and the need for its conservation. The Euro-
pean Parliament adopted its first reading on the pro-
posal in November 2007 by a majority of about two
thirds. At the March 2010 Environment Council, a
minority of Member States blocked further progress
on grounds of subsidiarity, excessive cost and admin-
istrative burden. No further progress has since been
made by the Council. The proposal remains on the
Council’s table.
The 2006 EEA report states that: all available ev-
idence demonstrates conclusively that urban sprawl
has accompanied the growth of urban areas across
Europe over the past 50 years. This is shown from
a recent European perspective. The areas with the
most visible impacts of urban sprawl are in coun-
tries or regions with high population density and eco-
nomic activity (Belgium, the Netherlands, southern
and western Germany, northern Italy, the Paris re-
gion) and/or rapid economic growth (Ireland, Portu-
gal, eastern Germany, the Madrid region). Sprawl is
particularly evident where countries or regions have
benefited from EU regional policies.
When we speak about land uptake, more precisely
we consider ”urbanized soil”, in accord to the follow-
ing classification (Romano and Zullo, 2013a):
• Built-up Land: surfaces covered by buildings and
identifiable through the ground projection of the
perimeter of the latter. Natural soil has been re-
moved completely in order to build foundations
• Urbanized Soil: land used for urban functions,
involving the replacement or retention of natural
soil: it includes built-up land and land used for
ancillary settlement functions, such as public and
private gardens, sports facilities, unpaved roads
and other service areas, either permeable or im-
permeable to water
• Artificial Land: areas where the natural layer has
been replaced by other materials, either permeable
or impermeable to water, to allow different uses:
it includes parts of built-up land, but also streets,
squares and parking lots (paved or permeable), as
well as sports fields or excavation areas
• Sealed Soil: surfaces covered by layers of im-
permeable material preventing the absorption of
surface water. It includes built-up land and land
used for other purposes that require paving, such
as streets, squares and parking lots and all those
cases where the natural soil layers are removed
entirely and replaced by other materials that im-
prove the stability and indeformability of sur-
faces.
3 LUC TECHNIQUES
We consider the case of a region, divided into a num-
ber of municipalities. We assume that the control ob-
jective is defined at the regional level, but the deci-
sions are taken at the municipal level. The regional
authorities have the responsibility of system monitor-
ing and control.
Let i ∈
{
1..N
}
be the index for the municipali-
ties. The integer k denotes a time step. The physi-
cal time between k and k + 1 mainly depends on the
adjourning rate of geographical data (typically one or
more years between two successive remote sensing
actions). For simplicity we will call ”time” the in-
dex k. Let x
i
(k) be the amount of urbanized areas in
municipality i at time k, u
i
(k) the amount of new ar-
eas, which will be urbanized between time k and time
ICINCO2013-10thInternationalConferenceonInformaticsinControl,AutomationandRobotics
452

k + 1, as planned by municipality i at the beginning
of time period k. Finally, let δ
i
(k) a ”disturbance”
such that u
i
(k)+δ
i
(k) is the actual urbanized areas be-
tween time k and time k+1, as measured at the end of
kth period of time. The disturbance δ
i
(k) models the
deviations with respect to the planned behavior (e.g.
unauthorized building). Obviously δ
i
(k) can be neg-
ative, and in this case it models delays in the execu-
tion of planned intervention or the fact that some pre-
viously urbanized areas have been de-urbanized (e.g.
caves re-naturalization). The value u
i
(k) + δ
i
(k) can
be negative, too. The value x
i
(k) comes as the result
of a measurement at time k.
In the first model we assume that the central-
ized controller defines for each municipality the up-
per bound of the urbanized areas, with a defined time
horizon T . At time T a new upper bound will be de-
fined and so on. This upper bound could be computed
on the base e.g. of demography, within clusters of
homogeneous territorial units (municipalities, in our
cases). See (Romano and Zullo, 2013a) for a compu-
tation of the per capita land uptake, called hereafter
urbanization standard or simply standard, denoted by
σ. The reward or the penalty is computed step by step
on the base of the deviation of the actual urbanized
value with respect to the upper bound. The goal is that
of reaching a ratio between urbanized areas and pop-
ulation which is close, and possibly below, the stan-
dard. Therefore the bound is an important starting
point in the control process, and the decisions about it
have to be shared in the involved social communities.
The second model considers possible interactions
among the municipalities, given the bounds and the
time horizon: in this model a municipality can buy
the right of urban transformation from another mu-
nicipality. This one is in our opinion a very impor-
tant mechanism, because it introduce the concept that
avoiding unnecessary transformations gives quantifi-
able advantages. On the other side transforming be-
yond the initially given bound has an additional cost,
and hence the decision about it has to be carefully
taken.
Finally this last model is reformulated in a reced-
ing horizon framework (see e.g. (Camacho and Bor-
dons, 1995) and (Soeterboek, 1992) for introductory
readings on this theme), where the bounds on the ur-
banized areas are dynamically adjourned, taking into
account the predicted population after H steps.
In this paper we assume that the standard σ is time
invarying, but in order to meet the zero-balance objec-
tive it could be possible to consider a weighted stan-
dard, by means of a decreasing function: e.g. σ(k) =
σ(0)exp(−λk)+
b
σ(1 − exp(−λk)), where σ(0) is the
current standard, λ > 0 and
b
σ is the minimal standard,
which is compatible with a predicted social and eco-
nomic scenario. Determining such a minimal stan-
dard is not an easy task: some research effort is in
progress on this point, but we are not aware of any
established result.
3.1 Single Municipality Model
Given the horizon T , let x
i
the upper bound for ur-
banized areas in municipality i, computed by con-
sidering the standard and the expected population
at time T . The state of the system is x(k) =
x
1
(k) x
2
(k) . . . x
N
(k)
0
. The state equation
is
x
i
(k + 1) = x
i
(k) + u
i
(k) + δ
i
(k) (1)
k = 0, ..., T − 1, i = 1..N
and let the input be
u
i
(k) = F
i
(k)
x
i
0
− x
i
(k)
, if x
i
≥ x
i
(k)
u
i
(k) = 0, if x
i
0
< x
i
(k)
with F
i
(k) ∈ [0, 1]. The condition x
i
< x
i
(k) means
that the current degree of urbanization is above the
standard, with respect to the future estimated popu-
lation. This could be due or to a decreasing trend in
population or to the fact that the urbanization is above
the standard with respect to the current population and
to the expected population. Consider moreover the
cost function
J
T
= c
T
∑
k=1
x
i
0
− x
i
(k)
with c > 0. The value c
x
i
0
− x
i
(k)
is a ”reward” for
being below the bound, or a penalty, for being above
the bound.
We assume that the centralized controller knows
x
i
(k), i = 1...N, k = 0...T , and x
i
(see Fig. 3).
Step by step, the value c
x
i
− x
i
(k)
if positive has
to be considered as a reward, if negative is a penalty
to be paid. The value F
i
(k) in equation (1) is a pa-
rameter each municipality can decide by its own, to
distribute in time the transformability power: the idea
is that of gradually reducing the transformed areas, in
order to make this process feasible and socially sus-
tainable. Therefore it is a tool to plan the future ac-
tions, on the base of the deviation of the measured
state with respect to the bound.
3.2 Multi-municipalities Model
In this case we assume that the municipalities can buy
the transformability right from other municipalities,
LUC-LandUptakeControl-AGIS-basedApproach
453

Figure 3: Single-municipality model. R is the central con-
troller (regional authority). Mi is the ith municipality.
with a price determined by the market. Therefore we
need another state variable, x
i
(k), which is the resid-
ual amount of urbanizable area at time k, which is ini-
tialized at time 0 with the value x
i
, calculated as the
preceding section. The centralized controller knows
x
i
(k) (from the measurements) and x
i
(k) (communi-
cated by the municipality i), i = 1...N, k = 0...T . The
state equations become:
x
i
(k + 1) = x(k) + u
i
(k) + δ
i
(k) (2)
x
i
(k + 1) = x
i
(k) +
N
∑
j=1
ρ
i j
(k)x
j
(k) −
N
∑
j=1
η
i j
(k)x
i
(k)
x
i
(k) = x
i
k = 0, ..., T − 1, i = 1..N
where
ρ
i j
(k) ≥ 0, η
i j
(k) ≥ 0
ρ
ii
(k) = 0, η
ii
(k) = 0
0 ≤
N
∑
j=1
η
i j
(k) ≤ 1
η
i j
(k) = ρ
ji
(k)
and it is reasonable to assume that
ρ
i j
(k)η
i j
(k) = 0
i.e. it is not possible for municipality i to buy and to
sell the urban transformation right, at the same time,
with an interaction with the same municipality j. See
Fig. 4 .
The input is
u
i
(k) = F
i
(k)
x
i
(k) − x
i
(k)
, if x
i
(k) ≥ x
i
(k)
u
i
(k) = 0, if x
i
(k) < x
i
(k)
and
J
T
= c
T
∑
k=1
x
i
(k) − x
i
(k)
Figure 4: Multi-municipalities model. Dashed arrows de-
note interactions among municipalities.
3.3 Multi-municipalities Model with
Receding Horizon
Consider a modified version of multi-municipalities
model, with the additional input h
i
(k), and with un-
bounded time horizon
x
i
(k + 1) = x(k) + u
i
(k) + δ
i
(k) (3)
x
i
(k + 1) = x
i
(k) +
N
∑
j=1
ρ
i j
(k)x
j
(k)+
−
N
∑
j=1
η
i j
(k)x
i
(k) + h
i
(k)
x
i
(k) = x
i
k = 0, 1, 2..., i = 1..N
Let σ be the standard and p
iH
(k) the value of pop-
ulation at time k + H, as predicted at time k. Such a
value will be in general a function of the population
records of the municipality i, in the time interval 0...k,
denoted by p
|
[0,k]
. In symbols,
p
iH
(k) = f
i
( p
|
[0,k]
)
The value h
i
(k) depends on p
iH
(k) and on p
iH
(k −1),
as defined in the next Table.4.
Let ∆
i
x
(k) = x
i
(k) − x
i
(k), ∆
i
pH
(k) = p
i
H
(k) −
p
i
H
(k − 1). Then
if ∆
i
x
(k) < 0 and ∆
i
pH
(k) < 0 h
i
(k) = 0
if ∆
i
x
(k) ≥ 0 and ∆
i
pH
(k) < 0 h
i
(k) = −γ
if ∆
i
x
(k) < 0 and ∆
i
pH
(k) ≥ 0 h = σ∆
i
pH
(k)
if ∆
i
x
(k) ≥ 0 and ∆
i
pH
(k) ≥ 0 h = σ∆
i
pH
(k)
(4)
where
γ = min
−σ∆
i
pH
(k), ∆
i
x
(k)
See Fig. 5 for a pictorial description.
ICINCO2013-10thInternationalConferenceonInformaticsinControl,AutomationandRobotics
454

Figure 5: Multi-municipalities model with receding hori-
zon.
The input is
u
i
(k) = F
i
(k)
x
i
(k) − x
i
(k)
, if x
i
(k) ≥ x
i
(k)
u
i
(k) = 0, if x
i
(k) < x
i
(k)
and
J
k
= c
k
∑
j=1
x
i
( j) − x
i
( j)
4 SPRAWL CONTROL
The cost function defined in the above models can
take into account also the problem of sprawl control.
Let σ
i
(k) some sprawl index (e.g. as defined in (Ro-
mano, 2004)), of municipality i at time k. Let s be
a positive real, so that s ∗
σ
i
(k − 1) − σ
i
(k)
, if pos-
itive, is a reward because the sprawl index decreased
in time period k, with respect to k − 1. Then the cost
function is defined as:
J
k
=
k
∑
j=1
c ∗
x
i
( j) − x
i
( j)
+ s ∗
σ
i
(k − 1) − σ
i
(k)
Moreover the function J could take into account also
other phenomena, related with the sprawl, and shape-
dependent. We do not dwell here on this point.
5 DISCUSSION
In this paper we described a land uptake control pro-
cedure. It is a first attempt to introduce the output-
feedback paradigm in the urban planning process. In-
stead of giving hard constraints on the land uptake
amount, our proposal is based on a reward-penalty
approach, by means of a suitable cost function, that
gives a monetary value to the untransformed land.
We considered three different models: the first one
is the simpler, but it is rather strict, since the bound
of the transformable land is given at the beginning
for each municipality, and it remain fixed, for all the
fixed, given a priori time horizon. The second model
consider the possibility of interaction among the mu-
nicipalities. In this case the bounds evolve in time,
and there is an adaptation mechanism, with respect to
the different needs of municipalities, while the over-
all regional urbanization bound is met. The last model
introduces the adaptation of the bounds also with re-
spect to the population dynamics, in the framework of
receding horizon philosophy. In this case the proce-
dure has the advantage of remaining effective for an
unbounded time horizon. Moreover, changes in the
bounds due to population variation are gradually con-
sidered, ad hence too hard variations are avoided (as
could happen for the first and the second model, when
at time T the parameters are reset, to start a new con-
trol period) with obvious advantages.
The models are very simple, but some work has to
be done for the parameters identification. Urbaniza-
tion standard are to be evaluated and shared in the sci-
entific and social communities. The time duration of
each time period has to be defined, in relation with the
availability of adjourned geographical data, time hori-
zons have to be defined, with respect to time constants
of the involved processes, and finally the parameters
in the cost function has to be carefully designed, in or-
der to produce the desired control effects. The simu-
lation of the models, with the construction of possible
scenarios could be useful.
In all the models we considered, the regional au-
thority has the task of maintaining the information on
the state of the system (or a ”land register”), super-
vising the process and computing the cost functions
for each municipality. But its control role could be
emphasized, if one consider the possibility of incen-
tivizing exchanges of transformation rights between
municipalities, as in the second and third model, when
there is some overall interest in the transaction. Only
to give an example, suppose there is municipality i
that wants to buy from municipality j some more ad-
ditional transformable area, needed for a new factory
settlement. Municipality j could agree to sell this
right, because, for geographic proximity the factory
will be an opportunity also for its citizens. On the
other side municipality j could own natural resources,
which furnish ecosystem services to municipality i, as
well as to others municipalities. Hence this kind of
transaction could be incentivized by regional author-
LUC-LandUptakeControl-AGIS-basedApproach
455

ity.
In the framework we defined, a number of addi-
tional problems can be considered. For example in
the third model the current urbanization bound is ad-
journed with respect to the expected future popula-
tion. But the population level could in some case de-
pend on the actual planned new urbanization. Hence
there is a modelling problem to be solved. As an opti-
mization example problem, we can consider the case
in which a municipality wants to maximize its util-
ity, by deciding the amount of transformation rights
to sell (or to buy) in each time period, and their price,
known the state of the system and the population
trend.
Finally, at this stage, the LUC procedure is just a
proposal, based on technical ideas and analysis. In
order to become effective, normative and legal frame-
work should be faced. This task is beyond the scope
of this paper.
REFERENCES
Camacho, E. and Bordons, C. (1995). Model predictive
control in the process industry. Springer Verlag, New
York.
EEA (2006). European commission, urban sprawl in eu-
rope, the ignored challenge. Directorate general Joint
Researche Center, Copenaghen.
Ellis, C. and Ramankutty, N. (2008). Putting people in the
map: anthropogenic biomes of the world. Frontier in
Ecology and the Environment: 6.
Glaeser, E. (2011). Triumph of the City: How Our Greatest
Invention Makes Us Richer, Smarter, Greener, Health-
ier, and Happier. The Penguin Press.
Henger, R. and Bizer, K. (2010). Tradable planning per-
mits for land-use control in germany. Land Use Pol-
icy, 27(3):843–852.
Lynette I. Millett and Deborah L. Estrin (2012). Computing
Research for Sustainability. The National Academies
Press.
McLoughlin, J. (1969). Urban and regional planning: A
systems approach. Faber and Faber.
Pileri, P. and Maggi, M. (2010). Sustainable planning? first
results in land uptakes in rural, natural and protected
areas: the lombardia case study (italy). Journal of
Land Use Science, 5(2):105–122.
Plotkin, S. (1987). Property, policy and politics: towards a
theory of urban land-use conflict. IJURR, 11(3):382–
404.
RNE (2008). Germany federal government, for a sustain-
able Germany. Progress Report 2008 on the National
Strategy for Sustainable Development.
Romano, B. (2004). Environmental fragmentation ten-
dency: the sprawl index. In ERSA 2004 Congress,
Porto, Portugal.
Romano, B. and Zullo, F. (2013a). Land urbanization in
central italy: 50 years of evolution. Journal of Land
Use Science. DOI:10.1080/1747423X.2012.754963.
Romano, B. and Zullo, F. (2013b). Models of urban land
use in europe assessment tools and criticalities. In-
ternational Journal of Agricultural and Environmen-
tal Information Systems. To appear.
Sala, E., Chapin, F., Armesto, J., Berlow, E., Bloom-
field, J., Dirzo, R., Huber-Sanwald, E., Huenneke,
L., Jackson, R., Kinzig, A., Leemans, R., Lodge, D.,
Mooney, H., Oesterheld, M., Poff, N., Sykes, M.,
Walker, B., Walker, M., and Wall, D. (2000). Global
biodiversity scenarios for the year 2100. Science,
287(5459):1770–1774.
Sharma, L., Pandey, P. C., and Nathawat, M. (2012). As-
sessment of land consumption rate with urban dynam-
ics change using geospatial techniques. Journal of
Land Use Science, 7(2):135–148.
Siedentop, S. and Fina, S. (2010). Monitoring urban sprawl
in Germany: towards a gis-based measurement and
assessment approach. Journal of Land Use Science,
5(2):73–104.
Soeterboek, R. (1992). Predictive control- a unified ap-
proach. Prentice Hall International.
Thompson, A. and Prokopy, L. S. (2009). Tracking urban
sprawl: Using spatial data to inform farmland preser-
vation policy. Land Use Policy, 26(2):194–202.
ICINCO2013-10thInternationalConferenceonInformaticsinControl,AutomationandRobotics
456
