Optical Couplers in Multimode MIMO Transmission Systems

Measurement Results and Performance Analysis

Andreas Ahrens and Steffen Lochmann

Hochschule Wismar, University of Technology, Business and Design, Philipp-M¨uller-Straße 14, 23966 Wismar, Germany

Keywords:

Multiple-Input Multiple-Output (MIMO) System, Optical Fibre Transmission, Multimode Fiber (MMF).

Abstract:

The concept of MIMO (multiple input multiple output) transmission over multimode ﬁbers has attracted in-

creasing interest within the last years. Theoretically, the performance of the optical MIMO multimode channel

is well predictable. However, the realization of the optical MIMO channel requires substantial further research.

In this work the efﬁciency of optical couplers in MIMO systems is studied in a 1,4 km multimode testbed.

Optical couplers have long been used as passive optical components able to combine or split SISO (single-

input single-output) data transmission from optical ﬁbers. Our results show by the obtained measured impulse

responses together with the simulated BER performance that optical couplers are well suited for the optical

MIMO transmission despite their insertion losses and asymmetries. Comparing the different couplers those

which maintain the different optical mode groups support the MIMO transmission more efﬁciently.

1 INTRODUCTION

In the recent past the concept of MIMO (multiple in-

put multiple output) transmission (K¨uhn, 2006; Fos-

chini, 1996) over multimode ﬁbers has attracted in-

creasing interest in the optical ﬁber transmission com-

munity, targeting at increased ﬁber capacity (Singer

et al., 2008; Aust et al., 2012). The ﬁber capacity

of a multimode ﬁber is limited by the modal disper-

sion compared to single-mode transmission where no

modal dispersion except for polarization exists.

In MIMO transmission dispersion can be used to

outperform the SISO transmission from an informa-

tion theoretic point of view leading to higher ﬁber

capacities and under practical circumstances to lower

bit-error probabilities. The description of the optical

MIMO channel has attracted attention and reached

a state of maturity (Singer et al., 2008; Hsu et al.,

2006; B¨ulow et al., 2011). However, the realiza-

tion of the optical MIMO channel requires substantial

further research (Sch¨ollmann and Rosenkranz, 2007;

Sch¨ollmann et al., 2008).

Against this background, the novel contribution

of this paper is the use of optical couplers within a

1,4 km multimode (2 × 2) MIMO testbed. Based

on the channel measurements, the different propaga-

tion paths within the MIMO system are described.

Together with the appropriate MIMO modeling and

the corresponding signal processing (e.g. singular

value decomposition), the efﬁciency of different opti-

cal couplers in MIMO communication is elaborated.

The remaining part of this contribution is orga-

nized as follows: Section 2 reviewsthe optical MIMO

basics. Practical issues of optical MIMO including

the use of optical couplers are introduced and dis-

cussed in section 3. The performance criteria used to

evaluate the different optical couplers are introduced

in section 4, while associated performance results are

presented and interpreted in section 5. Finally, section

6 provides our concluding remarks.

2 OPTICAL MIMO

An optical MIMO system can be formed by feeding

different sources of light into the ﬁber, which support

different optical modes. Theoretically, it can be done

by using two single mode ﬁbers as shown in Fig. 1.

The different sources of light lead to different power

distribution patterns at the ﬁber end depending on the

transmitter side light launch conditions. Fig. 2 high-

lights the measured mean power distribution pattern

at the end of a 1,4 km multimode ﬁbre. Together

with the appropriate receiver side spatial conﬁgura-

tions, i. e. spot and ring ﬁlter (see Fig. 3), the electri-

cal MIMO channel can be formed. Fig. 3 illustrates

the corresponding transmitter and receiver side con-

ﬁguration. Fig. 4 highlights the resulting electrical

398

Ahrens A. and Lochmann S..

Optical Couplers in Multimode MIMO Transmission Systems - Measurement Results and Performance Analysis.

DOI: 10.5220/0004497303980403

In Proceedings of the 4th International Conference on Data Communication Networking, 10th International Conference on e-Business and 4th

International Conference on Optical Communication Systems (OPTICS-2013), pages 398-403

ISBN: 978-989-8565-72-3

 2013 SCITEPRESS (Science and Technology Publications, Lda.)

Figure 1: Transmitter side conﬁguration with center and

offset light launch condition.

Figure 2: Measured mean power distribution pattern as

a function of the light launch position (left: eccentricity

δ = 0µm, right: eccentricity δ = 18µm); the dotted line rep-

resents the 50µm core size.

MIMO system model.

Investigation in (Ahrens et al., 2011) have shown

that an eccentricity of δ = 10µm and a mask diame-

ter of r = 15µm was found to be beneﬁcial for mini-

mizing the overall BER at a ﬁxed data rate. However

technologically an eccentricity of δ = 10µm can not

be realized with two single mode ﬁbres with a core

size of 10µm each. Therefore other transmitter side

light launch conditions are in the focus of interest.

3 PRACTICAL ISSUES

OF OPTICAL MIMO

A possible solution for feeding different sources of

light in parallel in the ﬁbre can be provided by optical

couplers. In this section, various optical couplers are

analyzed for their suitability in optical MIMO trans-

mission systems (see Fig. 5). The focus within this

section is on the transmitter side.

It is well known that optical couplers may show a

very mode selective behavior (Lochmann and Becker,

1983). In general this behavior depends on the fabri-

cation technique (Lochmann et al., 1983). Although

the term ’mode selectivity’ usually referred to the un-

wanted coupling ratio’s dependency on the launching

conditions we can make use of this parameter to con-

trol or better to maintain the mode groups within such

a device.

10µm

Figure 3: Forming the optical MIMO channel (left: light

launch positions at the transmitter side with a given eccen-

tricity δ, right: spatial conﬁguration at the receiver side as a

function of the mask diameter r).

s 1

(t)

s 2

(t)

k 1

(t)

k 2

(t)

Figure 4: Electrical MIMO system model (example: n = 2).

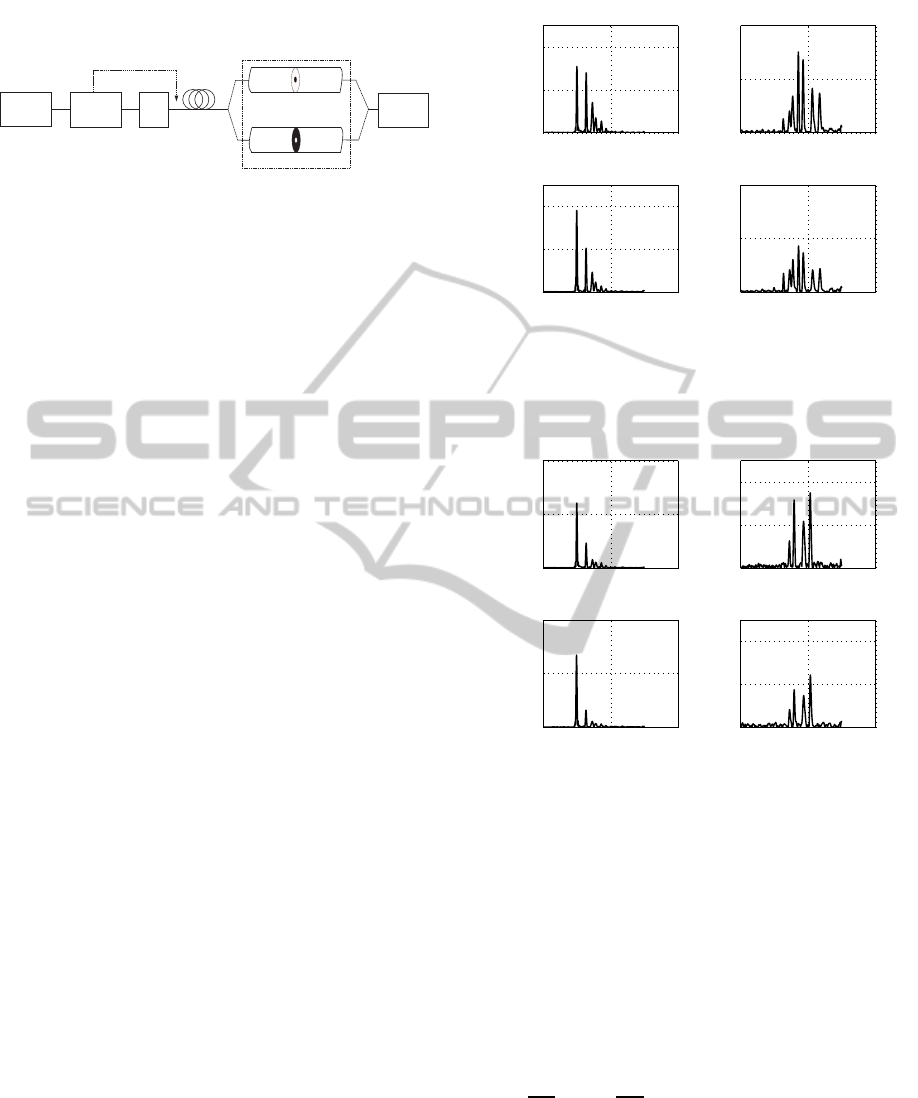
Fig. 6–9 show the measured mean power distri-

bution patterns at the ﬁbre end when using different

couplers at the transmitter side for feeding different

sources of light into the ﬁbre. The obtained intensity

patterns aren’t normalized nor related to each other.

However, a good insight into the spatial mode struc-

ture is obtained.

(low order mode path)

(high order mode path)

Figure 5: Transmitter side coupler for launching different

sources of light into the MMF.

Due to its inherent coupling mechanism the so

called surface or evanescent ﬁeld couplers, e.g. the

fusion couplers start to couple light into the neigh-

bor waveguide with the high order modes ﬁrst. The

strength of this behavior can be controlled by the de-

gree of fusion. Therefore, the fusion coupler with the

asymmetric coupling ratio which is realized by ap-

plying a low degree of fusion can easily split mode

groups into high and low orders or combine them as

can be seen when comparing Fig. 6 and 7. Unfortu-

nately these asymmetric fusion couplers give also rise

OpticalCouplersinMultimodeMIMOTransmissionSystems-MeasurementResultsandPerformanceAnalysis

399

Figure 6: Measured mean power distribution pattern when

using the symmetric fusion coupler (SFC) at the transmitter

side (left: center light launch condition; right: off-center

light launch condition, δ = 11µm); the dotted line represents

the 50µm core size.

Figure 7: Measured mean power distribution pattern when

using the asymmetric fusion coupler (AFC) at the transmit-

ter side (left: center light launch condition; right: off-center

light launch condition, δ = 15µm); the dotted line represents

the 50µm core size.

to very asymmetric MIMO channels which is caused

by their coupling ratio (see Tab. 1). The coupling ra-

tio a

can be obtained from the insertion losses a

and a

and results in

= |a

− a

| . (1)

Table 1: Parameters of the transmitter-side couplers mea-

sured with restricted mode launch conditions as speciﬁed in

Fig. 6–9 (in dB).

SFC AFC PC MC

excess loss a

2,3 2,5 3,2 3,8

coupling ratio a

2,0 7,8 0,7 1,4

On the other hand ’butt-end’ or end face couplers

are usually considered to be less mode selective which

is not true in general as can be seen from both Fig. 8

and Fig. 9.

Here the polished coupler shows strong support of

the fundamental mode but it mixes several high order

mode groups. Since it is all but impossible to pol-

ish the two ﬁber branches symmetrically and the op-

tical modes respond very sensitive to these geomet-

rical distortions the polished couplers show more or

less mode selective behavior, too. Compared to fu-

sion couplers they have the disadvantage of generally

Figure 8: Measured mean power distribution pattern when

using the polished coupler (PC) at the transmitter side (left:

center light launch condition; right: high order mode path,

δ = 0µm); the dotted line represents the 50µm core size.

Figure 9: Measured mean power distribution pattern when

using the mirror coupler (MC) at the transmitter side (left:

center light launch condition; right: off-center light launch

condition, δ = 10µm); the dotted line represents the 50µm

core size.

higher excess losses (see Tab. 1). The same holds true

for couplers using micro optical parts, e. g. the mirror

coupler. However, they may offer better mode control

due to the easy access to the expanded optical ﬁelds.

Fig. 9 shows qualitatively that the high order mode

group is better maintained in comparison to the pol-

ished coupler in Fig. 8. In summery the three main pa-

rameters of couplers depend on fabrication techniques

as indicated in Fig. 10.

Now, all impulse responses have been measured as

described in (Pankow et al., 2011) and (Ahrens et al.,

maintaining

selective

mode groups:

excess loss:

coupling ratio:

strong

weak

low

high

symmetry can be controlled by more or less all fabrication techniques

but may be linked to mode selectivity

asymmetric

fusion coupler

symmetric

fusion coupler

polished

coupler

mirror

coupler

<< <

in-fiber devices: micro optic devices:

asymmetric

fusion coupler

mirror

coupler

polished

coupler

symmetric

fusion coupler

Figure 10: Relationship between fabrication techniques and

coupler parameters.

OPTICS2013-InternationalConferenceonOpticalCommunicationSystems

400

2013) (see Fig. 11).

Pulse

Generator

Attenuator

fixed transmit

Power

Coupler

GI 50/125

50/125

ring

center

Spatial Filter Characteristic

Oscilloscope

Analysis

Figure 11: Measurement setup for measuring the MIMO

speciﬁc impulse responses.

In order to exclude the impact of the different ex-

cess losses of the couplers (see Tab. 1) which can be

related to fabrication deﬁciencies the overall powers

at output 1 (Fig. 5) were equalized.

Though the power is equalized it spreads across

the different mode groups supported by the respec-

tive couplers. Therefore different couplers will pro-

duce differing MIMO channels. Comparing g

(t)

in Fig. 12 to 15 the power spreading across the high

order modes is emphasized. This is expected since

the number of excited mode groups increases with

the radial launching offset δ. However the different

couplers may further increase this number by mode

coupling. The lowest spreading shows the asymmet-

ric fusion coupler with four mode groups whereas the

polished coupler produces eight mode groups. Like-

wise the fundamental mode is mainly supported by

both the asymmetric fusion and the polished couplers

whereas the other couplers also excite the next higher

mode. The disadvantage of the unequal coupling ratio

of an asymmetric fusion coupler compared to a sym-

metric one is not as evident as expected which can be

seen from g

(t) in Fig. 12 and 13. This is caused by

the restricted mode launching conditions and how the

coupler maintains certain modes.

4 PERFORMANCE CRITERIA

For the performance evaluation of the different

MIMO conﬁgurations, coherent transmission and de-

tection is assumed together with the modulation for-

mat QAM (quadrature amplitude modulation) per

MIMO transmission mode. The block-oriented sys-

tem for frequency selective channels is modeled by:

u = H· c+ w . (2)

In (2), the transmitted signal vector c is mapped

by the channel matrix H onto the received vector

u. Finally, the vector of the additive, white Gaus-

sian noise (AWGN) is deﬁned by w (Pankow et al.,

2011; Raleigh and Ciofﬁ, 1998). Details on the

transmission model, which has been determined by

0 2 4

0.2

0.4

0 2 4

0.05

0.1

0 2 4

0.2

0.4

0 2 4

0.05

0.1

t (in ns) →t (in ns) →

1 1

(t) →

1 2

(t) →

2 1

(t) →

2 2

(t) →

Figure 12: Measured electrical MIMO impulse responses

with respect to the pulse frequency f

= 1/T

= 5,00 GHz

at 1326 nm operating wavelength when using the symmetric

fusion coupler at the transmitter side.

0 2 4

0.5

0 2 4

0.02

0.04

0 2 4

0.5

0 2 4

0.02

0.04

t (in ns) →t (in ns) →

1 1

(t) →

1 2

(t) →

2 1

(t) →T

2 2

(t) →

Figure 13: Measured electrical MIMO impulse responses

with respect to the pulse frequency f

= 1/T

= 5,00 GHz

at 1326 nm operating wavelength when using the asymmet-

ric fusion coupler at the transmitter side.

channel measurements, are given in (Pankow et al.,

2011). Singular-value decomposition (SVD) can now

be used to transfer the whole MIMO system into in-

dependent, non-interfering layers exhibiting unequal

gains per layer as highlighted in Fig. 16, where

as a result weighted additive, white Gaussian noise

(AWGN) channels appear. The data symbols at the

time k, i. e. c

and c

are weighted by the positive

square roots of the eigenvalues of the matrix H

i. e.

and

. Finally, some noise is added,

i. e. w

and w

OpticalCouplersinMultimodeMIMOTransmissionSystems-MeasurementResultsandPerformanceAnalysis

401

0 2 4

0.2

0.4

0 2 4

0.1

0.2

0 2 4

0.2

0.4

0 2 4

0.1

0.2

t (in ns) →t (inns) →

1 1

(t) →

1 2

(t) →

2 1

(t) →

2 2

(t) →

Figure 14: Measured electrical MIMO impulse responses

with respect to the pulse frequency f

= 1/T

= 5,00 GHz

at 1326 nm operating wavelength when using the polished

coupler at the transmitter side.

0 2 4

0.2

0.4

0 2 4

0.1

0.2

0 2 4

0.2

0.4

0 2 4

0.1

0.2

t (in ns) →t (inns) →

1 1

(t) →

1 2

(t) →

2 1

(t) →

2 2

(t) →

Figure 15: Measured electrical MIMO impulse responses

with respect to the pulse frequency f

= 1/T

= 5,00 GHz

at 1326 nm operating wavelength when using the mirror

coupler at the transmitter side.

5 RESULTS

For comparing the different MIMO conﬁgurations, a

ﬁxed transmission bit rate is assumed. Furthermore,

for numerical analysis it is assumed, that each optical

input within the multimode ﬁber is fed by a system

with identical mean properties with respect to trans-

mit ﬁlter and pulse frequency f

= 1/T

. Rectangu-

lar pulses are used for transmit and receive ﬁltering.

For numerical assessment within this paper, the pulse

frequency is chosen to be f

= 5,00 GHz, the aver-

age transmit power is supposed to be P

= 1V

– this

equals 1 W at a linear and constant resistance of 1Ω –

and as an external disturbance a white Gaussian noise

with power spectral density N

is assumed (Pankow

1 k

2 k

1 k

2 k

1 k

2 k

1 k

2 k

Figure 16: SVD-based layer-speciﬁc transmission model.

et al., 2011). Tab. 2 highlights the different transmis-

sion modes to be investigated when minimizing the

overall BER.

In order to transmit at a ﬁxed data rate while main-

taining the best possible integrity, i.e. bit-error rate

(BER), an appropriate number of MIMO layers has

to be used, which depends on the speciﬁc QAM con-

stellation size as well as the layer-speciﬁc weighting

factors, i.e.

and

Table 2: Parameters for bitloading: Investigated QAM

transmission modes for ﬁxed transmission bit rate.

throughput layer 1 layer 2

4 bit/s/Hz 16 0

4 bit/s/Hz 4 4

20 25 30 35 40

−6

−4

−2

10 · log

) (in dB) →

bit-error rate →

16-QAM, SISO

(16,0)-QAM, MIMO

(4,4)-QAM, MIMO

Figure 17: BER performance at 1326 nm operating wave-

length when using the asymmetric fusion coupler at the

transmitter side, the transmission modes introduced in

Tab. 2 and transmitting 4 bit/s/Hz over frequency selective

optical MIMO channels.

For a given MIMO conﬁguration, i.e. the asym-

metric fusion coupler at the transmitter side and the

spatial ﬁlters at the receiver side, the corresponding

BER performance is depicted in Fig. 17. As shown

by the BER results, the achievable performance of

the MIMO system is strongly affected by the number

of bits transmitted per activated MIMO layer. Using

SVD, the singular values are ordered in descending

order. That’s why only the strongest layers should be

OPTICS2013-InternationalConferenceonOpticalCommunicationSystems

402

used for the data transmission with appropriate QAM

modulation levels.

20 25 30 35 40

−6

−4

−2

10 · log

) (in dB) →

bit-error rate →

SFC

AFC

Figure 18: Coupler speciﬁc BER performance at 1326 nm

operating wavelength when using the (16,0) QAM trans-

mission mode.

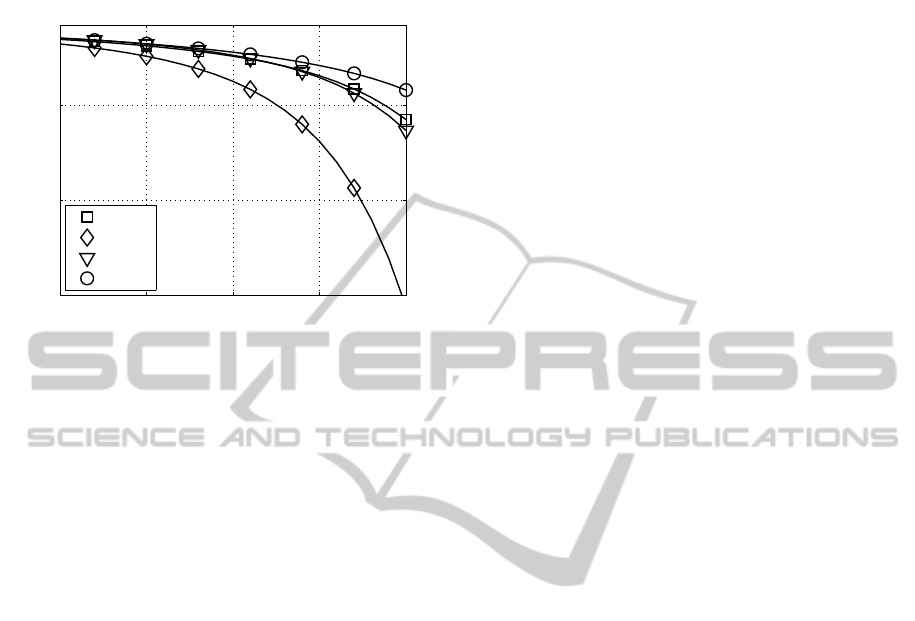
Fig. 18 shows the obtained BER performance

when using the different transmitter side coupler con-

ﬁgurations. As highlighted by the BER results, the

asymmetric fusion coupler (AFC) shows the best per-

formance among the investigated coupler conﬁgura-

tions. Therein, unequal coupling ratio of an asymmet-

ric fusion coupler seems to be highly beneﬁcial when

minimizing the overall BER.

6 CONCLUSIONS

In this work the use of optical couplers in a (2 × 2)

MIMO testbed is studied and analyzed for a 1,4 km

multimode MIMO channel. As shown by the obtained

measured impulse responses as well as the simulated

BER results, optical couplers are well suited for the

optical MIMO transmission. As shown by the simu-

lation results, couplersmaintaining the different mode

groups without additional mixing seem to be beneﬁ-

cial when minimizing the overall BER.

REFERENCES

Ahrens, A., Pankow, J., Aust, S., and Lochmann, S. (2011).

Optical MIMO Multimode Fiber Links: Channel

Measurements and System Performance Analysis. In

International Conference on Optical Communication

Systems (OPTICS), Seville (Spain).

Ahrens, A., Schr¨oder, S., and Lochmann, S. (2013). Disper-

sion Analysis within a Measured 1,4 km MIMO Mul-

timode Channel. In International Conference on Op-

tical Communication Systems (OPTICS), Reykjavik

(Iceland).

Aust, S., Ahrens, A., and Lochmann, S. (2012). Channel-

Encoded and SVD-assisted MIMO Multimode Trans-

mission Schemes with Iterative Detection. In Interna-

tional Conference on Optical Communication Systems

(OPTICS), pages 353–360, Rom (Italy).

B¨ulow, H., Al-Hashimi, H., and Schmauss, B. (2011).

Coherent Multimode-Fiber MIMO Transmission with

Spatial Constellation Modulation. In European Con-

ference and Exhibition on Optical Communication

(ECOC), Geneva, Switzerland.

Foschini, G. J. (1996). Layered Space-Time Architecture

for Wireless Communication in a Fading Environment

when using Multiple Antennas. Bell Labs Technical

Journal, 1(2):41–59.

Hsu, R. C. J., Tarighat, A., Shah, A., Sayed, A. H., and

Jalali, B. (2006). Capacity Enhancement in Coherent

Optical MIMO (COMIMO) Multimode Fiber Links.

IEEE Communications Letters, 10(3):195–197.

K¨uhn, V. (2006). Wireless Communications over MIMO

Channels – Applications to CDMA and Multiple An-

tenna Systems. Wiley, Chichester.

Lochmann, S. and Becker, M. (1983). Cover Photo of the

Journal. Nachrichtentechnik, Elektronik, 33(11).

Lochmann, S., Scheel, W., Labs, J., and Wallstein,

T. (1983). Passive Optical Splitters (in German).

Nachrichtentechnik, Elektronik, 33(11):444–448.

Pankow, J., Aust, S., Lochmann, S., and Ahrens, A. (2011).

Modulation-Mode Assignment in SVD-assisted Op-

tical MIMO Multimode Fiber Links. In 15th Inter-

national Conference on Optical Network Design and

Modeling (ONDM), Bologna (Italy).

Raleigh, G. G. and Ciofﬁ, J. M. (1998). Spatio-Temporal

Coding for Wireless Communication. IEEE Transac-

tions on Communications, 46(3):357–366.

Sch¨ollmann, S. and Rosenkranz, W. (2007). Experimen-

tal Equalization of Crosstalk in a 2 x 2 MIMO Sys-

tem Based on Mode Group Diversity Multiplexing

in MMF Systems @ 10.7 Gb/s. In 33rd European

Conference and Exhibition on Optical Communica-

tion (ECOC), Berlin.

Sch¨ollmann, S., Schrammar, N., and Rosenkranz, W.

(2008). Experimental Realisation of 3 x 3 MIMO Sys-

tem with Mode Group Diversity Multiplexing Limited

by Modal Noise. In Optical Fiber Communication

Conference (OFC), San Diego, California.

Singer, A. C., Shanbhag, N. R., and Bae, H.-M. (2008).

Electronic Dispersion Compensation– An Overwiew

of Optical Communications Systems. IEEE Signal

Processing Magazine, 25(6):110 – 130.

OpticalCouplersinMultimodeMIMOTransmissionSystems-MeasurementResultsandPerformanceAnalysis

403