Equalisation of Measured Optical MIMO Channels

Andr´e Sandmann, Andreas Ahrens and Steffen Lochmann

Hochschule Wismar, University of Technology, Business and Design, Philipp-M¨uller-Straße 14, 23966 Wismar, Germany

Keywords:

Multiple-Input Multiple-Output System, Optical Fibre Transmission, Multimode ﬁber (MMF), Signal

Deconvolution, Equalisation.

Abstract:

Within the last years multiple-input multiple-output (MIMO) transmission has reached a lot of attention in

the optical ﬁbre community. Theoretically, the concept of MIMO is well understood. However, practical

implementations of optical components are in the focus of interest for further computer simulations. That’s

why in this contribution the speciﬁc impulse responses of the (2 × 2) MIMO channel, including a 1.4 km

multi-mode ﬁbre and optical couplers at both ends, are measured for operating wavelengths of 1326 nm and

1576 nm. Since semiconductor diode lasers, capable of working at different wavelengths, are used for the

characterization of the underlying optical MIMO channel, inverse ﬁltering is needed for obtaining the respec-

tive impulse responses. However, the process of inverse ﬁltering also known as signal deconvolution is critical

in noisy environments. That’s why different approaches such as Wiener and parametric ﬁltering are studied

with respect to different optimization criteria. Using these obtained impulse responses a baseband MIMO data

transmission is modelled. In order to create orthogonal channels enabling a successful transmission, a MIMO

zero forcing (ZF) equaliser is implemented and analysed. Our main results given as an open eye-diagram and

calculated bit-error rates show the successful implementation of the MIMO transmission system.

1 INTRODUCTION

Aiming at further increasing the ﬁbre capacity in opti-

cal transmission systems the concept of MIMO, well

studied and wide-spread in radio transmission sys-

tems, has led to increased research activities in this

area (Singer et al., 2008; Winzer, 2012; Richardson

et al., 2013). Theoretical investigations have shown

that similar capacity increases are possible compared

to wireless systems (K¨uhn, 2006; Tse and Viswanath,

2005). The basis for this approach is the exploitation

of the different optical mode groups. However, the

practical implementation has to cope with many tech-

nological obstacles such as mode multiplexing and

management. This includes mode combining, mode

maintenance and mode splitting. In order to improve

existing simulation tools practical measurements are

needed. That’s why in this contribution a whole opti-

cal transmission testbed is characterized by its respec-

tive impulse responses obtained by high-bandwidth

measurements.

In order to describe the optical MIMO testbed at

different operating wavelengths semiconductor laser

diodes with a pulse width of 25 ps are used. Since

the used picosecond laser generator doesn’t guaran-

tee a fully ﬂat frequency spectrum in the region of

interest, inverse ﬁltering has to be applied to obtain

the MIMO impulse responses. However, the process

of inverse ﬁltering also known as signal deconvolu-

tion is critical in noisy environments. That’s why dif-

ferent approaches such as Wiener and parametric ﬁl-

tering are studied with respect to different optimiza-

tion criteria such as the mean square error (MSE)

and the imaginary error parameter introduced by Gans

(Gans, 1986). Using the measured impulse responses

a MIMO baseband transmission system can be con-

structed. In order to exploit the full potential of the

MIMO system, properly selected signal processing

strategies haveto be applied. The focus of this work is

on the whole testbed functionality including the signal

processing needed to separate the data streams. Based

on computer simulations the end-to-end functionality

of the whole testbed is demonstrated and appropriate

quality criteria such as the eye-diagram and the the

bit-error rate (BER) are calculated.

The novelty of this paper is given by the proven

testbed functionality, which includes the whole

electro-optical path with the essential optical MIMO

components of mode combining and splitting. The

next logical step is the implementation of the MIMO

Sandmann A., Ahrens A. and Lochmann S..

Equalisation of Measured Optical MIMO Channels.

DOI: 10.5220/0005019600370044

In Proceedings of the 5th International Conference on Optical Communication Systems (OPTICS-2014), pages 37-44

ISBN: 978-989-758-044-4

 2014 SCITEPRESS (Science and Technology Publications, Lda.)

receiver modules such as automatic clock recovery,

frame synchronisation, channel estimation and equal-

isation as demonstrated in (K¨ohnke et al., 2014).

The remaining part of the paper is structured as

follows: In section 2 the optical MIMO testbed and

its corresponding system model are introduced. The

further processing of the measured impulse responses,

which is carried out by inverse ﬁltering, is described

in section 3. The obtained results are given in Section

4. Finally, Section 5 shows our concluding remarks.

2 OPTICAL MIMO SYSTEM

MODEL

An optical MIMO system can be formed by feeding

different sources of light into the ﬁbre, which activate

different optical mode groups. This can be carried out

by using centric and eccentric light launching condi-

tions and subsequent combining of the activated dif-

ferent mode groups with a fusion coupler as show in

Fig. 1 (Ahrens and Lochmann, 2013; Sandmann et al.,

2014).

(low order mode path)

(high order mode path)

Figure 1: Transmitter side fusion coupler for launching dif-

ferent sources of light into the MMF.

The different sources of light lead to different

power distribution patterns at the ﬁbre end depending

on the transmitter side light launch conditions. Fig. 2

highlights the measured mean power distribution pat-

tern at the end of a 1.4 km multi-mode ﬁbre. At the

end of the MMF transmission line a similar fusion

coupler is used for splitting the different mode groups.

The measurement setup depicted in Fig. 3 shows

the testbed with the utilized devices for measuring

the system properties of the optical MIMO channel

in form of its speciﬁc impulse responses needed for

modelling the MIMO data transmission.

A picosecond laser unit is chosen for generating

the 25 ps input pulse. This input pulse is used to

measure separately the different single-input single-

output (SISO) channels within the MIMO system.

Since the used picosecond laser unit doesn’t guar-

antee a fully ﬂat frequency spectrum in the region

of interest, the captured signals have to be decon-

volved. The obtained impulse responses are forming

the base for modelling the MIMO transmission sys-

Figure 2: Measured mean power distribution pattern when

using the fusion coupler at the transmitter side (left: cen-

tric mode excitation; right: eccentric mode excitation); the

dotted line represents the 50µm core size.

tem. Fig. 4 highlights the resulting electrical MIMO

system model.

s 1

(t)

s 2

(t)

k 1

(t)

k 2

(t)

Figure 4: Electrical MIMO system model (example: n = 2)

3 MEASUREMENT CAMPAIGN

AND SIGNAL

DECONVOLUTION

Since the process of signal deconvolution is critical in

noisy environments, different ﬁltering processes such

as Wiener and parametric ﬁltering are studied in order

to guarantee a high quality of the deconvolution pro-

cess deﬁned by the mean square error (MSE) and the

imaginary error parameter introduced by Gans (Gans,

1986).

A linear time-invariant system is deﬁned uniquely

by its impulse response, or its Fourier transform as

the corresponding transfer function. For the determi-

nation of the impulse response g

(t) (see also Fig. 5)

an appropriate formed input signal u

(t) is needed.

Unfortunately, an ideal Dirac delta pulse with a fre-

quency independent transfer function is practically

not viable. In real systems adequate impulses com-

pared to the Dirac delta pulse must be used. For

the determination of the impulse response in optical

transmission systems impulses as speciﬁed in (Ahrens

OPTICS2014-InternationalConferenceonOpticalCommunicationSystems

Light Launching Unit (splicer) 1.4 km multi-mode fibre channel Sampling Oscilloscope with MSM Photo Detector

Picosecond Laser Laser-diode ( ≈ 1.3 μm or 1.55 µm)Fusion Couplers

Figure 3: Measurement setup for determining the MIMO speciﬁc impulse responses.

et al., 2013) have proven to be useful. Addition-

ally, when analysing the characteristics of any prac-

tical system, the measured impulse u

(t) is affected

by noise. The resulting transmission system model is

depicted in Fig. 5. The measured impulse u

(t) can

(t)

n(t)

h(t)

(t) u

(t)

Figure 5: Transmission system model.

be decomposed into two parts, namely, the low-pass

ﬁltered output signal u

(t) and the noise part n(t) re-

sulting in

(t) = u

(t) ∗ g

(t) + n(t) . (1)

In the absence of the noise term, i. e. n(t) = 0, the

system characteristic g

(t) can be easily obtained by

inverse ﬁltering and is given as

(t)

( f) =

( f)

. (2)

Unfortunately, the measured impulse u

(t) is affected

by the noise term n(t), which is assumed the be white

and Gaussian distributed. Under these conditions in-

verse ﬁltering is not working properly anymore. In

order to improve the quality of the signal deconvolu-

tion different ﬁlter functions h(t) are applied and the

ﬁltered signal results in

(t) = u

(t) ∗ g

(t) ∗ h(t) + n(t) ∗ h(t) . (3)

This ﬁlter operation affects both the low-pass ﬁltered

output signal u

(t) and the noise term n(t). With an

appropriate selected ﬁlter function the estimation of

the impulse response g

(t) yields to

ˆg

(t)

( f) =

( f)

. (4)

Hereinafter, two different ﬁlter functions are stud-

ied to estimate the impulse response g

(t) based on

the measured impulse u

(t). Commonly, the mean

square error (MSE) between the impulse response

(t) and the estimated impulse response ˆg

(t) is cho-

sen as a quality indicator. It is expressed as

MSE

= E{[g

(t) − ˆg

(t)]

} −→ min. , (5)

where E{·} denotes the expectation functional.

Firstly, the Wiener ﬁlter h

(t) is investigated. It is

based on ﬁnding the optimal solution for minimizing

the MSE when comparing the signal u

(t) with the

ﬁlter output signal u

(t). It is calculated as

E{[u

(t) − u

(t) ∗ h

(t)]

} −→ min. , (6)

Assuming the signal u

(t) and the noise n(t) are un-

correlated, the Wiener ﬁlter transfer function results

in (Vaseghi, 2000, pp. 191-194)

( f) =

( f)

( f)+ S

( f)

, (7)

where S

( f) is the power spectral density (PSD) of

the signal u

(t) and S

( f) is the noise PSD of the

signal n(t).

A more simple ﬁlter choice when estimating the

impulse response g

(t) is represented by predeﬁned

parametric ﬁlter functions. A possible transfer func-

tion presented in (Nahman and Guillaume, 1981) and

EqualisationofMeasuredOpticalMIMOChannels

studied more closely in (Sandmann et al., 2013) is

given by

( f) =

( f)|

+ γ· |C( f)|

, γ ∈ R , (8)

with:

|C( f)|

= 6− 8cos(2πfT

) + 2cos(4πfT

) , (9)

where T

is the sampling period. The regularisation

function H

( f) is a low-pass ﬁlter with the parame-

ter γ inﬂuencing the sharpness of the ﬁlter and hence

determining the cutoff frequency. In order to appro-

priately select this parameter the MSE criterion (5)

can be applied for the optimisation. In practical mea-

surements the knowledge of the original impulse re-

sponse g

(t) is not given. Therefore, another criterion

is needed in order to properly select the γ-parameter

for practical measurements. A promising criterion

was introduced by Gans (Gans, 1986), where the root

mean square of the deconvolved imaginary part of

ˆg

(t) is used for ﬁnding the parameter of the regu-

larisation function. This optimisation criterion can be

expressed as

Gans

= E{[Im{ ˆg

(t,γ)}]

} −→ min. . (10)

Using this criterion multiple local minima can occur

and therefore another criterion described by Nahman

and Guillaume in (Nahman and Guillaume, 1981, pp.

22) should be taken into consideration when choos-

ing the γ value of the regularisation ﬁlter. This error

criterion is deﬁned as the MSE between the measured

receive signal u

(t) and the simulated receive signal

(t) ∗ ˆg

(t,γ), where u

(t) is the measured input im-

pulse. It is described as follows

Error

(γ) = E{[u

(t) − u

(t) ∗ ˆg

(t,γ)]

} . (11)

In order to compare the quality of the estimated

impulse responses using the regularisation ﬁlter to the

quality achieved by the Wiener ﬁlter, the following

system is studied: The input impulse is a Dirac delta

pulse with u

(t) = U

δ(t), withU

= 1 V, T

= 1 ms

and T

= 20. The chosen impulse response is

(t) =

rect





. (12)

In this case the ﬁlter output signal u

(t) is an rectan-

gular impulse with the amplitude U

. The deconvolu-

tion quality results are depicted in Fig. 6 as a function

of the signal-to-noise-ratio E

with the parameter

deﬁning the signal energy of u

(t) and the noise

power spectral density N

of the signal n(t).

When applying the regularisation ﬁlter H

( f) the

optimal γ values as well as the MSE are decreasing

25 30 35 40 45 50

10 · lg(E

) (indB) →

MSE

→

without ﬁltering

(f) with MSE Criterion

Wiener ﬁlter

Figure 6: Quality F

MSE

of the deconvolved impulse re-

sponses as a function of signal energy to noise power spec-

tral density using different ﬁlter functions.

with increasing E

. Therein the parameter E

de-

scribes the average symbol energy und N

the power

spectral density of the white noise process n(t). The

achievable quality of the estimated impulse responses

using the regularisation ﬁlter together with the MSE

optimisation criterion comes close to the Wiener ﬁlter

results. The beneﬁt of using a ﬁlter function is clearly

visible.

In order to determine the quality of the estimated

impulse responses, which are practically obtainable

using the Gans’ criterion (10), the following opti-

cal system conﬁguration at a operating wavelength at

1576 nm is studied: The measured input impulse of

the picosecond laser is depicted in Fig. 7 for different

operating wavelengths with a pulse width of approxi-

mately 25 ps. The impulse response is now assumed

0 0.1 0.2 0.3 0.4 0.5

t (inns) →

(t) (inmV) →

λ = 1326 nm

λ = 1576 nm

Figure 7: Measured input impulses at different operating

wavelengths λ.

to be Gaussian

(t) = Ae

−π(t/T

)

, (13)

where T

= 0.8 ns and T

= 200. The scaling fac-

tor A is chosen to maintain E

= 1V

of the sig-

OPTICS2014-InternationalConferenceonOpticalCommunicationSystems

nal u

(t) and to ensure the unit s

−1

of the impulse

response. Fig. 8 shows the quality of the obtained

impulse responses using the two ﬁlter functions men-

tioned before. The regularisation ﬁlter is applied for

both optimisation criteria resulting in γ values de-

picted in Fig. 9. The γ values are also decreasing with

30 35 40 45 50

10 · lg(E

) (indB) →

MSE

→

(f) with MSE Criterion

(f) with Gans Criterion

Wiener ﬁl ter

Figure 8: Quality F

MSE

of the deconvolved impulse re-

sponses as a function of signal energy to noise power spec-

tral density using different ﬁlter functions.

30 35 40 45 50

−18

−17

10 · lg(E

) (indB) →

γ →

opt

(MSE Criterion)

opt

(Gans Criterion)

Figure 9: Choice of optimal γ when ﬁltering with H

( f)

minimizing the MSE and using the Gans criterion.

increasing E

for both criteria. It should be noted,

that the γ values using the Gans’ criterion are lower

compared to the MSE criterion. This signiﬁes that the

measured signal u

(t) is ﬁltered less when applying

the ﬁlter using the Gans’ criterion in contrast to us-

ing the MSE criterion. As expected, the deconvolved

impulse responses using the Wiener ﬁlter are showing

the best quality of all applied ﬁlter functions closely

followed by the estimated impulse responses ﬁltered

with the regularisation function using the MSE opti-

misation criterion. The quality of the estimated im-

pulse responses using the Gans’ criterion is still ac-

ceptable for a wide range of E

values and pro-

vides a major improvement compared to the quality

without ﬁltering. The obtained results show further

that the parametric regularisation ﬁlter function is a

good compromise compared to the Wiener ﬁlter with

its high complexity.

Applying the described deconvolution processing

to the (2 × 2) MIMO testbed, the obtained impulse

responses are depicted in Fig. 10-11. They are calcu-

lated by applying the regularisation ﬁlter in the decon-

volution process with γ values respecting the Gans’

and Error criterion. At an operating wavelength of

1 2 3 4

0.5

1 2 3 4

t (inns) →t (inns) →

1 1

(t) →

1 2

(t) →

2 1

(t) →

2 2

(t) →

γ ≈ 1.20E − 10

γ ≈ 8.61E − 10

γ ≈ 1.43E − 10 γ ≈ 6.76E − 10

Figure 10: Deconvolved measured electrical MIMO im-

pulse responses with respect to the pulse frequency f

1/T

= 620 MHz at 1326 nm operating wavelength using

the regularisation ﬁlter function with γ values according to

the Gans’ criterion.

2 4

0.0

0.5

1.0

2 4

0.1

0.2

0.3

2 4

0.1

0.2

2 4

0.05

0.1

0.15

t (inns) →t (inns) →

1 1

(t) →

1 2

(t) →

2 1

(t) →

2 2

(t) →

γ = 0.0146

γ = 0.0168

γ = 0.0174

γ = 0.0229

Figure 11: Deconvolved measured electrical MIMO im-

pulse responses with respect to the pulse frequency f

1/T

= 620 MHz at 1576 nm operating wavelength using

the regularisation ﬁlter function with γ values according to

the Gans’ criterion.

1326 nm the modal structure can be identiﬁed. Con-

sidering the 1576 nm results the additional inﬂuence

of the chromatic dispersion is clearly visible.

EqualisationofMeasuredOpticalMIMOChannels

4 MIMO EQUALISATION AND

SIMULATION RESULTS

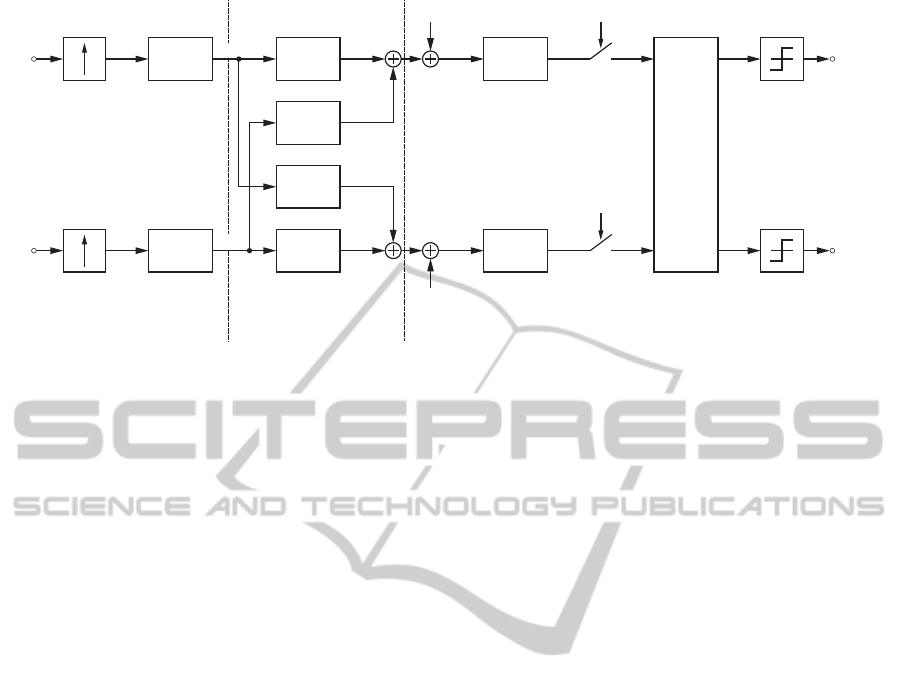
In this section the MIMO baseband transmission sys-

tem is constructed as illustrated in Fig. 12. It uses

the deconvolved (2 × 2) MIMO speciﬁc impulse re-

sponses g

i, j

(t) (for i = 1, 2 and for j = 1,2) depicted

in Fig. 11 at 1576 nm operating wavelength. In

this baseband system model the transmitter forms a

rectangular pulse train and hence the transmit ﬁlter

(t) and the receive ﬁlter g

(t) are considered to be

matched ﬁlters and are described in its non causal no-

tation with

(t) = g

(t) =

rect





. (14)

The total transmit power is normalised to P

and a symbol pulse frequency of f

= 1/T

620 MHz per data channel is used resulting in a total

bit rate of 1.24 Gb/s for both channels. Both transmit

signals u

s, j

(t) are launched onto the (2 × 2) MIMO

channel. The ﬁltered receive signals u

e,i

(t) are sam-

pled with kT

, where k ∈ Z. The system can be sim-

pliﬁed by introducing the cumulativechannel impulse

response h

i, j

(t) and the ﬁltered noise w

(t) expressed

as follows

i, j

(t) = g

(t) ∗ g

i, j

(t) ∗ g

(t), h

i, j

(k) = h

i, j

(t)



(t) = n

(t) ∗ g

(t), w

(k) = w

(t)



(15)

By utilising a data block transmission model (Raleigh

and Ciofﬁ, 1998; Raleigh and Jones, 1999) a vectorial

notation can be applied as follows

c =



c[1] c[2] ··· c[K]



i, j



i, j

[1] h

i, j

[2] ·· · h

i, j

[L]



(16)

Using the convolution matrices H

i, j

the transmission

model can be described as

= H

· c

+ H

· c

+ w

= H

· c

+ H

· c

+ w

(17)

Written in matrix notation

















. (18)

Simplifying this equation results in

u = H· c+ w , (19)

where the channel matrix H contains the ISI as well

as the crosstalk information. For obtaining the trans-

mitted symbols unaffected from the channel

F· H = I (20)

has to be fulﬁlled, where I is a identity matrix and

thus the equaliser matrix F can be obtained as follows

F = (H

−1

. (21)

Hereinafter, the equaliser matrix F is applied to the

received data vector u

y = F· u

y = c+ F· w .

(22)

The beneﬁt of applying this zero forcing (ZF)

equaliser is the orthogonalisation of the transmission

channels. Thus, the resulting equalised MIMO sys-

tem can be described by two independent single input

single output (SISO) channels. The disadvantage of

using the ZF equaliser is the weighting of the noise

term.

Eye diagrams of both received signals in the

MIMO system after equalisation are shown in Fig. 13.

Using the ZF equaliser both eyes are fully opened

−0.5 0 0.5

−4

−2

t/T

→

(t) (inV) →

−0.5 0 0.5

−4

−2

t/T

→

(t) (inV) →

Figure 13: Eye diagram patterns of both received signals

when applying zero forcing equalisation.

conﬁrming its functionality. The MIMO bit-error rate

(BER) simulation results are depicted in Fig. 14 and

underline the functionality of the equaliser.

5 10 15 20 25

−3

−2

−1

BER

→

10 · lg(E

) (indB) →

without equaliser

ZF equaliser

Figure 14: (2× 2) MIMO BER probability results with and

without applying the zero forcing equalising method using

the deconvolved MIMO impulse responses at 1576 nm op-

erating wavelength and transmitting with a bit rate of 1.24

Gb/s.

OPTICS2014-InternationalConferenceonOpticalCommunicationSystems

(k)

(t)

(k)

(t)

transmitter

MIMO − channel

receiver

Figure 12: (2× 2) MIMO baseband transmission system model with discrete zero forcing equaliser.

5 CONCLUSIONS

In this contribution a (2 × 2) optical MIMO commu-

nication system, consisting of a 1.4 km multi-mode

ﬁbre and optical couplers attached to both ends, has

been analysed. The estimations of the MIMO spe-

ciﬁc impulse responses have been obtained for op-

erating wavelengths of 1576 nm and 1326 nm using

optimized signal deconvolution by applying the para-

metric regularisation ﬁlter. It has been shown that

the quality of the estimated impulse responses signiﬁ-

cantly improvesand is comparable to Wiener ﬁltering.

These estimated impulse responses have been used

for modelling a baseband MIMO data transmission

system. In order to receive the transmitted data unaf-

fected from the data send on the neighbouring channel

zero forcing equalisation has been investigated. The

successful implementation has been shown by the bit-

error curves as well as by the open eye-diagram.

ACKNOWLEDGEMENTS

This work has been funded by the German Ministry

of Education and Research (No. 03FH016PX3).

REFERENCES

Ahrens, A. and Lochmann, S. (2013). Optical Couplers in

Multimode MIMO Transmission Systems: Measure-

ment Results and Performance Analysis. In Interna-

tional Conference on Optical Communication Systems

(OPTICS), pages 398–403, Reykjavik (Iceland).

Ahrens, A., Schr¨oder, A., and Lochmann, S. (2013). Disper-

sion Analysis within a Measured 1,4 km MIMO Mul-

timode Channel. In International Conference on Op-

tical Communication Systems (OPTICS), pages 391–

397, Reykjavik (Island).

Gans, W. L. (1986). Calibration and Error Analysis of a

Picosecond Pulse Waveform Measurement System at

NBS. Proceedings of the IEEE, 74(1):86–90.

K¨ohnke, H., Schwinkendorf, R., Daase, S., Ahrens, A., and

Lochmann, S. (2014). Receiver Design for an Optical

MIMO Testbed. In International Conference on Opti-

cal Communication Systems (OPTICS), Vienna (Aus-

tria).

K¨uhn, V. (2006). Wireless Communications over MIMO

Channels – Applications to CDMA and Multiple An-

tenna Systems. Wiley, Chichester.

Nahman, N. S. and Guillaume, M. E. (1981). Deconvolution

of Time Domain Waveforms in the Presence of Noise.

National Bureau of Standards Technical Note 1047,

Boulder, Colorado 80303.

Raleigh, G. G. and Ciofﬁ, J. M. (1998). Spatio-Temporal

Coding for Wireless Communication. IEEE Transac-

tions on Communications, 46(3):357–366.

Raleigh, G. G. and Jones, V. K. (1999). Multivariate

Modulation and Coding for Wireless Communication.

IEEE Journal on Selected Areas in Communications,

17(5):851–866.

Richardson, D. J., Fini, J., and Nelson, L. (2013). Space

Division Multiplexing in Optical Fibres. Nature Pho-

tonics, 7:354–362.

Sandmann, A., Ahrens, A., and Lochmann, S. (2013).

Signal Deconvolution of Measured Optical MIMO-

Channels. In XV International PhD Workshop OWD

2013, pages 278–283, Wisa, Poland.

Sandmann, A., Ahrens, A., and Lochmann, S. (2014). Ex-

perimental Description of Multimode MIMO Chan-

nels utilizing Optical Couplers. In 15. ITG-

Fachtagung Photonische Netze, Leipzig (Germany).

Singer, A. C., Shanbhag, N. R., and Bae, H.-M. (2008).

Electronic Dispersion Compensation– An Overwiew

of Optical Communications Systems. IEEE Signal

Processing Magazine, 25(6):110–130.

EqualisationofMeasuredOpticalMIMOChannels

Tse, D. and Viswanath, P. (2005). Fundamentals of Wireless

Communication. Cambridge, New York.

Vaseghi, S. (2000). Advanced Digital Signal Processing

and Noise Reduction, Second Edition. John Wiley &

Sons Ltd, Chichester.

Winzer, P. (2012). Optical Networking beyond WDM.

IEEE Photonics Journal, 4:647–651.

OPTICS2014-InternationalConferenceonOpticalCommunicationSystems