
Research on Movement Rules of Soil Particles during EPB Machine
Tunneling with Spoke Cutterhead
Wu Li
1
, Chen Long
1
and Ju Ernan
2
1
Institute of Mechanical Engineering, Dalian Jiaotong University, Huanghe Road 794#, Shahekou Distict, Dalian, China
2
Department of Electronic Engineering, Dalian Neusoft Information Institute, Soft park Road, Dalian, China
Keywords: Spoke Cutterhead, Discrete Element, Soil Particles, Movement Rules, Epb Tunnelling.
Abstract: To discover the movement rules of soil particles around cutterhead during shield machine tunnelling, the
discrete element model of shield machine tunnelling is established. The model is able to simulate the
structure of shield machine, the pressure of ground, the excavation operation process and the flow process
of soils. The cutterhead torque is obtained by running the model, which is accord with the theoretical
calculation results. The displacements of soil particles in the cutterhead system of shield machine at the
different tunnelling time are displayed. The movement of soil particle is periodic, and the period is related to
the cutterhead rotation speed.
1 INTRODUCTION
The tunnel boring machine (TBM), including the
hard rock TBM and the soft ground TBM, is the
advanced large special equipment for tunnel
excavation. A lot of scholars have conducted
extensive research on the hard rock TBM
construction and design (Rostami and
Ozdemir,1993; Burger,2006; Karlheinz,2009;
Abdolreza and Siamak,2012; Peter,2009). For the
soft ground TBM, such as earth pressure balance
(EPB) machine, there are many research on the
relation between the tunneling operation parameters
(Hehua, 2007; Hongxin and Deming, 2006).
Although the EPB machine is already used to
excavate tunnels, and there are some research on the
construction technology, the dynamic interaction
rules between the shield machine and the soils,
which has an important role in improving and
optimizing the system structure, is not clear.
The paper simulates the tunneling process of the
EPB machine with spoke cutterhead by the discrete
element software PFC3D. The displacement
distributions of soil particles in the cutterhead and in
the chamber at different tunnelling time are
obtained. The movement rules of soil particles
during the machine tunnelling are revealed.
2 SIMULATION
The Discrete Element Method (DEM) model of EPB
shield machine tunnelling, which is shown in
Figure1, consists of two parts. One is the simulated
ground; the other is the tunnelling shield machine.
The model of the shield machine is made up with the
cutterhead, the chamber and the screw conveyor.
The cutterhead is composed of six spokes. The 84
cutters are located on both edges of the spokes. The
opening ratio of the cutterhead is 70%. The structure
parameters of the model are listed in Table.1.
Figure 1: DEM model of shield machine tunnelling.
The DEM model of the shield machine is set up by
wall elements in PFC3D. The cutterhead and the
chamber board are made up with plane walls. The
vertex coordination of the plane walls are obtained
243
Li W., Long C. and Ernan J..
Research on Movement Rules of Soil Particles during EPB Machine Tunneling with Spoke Cutterhead.
DOI: 10.5220/0005103002430248
In Proceedings of the 4th International Conference on Simulation and Modeling Methodologies, Technologies and Applications (SIMULTECH-2014),
pages 243-248
ISBN: 978-989-758-038-3
Copyright
c
2014 SCITEPRESS (Science and Technology Publications, Lda.)

by CAD model of cutterhead. The shield shell and
the outer barrel of screw conveyor are built by
cylinder wall, and the screw is built by spiral wall.
A homogeneous packing of 20,715 particles in a
cylinder container of 6.28 m in diameter and 2 m in
length has been chosen to simulate the soil where the
excavation takes place. The compressive strength of
the soils is 50kPa. The contact models between
particles is selected the standard linear model in
PFC3D. The contact model parameters between
particles are shown in Table 2. The simulated
excavation depth of the ground is 20 m. The ground
pressure is controlled by the servo-mechanism,
which is realized by adjusting the displacement of
the wall constantly to keep the pressure stable (Li,
2013). The rotation speed of the cutterhead is set 2
rpm, the thrust speed is set 60 mm/min, and the
rotation speed of screw conveyor is set 5 rpm. In
PFC3D, the wall has 6 velocity components, so the
machine speed can be realized easily by setting the
velocity components of walls.
Table 1: Structure parameters of DEM model.
Shield
diameter
(m)
Shield
length
(m)
Conveyor
diameter
(m)
Conveyor
length
(m)
Screw
pitch
(m)
Conveyor
angle
(º)
6.28 2 0.84 5 0.56 22
Table 2: Particle parameters.
Normal
stiffness
(N/m)
Shear
stiffness
(N/m)
Friction
coefficient
Particle
density
(kg/m
3
)
Particle
radius
(m)
540000 540000 0.5 2637 0.0625
1) 5s 2) 20s
3) 60s 4) 120s
Figure 2: Shield machine tunnelling process.
The DEM model can reproduce the dynamic
tunnelling process of the shield machine, which is
shown in figure 2.
The DEM model of the EPB shield machine
tunnelling is run for 120 s. When the run time is 120
s, the chamber and screw conveyor are filled with
particles. This means that the cutterhead system
comes into the stable state.
3 MODEL VERIFICATION
3.1 Simulated Torque
The cutterhead torque is measured in the process of
the tunnelling simulation. The simulation torque is
compared to the theoretical calculation to verify the
DEM model. The cutterhead torque is extracted
every 5 s from 0 s to 120 s (Figure 3). The average
simulated cutterhead torque is 1435kNm.
1000
1200
1400
1600
1800
0 102030405060708090100110120
Cutterhead torque / kNm
Time/ s
Simulation torque
Theory torque
Figure 3: Simulated cutterhead torque.
3.2 Calculation Torque
In the DEM model, the simulated cutterhead torque
is composed of cutting torque, the friction torque of
the front side and the back side of the cutterhead.
The theoretical calculation equation of the above
mentioned torque is concluded (Kui, 2011).
The cutterhead cutting torque T
1
is shown in
Equation (1).
22
1maxu
TnqhDn
(1)
Where q
u
is the compressive strength of soils, kPa;
h
max
is the penetration of cutterhead, m, h
max
=v/n, v
is the thrust speed, m/h, n is the cutterhead rotation
speed, rpm; D is the diameter of the cutterhead, m.
The friction torque of the front side of cutterhead
is illustrated in Equation (2).
22
1maxu
TnqhDn
(2)
SIMULTECH2014-4thInternationalConferenceonSimulationandModelingMethodologies,Technologiesand
Applications
244

Where
is the non opening ratio;
1
is the friction
coefficient between soils and the cutterhead; R
c
is
the radius of the cutterhead, m; R
d
is the active
pressure of soils, kPa.
The friction torque of the back side of the
cutterhead is regard as the same as that of the front
side.
Through calculation, the cutting torque is 473
kNm, the friction torque for each side of cutterhead
is 512 kNm. So the total torque is 1497 kNm. The
average error between the simulated torque and the
calculation torque is 4%, which illustrates the DEM
model is correct.
4 PARTICLE MOVEMENT
RULES
During the DEM simulation, the displacement
distributions of soil particles in the chamber and in
the cutterhead are displayed at tunnelling time 0s,
10s, 15s, 20s, 30s, 45s and 60s.
As shown in Figure 4, at time 0 s, the cutterhead,
the shield and the screw conveyor haven’t started to
work, the particles in the cutterhead and in the
chamber both move downward to the chamber board
under the gravity and the ground pressure. The soil
displacement in the cutterhead is higher than that in
the chamber.
At time 10 s (Figure 5), the particles in the
cutterhead rotate along the axis of y anticlockwise. It
is because that the friction torque caused by the
cutterhead conquers the interaction force between
particles, and the cutterhead leads the particle to
move. The bottom particles in the chamber march
toward the screw entrance for the screw rotation.
The above particles in the chamber rotate
anticlockwise.
At time 15 s (Figure 6), in the cutterhead, the
particles displacement in XZ plane is radiate. This is
because that in the circumferential direction, the
distance between particles increases with the
cutterhead rotation further, leading to the interaction
force between particles increases; When the
interaction force is larger than the friction caused by
the cutterhead rotation, the particles slow down the
movement anticlockwise until the velocity of the
particles in circumferential direction reach 0. At this
time, the displacement of particles is subjected to the
radial ground pressure, so the displacement of
particles is radial in XZ plane. The displacement of
particles in the chamber is similar to that at time 10.
At time 20 s (Figure 7), the particles rotate
clockwise in the cutterhead. This is because that the
interaction force between particles is much larger
than the force caused by the cutterhead rotation. At
time 15 s, the force between particles reaches
maximum. By the particles rotate clockwise, the
distance between particles reduces, and the
interaction force between particles reduces too.
When the interaction force reaches 0 N, the particles
begin rotate anticlockwise again. In the chamber, the
bottom particles move toward the inlet of the
conveyor for the screw rotation, and the movement
direction of the above particles is caused by the
ground pressure mainly.
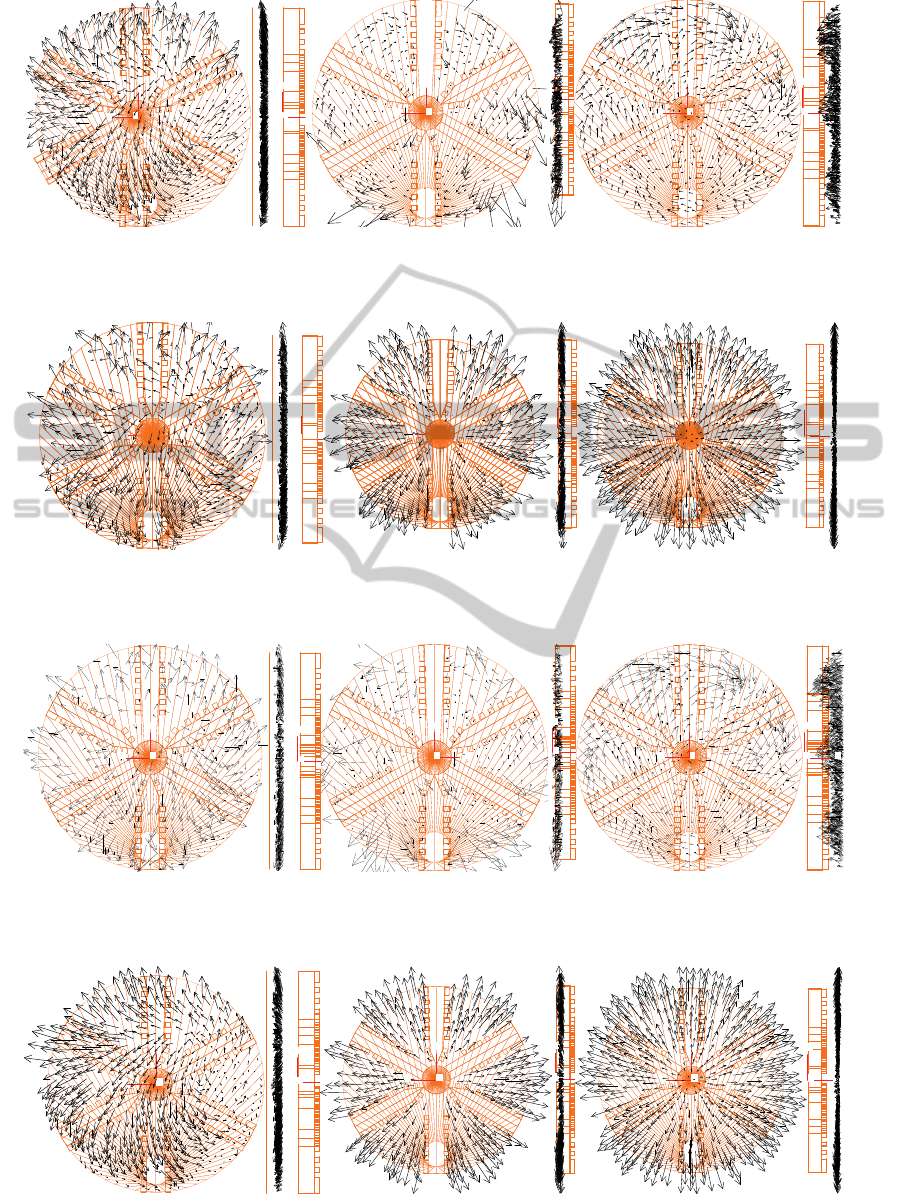
At time 30 s and 60 s (Figure 8 and Figure 10),
the displacement distribution of particles is similar
to that at time 0 s. As shown in Figure 9, the
displacement is similar to Figure 6. It implies that
the motion period of the soil particles is 30 s.
In order to study the relation between the rotation
speed of the cutterhead and the soil particles
movement rules, the DEM model is run again setting
the cutterhead rotation speed 1 rpm. The
displacement distribution of soil particle at run time
30 s is shown in Figure 11. It is shown that the
displacement at time 30 s when cutterhead rotation
speed is 1 rpm is similar to the displacement at time
15s when cutterhead rotation speed is 2 rpm. So the
particle movement period T can be deduced as
equation (3)
60
T
n
(3)
5 CONCLUSIONS
The DEM model of the EPB shield machine
tunnelling was established, and the simulated
cutterhead torque was consistent with the theoretical
calculation results, which illustrated the model was
correct.
The displacement distribution of soils in the
chamber and in the cutterhead during the shield
machine tunnelling was obtained. The distribution
presented periodic variation, and the movement
period of soil particles is related to the cutterhead
rotation speed.
Seen from the figures of displacement
distribution, the soil displacement in the cutterhead
is determined by the cutterhead rotation, the
ResearchonMovementRulesofSoilParticlesduringEPBMachineTunnelingwithSpokeCutterhead
245

X Y
Z
X Y
Z
X Y
Z
X Y
Z
XY
Z
X Y
Z
1) in chamber 2) in cutterhead 3) in front of cutterhead
Figure 4: Displacement distribution of soil particles at 0 s.
X Y
Z
X
Z
X Y
Z
X
Z
X Y
Z
X
Y
Z
1) in chamber 2) in cutterhead 3) in front of cutterhead
Figure 5: Displacement distribution of soil particles at 10 s.
X Y
Z
X
Z
X Y
Z
X
Z
X Y
Z
X
Y
Z
1) in chamber 2) in cutterhead 3) in front of cutterhead
Figure 6: Displacement distribution of soil particles at 15 s.
X Y
Z
X
Z
X Y
Z
X
Z
X Y
Z
X
Y
Z
1) in chamber 2) in cutterhead 3) in front of cutterhead
Figure 7: Displacement distribution of soil particles at 20 s.
SIMULTECH2014-4thInternationalConferenceonSimulationandModelingMethodologies,Technologiesand
Applications
246
X Y
Z
X
Z
X Y
Z
X
Z
X Y
Z
X Y
Z
1) in chamber 2) in cutterhead 3) in front of cutterhead
Figure 8: Displacement distribution of soil particles at 30 s.
X Y
Z
X
Z
X Y
Z
X
Z
X Y
Z
X
Y
Z
1) in chamber 2) in cutterhead 3) in front of cutterhead
Figure 9: Displacement distribution of soil particles at 45 s.
X Y
Z
X
Z
XY
Z
X
Z
X Y
Z
X Y
Z
1) in chamber 2) in cutterhead 3) in front of cutterhead
Figure 10: Displacement distribution of soil particles at 60 s.
X Y
Z
X
Z
X Y
Z
X
Z
X Y
Z
X
Y
Z
1) in chamber 2) in cutterhead 3) in front of cutterhead
Figure 11: Displacement distribution of soil particles at 30 s with cutterhead rotation speed 1rpm.
ResearchonMovementRulesofSoilParticlesduringEPBMachineTunnelingwithSpokeCutterhead
247

interaction forces between particles and the ground
pressure; in the chamber, the displacement of above
soils were subjected to the ground pressure mainly,
and the displacement of the bottom particles were
caused by the rotation of the screw conveyor.
ACKNOWLEDGEMENTS
The authors are grateful to the support of the
National Natural Science Foundation of China
(Study on Dynamic Interaction between Cutterhead
of Shield Tunneling Machine and Soils and Design
Theory of Cutterhead System, Award No.
51105048).
REFERENCES
Rostami,J.,Ozdemir,L.,1993. New model for performance
prediction of hard rock TBMs. In: Proceedings, Rapid
Excavation and Tunneling Conference, 793–809.
Burger,W., 2006. Hard rock cutterhead design. In:
Proceedings of the North American Tunneling 2006
Conference,pp.257–263.
Karlheinz,G., 2009. The influence of TBM design and
machine features on performance and tool wear in
rock. Geomech.Tunnelbau, 2,140–155.
Abdolreza,Y.C., Siamak,H.Y., 2012. Tunnel Boring
Machine (TBM) selection using fuzzy multicriteria
decision making methods. Tunn.Undergr.Space
Technol.30,194–204.
Peter,J.T.,2009.Simple and practical TBM performance
prediction. Geomech. Tunnelbau., 2,128–139.
Hehua Z., Qianwei X., Qizhen Z., Shaoming L.,2007,
Experimental study on the working parameters of EPB
shield tunneling in soft ground. China Civil
Engineering Journal, 40(9):87-94.
Hongxin,W., Deming, Fu., 2006. Mathematical model and
the related parameters for EPB shield tunneling. Tumu
Gongcheng Xuebao, 39, 86–90.
Li W., Tianmin G., Lei L., 2013. Discrete element model
for performance analysis of cutterhead excavation
system of EPB machine. Tunnelling and Underground
Space Technology, 37, 37–44.
Kui C., Kairong H., Xuesong W., 2011. Shield
Construction Technique. China Communications
Press, Beijing.
SIMULTECH2014-4thInternationalConferenceonSimulationandModelingMethodologies,Technologiesand
Applications
248
