
Antennas’ Correlation Influence on the GMD-assisted MIMO Channels
Performance
C´esar Benavente-Peces
1
, Andreas Ahrens
2
, Francisco Javier Ortega-Gonz´alez
1
and Jos´e Manuel Pardo-Mart´ın
1
1
Universidad Polit´ecnica de Madrid, ETS de Ingenier´ıa y Sistemas de Telecomunicaci´on,
Department of Signal Theory and Communications, Ctra. Valencia km. 7, 28031 Madrid, Spain
2
Hochschule Wismar, University of Technology, Business and Design, Faculty of Engineering, Department of Electrical
Engineering and Computer Science, Philipp-M¨uller-Straße, PO box 1210, 23952 Wismar, Germany
Keywords:
Multipe-Input Multile-Output (MIMO), Geometric Mean Decompositioin, Trasnmission Mode, Bit Alloca-
tion, Power Allocation.
Abstract:
The use of multiple antennas in MIMO (multiple-input multiple-output) systems at both the transmit and re-
ceive sides produces the effect known as antennas correlation which impact the overall channel performance,
throughput and bit-error rate (BER). The geometric mean decomposition (GMD) is a signal processing tech-
nique which can be used to process transmit and receive signals in MIMO channels. The GMD pre- and
post-procesing in conjunction with dirty-paper precoding shows some advantages over the popular singular
value decomposition (SVD) technique which provides GMD-assisted MIMO systems a superior performance
particularly when the channel is affected by antennas correlation. This paper analyses the impact of antennas
correlation on the performance of GMD-assisted wireless MIMO channels highlighting the advantages over
SVD-assisted ones.
1 INTRODUCTION
In the last decades researchers and engineers are fac-
ing the uphill to obtain higher transmission data rates
and wider bandwidths required for the current and
future high-speed services demanded by the indus-
try and society, as video streaming, video-conference,
massive data transference, multi-user services, etc. In
this context multiple-input multiple-output (MIMO)
systems are playing a key role due to their capability
to increase the channel throughput and performance
compared with single-input single-output (SISO) sys-
tems (Foschini and Gans, 1998), (Ozgur et al., 2013).
Due to their potential capabilities MIMO wireless
communication systems have attracted a lot of atten-
tion from the research community. The use of spatial
diversity in MIMO systems can considerably increase
data rate and significantly improve the system robust-
ness, reliability and coverage (Yang et al., 2011).
The use of multiple transmit and receive antennas
causes effects which affect the channel performance.
First, due to the multi-antenna configuration and the
multi-path transmission inter-antennas interferences
disturb the channel behaviour. MIMO systems benefit
from multipath by using additional signal processing
in order to improve the channel performance. Sec-
ond, due to physical limitations the antennas at each
side are really close compared to the wavelength and
the correlation effectappears negativelyimpacting the
MIMO channel performance (Janaswamy, 2002).
As stated above, in order to benefit the MIMO
channels capabilities additional signal processing
is required. The SVD is a popular technique
widely used to improve MIMO channels performance
(Haykin, 2002). Given perfect channel state infor-
mation (PCSI) is available at both the transmit and
receive link sides, the SVD is used to perform pre-
and post-processing on the transmit and receive sig-
nals (respectively) to completely eliminate the ex-
isting inter-antennas interferences. As a result the
MIMO channel is transformed into several parallel,
independent and non-interfering single-input single-
output (SISO) unequally weighted channels.
GMD-assisted signal processing seems to be an
advantageous alternative to SVD-assisted signal pro-
cessing in MIMO systems. The GMD can be used
to process transmit and receive signals decomposing
the MIMO channel into several SISO channels with
325
Benavente-Peces C., Ahrens A., Pardo-Martín J. and Ortega-González F..
Antennas’ Correlation Influence on the GMD-assisted MIMO Channels Performance.
DOI: 10.5220/0005363603250334
In Proceedings of the 5th International Conference on Pervasive and Embedded Computing and Communication Systems (AMC-2015), pages 325-334
ISBN: 978-989-758-084-0
Copyright
c
2015 SCITEPRESS (Science and Technology Publications, Lda.)

remaining inter-antennas interferences which must be
eliminated by using additional signal processing (e.g.,
Tomlinson-Harashima pre-coding) to obtain the best
channel performance. Along the investigation the
Tomlinson-Harashima pre-coding is used in a fre-
quency non-selectiveGMD-assisted MIMO system to
perfectly cancel the inter-antenna interferences (Kinjo
and Ohno, 2013).
In order to improve the SVD-assisted MIMO sys-
tem performance, where the resulting SISO channels
have different particular layer gains, bit and power al-
location techniques based on the varying channel con-
dition can be used (Zhou et al., 2005), which is syn-
onymous of adaptive modulation. One of the main
advantages of using the GMD is that the resulting in-
dependent layers have the same particular SISO chan-
nel gain coefficient (the geometric mean of the sin-
gular values), assuming that the inter-antenna inter-
ferences are perfectly eliminated by dirty-paper pre-
coding. Hence, power allocation doesn’t make sense
in GMD-assisted MIMO systems (a priori) avoiding
the required computational overhead.
Antennas correlation is characterized by the an-
tennas’ correlation coefficients which affect the chan-
nel matrix and hence its behaviour (Lee, 1973). The
higher the antennas’ correlation the lower the chan-
nel scatter richness condition (required by MIMO sys-
tems to get a better behaviour) and the lower the over-
all performance. The correlation effect affects the ge-
ometric mean PDF which impacts the channel perfor-
mance. The geometric mean PDF and the CCDF can
be used to predict and optimize the MIMO channel
performance by activating a proper number of layers
which define different transmission modes configura-
tions.
In (Benavente-Peces et al., 2013) the authors fo-
cused the investigation on the analysis of the singular
values CCDF to evaluate the receiver-side antennas
correlation effect on the channel performance and the
outcomes of the appropriate antennas usage in a SVD-
assisted MIMO system.
The noveltyof this contribution is that a frequency
non-selective MIMO link is studied independently of
the antennas electrical properties to analyse the im-
pact of antennas’ correlation on the performance of
GMD-assisted MIMO systems. The effects on the
channel matrix are highlighted and the resulting ge-
ometric mean PDF and CCDF are studied.
Additionally the benefits of having equal values of
layer-specific weighting factors (i.e. gain coefficients)
in GMD-based MIMO systems are remarked against
the SVD-assisted ones using different number of ac-
tive layers, highlighting the effect of correlation com-
pared to classical uncorrelated channels. The geomet-
ric mean CCDF curves are used to analyse and pre-
dict the behaviour of the MIMO channel. The BER
is computed for various active layers and the effect
of antennas’ correlation is remarked to find the best
transmission mode. A 4×4 MIMO system transmit-
ting QAM signals along the active layers is consid-
ered as an example.
The remaining part of this paper is structured as
follows. Section 1 shows the computation of the
geometric mean of the channel matrix singular val-
ues. Section 3 describes the channel model for the
GMD-assisted MIMO system, including the anten-
nas’ correlation model. The analysed transmission
modes are introduced in Section 4. Section 5 com-
pares the GMD-assisted MIMO system versus the
SVD-assisted one. In Section 6 the main results of the
investigation are introduced including the geometric
mean PDF and CCDF analysis, the antennas’ corre-
lation effects and the considered transmission modes.
Finally, Section 7 summarizes and highlightsthe main
outcomes.
2 THE GEOMETRIC MEAN
The GMD with remaining interference elimination
decomposes the MIMO channel into several indepen-
dent SISO channels with equal performance. The
main advantage that GMD-assisted MIMO systems
present over the SVD-assisted ones is that those inde-
pendent layers have the same gain coefficient which is
the geometric mean of the singular values of the chan-
nel matrix. Hence, the additional computational load
required to perform bit and power allocation to im-
prove and optimize the MIMO channel performance
is reduced. The geometric mean can be computed
from the channel matrix singular values as:
µ =
L
∏
i=1
p
ξ
i
!
1/L
, (1)
where L is the number of activated layers (with L ≤
min(n
T
,n
R
), n
T
and n
R
are the number of transmit and
receive antennas respectively) and
p
ξ
i
(singular val-
ues) states for the positive square roots of the eigen-
values ξ
i
of H· H
H
, where H is the channel matrix
and (·)
H
is the hermitian operator.
3 CHANNEL MODEL
The MIMO channel can be described in general terms
as
y = H· c+ n (2)
PECCS2015-5thInternationalConferenceonPervasiveandEmbeddedComputingandCommunicationSystems
326

where c is the (n
T
× 1) transmit data vector, H is the
(n
R
×n
T
) channel matrix, n is the (n
R
×1) noise vector
at the receive antennas and y is the (n
R
× 1) receive
data vector (with n
T
the number of transmit antennas
and n
R
the number of receive antennas). By using the
GMD the channel matrix can be decomposed as:
H = Q · R· P
H
(3)
where R is an upper triangular matrix and Q and P
are unitary matrices whose rows are orthonormal. As-
suming the PCSI condition at both the transmit and
receive sides, pre (P) and post (Q
H
) processing can
be performed at the transmit and receive sides result-
ing in
˜
y = R· c+
˜
n, (4)
where R is an upper triangular matrix whose elements
in the main diagonal equal the geometric mean of the
singular values and the upper non-zero elements de-
scribe the remaining inter-antenna interferences,
˜
n is
the post-processed noise vector and
˜
y is the resulting
receive data vector. By using perfect interference can-
cellation (e.g. Tomlinson-Harshima pre-coding) the
remaining interference can be removed and the chan-
nel can be finally described as
˜
y =
˜
R· c+
˜
n (5)
where
˜
R is a diagonal matrix whose non-zero ele-
ments equal the geometric mean of the singular val-
ues. In order to improve the channel performance it
is possible to select the appropriate number of active
layers obtaining an extra gain in the geometric mean
computation as only the largest singular values are
considered (Jiang et al., 2005).
3.1 Singular Values vs. Geometric Mean
The SVD decomposes the channel matrix as H = S·
V· D
H
, where V is a diagonal matrix containing the
singular values of H in descending order, and S and D
are unitary matrices. After pre- and post-processing
the transmit and receive data vectors with matrices D
and S
H
respectively, the resulting receive data vector
is givenby
˜
y = V·c+
˜
n, where
˜
n is the post-processed
noise vector, described a system composed of several
independent layers (SISO channels).
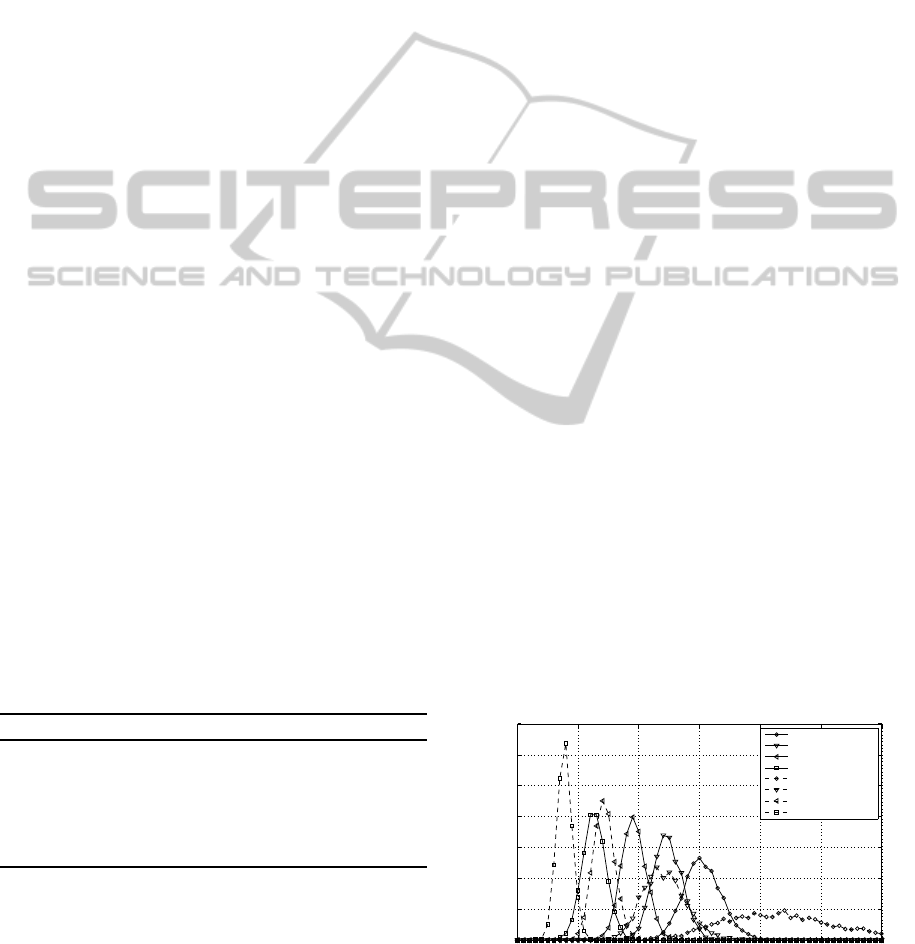
Figure 1 represents and compares the matrices V
(containing the singular values), R (containing the ge-
ometric mean and remaining inter-antenna interfer-
ences) and
˜
R (containing the geometric mean) for an
exemplary (4 × 4) MIMO channel. Independently of
the number of active layers the value of the singular
values doesn’t change. On the other hand the value of
the geometric mean depends on the number of active
layers as shown in Fig. 1(b)-(f). For one active layer
((a) and (f)) the systems behave in the same way as the
layer coefficient is the same in both cases. For four
active layers the SVD-assisted MIMO system shows
a weak layer which drops the overall system perfor-
mance and the GMD-assisted one shows a higher per-
formance. The cases concerning two and three active
layers requires a more detailed analysis as different
transmission modes can be considered and the final
results depend on the real channel status.
3.2 Antennas’ Correlation
Antennas correlation is characterized by the correla-
tion matrix which is composed of the correlation coef-
ficients describing the dependencies of the multipath
transmission. The correlation between antennas k and
ℓ is denoted as ρ
kℓ
. Given a set of n
N
antennas, the
correlation matrix is a (n
N
× n
N
) one. As an example,
the receiver side correlation matrix for a four receive
antennas is given by
R
(4×4)
RX
=
1 ρ
(RX)
12
ρ
(RX)
13
ρ
(RX)
14
ρ
(RX)
21
1 ρ
(RX)
23
ρ
(RX)
24
ρ
(RX)
31
ρ
(RX)
32
1 ρ
(RX)
34
ρ
(RX)
41
ρ
(RX)
42
ρ
(RX)
43
1
. (6)
Therein, the correlation coefficient ρ
(RX)
k,ℓ
de-
Figure 1: Singular values vs. geometric mean: (a) Matrix V,
(b) Matrix R, (c) Matrix
˜
R (4 active layers), (d) Matrix
˜
R,
(3 active layers), (e) Matrix
˜
R (2 active layers), (f) Matrix
˜
R (1 active layer).
Antennas'CorrelationInfluenceontheGMD-assistedMIMOChannels
Performance
327
scribes the receiver side correlation between the trans-
mit antenna k and ℓ. It can be demonstrated that
ρ
(RX)
ℓ,k
= ρ
∗(RX)
k,ℓ
and the matrix in (6) can be simplified.
The transmit correlation matrix R
TX
can be described
in a similar way. In the case of uncorrelated antennas,
the off-diagonal elements are zero.
According to (Ahrens et al., 2013) the (n
T
× n
R
)
channel matrix H
c
which models a MIMO system af-
fected by antennas’ correlation can be obtained from
the channel matrix of an uncorrelated MIMO system
and the matrix modelling the antennas’ correlation as:
vec(H
c
) = R
1/2
HH
· vec(H) , (7)
where H is a (n
T
× n
R
) uncorrelated channel matrix
with independent, identically distributed complex val-
ued Rayleigh elements, vec(·) is the vector operator
which stacks the matrix H into a vector column-wise
and R
HH
is the correlation matrix which includes both
the transmit and receive antennas’ correlation. Taking
into consideration the common assumption that the
correlation between the various antennas composing
the transmitter side array is independent from the cor-
relation between the differentantennas composing the
receiver side array, the correlation matrix R
HH
can be
described by the Kronecker product of the transmit-
ter side correlation matrix R
TX
and the receiver side
correlation matrix R
RX
as:
R
HH
= R
TX
⊗ R
RX
. (8)
4 TRANSMISSION MODES
In this investigationa 4 × 4 MIMO system with QAM
modulation and a constant data rate with an over-
all throughput of 8 bits/s/Hz is considered. Hence,
the possible transmission modes defined by the active
layers are those shown in Table 1.
Table 1: Investigated QAM transmission modes.
throughput layer 1 layer 2 layer 3 layer 4
8 bit/s/Hz 256 0 0 0
8 bit/s/Hz 64 4 0 0
8 bit/s/Hz 16 16 0 0
8 bit/s/Hz 16 4 4 0
8 bit/s/Hz 4 4 4 4
The different transmission modes are defined by
the transmission of distinct QAM constellation sizes
along the available (active) layers.
5 GEOMETRIC MEAN VS.
SINGULAR VALUES
In order to improve the SVD-assisted MIMO systems
performance bit and power allocation strategies can
be used by selecting the appropriate number of active
layers, the modulation order and the transmit power
per layer in order to obtain the best performance, re-
quiring additional computational load and transmis-
sion overhead. In GMD-assisted MIMO systems all
the active layers have the same gain coefficient (the
geometric mean) performing with the same quality,
and hence power allocation is not required to improve
the overall MIMO channel performance.
A concrete number of active layers can be selected
to compute the geometric mean using (1) resulting
in different MIMO channel performances. By se-
lecting just one layer the geometric mean coincides
with the singular value of that layer (the one with
the largest value). Activating more layers with dif-
ferent singular values results in a geometric mean
whose value is lower than the largest singular value.
Even so the GMD-assisted MIMO performance is not
lower than the SVD-assisted one given there are lay-
ers with low valued singular values. In fact GMD-
assisted MIMO systems are (in general) more robust
than SVD-assisted without requiring power allocation
techniques. Nonetheless, the appropriate selection of
the number of active layers (which is synonymous of
bit allocation) can lead to the best performance, par-
ticularly under antennas’ correlation effect.
Fig. 2 represents the geometric mean PDF for un-
correlated (solid lines) and correlated (dashed lines)
4×4 GMD-assisted MIMO channels for a different
number of active layers. The analysis reveals that
the geometric mean decreases with the number of ac-
tive layers, which is event more evident under an-
tennas’ correlation effect. As the considered number
0 1 2 3 4 5 6
0
0.05
0.1
0.15
0.2
0.25
0.3
0.35
layer gain coefficient
PDF
Geometric mean PDF for 1 to 4 layers
uncorrelated, 1 layer
uncorrelated, 2 layers
uncorrelated, 3 layers
uncorrelated, 4 layers
correlated, 1 layer
correlated, 2 layers
correlated, 3 layers
correlated, 4 layers
Figure 2: Geometric mean PDF representation for uncorre-
lated (solid line) and correlated (dashed line) 4×4 MIMO
channels activating 1-4 layers.
PECCS2015-5thInternationalConferenceonPervasiveandEmbeddedComputingandCommunicationSystems
328

0 1 2 3 4 5 6
0
0.02
0.04
0.06
0.08
0.1
0.12
0.14
0.16
0.18
0.2
layer gain coefficient
PDF
2 layers MIMO: singular values vs. geometric mean
layer #2 singular value (uncorrelated)
layer #2 singular value (correlated)
layer #1 singular value (uncorrelated)
layer #1 singular value (correlated)
2 layers geometric mean (uncorrelated)
2 layers geometric mean (correlated)
Figure 3: Singular values vs. geometric mean PDF for a
two active layers MIMO channel.
of active layers increases lower valued singular val-
ues (weak layers) are used to compute the geomet-
ric mean through equation (1) obtaining a lower layer
gain coefficient (geometric mean). In conclusion, due
to antennas’ correlation weak layers results in lower
singular values and the geometric mean drops and
wider spreads when various layers are activated.
A key different between SVD-assisted and GMD-
assisted MIMO systems is that in the first ones re-
ducing the number of active layers doesn’t change the
singular values and the individual layer gain isn’t al-
tered. In contrast, in the second ones (i.e. GMD) se-
lecting a lower number of active layers results in a
larger geometric mean, which is the layer coefficient
gain.
Fig. 3 depicts the PDF of the gain coefficients
for a two active layers SVD-assisted MIMO sys-
tem (with singular values
p
ξ
i
) and GMD-assisted
one (geometric mean µ) for uncorrelated (solid lines)
and correlated (dashed lines) cases. In the SVD-
assisted MIMO channel the antennas’ correlation ef-
fect favours the existence of strong (layer # 1) and
weak (layer #2) layers as the active layers singu-
lar values PDF curves become more spaced and
smoothed. Hence power and bit allocation is required
to optimize the performance. Conversely, in the
GMD-assisted MIMO channel the geometric mean
wider spreads with correlation but the mean value
doesn’t significantly change (it slightly diminishes its
value). In consequence it can be concluded that the
GMD-assisted MIMO system behaves more robustly
than the SVD-assisted one under the effect of the an-
tennas’ correlation. SVD-assisted MIMO systems
are more sensitive to antennas’ correlation. In these
systems, as the correlation increases the strongest
layer becomes indeed stronger (larger
p
ξ
i
) and the
weakest gets a lower singular value. Therein the over-
all MIMO channel performance drops due to the ex-
istence of low quality layers. In the GMD-assisted
one, as the correlation increases the geometric mean
decreases but in a reduced percentage and the overall
performance slightly drops.
6 RESULTS
This section analysis the results of the simulation
of the GMD-assisted MIMO channel under different
conditions. The goal is determining how the anten-
nas’ correlation affects the geometric mean of the
singular values (layer gain coefficient) for different
transmission modes and correlation indexes as well
how the channel performance is affected. For conve-
nience the correlation coefficients have been chosen
to be the same for all the pairs of antennas.
6.1 Geometric Mean PDF and CCDF
Analysis
In GMD-assisted MIMO systems (with pre-coding)
bit- and power allocation make no sense as all the ac-
tive layers perform with the same quality (BER) given
the layers coefficients gain are the same. Neverthe-
less the selection of the appropriate number of active
layers leads to different overall performances as the
geometric mean differs. The larger the number of se-
lected layers the lower the geometric mean and the
lower the transmit QAM constellation size per layer
at a given quality.
Fig. 4 shows the CCDF of the two largest singular
values and the geometric mean of a 4×4 MIMO chan-
nel when the two best layers are selected (two active
layers). Under antennas’ correlation effect the sin-
gular value CCDF curve of the strongest layer shifts
right while the weak layer one shifts left. In conse-
quence the overall SVD-assisted MIMO system per-
formance diminishes. In the GMD-assisted one the
geometric mean CCDF doesn’t significantly vary with
antennas’ correlation and the overall channel perfor-
mance is approximately the same. Then, the conclu-
sion is drawn that the GMD-assisted MIMO system
is more robust against the antennas’ correlation effect
than the SVD-assisted one.
The separation between the CCDF curves pro-
vides information to anticipate the system perfor-
mance. When the CCDF curves are more spaced it
seems to be more convenient the activation of a re-
duced number of layers to reach a better performance.
This is because the weakest layer drops the computed
geometric mean. Comparing the CCDF curves for
uncorrelated and correlated MIMO channels, the last
ones spread wider showing that for correlated MIMO
channels choosing a reduced number of layers is more
Antennas'CorrelationInfluenceontheGMD-assistedMIMOChannels
Performance
329

0 1 2 3 4 5 6
0
0.1
0.2
0.3
0.4
0.5
0.6
0.7
0.8
0.9
1
layer gain coefficient
CCDF
2 layers MIMO: singular values vs. geometric mean CCDF
layer #2 singular value CCDF (uncorrelated)
layer #2 singular value CCDF (correlated)
layer #1 singular value CCDF (uncorrelated)
layer #1 singular value CCDF (correlated)
2 layers geometric mean CCDF (uncorrelated)
2 layers geometric mean CCDF (correlated)
Figure 4: Singular values and geometric mean CCDF for a
two active layers MIMO channel: uncorrelated (solid lines)
and correlated (dashed lines) cases.
appropriate. This effect is event larger in systems with
antennas’ correlation.
Figures 5 to 8 depict the geometric mean PDF
for a different number of active layers and distinct
correlation indexes. For simplicity, in the investiga-
tion the same correlation coefficient is considered for
each pair of antennas. Figure 5 represents the PDF
when just one active layer is active for uncorrelated
and correlated conditions, considering different cor-
relation degrees (ρ={0.0, 0.2, 0.4, 0.6}). In this case
the geometric mean takes the value of the largest sin-
gular value (the stronger layer) of the resulting chan-
nel matrix. Increasing the correlation index augments
the probability of having larger values, i.e., antennas’
correlation causes the strongest layer become even
stronger because the singular value increases (and in
this case the geometric mean).
The analysis of figures 6 to 8 gives different con-
clusions. Comparing the geometric mean PDF when
2, 3 and 4 layers are active for uncorrelated and corre-
lated cases with a correlation index ρ=0.2 (weak cor-
relation) it can be observed that the geometric mean
PDF doesn’t significantly change. As outcome, it
can be concluded that GMD-assisted MIMO systems
0 1 2 3 4 5 6 7 8 9 10
0
0.05
0.1
0.15
layer gain coefficient (geometric mean)
PDF
Geometric mean PDF for 1 layer and various correlation indexes (ρ)
uncorrelated
correlated, ρ=0.2
correlated, ρ=0.4
correlated, ρ=0.6
Figure 5: Geometric mean PDF for 1 active layer MIMO
and various correlation indexes (ρ=0, 0.2, 0.4, 0.6).
seem to be robust against antennas’correlation. When
two active layers are active the GMD-assisted MIMO
seems to robustly behave under the antennas’ corre-
lation effect. For the correlation indexes considered
in our analysis the geometric mean PDF curves ap-
proximately centre in the same value. As the two
weakest values are discarded the impact of the cor-
relation index on the geometric mean is not quite re-
markable and the system performance doesn’t notice-
ably change, except for the highest correlation index.
Figures 7 and 8 show the results when activating
three and four layers respectively. Now the geometric
mean value is more sensitive to correlation. This is
due to the activation of the weakest layers (three and
four) with low valued singular values which tend to
take lower values as the correlation index increases.
The first case shows to be more robust for low cor-
relation indexes while the second one is more sensi-
tive to correlation because the weakest layer (with the
lowest singular value) is much more sensitive to the
correlation effect, i.e., the singular value remarkably
decreases with the increment of the correlation index.
0 1 2 3 4 5 6
0
0.05
0.1
0.15
layer gain coefficient (geometric mean)
PDF
Geometric mean PDF for 2 layers and various correlation indexes (ρ)
uncorrelated
correlated, ρ=0.2
correlated, ρ=0.4
correlated, ρ=0.6
Figure 6: Geometric mean PDF for 2 active layers MIMO
and various correlation indexes (ρ=0, 0.2, 0.4, 0.6).
0 1 2 3 4 5 6
0
0.05
0.1
0.15
layer gain coefficient (geometric mean)
PDF
Geometric mean PDF for 3 layers and various correlation indexes (ρ)
uncorrelated
correlated, ρ=0.2
correlated, ρ=0.4
correlated, ρ=0.6
Figure 7: Geometric mean PDF for 3 active layers MIMO
and various correlation indexes (ρ=0, 0.2, 0.4, 0.6).
PECCS2015-5thInternationalConferenceonPervasiveandEmbeddedComputingandCommunicationSystems
330

0 1 2 3 4 5 6
0
0.05
0.1
0.15
0.2
0.25
layer gain coefficient (geometric mean)
PDF
Geometric mean PDF for 4 layers and various correlation indexes (ρ)
uncorrelated
correlated, ρ=0.2
correlated, ρ=0.4
correlated, ρ=0.6
Figure 8: Geometric mean PDF for 4 active layers MIMO
and various correlation indexes (ρ=0, 0.2, 0.4, 0.6).
6.2 The Effect of Correlation on the
System Performance
Figures 9 to 13 show the effect of the antennas’ cor-
relation on the performance of GMD-assisted MIMO
systems for the transmission modes considered in Ta-
ble 1. As reference, the BER for the equivalent SVD-
assisted MIMO transmission mode is depicted. In
the case in which just one active layer is active the
GMD- and SVD-assisted MIMO systems show the
same behaviour. The analysis of figures 8 to 12,
where a reduced number of available layers are ac-
tivated, reveals that the GMD-assisted MIMO system
performance increases with correlation (for low val-
ues). The reduction of the number of active layers dis-
cards weak layers in the computation of the geomet-
ric mean. Hence, the geometric mean is higher with
a lower number of active layers. Under the anten-
nas’ correlation effect, weak layers take indeed lower
singular values and strong layers become stronger
(higher singular values). As a result, the geometric
mean takes higher values in correlated systems with a
reduced number of active layers. This behaviour re-
verses when all layers are active. The increase in the
correlation coefficient changes the described perfor-
mance behaviourfor an intermediate number of active
layers.
6.3 Transmission Modes Comparison
Figures 14 to 16 represent the GMD-assisted MIMO
channel performance (BER) for the analysed trans-
mission modes described in Table 1 for different an-
tennas’ correlation degrees. Power allocation is not
considered in the different transmission modes and
the same power is transmitted along the active lay-
ers. Figure 14 compares the performances obtained
by the GMD-assisted MIMO system for the different
transmission modes when affected by antennas’ cor-
0 2 4 6 8 10 12 14 16 18 20
10
−6
10
−5
10
−4
10
−3
10
−2
10
−1
10
0
SNR (dB)
BER
BER for GMD−assisted MIMO with 1 active layer (256−0−0−0)
uncorrelated
correlated, ρ=0.2
Figure 9: BER for a GMD-assisted MIMO system with 1
active layer (TM 256-0-0-0): uncorrelated (solid line) vs.
correlated with ρ=0.2 (dashed line).
0 2 4 6 8 10 12 14 16 18 20
10
−9
10
−8
10
−7
10
−6
10
−5
10
−4
10
−3
10
−2
10
−1
10
0
SNR (dB)
BER
BER for GMD− vs. SVD−assisted MIMO with 2 active layers (16−16−0−0)
GMD uncorrelated
GMD correlated, ρ=0.2
SVD uncorrelated
SVD correlated, ρ=0.2
Figure 10: BER for a GMD- vs. SVD-assisted MIMO
system with 2 active layers (TM 16-16-0-0): uncorrelated
(solid line) vs. correlated with ρ=0.2 (dashed line).
0 2 4 6 8 10 12 14 16 18 20
10
−10
10
−8
10
−6
10
−4
10
−2
10
0
SNR (dB)
BER
BER for GMD− vs. SVD−assisted MIMO with 2 active layers (64−4−0−0)
uncorrelated
correlated, ρ=0.2
SVD uncorrelated
SVD correlated, ρ=0.2
Figure 11: BER for a GMD- vs. SVD-assisted MIMO sys-
tem with 2 active layers (TM 64-4-0-0): uncorrelated (solid
line) vs. correlated with ρ=0.2 (dashed line).
relation with a factor ρ = 0.2 (weak correlation). The
results reveal that the transmission mode 16-16-0-0
(with two active layers) is the one showing the best
performance.
The increase in the correlation coefficient affects
the MIMO performance as described above. Figure
15 represents the performance for the various trans-
Antennas'CorrelationInfluenceontheGMD-assistedMIMOChannels
Performance
331

0 2 4 6 8 10 12 14 16 18 20
10
−6
10
−5
10
−4
10
−3
10
−2
10
−1
10
0
SNR (dB)
BER
BER for GMD− vs. SVD−assisted MIMO with 3 active layers (16−4−4−0)
GMD uncorrelated
GMD correlated, ρ=0.2
SVD uncorrelated
SVD correlated, ρ=0.2
Figure 12: BER for a GMD- vs. SVD-assisted MIMO sys-
tem with 3 active layers (TM 16-4-4-0): uncorrelated (solid
line) vs. correlated with ρ=0.2 (dashed line).
0 2 4 6 8 10 12 14 16 18 20
10
−5
10
−4
10
−3
10
−2
10
−1
10
0
SNR (dB)
BER
BER for GMD− vs. SVD−assisted MIMO with 4 active layers (4−4−4−4)
GM uncorrelated
GMD correlated, ρ=0.2
SVD uncorrelated
SVD correlated, ρ=0.2
Figure 13: BER for a GMD- vs. SVD-assisted MIMO sys-
tem with 4 active layers (TM 4-4-4-4): uncorrelated (solid
line) vs. correlated with ρ=0.2 (dashed line).
mission modes and a correlation factor ρ = 0.4 (mod-
erate). Now the effect of the correlation is noticed.
The best performance is obtained with the transmis-
sion mode 256-0-0-0, i.e., activating just one layer.
Finally, figure 16 depicts the BER performance for a
correlation factor Figure ρ = 0.6 (strong). Now the an-
tennas’ correlation effect is noticeable and the trans-
mission mode with the best performance is (TM 256-
0-0-0), i.e., the case in which just one layer is active.
The analysis of the three figures provides clear
conclusions. The transmission mode 4-4-4-4 with
four active layers shows the worst performance in all
the cases. This is because in the computation of the
geometric mean we are considering the layer fourth,
the one with the lowest singular value. Moreover, the
correlation effect favours the appearance of weak lay-
ers which negatively affects the resulting geometric
mean of the singular values. Furthermore, the correla-
tion also favours the appearance of very strong layers.
In this case, the lower the number of active layers the
higher the resulting geometric mean. This is because
the transmission mode 256-0-0-0 shows the best per-
formance for moderate and strong correlation.
A key point in this discussion is the comparison
between transmission mode 16-4-4-0 (with three ac-
tive layers) and transmission modes 64-4-0-0 and 16-
16-0-0 (with two active layers). For moderate correla-
tion transmission mode 16-4-4-0 performs better than
64-4-0-0. As correlation increases the third active
layer shows a lower singular value and the geomet-
ric mean drops resulting in a worse performance (as
shown for ρ=0.4 and ρ=0.6). Furthermore, the trans-
mission mode 16-16-0-0 show a better performance
than 64-4-0-0 (in this example). The equal distribu-
tion of bits along the active layers seems to be better
than the unequal distribution given by transmission
mode 64-4-0-0. Nevertheless this is not a general rule
and depends on the resulting geometric mean.
0 2 4 6 8 10 12 14 16 18 20
10
−6
10
−5
10
−4
10
−3
10
−2
10
−1
10
0
SNR (dB)
BER
BER for correlated GMD−assisted MIMO with various active layers (1 to 4), ρ=0.2
TM 256−0−0−0
TM 16−16−0−0
TM 64−4−0−0
TM 16−4−4−0
TM 4−4−4−4
Figure 14: BER comparison for a GMD-assisted MIMO
system with various active layers and a Tx/Rx correlation
factor ρ=0.2.
0 2 4 6 8 10 12 14 16 18 20
10
−6
10
−5
10
−4
10
−3
10
−2
10
−1
10
0
SNR (dB)
BER
BER for correlated GMD−assisted MIMO with various active layers (1 to 4), ρ=0.4
TM 256−0−0−0
TM 16−16−0−0
TM 64−4−0−0
TM 16−4−4−0
TM 4−4−4−4
Figure 15: BER comparison for a GMD-assisted MIMO
system with various active layers and a Tx/Rx correlation
factor ρ=0.4.
7 CONCLUSIONS
This paper analyses the performance of exemplary
4×4 GMD-assisted MIMO systems affected by an-
tennas’ correlation focussing on the geometric mean
PECCS2015-5thInternationalConferenceonPervasiveandEmbeddedComputingandCommunicationSystems
332
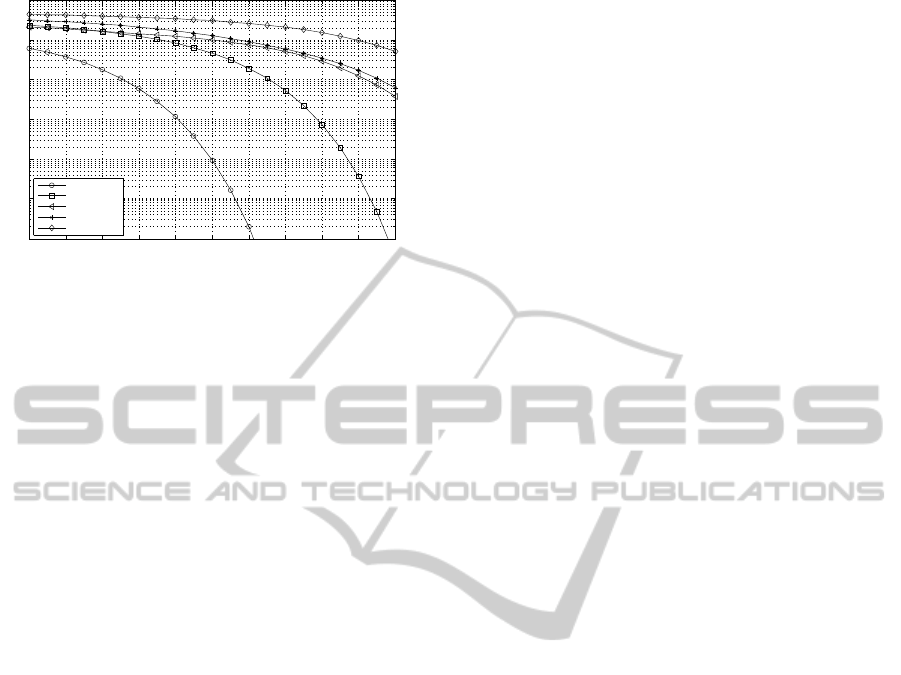
0 2 4 6 8 10 12 14 16 18 20
10
−6
10
−5
10
−4
10
−3
10
−2
10
−1
10
0
SNR (dB)
BER
BER for correlated GMD−assisted MIMO with various active layers (1 to 4), ρ=0.6
TM 256−0−0−0
TM 16−16−0−0
TM 64−4−0−0
TM 16−4−4−0
TM 4−4−4−4
Figure 16: BER comparison for a GMD-assisted MIMO
system with various active layers and a Tx/Rx correlation
factor ρ=0.6.
PDF and CCDF of the singular values as well as the
BER, comparing with the uncorrelated one. Several
transmission modes have been defined by the acti-
vation of a different number of layers. The analy-
sis takes into consideration various antennas’ correla-
tion indexes to show the robustness of GMD-assisted
MIMO systems against correlation.
The simulations outcomes demonstrate that an-
tennas’ correlation affects the SVD-assisted MIMO
channel performance by decreasing its throughput
and increasing the BER. This behaviour is caused by
the existence of predominant weak and strong lay-
ers with corresponding small and large valued singu-
lar values respectively, which are the particular layer
gain coefficient. In the case of GMD-assisted MIMO
systems the number of active layers leads to differ-
ent conclusions. The PDF and CCDF of the singu-
lar values and their geometric mean seems to be a
proper way to anticipate the SVD-assisted and GMD-
assisted MIMO systems performance.
As shown, for a given number of active lay-
ers, antennas’ correlation significantly spreads the
singular values CCDF curves dropping the overall
channel performance. Nevertheless the geometric
mean CCDF curves don’t significantly change with
correlation. As the separation between the singu-
lar values CCDF curves increases, the overall SVD-
assisted MIMO channel performance drops due to
the poor performance of weak layers (with low val-
ued gain coefficients). Conversely, the geometric
mean CCDF curve for a given number of active layers
doesn’t remarkably change with correlation, conclud-
ing that GMD-assisted MIMO systems are more ro-
bust against antennas’ correlation than SVD–assisted
ones. Then, in general terms GMD-assisted MIMO
systems performs better than SVD-assisted ones, spe-
cially when weak layers exist (particularly in corre-
lated channels).
The activation a different number of layers results
in distinct transmission modes which show different
performances as shown in the results. In order to
minimize the overall BER the same constellation size
as well as the same transmit power per layer should
be used. Although individual layers in GMD-assisted
MIMO systems perform in the same way as the gain
coefficient is the same, the appropriate usage of dif-
ferent constellations per layer can improve the overall
MIMO channel performance.
Activating a larger number of layers takes into ac-
count weak layers. In consequence, due to the low
valued singular values of weak layers the computed
geometric mean diminishes and the GMD-assisted
MIMO system performance drops. This outcome is
much more remarkable as the antennas’ correlation
increases. At the opposite side, activating just one
layer leads to the largest geometric mean value. Nev-
ertheless the best performance is not reached because
a high order constellation is transmitted. An interme-
diate number of active layers seems to be the most
appropriate solution which depends on the particular
correlation index.
REFERENCES
Ahrens, A., Cano-Broncano, F., and Benavente-Peces, C.
(2013). Power distribution for svd-aided mimo trans-
mission with receiver-side antennas correlation. In
10th IASTED International Conference on Signal Pro-
cessing, Pattern Recognition, and Applications (SP-
PRA), Innsbruck (Austria).
Benavente-Peces, C., Cano-Broncano, F., Ahrens, A.,
Ortega-Gonzalez, F., and Pardo-Martin, J. (2013).
Analysis of singular values pdf and ccdf on receiver-
side antennas correlated mimo channels. Electronics
Letters, 49(9):625–627.
Foschini, G. J. and Gans, M. J. (1998). On limits of wire-
less communications in a fading environment when
using multiple antennas. Wireless Personal Commu-
nications, 6:311–335.
Haykin, S. S. (2002). Adaptive Filter Theory. Prentice Hall,
New Jersey.
Janaswamy, R. (2002). Effect of element mutual coupling
on the capacity of fixed length linear arrays. Antennas
and Wireless Propagation Letters, IEEE, 1(1):157–
160.
Jiang, Y., Li, J., and Hager, W. W. (2005). Joint transceiver
design for mimo communications using geometric
mean decomposition. Signal Processing, IEEE Trans-
actions on, 53(10):3791–3803.
Kinjo, S. and Ohno, S. (2013). A tomlinson-harashima pre-
coding for correlated mimo channels. In Intelligent
Signal Processing and Communications Systems (IS-
PACS), 2013 International Symposium on, pages 257–
262.
Antennas'CorrelationInfluenceontheGMD-assistedMIMOChannels
Performance
333

Lee, W.-Y. (1973). Effects on correlation between two mo-
bile radio base-station antennas. IEEE Transactions
on Vehicular Technology, 22(4):130140.
Ozgur, A., Leveque, O., and Tse, D. (2013). Spatial de-
grees of freedom of large distributed mimo systems
and wireless ad hoc networks. Selected Areas in Com-
munications, IEEE Journal on, 31(2):202–214.
Yang, P., Xiao, Y., Yu, Y., and Li, S. (2011). Adaptive spa-
tial modulation for wireless mimo transmission sys-
tems. IEEE Communications Letters, 15:602–604.
Zhou, Z., Vucetic, B., Dohler, M., and Li, Y. (2005). Mimo
systems with adaptive modulation. IEEE Transactions
on Vehicular Technology, 54:1828 – 1842.
PECCS2015-5thInternationalConferenceonPervasiveandEmbeddedComputingandCommunicationSystems
334
