
Study of Energy Evaluation Control
Yasushi Yamamoto
1
, Shinya Hasegawa
2
, Satoru Iwamori
2
and Shigeru Yamaguchi
3
1
ISUZU Advanced Engineering Center Ltd., Fujisawa, Kanagawa-ken, Japan
2
Department of Mechanical Engineering, School of Science, Tokai University, Kanagawa-ken, Japan
3
Department of Physics, School of Science, Tokai University, Kanagawa-ken, Japan
Keywords: Lagrangian, Hamiltonian, Energy, Bang-bang Controller.
Abstract: One of the objectives in control theory is to ensure that a control system converges to a target state in the
shortest possible time. To achieve that objective, we studied a control method that combines the Lagrangian,
the Hamiltonian, and a bang-bang controller. Referred to as energy evaluation control (EEC), this method
evaluates the control state using the Lagrangian, and evolves the control output using the Hamiltonian. Here,
the Lagrangian and the Hamiltonian are defined for the deceleration field. The control result from the EEC
is fast and robust. Moreover, EEC has the same control strategy as the sliding-mode control, and hence can
be incorporated within it.
1 INTRODUCTION
The Lagrangian and Hamiltonian have been used in
control theory to establish robust control methods
(Bloch, et al., 2000), (Choi, et al., 1997). In addition,
the control logic by using these energys are
examined, and the application experiment to the
inverted pendulum is conducted (Fantoni et al.,
2000), (Ortega, and Spong, 2000),(Ortega, et al.,
2000).
Moreover, one objective in control execution is
to direct the control system towards target states in
the shortest possible time. A bang-bang controller is
one that is able to realize this objective. However, a
bang-bang controller has one drawback in that it
does not perform well when external forces are
changed. Although in examining the control rule,
which determines the feedback of a state quantity to
the change in output of the bang-bang controller
performed on the control object, the output is
changed in the second-half of the cycle and has a
slight complicated control structure (Vakilzadeh and
Keshavarz, 1982).
In this report, we studied a control method which
determines the control output of the bang-bang
controller using the Lagrangian and its convergence
control output using the Hamiltonian. We refer to it
as the “energy evaluation controller” (EEC) because
this controller evaluates the energy of the control
state. The features of EEC is that a formula can be
made simpler than the conventional energy method,
and that a control result becomes the shortest time
control because EEC based on the bang-bang
controller.
We begin by explaining the control rule of the
EEC for the simple control model. Next, we propose
the adjustment method for the external force of the
damper and friction. Finally, we explain that the
EEC as a kind of sliding-mode control (SMC).
2 SWITCHING OF CONTROL
OUTPUT USING THE
LAGRANGIAN
A bang-bang controller is a controller that using
maximum thrust enables abrupt changes in state
through acceleration and deceleration of a controlled
object. A simple model of the bang-bang controller
(Fig. 1) and its control cycles (Fig. 2) assumes that
the actuator can generate a fixed thrust ±F
max
. The
notation and significance of the variables are: x:
stroke, : velocity, X: target position, T: kinetic
energy and -F
max
(X-x): braking work. The switching
of the actuator thrust is the instant when the braking
energy and the kinetic energy are equal.
Next, the Lagrangian is calculated for the
deceleration field which is generated by the thrust
from the deceleration force of the actuator. Here, the
reference position of the deceleration field is set to a
target position X. The Lagrangian L is defined as the
550
Yamamoto Y., Hasegawa S., Iwamori S. and Yamaguchi S..
Study of Energy Evaluation Control.
DOI: 10.5220/0005538105500553
In Proceedings of the 12th International Conference on Informatics in Control, Automation and Robotics (ICINCO-2015), pages 550-553
ISBN: 978-989-758-122-9
Copyright
c
2015 SCITEPRESS (Science and Technology Publications, Lda.)

-F
max
+F
max
Actuator
X
0
Target Position
Controller
x
Figure 1: Simple model of a bang-bang controller.
+F
max
−F
max
Actuator
Force
Stroke
X
x
0
0
Velocity
0
0
F
max
X
x
T
U
Energy
Time
0
x
Figure 2: Operation cycle of the bang-bang Controller.
difference between kinetic energy T and potential
energy U:
L = T - U (1)
2
2
1
xmT
=
(2)
()
.
max
−=
x
X
dxFU
(3)
The potential energy of the decelerating field
corresponds to the possible work during braking.
Therefore the switching time to apply thrust from
the actuator is the instance when the value of the
Lagrangian takes value 0. It is necessary to consider
the direction of motion of the controlled object to
decide the control output. Hence the sign (plus or
minus) of the velocity is added to the kinetic energy,
and (1) is modified to:.
()
.
2
'
max
2
−−=
x
X
dxFxm
x
x
L
(4)
The sign of the Lagrangian L' then determines
the direction of the control output u:
.
'
'
max
F
L
L
u =
(5)
Here F
max
is the maximum thrust that can be
generated by the actuator. The control is simple and
seems to work fast. However, in actual control
systems, it does not work properly because the
estimation error for the Lagrangian is generated by
the external force that cannot be predetermined. To
address this issue, calculations of the Lagrangian
and the control output are done repeatedly at every
control cycle, in trying to move the controlled object
towards the target position despite the estimation
errors associated with the Lagrangian.
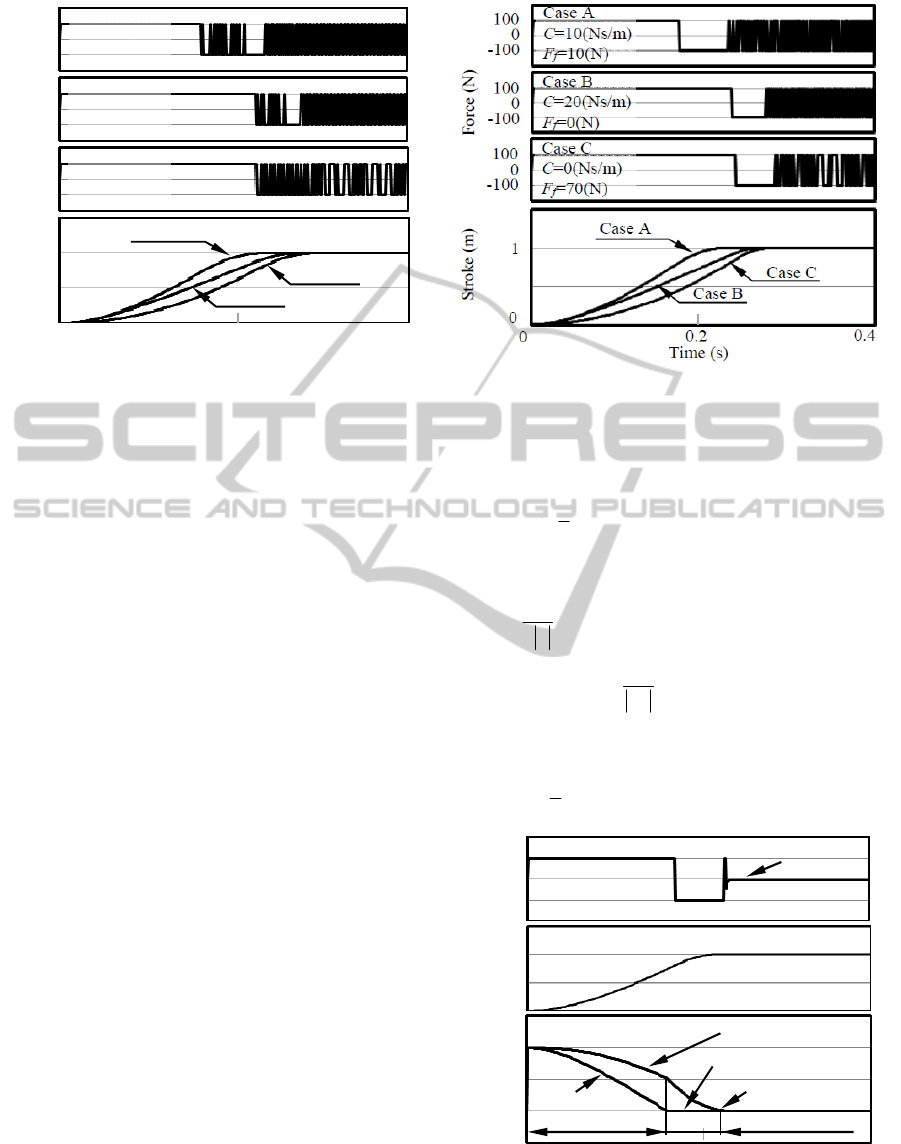
For the control target illustrated in Fig. 3, we
obtain the EEC control result presented in Fig. 4.
The simulation settings are actuator thrust:
()
N100± , mass: 1(kg), initial position:-1(m), target
position: 0(m). Coefficient of damping C(Ns/m) and
frictional force F
f
(N) is set as follows: Case A
(C=10, F
f
=10), Case B (C=20, F
f
=0), Case C (C=0,
F
f
=70),. Furthermore, switching of the control
output is assumed to occur abruptly. The simulation
result shows that the EEC can control the object to
the target and it's result seems robust. At the same
time, this simulation result shows that the control out
put is not shape of ideal bang-bang controller's
output.
Figure 3: System of control target with dumper.
3 CORRECTION OF THE
POTENTIAL ENERGY
EEC can converge the control object to the target
position even if the external force act on the control
ovject. However, hunting of control output occurs,
as shown in Fig. 4. This hunting is caused by the
estimation error associated with the potential energy
of the deceleration field which generated by the
damping force and frictional force. On the other
hand the braking work done by damping force W
d
is
calculated from the equation (6) and the braking
work done by frictional force W
f
is given by (7).
Here the Coulomb's friction model is adopted and
the coefficient of friction F
f
is assumed as constant.
Therefor the potential energy is corrected to (8).
Figure 5 shows the simulation result of using
equation (8). The simulation result shows that the
bang-bang control is almost realised by this
correction.
StudyofEnergyEvaluationControl
551
Force (N)
0
0.4
0.2
Stroke (m)
Time
(
s
)
Case A
Case A
C=10(Ns/m)
F
f
=10(N)
Case B
C=20(Ns/m)
F
f
=0(N)
Case C
C=0(Ns/m)
F
f
=70(N)
Case B
Case C
1
100
0
-100
100
0
-100
Figure 4: Control result of the EEC Simulation conditions
are changed over 3 cases.
ddc
xCxW
=
(6)
dff
xFW =
(7)
fc
x
X
WWdxFU ++−=
)(
max
(8)
C : coefficient of damper, F
f
: frictional force.
4 CONVERGENCE OF
CONTROL OUTPUT USING
THE HAMILTONIAN
Figure 5 also shows the actuator output after the
controlled object has reached its target state. To
reduce energy consumption, it is desirable to stop
the control output after control finishes (velocity is
zero and positional deviation is zero). Here, the
Hamiltonian H for the deceleration field (9) is
introduced into the EEC procedure. As the
Hamiltonian represents the total energy of the
deceleration field, takes the value zero when the
object velocity and positional deviation become
zero. Therefore, to stop the actuator thrust at the end
of the control period, the convergence rate of the
actuator thrust must be determined; this is achieved
by multiplying the Hamiltonian by the actuator
thrust F
max
and the gain K
h
. To take into account the
upper and lower limits of the control output, HK
h
is
restricted to values between 0 and 1. Therefore, the
formula expressing the thrust of the actuator is
modified to (10). Because of this, the Lagrangian is
Figure 5: Control result of the EEC Effect of the potential
energy correction potential energy.
also corrected to (11). Finally, the control output is
modified to give (12)
()
fc
x
X
WWdxFxmH ++−+=
max
2
2
1
(9)
maxmax
' FHKF
h
= )10( ≤⋅≤
h
KH
(10)
++−=
x
X
fc
WWdxFxm
x
x
L
max
2
'
2
''
(11)
max
'
''
''
F
L
L
u =
(12)
Because F
max
is changed in the next control step,
the Hamiltonian also changes:.
fc
x
X
WWdxFxmH ++−+=
max
2
'
2
1
(13)
0
-1
Stroke (m)
Force (N)
100
0
-100
Control output is converged
0
0.4
0.2
Time (s)
0
100
Energy (J)
Hamiltonian
Lagrangian
L=0
Accel. field
Deceleration. field
H=0
Figure 6: Using the Hamiltonian to control output
convergence.
ICINCO2015-12thInternationalConferenceonInformaticsinControl,AutomationandRobotics
552

Convergence of the control output using the
Hamiltonian (Fig. 6) shows that the control output
has converged to zero when the control converges.
On the other hand the Lagrangian is restrained to 0.
It is means that the control object reached to the
target position according to the deceleration field.
5 CONTROL STRATEGY OF ECC
Figure 7 shows the phase plane, obtained using (7),
that determines the control output in EEC. For
comparison, a switching phase plane for SMC is sho
wn in Fig. 8. SMC has a switching phase plane of
control output that is similar to EEC (
Efe, et al., 2000),
and hence these two methods have similar control
structures. Usually, the displacement and velocity
are associated with the axes of the phase plane of
SMC. However, other axes can be used because
SMC can be extended to any dimension. This
implies that the EEC is included in SMC.
EEC has good characteristics in that the gradient
of the output switching line is always set to −1,
because the kinetic energy and braking energy have
the same units (J). Moreover, the Lagrangian is
restricted to zero within the SMC context. This
behavior indicates that the controlled object will
move naturally. Thereby EEC can simplify the
problem of control, as mentioned above.
Figure 7: Phase plane for EEC and conventional SMC.
6 CONCLUSIONS
EEC was proposed that switches the control output
using the Lagrangian and uses the Hamiltonian to
converge the control output. And we proposed the
correction method for the damping force and
frictional force. Finaliy the control output of EEC
becams almost same as the bang-bang controller's
output. Therefor the EEC can control the controlled
object in shortest time.
EEC was found to have the same phase space
structure as SMC and hence is included to the SMC.
However, the EEC has practical advantages in that
the phase plane can be simplified by choosing
energy as one of the axis variables in the phase plane
for the control output. In consequence, the output
switching line for EEC is the diagonal of gradient −1.
REFERENCES
A. M. Bloch, N. E. Leonard and J. E. Marsden, 2000.
Controlled Lagrangians and the Stabilization of
Mechanical Systems I: The First Matching Theorem.
IEEE Transactions on Automatic Control, vol. 45, no.
12, pp. 2253–2270.
Y. Choi, W. K. Chung and Y. Youm, 1997 Robust
Control of Manipulators using Hamiltonian
Optimization. IEEE International Conference on
Robotics and Automation, pp. 2358–2364.
I. Fantoni, R. Lozano, and M. W. Spong, 2000. Energy
Based Control of the Pendubot, IEEE Transactions on
Automatic Control, vol. 45, pp. 725–729.
R. Ortega, M. W. Spong, 2000, Stabilization of
underactuated mechanical systems via interconnection
and damping assignment, IFAC 2000 workshop on
Lagrangian and Hamiltonian Methods, vol. 47,
pp.1218-1233.
R. Ortega, A. J. van der Schaft, I. Mareels, and B. M.
Maschke, 2000. Putting energy back in control.
Control System Magazine, vol. 21, pp. 18-33.
I. Vakilzadeh, A. A. Keshavarz, 1982. Bang-bang Control
of a Second-Order Non-Linear Stable Plant with
Second-Order Nonlinearity, KYBERNETIKA. vol. 18,
no. 1, pp.66-71.
M. O. Efe, O. Kaynak and X. Yu, 2000. Sliding Mode
Control of a Three Degrees of Freedom Anthropoid
Robot by Driving the Controller Parameters to an
Equivalent Regime. Journal of Dynamic Systems,
Measurement, and Control, vol. 122, pp. 632–640.
StudyofEnergyEvaluationControl
553
