Impact of Optical Preamplifier Beat Noise on Inter-Satellite Coherent

Optical Communication System

Xiangnan Liu, Chengyong Xiang, Liang Zhang and Yingfei Li

Beijing Research Institute of Telemetry, South Dahongmen Road 1, Feng Tai District, Beijing, China

Keywords: Optical Preamplifier, Coherent Optical Communication, ASE Noise.

Abstract: The performance of Inter-Satellite coherent optical communication systems is partly limited by the beat

noise between the amplified spontaneous emission (ASE) noises and local oscillator. In order to improve the

optical detection sensitivity in inter-satellite optical communication links, a model of inter-satellite coherent

optical communication is established. And the origin of noise in this system with optical pre-amplifier is

investigated. The impact of optical pre-amplifier key parameters on performance of inter-satellite coherent

optical communication systems is discussed. Besides, bit error rate (BER) versus signal power, noise figure

and gain are numerically calculated. The results illustrated that it is effective to improve the optical

detection sensitivity and degrade the bit error rate using an optical pre-amplifier with low noise figure.

1 INTRODUCTION

Optical communication in space is an attractive and

available alternative to classical RF communication.

Optical space communication systems have the

potential for substantially higher data rates than RF-

based solutions with similar onboard mass, volume

and power consumption. Besides, optical space

communication does not require frequency

regulation and provides inherently secure data links

by means of a high beam directivity (Björn Gütlich,

2013; Patricia Martin-Pimentel, 2014).

Several optical space communication missions have

been successfully demonstrated and verified in the

recent past, and are today used in commercial

system (F. Heine, 2015; Daniel Troendle, 2014;

Mark Gregory, 2012).

Nowadays, inter-satellite coherent optical

communication draws more research interests as a

coherent BPSK data transmission at a data rate of

5.625 Gbps has been performed in-orbit between

satellite NFIRE and satellite TerraSAR-X (B.

Smutny, 2008).

In the inter-satellite coherent optical communication

system, the optical preamplifier could provide the

higher gain and detection sensitivity. However, as

the introduction of optical preamplifier, which

enhances the system performance but brings a new

challenge. That is the beat noise between the

amplified spontaneous emission (ASE) noises from

optical preamplifier and local oscillator (LO),

reducing the received signal-to-noise ratio (SNR)

(P. C. Becker, 1999).

As far as we know, there are not many literatures

examining the impact of beat noise on Inter-Satellite

Coherent Optical Communication System

systematically.

In this paper, we first describe the mathematical

expression of beat noise between the ASE noise and

LO in an inter-satellite coherent receiver.

Subsequently, the impacts of different parameters on

the system’s performance are investigated.

2 SYSTEM SETUP AND NOISE

ANALYSIS

2.1 System Setup

A system setup of an inter-satellite coherent optical

communication link is showed in Figure 1. The

coherent receiver is homodyne BPSK synchronous

receiver. In the inter-satellite link, the main noise to

impair the system includes the beat noise induced by

LO and ASE noises, shot noise, thermal noise, and

background noise. Throughout this paper, all the

parameters used in analysis are listed in Table 1

unless specified.

Liu X., Xiang C., Zhang L. and Li Y..

Impact of Optical Preampliﬁer Beat Noise on Inter-Satellite Coherent Optical Communication System.

DOI: 10.5220/0005570900480051

In Proceedings of the 6th International Conference on Optical Communication Systems (OPTICS-2015), pages 48-51

ISBN: 978-989-758-116-8

 2015 SCITEPRESS (Science and Technology Publications, Lda.)

Figure 1: System setup of an inter-satellite coherent

optical communication link.

Table 1: Parameters used in the calculation.

Optical Wavelength(λ) 1550nm

Range(Z) 40000km

Transmit Power(P

) 30dBm

Transmit telescope Diameter(D

) 15cm

Receive telescope Diameter(D

) 30cm

Transmit/ Receive efficiency(τ

) 0.8

Thermal Noise temperature(T) 300K

R 50Ω

RIN -145dB/Hz

Optical Bandwidth (B

) 10GHz

Electrical Bandwidth (B

) 7.5GHz

coupler efficiency(η

) 0.8

The input signals to a coherent receiver consist of

signal E

(t), LO E

(t) and ASE noise E

(t)

(N.A.OLSSON, 1989; Hongbo Lu, 2010;

J.G.Proakis, 2000), which are represented by:

() ()

=cos+

srs

Et GP t

(1)

() ()

=cos+

lo lo lo lo

Et P t

(2)

() ()

=cos[2(+)+]

sp s k

Et N k t

δυ π υ δυ



(3)

()

NnG h

=−

(4)

2MB

δυ

= (5)

where P

and P

represent power of signal and LO

respectively,

and

denote optical phase of

signal and LO respectively , G is amplifier gain, n

is noise figure, h is the Planck constant, ν is optical

carrier frequency.

is a random phase for each

component of spontaneous emission. In equation 5,

is optical Bandwidth and δv is an arbitrarily small

frequency width and is chosen so that M is an

integer value.

The recovered inphase and quadrature signals can be

easily shown as

()

() () ()

()

-+-

ssplo

Ish

ssplo

Et E t E t

it R i t

Et E tE t







(6)

()

() () ()

()

++j

-+-j

ssp lo

Qsh

ssplo

Et E t E t

it R it

Et E t E t







(7)

2.2 Noise Analysis

In the coherent receiver, the variance of the beat

noises between LO and ASE noise is given by

-0

lo sp lo e

NRPNB

(8)

where R is the responsivity, B

is electrical

bandwidth.

The variance of shot noise is written as

()

=2 + +

hot e o r lo

NeRBNBGPP

(9)

where e is electron quantity.

The variance of thermal noise is given by

th b e

kTB R

(10)

where k

is Boltzmann constant, R

is equivalent

resistance, T is temperature, and RIN is relative

intensity noise.

For the inter-satellite link, the background radiation

is a shot noise with variance as

()

=2 +

bg e sky sun

NeRBPP

(11)

where P

sky

and P

sun

represent the background

irradiance of the Sun and the sky respectively.

Consequently, the electrical SNR in the considered

coherent receiver has the expression as

+++

rlo

lo sp shot th bg

RGPP

SNR

NNNN

(12)

To investigate the BER of BPSK for homodyne

detection, its analytical BER is calculated by

equation (13), where the probability density function

p(Δ

) of phase noise is assumed to be Gaussian-

distributed with mean zero.

()()()

cos

BER erfc SNR p d

φφφ



=⋅ΔΔΔ





(13)

3 NUMERICAL RESULTS

First we calculate the SNR of system versus

preamplifier gain with noise figure as parameter. In

the calculation, the received power P

is -40dBm and

LO power P

is 10dBm. As shown in Figure 2, the

SNR of the system is monotonically increasing as

the surge of preamplifier gain, but gradually reach

saturation. This can be mainly attributed to both the

ImpactofOpticalPreamplifierBeatNoiseonInter-SatelliteCoherentOpticalCommunicationSystem

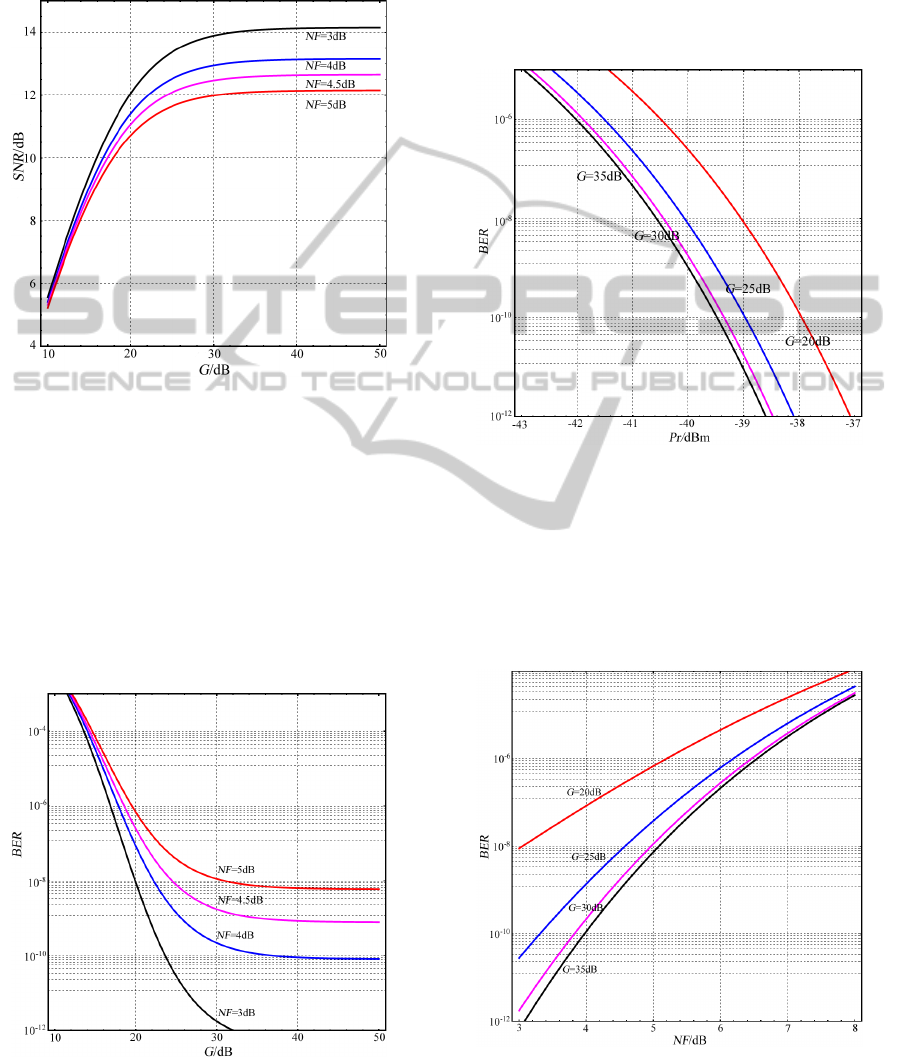
received power and the LO-ASE beat noise increase

as the surge of preamplifier gain. It is not used for

G=10dB because of the SNR is less than 6dB. And it

is also no enhancement for SNR while the

preamplifier gain reaches to 30dB.

Figure 2: SNR versus gain of preamplifier with different

noise figure. (NF=5dB red line, NF=4.5dB magenta line,

NF=4dB blue line, NF=3dB black line).

Then we calculate the BER versus preamplifier gain

with noise figure as parameter. The results are

shown in Figure 3.The BER of the system is

decreasing as preamplifier gain increasing, but

gradually reach a minimum. It is worthy to know

that the BER is 10

-10

, 10

-9

, 10

-8

for the NF of 4dB,

4.5dB and 5dB, respectively when the gain larger

than 30dB. The BER will be increasing an order as

the NF grows up 0.5dB.

Figure 3: BER versus gain of preamplifier with different

noise figure. (NF=5dB red line, NF=4.5dB magenta line,

NF=4dB blue line, NF=3dB black line).

We set noise figure at the value of 4.5dB, and

calculate BER versus receive power P

with

preamplifier gain as parameter. As the results shown

in Figure 4, the sensitivity will be enhanced 1.5dB

when the BER is 10

-9

for comparing the gain of

20dB and 30dB. It is possible to enhance the

sensitivity via increasing the preamplifier gain with

the same noise figure to maintain BER.

Figure 4: BER versus received power with different gain.

(G=20dBm red line, G=25dB magenta line, G=30dB blue

line, G=35dB black line).

BER versus NF with different gain are calculated

and illustrated in Figure 5. The BER is

monotonically increasing as the surge of NF. It is

possible to decrease the BER via increasing the gain

if the NF maintains constant.

Figure 5: BER versus NF with different gain. (G=20dBm

red line, G=25dB magenta line, G=30dB blue line,

G=35dB black line).

OPTICS2015-InternationalConferenceonOpticalCommunicationSystems

As shown in Figure 6, it also concludes that we

could enhance the sensitivity via increasing the

preamplifier gain if the BER maintains constant.

Figure 6: Received power Pr versus gain with different

noise figure. (NF=5dB red line, NF=4.5dB magenta line,

NF=4dB blue line, NF=3dB black line).

We last calculate BER versus the rms phase error

with noise figure as parameter. And we set the gain

at the value of 30dB. The results are shown in Figure

7. In order to maintain BER at 10

-9

and the rms

phase error less than 0.175rad, it shows that the

noise figure should less than 4.5 dB if the gain is

30dB.

Figure 7: BER versus rms phase error with different noise

figure. (NF=5dB red line, NF=4.5dB magenta line,

NF=4dB blue line, NF=3dB black line).

4 CONCLUSIONS

We have studied the impact of optical preamplifier

ASE beat noise on an inter-satellite coherent optical

communication system. It is numerically shown that

the presence of preamplifier would enhance the

received sensitivity at a certain LO power level.

REFERENCES

Björn Gütlich, Rolf Meyer, Sabine Phillip-May et al, 2013.

German Roadmap on Optical Communication in

Space. Advanced Solid-State Lasers Congress

Technical Diges.

Patricia Martin-Pimentel, Christoph Rochow, Mark

Gregory et al, 2014. Laser Com in space, the

operational concept. SpaceOps 2014 Conference.

F. Heine, G. Mühlnikel, H. Zech et al., 2015. LCT for the

European data relay system in orbit commissioning of

the Alphasat and Sentinel 1A LCTs. Proc. of SPIE.

Daniel Troendle, Christoph Rochow, Patricia Martin

Pimentel et al, 2014. Optical LEO-GEO Data Relays

from Demonstrator to Commercial Application. 32nd

AIAA International Communications Satellite Systems

Conference.

Mark Gregory, Frank Heine, Hartmut Kämpfner et al,

2012. Commercial optical inter-satellite

communication at high data rates. Optical Engineering.

B. Smutny, R. Lange, H. Kämpfner et al, 2008. 5.625

Gbps optical INTER-SATELLITE communication

link verified in orbit. Proc. of the Ka and Broadband

Communications Conference.

P. C. Becker, N. A. Olsson and J. R. Simpson, 1999.

Erbium-doped fiber amplifiers: fundamentals and

technology. CD: Academic Press. San Diego.

N. A. Olsson, 1989. Light wave systems with optical

amplifiers .Journal of Lightwave Technology.

Hongbo Lu, Shaoliang Zhang, Changyuan Yu and Pooi

Yuen Kam, 2010. Impact of amplifier beat and

nonlinear phase noises on coherent optical

communication system, The 9th International

Conference on Optical Communications and Networks

(ICOCN2010).

J. G. Proakis, 2000. Digital Communications, McGraw-

Hill. New York, 4th edition.

ImpactofOpticalPreamplifierBeatNoiseonInter-SatelliteCoherentOpticalCommunicationSystem