Modeling Oxygen Dynamics under Variable Work Rate

Alexander Artiga Gonzalez

, Raphael Bertschinger

, Fabian Brosda

, Thorsten Dahmen

Patrick Thumm

and Dietmar Saupe

Dept. of Computer and Information Science, University of Konstanz, Konstanz, Germany

Dept. of Sport Science, University of Konstanz, Konstanz, Germany

Keywords:

Mathematical Modeling, Simulation, Oxygen Dynamics, Variable Work Rate.

Abstract:

Measurements of oxygen uptake and blood lactate content are central to methods for assessment of physical

ﬁtness and endurance capabilities in athletes. Two important parameters extracted from such data of incre-

mental exercise tests are the maximal oxygen uptake and the critical power. A commonly accepted model of

the dynamics of oxygen uptake during exercise at constant work rate comprises a constant baseline oxygen

uptake, an exponential fast component, and another exponential slow component for heavy and severe work

rates. We generalized this model to variable load protocols by differential equations that naturally correspond

to the standard model for constant work rate. This provides the means for prediction of oxygen uptake re-

sponse to variable load proﬁles including phases of recovery. The model parameters were ﬁtted for individual

subjects from a cycle ergometer test. The model predictions were validated by data collected in separate tests.

Our ﬁndings indicate that oxygen kinetics for variable exercise load can be predicted using the generalized

mathematical standard model, however, with an overestimation of the slow component. Such models allow

for applications in the ﬁeld where the constant work rate assumption generally is not valid.

1 INTRODUCTION

Physiological quantities such as heart rate, lactate

concentration, or respiratory gas exchange are impor-

tant parameters to assess the performance capabilities

of athletes in competitive sports. In particular the res-

piratory gas exchange is a valuable source of infor-

mation since it allows for a non-invasive, continuous,

and precise measurement of the gross oxygen uptake

and carbon dioxide output of the whole body. Partic-

ularly in endurance sports, the metabolic rates of this

substantial fuel and the degradation product of the ex-

ercising muscles are reﬂected in that rate.

Characteristic responses to speciﬁc load proﬁles

in different intensity domains have been subject of re-

search effort in recent years (Poole and Jones, 2012;

Jones and Poole, 2005). The most distinctive parame-

ters in the description of

V O

kinetics are the highest

attainable oxygen uptake (

V O

2max

), the steady-state

level with submaximal load, and the rate of increase

V O

at the transition to a higher load level. Basi-

cally, the oxygen uptake mechanism may be viewed

as a composition of ﬁrst-order control systems, thus

responses to step-shaped load proﬁles are often de-

scribed as exponential functions that serve as a regres-

sion to measurement data.

In particular for endurance sports like cycling the

models for power demand due to mechanical resis-

tance are well understood. However, the individual

power supply model of an athlete is the bottleneck

that has hindered the design of an individual adequate

feedback control system that guides him/her to per-

form a speciﬁc task such as to ﬁnd the minimum-time

pacing in a race on a hilly track (Dahmen, 2012). For

such purposes, a dynamic model for the prediction of

gas exchange rates in response to load proﬁles given

by a particular race course would be beneﬁcial.

()stirling2008modeling provided a dynamic

model. However, it deviates signiﬁcantly from

several theoretical physiological aspects. E.g., it

does not consider separate fast and slow components

and any delays in the response to heavy and severe

work rates. Moreover, it does not provide a model

for the steady state oxygen demand as a function of

exercise load, and has not been applied to variable

load proﬁles.

We propose that the ﬁrst step towards dynamical

models for variable load should be derived from the

established models for constant work rate before more

general models are considered and can be compared

198

Gonzalez, A., Bertschinger, R., Brosda, F., Dahmen, T., Thumm, P. and Saupe, D..

Modeling Oxygen Dynamics under Variable Work Rate.

In Proceedings of the 3rd International Congress on Sport Sciences Research and Technology Support (icSPORTS 2015), pages 198-207

ISBN: 978-989-758-159-5

with the former ones. Therefore, in this contribution,

we generalize the original model equations towards

arbitrary load proﬁles and calibrate and validate them

using four load proﬁles of different characteristics.

2 PREVIOUS WORK

A detailed review and historical account of the math-

ematical modeling of the

V O

kinetics for con-

stant work rate (CWR) has recently been given by

()poole2012oxygen, containing over 800 references.

See also ()jones2005introduction and, for a clariﬁ-

cation, ()ma2010clarifying. Therefore, here we only

brieﬂy summarize the established model as far as nec-

essary for an understanding of our generalization and

refer to the above mentioned works for further expla-

nations and references to literature.

According to the commonly accepted and widely

applied model the

V O

kinetics can be separated into

four distinct components.

• Baseline Component. This constant component

accounts for the oxygen consumption at rest, i.e.,

for the time prior to the onset of exercise.

• Rapid, Initial Increase (Phase I). At the start of the

exercise a rapid but small initial increase of

V O

occurs and is completed within the ﬁrst 20 s.

• Primary, fundamental, or Fast Component (Phase

II). This phase is characterized by a (typically

larger) exponential increase of

V O

with a time

constant of 20–45 s. After saturation and for given

work rate below the lactate threshold, this compo-

nent represents the required steady-state demand

of oxygen.

• Secondary, Slow Component (Phase III). The

slow component occurs only for work rates above

the lactate threshold. It brings in an additional in-

crease of

V O

to a total that for severe work rates

above the critical power is roughly equal to the

maximal oxygen consumption,

V O

2max

Each of the components in Phases I to III are modeled

as exponential functions of type



1 −exp



−

t −T



(1)

where time is denoted by t and with different am-

plitudes A, time delays T , and time constants τ, see

Figure 1. The time constant τ determines the time

required for the dynamics of the corresponding com-

ponent to diminish the difference with the asymptotic

amplitude A by a factor of 1 −1/e ≈ 0.632. Thus,

after a time of 3τ about 95% of the amplitude A is

Figure 1: Steady-state dynamics consisting of a base-

line component

V O

2base

together with three exponentially

asymptotic functions x

(t), x

(t) (fast component), and

(t) (slow component) of different amplitudes, decay rates,

and delays.

realized and the corresponding phase is regarded as

effectively having reached its ﬁnal value. It is impor-

tant to take note that the time delay T is intended to

imply that only after that time the oxygen consump-

tion of the corresponding component is beginning. To

complete the model, we therefore apply the Heaviside

step function (Ma et al., 2010)

H(t) =



1, t ≥ 0

0, t < 0

(2)

setting

(t) = A

H(t −T

)



1 −exp



−

t −T



(3)

yielding the complete model in one formula as

V O

(t) =

V O

2base

∑

k=0

(t). (4)

Here,

V O

2base

is the baseline component, and the in-

dex k = 0,1,2 refers to the components of the three

phases, which are parametrized by their correspond-

ing amplitudes A

, time delays T

, and time con-

stants τ

. In Phase I there is no delay; T

= 0. This is

the standard form of oxygen dynamics and illustrated

in Figure 1.

Phase I typically lasts only a couple of breaths un-

til reaching its target amplitude A

and during this

short period of time at the onset of exercise there is

a large variability of the inter breath gas exchange

making it difﬁcult to ﬁt a model to an individual ven-

tilatory data series (Whipp et al., 1982). Therefore,

many researchers remove that time period from the

data and consider only the ﬁrst, fast response and

the second, slow component (Jones and Poole, 2005,

page 26). The baseline amplitude would then have

to be incremented by the amplitude A

to compensate

for the deletion of Phase I. In this study we also follow

Modeling Oxygen Dynamics under Variable Work Rate

199

this procedure. Thus, from here on,

V O

2base

refers to

the above mentioned baseline component (renamed as

V O

2min

and measured directly) plus an estimated add-

on accounting for A

In this kinetic model of

V O

consumption for con-

stant work rate the amplitudes A

must be chosen

adaptively. They depend on the physiological and

metabolic condition of the subject and on the applied

constant work rate P. Thus, A

= A

(P). As a ﬁrst ap-

proximation the amplitude of the ﬁrst, fast component

can be taken as a linear function of exercise intensity

(power), with slope of 9–11 ml/min per Watt of power

increase and bounded by

V O

2max

(Poole and Jones,

2012, page 939).

The second, slow component is more complex. It

is the sum of two parts. The ﬁrst part is an increasing

function, which seems to start having nonzero values

from about the lactate threshold up to the value of crit-

ical power P

, where the sum of all components is still

less than

V O

2max

. The exact form of this function has

not been determined. For power greater than critical

power the slow component for constant work rate ex-

ercise eventually raises the total oxygen consumption

up to

V O

2max

. Thus, for P > P

, it can be modeled

as an afﬁne linear, decreasing function which is the

difference between

V O

2max

and the sum of the ampli-

tudes of the baseline and ﬁrst, fast component.

This model has been validated with CWR and also

incremental exercise, where the slow component is

not or at least not fully apparent. In the following

section we propose a concrete parametrized model of

the total oxygen consumption following these ﬁnd-

ings, summarized in Figure 5.

3 METHODS

Experimental Setup

Five healthy, recreationally to well trained subjects

(age 37.8±14.8 yrs, height 180.4±10.1 cm, weight

75.2±7.6 kg) gave written informed consent to take

part in the study and were thoroughly informed about

the testing procedure. The subjects completed four

different cycle ergometer (Cylus2, RBM elektronik-

automation GmbH, Leipzig, Germany) tests with con-

tinuous breath-by-breath gas exchange and ventila-

tion measurements at the mouth (Ergostik, Geratherm

Respiratory GmbH, Bad Kissingen, Germany). The

tests were designed to determine a set of neces-

sary physiological parameters of aerobic capacity

(

V O

2max

, ventilatory threshold 1 (V T

) and maximal

lactate steady state (MLSS)). Furthermore the tests

featured a variety of load proﬁles in order to compre-

hensively evaluate the model prediction quality.

The following paragraphs describe the four test

protocols in detail. See Figures 2 and 3 for a visual-

ization of the corresponding work rate and road gra-

dient proﬁles.

Test 1. (see Figure 2, top)

The testing procedure commenced with an incremen-

tal step test starting at a workload of 80 W with incre-

ments of 20 W every 3 minutes. In the initial step the

subjects were instructed to choose their preferred ca-

dence between 80–100 rpm and were then instructed

to keep the cadence constant in all four test trials.The

step test was terminated at volitional exhaustion of the

subject. After test termination subjects recovered ac-

tively at 80 W and at or near their self-selected ca-

dence for ﬁve minutes. In this test, additionally to

gas exchange recordings, blood lactate measurements

(Lactate Pro 2, Arkray Factory Inc., Shiga, Japan)

were sampled to determine the MLSS. Lactate probes

from the earlobe (0.3 µl) were taken at the end of ev-

ery step, at volitional exhaustion of the subject as well

as 1, 3, and 5 minutes after test termination. Physio-

logical parameter determination is explained in detail

in the next subsection below.

Test 2. (see Figure 2, bottom)

The second ergometer test consisted of four sprints of

6 s duration each and an incremental ramp test. Two

sprints were carried out before and two after the ramp

test to obtain the subjects’ maximal power output and

V O

proﬁles in a recovered and a fatigued state. Be-

fore each set of sprints subjects pedaled at 80 W for 5

minutes at their self-selected cadence. The two sprints

of each set were separated by 30 s of passive rest and

subsequent 2 min 24 s of active recovery at 80 W. The

ergometer load for the sprints was calculated on the

basis the subjects’ body weight multiplied by a fac-

tor of 5, expressed in Newton. Ten seconds before

each sprint subjects were instructed to increase their

cadence gradually in order to obtain their maximal

pedaling frequency directly at the start of the sprint

when the load was applied to the ﬂywheel of the er-

gometer. The subjects were able to time their effort

by a countdown visually presented on a large screen.

In order to obtain approximately the same ramp

test time of 10 minutes for every subject, the end load

of the ramp protocol was estimated individually by

the highest exercise intensity reached in the incremen-

tal step test multiplied by a factor of 1.3. The start

load was set to 80 W, hence the increment per minute

was obtained by the following formula: (Individual

end load of step test in Watt − start load of 80 W) / 10

icSPORTS 2015 - International Congress on Sport Sciences Research and Technology Support

200

Time (min)

0 10 20 30 40

Power (W)

100

150

200

250

300

350

Test 1

Time (min)

0 5 10 15 20 25 30

Power (W)

200

400

600

800

1000

Test 2

Figure 2: Test protocols of the ﬁrst (top) and second (bot-

tom) cycle ergometer test (see text for details). Dashed

lines indicate individual variability in maximal power out-

put achieved during the test. The angle α indicates indi-

vidual variability in the calculated slope of the workload

increase.

minutes. Workload was increased every second until

the subject terminated the test volitionally.

Test 3. (see Figure 3, top)

In the third test subjects had to complete a variable

step protocol. The steps varied in load and duration

and alternated between low and moderate or severe

intensity. The linearly in- or decreasing intensity be-

tween the steps was also varied in time. The intensi-

ties were calculated in relation to the MLSS. In short,

the step protocol looked as follows: 4 min at 80 W,

4 min at 75% MLSS, 2 min at 40% MLSS, 2 min

at 95% MLSS, 2 min at 45% MLSS, 4 min at 85%

MLSS, 3 min at 90 W, 2 min at 100% MLSS, 5 min at

80 W, 2 min at 105% MLSS, 2 min at 70 W, 1 min at

60% MLSS, and 2 min at 80 W. Fixed workloads for

some intervals have been used because the ergome-

ter was not able to handle workloads that were be-

low 40% of MLSS for some subjects. Subsequently,

a constant load all-out exercise at 140% MLSS fol-

lowed. This interval lasted from 1.5 to 4.5 minutes

between subjects. The ﬁnal interval was designed as

a recovery ride at 80 W for 5 minutes.

Time (min)

0 10 20 30 40 50 60 70

Power (% of MLSS)

100

150

Test 3

Distance (km)

0 5 10 15

Gradient (%)

Test 4

Figure 3: Test protocols of the third (top) and fourth

(bottom) cycle ergometer test (see text for details). The

dashed line in Test 3 indicates individual variability of time

achieved at 140% of MLSS workload.

Test 4. (see Figure 3, bottom)

For the ﬁnal ”synthetic hill climb test” the ergome-

ter was controlled by our simulator software (Dahmen

et al., 2011). The load was deﬁned by a mathemati-

cal model (Martin et al., 1998) to simulate the resis-

tance on a realistic track. The gradient of that track,

depicted in Figure 3, and the subjects’ body weight

were the major determinants of the load. While hold-

ing the same cadence as before, the subjects were able

to choose their exercise intensity by gear shifting. (On

the steepest section most subjects were not able to

hold the cadence even in the lowest gear.)

Before each session the gas analyzers were cali-

brated with ambient air and a gas mixture of known

composition (15% O

, 5% CO

and N as balance).

The ﬂow sensor was calibrated by means of a 3

liter syringe. For an adequate recovery, test sessions

were separated by at least 48 hours. Subjects were

instructed to visit the laboratory in a fully recovered

state and were asked to refrain from intense physical

activity two days prior testing. In all test sessions

subjects received strong verbal encouragement from

the investigators when they were in reach of their

physical limits. During all experiments subjects

were aware of relevant mechanical variables like

Modeling Oxygen Dynamics under Variable Work Rate

201

power and cadence displayed on a large screen.

Cadence was held constant throughout all tests

except the sprints in Test 2 and the steep sections

(>10% gradient) of Test 4. To obtain a minimal

V O

(

V O

2min

) value, subjects stayed seated for 30 s on the

ergometer before the start of each test.

Physiological Parameter Determination

MLSS was determined on the basis of the incremental

step test. For clarity MLSS is used here interchange-

ably with the term critical power (P

) as it has been

shown to occur broadly at the same workload (Vautier

et al., 1995). However, this ﬁnding is not unanimous

as, e.g., ()pringle2002maximal have found that the

MLSS underestimated critical power by about 20 W

in their studies.

The MLSS is deﬁned as ”the exercise intensity

that is associated with a substantial increase in blood

lactate” (Svedahl and MacIntosh, 2003, page 300),

hence it was determined as the workload before an

increase in lactate concentration of at least 1/10th of

the maximal lactate concentration (reached at the test

abortion or in the recovery phase) could be observed.

The step before this substantial blood lactate increase

was chosen for the MLSS intensity, see Figure 4.

To set the corresponding critical power P

, we

added ±3 W to the power at MLSS, depending on the

amount of increase before and after the MLSS in or-

der to avoid having a workload in the tests that oscil-

lates around the critical power P

at which point the

steady-state model for oxygen demand has a discon-

tinuity as explained in the next subsection.

100

120

140

160

180

200

220

240

260

280

300

POST 1

POST 3

POST 5

Lactate (mmol/l)

Power (W)

Figure 4: Example for the method of determining MLSS in

the lactate curve for Subject 5.

V T

was determined visually on the basis of the

ramp protocol in Test 2. The method used is de-

scribed in detail in (Beaver et al., 1986). Brieﬂy, plots

of V E/VCO

, VE/VO

, end-tidal PCO

(PETCO

Table 1: Parameters extracted from ergometer tests.

Param. Unit Description Mean±σ

V O

2min

l/min minimal

V O

0.354±0.070

V T

W ﬁrst vent. thresh. 183±26

V O

2max

l/min maximal

V O

4.708±0.873

W critical power 260±71

end-tidal PO

(PET O

) and respiratory exchange ra-

tio (RER) vs. time were analyzed. The ﬁrst criterium

for V T

is an increase in the V E/V O

curve after it

has declined or stayed constant, without an increase in

V E/VCO

. The second criterium is a slowly rising or

constant PETCO

curve together with a beginning in

the rise of the PET O

curve after it has followed a ﬂat

or declining shape. The third criterium is a marked in-

crease in RER after following a horizontal or slowly

rising shape.

V O

2max

was determined as the highest

V O

of a

10-value moving average obtained in any of the four

tests. In 4 out of 5 subjects the test with the highest

V O

2max

was the ramp test. The other subject reached

V O

2max

in the incremental step test.

V O

2min

was determined as the lowest

V O

value

obtained during the 30 s resting phase in any of the

four ergometer tests.

The Steady-state Oxygen Demand Model

Following the model assumptions from the literature

as summarized in Section 2 we propose a steady state

oxygen demand given by a constant baseline com-

ponent, the ﬁrst, fast component, and the second,

slow component with amplitudes

V O

2base

, A

(P), and

(P), respectively. The exact form of the slow com-

ponent for loads below the critical power is not spec-

iﬁed in the literature and we propose an exponential

function, parametrized by its amplitude and growth

rate. In terms of formulas, the amplitudes are

(P) = min(s ·P,

V O

2max

−

V O

2base

) (5)

(P) =







∆

·exp(−(P

−P)/∆) P ≤ P

V O

2max

−

V O

2base

−A

(P) P > P

(6)

where s is the slope (or gain) for the fast component of

about 9–11 ml/min/W, P

denotes the critical power,

∆

is the maximal amplitude of the slow component

for exercise load up to critical power, and ∆ is the

corresponding decay constant that governs the decay

of the steady-state slow component as the load is de-

creased from the critical power. Figure 5 depicts the

graph of the sum of all components in this model.

The parameters for this steady state oxygen

demand model were determined directly from er-

icSPORTS 2015 - International Congress on Sport Sciences Research and Technology Support

202

2base

2max

slow component

fast component

∆

(l/min)

P (W)

Figure 5: Model of the steady state oxygen demand for the

baseline, fast, and slow components.

Table 2: Summary of parameters for the model of the

steady state oxygen demand. We set the value for the upper

limit of the slow component amplitude, V

∆max

V O

2max

−

V O

2base

−sP

Param. Unit Description Range

V O

2base

l/min baseline

V O

[

V O

2min

,1.0]

s l/min/W exercise economy [

V O

2max

4·P

V O

2max

]

∆

l/min ampl. slow comp. [0,V

∆max

]

∆ W range slow comp. [0, P

−V T

]

gometer tests (

V O

2max

and P

, see Table 1) or by least

squares ﬁtting to data from one or more ergometer

tests (

V O

2base

,s,V

∆

, and ∆, see Table 2). For the

ﬁtting procedure appropriate search ranges for the

parameters are also speciﬁed in Table 2. These ranges

are based on experimental evidence (

V O

2base

, ∆) or

by constraints of the model. E.g., the upper bound

V O

2max

for the slope s stems from the extreme

case where

V O

2base

= 0 and

V O

) =

V O

2max

Dynamic Model of Oxygen Consumption

Now let us extend the steady state oxygen demand

model so that it becomes dynamic allowing for a

variable load proﬁle P(t). The response of the fast

and slow component in the case of a constant work

rate demand is given by Eq. (1), A



1 −exp



−

t−T



Note that this function is the solution to the linear or-

dinary differential equation initial value problem

˙x = τ

−1

(A −x), x(0) = 0 (7)

however, delayed by the delay time T or, equivalently,

the solution for initial value x(T ) = 0. This suggests

the following equations for the ﬁrst and second com-

ponent, x

(t), x

(t),

˙x

= τ

−1

(P) −x

), x

) = 0, k = 1, 2 (8)

Table 3: Parameters for the dynamic model of oxygen con-

sumption. Together with the model for steady state oxygen

demand there are 10 parameters to be estimated.

Param. Unit Description Range

s time constant fast comp. [20,45]

s time constant slow comp. [120,240]

s time delay fast comp. [0,20]

s time delay slow comp. [60,150]

deﬁned for times t ≥ T

(and setting x

(t) = 0 for

t < T

). Here, the power demand is a function of

time P = P(t) and A

(P), k = 1, 2, are the steady state

amplitudes for the fast and slow components given in

Eqs. (5,6). The total

V O

accordingly is given by

V O

(t) =

V O

2base

+ x

(t) + x

(t). (9)

The differential equations require the four param-

eters τ

,τ

, and T

that are listed together with

their corresponding ranges in Table 3. These ranges

are set in accordance with empirical ﬁndings reported

in the survey article of ()jones2005introduction.

We remark that the above

V O

model does not

distinguish on- and off-transient dynamics, i.e.,

the

V O

demand and time constants are the same

regardless of whether the current

V O

value is below

(on-transient case) or above the

V O

demand (off-

transient case). There is, however, some evidence for

an asymmetry of dynamics in some of the exercise

intensity domains, although this has received little

attention in the literature (Poole and Jones, 2012,

p. 940). For simplicity, in this contribution we stay

with the symmetric model and leave the modeling of

asymmetric dynamics for future work.

Data Preprocessing

In order to estimate the ten parameters of the dy-

namic model given by Eqs. (5,6,8) for a particu-

lar subject, data series of time-stamped values of

produced power and resulting breath-by-breath oxy-

gen consumption are required for exercise intensities

Figure 6: Data ﬁltering for ventilatory and power data.

Modeling Oxygen Dynamics under Variable Work Rate

203

Table 4: Root-mean-square errors of model ﬁt. Parameters have been ﬁtted for each case separately. The approximation

quality is expressed by root-mean-square error (rms), maximum error (max), and signal-to-noise ratio (snr). For each subject

the best rms, max, and snr values are highlighted in bold font.

Test 1 Test 2 Test 3 Test 4 Average

rms max snr rms max snr rms max snr rms max snr rms max snr

l/min l/min dB l/min l/min dB l/min l/min dB l/min l/min dB l/min l/min dB

Subject 1 0.10 0.59 32.0 0.41 1.25 17.7 0.17 0.71 26.3 0.28 0.76 23.4 0.24 0.83 24.8

Subject 2 0.20 0.94 23.2 0.20 0.80 22.2 0.13 0.65 26.2 0.35 0.89 19.3 0.22 0.82 22.7

Subject 3 0.17 0.49 23.4 0.18 0.61 21.7 0.12 0.55 24.7 0.38 1.00 17.0 0.21 0.66 21.7

Subject 4 0.15 0.66 25.2 0.17 0.64 22.2 0.26 1.16 19.1 0.30 0.63 19.9 0.22 0.77 21.6

Subject 5 0.23 1.12 22.2 0.24 0.90 21.0 0.17 0.54 24.1 0.40 0.81 18.9 0.26 0.84 21.6

Average 0.17 0.76 25.2 0.24 0.84 21.0 0.17 0.72 24.1 0.34 0.82 19.7 0.23 0.79 22.5

Table 5: Parameters of the ﬁtted model for the subjects from Test 3.

Parameter

V O

2max

V O

2base

s P

∆

∆ T

Unit l/min l/min l/min/W W l/min W min min min min

Subject 1 6.09 0.94 10.6×10

−3

383 0.33 98 0:04.9 0:32.2 2:38.5 3:34.4

Subject 2 4.63 0.83 10.5×10

−3

243 0.50 24 0:04.1 0:29.8 2:41.7 3:17.4

Subject 3 3.85 0.45 10.9×10

−3

203 0.24 24 0:06.6 0:29.0 2:42.2 3:42.2

Subject 4 4.10 0.96 8.1×10

−3

217 1.05 13 0:02.2 0:21.2 1:04.6 3:50.7

Subject 5 4.87 0.89 9.7×10

−3

257 0.71 56 0:06.6 0:28.2 1:16.8 3:24.7

Figure 7: Fitted models of steady state oxygen demand for

the ﬁve subjects.

ranging from moderate to severe. These time series

from ergometer laboratory experiments are typically

very noisy, have different sampling rates for

V O

and

power, and samples may be irregularly spaced.

Therefore, a combined smoothing and resam-

pling operator has to be applied before param-

eter estimation. In this study we have used

the standard Gaussian smoothing ﬁlter with kernel

(σ

√

2π)

−1

exp(−0.5t/σ

) and σ = 20 s and 3 s for

respiratory and power measurements, respectively.

The ﬁlter was applied at time instants uniformly

spaced at 1 s intervals. Figure 6 gives an example of

measured time series and their smoothed and resam-

pled version.

Parameter Estimation

Parameter estimation was done by least squares

ﬁtting, minimizing mean squared error between com-

puted model values and the (smoothed)

V O

data.

Testing has shown that ﬁtting versus the smoothed

V O

data produced better results than against noisy

measured data. Non-linear least squares ﬁtting may

suffer from the presence of many local minima. Thus,

commonly applied optimization algorithms like the

downhill simplex method typically get stuck in these

and results largely depend on the choice of initial

parameters. Thus, we adopted a genetic algorithm

(from MATLAB



) which provided better minima by

use of its stochastic elements.

Model Validation

We calculated model parameters for each ergometer

test of each participating subject. To express the qual-

ity of the model ﬁt to the data we computed the root-

mean-square differences. To validate the predictive

power of the model we selected Test 3 for each sub-

ject for parameter ﬁtting, and used the other tests for

comparing the model predictions of oxygen consump-

tion with the measured values.

4 RESULTS

The

V O

model as given by Eqs. (5,6,8,9) was ﬁtted

to the data for all four tests and ﬁve subjects, result-

ing in 20 sets of model parameters. The correspond-

ing model errors were calculated by solving the initial

value problems (8) and summing up the components

icSPORTS 2015 - International Congress on Sport Sciences Research and Technology Support

204

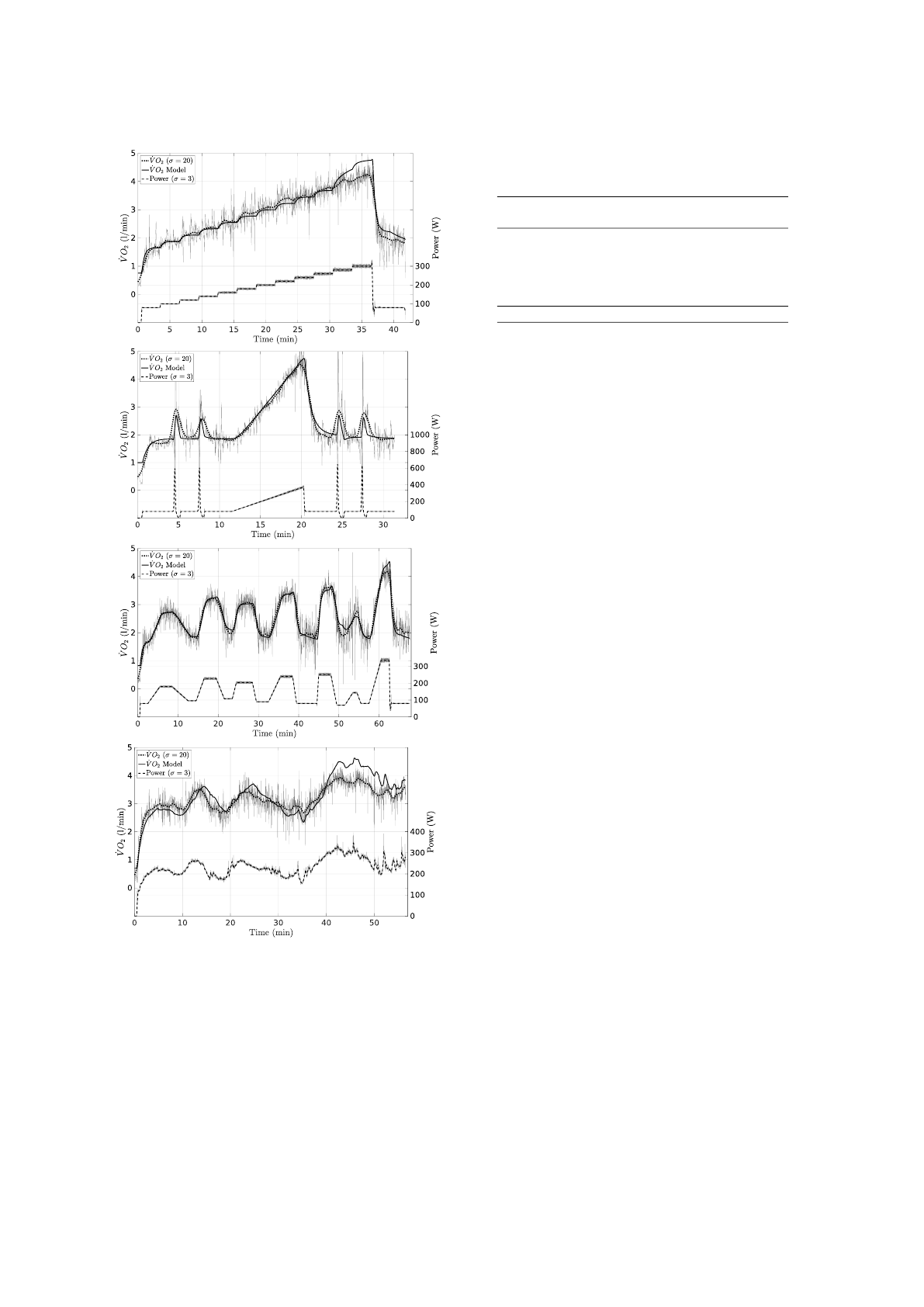
Figure 8:

V O

ﬁtting for Subject 2 on all four tests. The

lower curves show the power, and the upper two parts depict

the recorded and the best ﬁtting

V O

curves. The noisy grey

signals are the original (unﬁltered) measurements.

according to Eq. (9). The resulting model errors are

given in Table 4.

Overall, the average

V O

modeling rms error was

0.23 ±0.08 l/min. Figure 8 illustrates the

V O

and

power data, and the ﬁtting result for Subject 2, whose

Table 6: Root-mean-square errors of model predictions for

the three tests in the validation set.

Test 1 Test 2 Test 4 Average

l/min l/min l/min l/min

Subject 1 0.19 0.59 0.44 0.41

Subject 2 0.26 0.30 0.50 0.35

Subject 3 0.20 0.33 0.43 0.32

Subject 4 0.25 0.27 0.40 0.31

Subject 5 0.30 0.36 0.78 0.48

Average 0.24 0.37 0.51 0.37

average rms error is the median of errors for subjects.

In the modeling phase, we ﬁnd that Test 3 per-

formed best, with the smallest average

V O

rms error

of 0.17 l/min. The corresponding parameter sets for

each of the subjects are given in Table 5 and visual-

ized in Figure 7.

In the validation we used the parameters resulting

from the model ﬁtting using Test 3 for the prediction

of the

V O

consumption in the other tests. For the

model simulation we used the measured power as in-

put for the differential equations. We recorded the

corresponding rms prediction errors in Table 6.

Figures 10 and 11 visualize the prediction of the

V O

model. We present the graphs of the

V O

pre-

diction and the measured

V O

against time for the

incremental Test 1 and Test 4, where subjects were

self-pacing themselves. Note, that the model predic-

tion errors are mostly positive, i.e., the model over-

estimated the actual

V O

consumption, especially at

times of severe exercise intensity.

5 DISCUSSION

Overall, the results show that in principle the ap-

proach to transfer the dynamic steady-state model

from constant work rates to variable work rates was

successful. Parameters could be estimated such that

measured

V O

data could be approximated with a

small rms error of about 0.23 l/min. For model pre-

diction the average error was around 0.37 l/min. The

results are even better for work rates in the moderate

to heavy exercise intensity domain, as can be seen in

Figures 8 and 10.

To illustrate and discuss the dynamics of the dif-

ferential equation model for the oxygen dynamics

we provide Figure 9 that visualizes the total oxygen

consumption and the corresponding slow component,

both with respect to the oxygen demand,

V O

2base

(P(t)) + A

(P(t)) and A

(P(t)), respectively, and

the modeled responses of the system,

V O

(t),x

(t).

Moreover, the corresponding oxygen consumption

measurements as well as the applied power P(t) is

Modeling Oxygen Dynamics under Variable Work Rate

205

Figure 9: Steady-state demand and dynamic model for Sub-

ject 2 on Test 3. See text for details.

included. The exponential asymptotic dynamics for

piecewise constant demands are clearly visible. Also

note the delayed reaction, especially of the slow com-

ponent. A large oxygen demand in the slow compo-

nent may trigger a delayed overcompensation, lead-

ing total

V O

estimates that are to too large, e.g., near

t = 50 min.

Therefore, for severe work rates it seems that the

model overestimates the slow component, leading to

excess

V O

contributions. This can be clearly seen

in Figure 8 (bottom graph) at about 40 to 50 min-

utes in the test. During the whole 10 minutes the

modeled

V O

demand was above the critical power

(243 W). There, the slow component drove the mod-

eled

V O

towards

V O

2max

, while the measured

V O

stayed about half a liter per minute below that.

A possible explanation of this artifact is that the

estimation of the critical power by MLSS may have

been too small due to an imprecise empirical MLSS

estimate, or because in our cases the critical power

was signiﬁcantly above the MLSS, as in the exper-

iments of ()pringle2002maximal, already mentioned

before. Another possibility is that the slow com-

ponent generally is not well understood yet, thereby

having led to an inadequate steady state model upon

which our model is based. This limits the dynamic

Figure 10:

V O

model prediction for Test 4. The curves are

given for Subjects 1 to 5 from bottom to top, respectively.

Figure 11:

V O

model prediction for Test 1 for all ﬁve sub-

jects (from Subject 1 at the bottom to Subject 5 at the top),

based on parameters gained from parameter estimation on

Test 3.

phenomena that can be captured to those that are

known and were described in the literature. More-

over, some ﬁndings or assumptions about the type of

functional dependencies are controversial, in partic-

ular regarding the slow component, see (Poole and

Jones, 2012, page 953). For example, it is not clear

at all, that the slow component in constant work rate

exercise tests at heavy and severe work rates is asymp-

totically exponential as expressed in Eq. (1) (Gaesser

and Poole, 1996, pages 43, 44).

Therefore, instead of enforcing the slow compo-

nent one might conjecture that a dynamic model that

discards the slow component would yield better pre-

diction results. In order to check this in a quick test,

we zeroed the slow component in the parameter ﬁt-

ting procedure. The results for prediction of Test 4

are shown in Figure 12, compare with Figure 10. We

see that the results indeed look better than with the

slow component for most of the model predictions.

However, for the other validation tests the predictions

using the slow component are better. Still, this in-

dicates that there is a good opportunity for improve-

ments of the mathematical model beyond the direct

transfer from constant to variable work rates.

Figure 12:

V O

model prediction for Test 4 as in Figure 10.

Here, the model was trained without the slow component.

icSPORTS 2015 - International Congress on Sport Sciences Research and Technology Support

206

6 CONCLUSIONS AND FUTURE

WORK

We contributed to the generalization of the commonly

accepted model of the dynamics of oxygen uptake

during exercise at constant work rates to variable load

protocols by means of differential equations. We

showed how parameters in the model can be esti-

mated and that the mathematical dynamical model

can be used to predict the oxygen consumption for

other given load proﬁles. We found for ﬁve subjects

and four very different test protocols (of up to about

one hour length) that on average the modeling rms er-

ror of

V O

was 0.23 ±0.08 l/min and the prediction

rms error in three tests was 0.37 ±0.16 l/min.

The model overestimated the slow component,

however. Therefore, we plan to let the critical power

be a parameter that can be ﬁtted to training data in-

stead of using the MLSS as an estimate. For such a

study the MLSS can serve as a lower limit of the al-

lowable range of values for critical power.

Moreover, a closer study of the slow component

in a set of constant work rate (CWR) tests should be

carried out by our ﬁve subjects. From such lab tests

one can also compare the modeling error for the vari-

able work load exercises undertaken for this contribu-

tion with those for CWR tests in order to gain an un-

derstanding of how much of the modeling precision

of CWR carries over to the variable work load case

when using the direct generalization of the mathemat-

ical modeling as given in this paper.

An alternative approach to modeling would be

to allow for different, more appropriate degrees of

freedom in the mathematical model, again ﬁtting the

model type and parameters to empirical data, and

calculating model and prediction errors. For exam-

ple, we may assume as above two additive model

components (besides a constant baseline oxygen con-

sumption) with different delay times and decay rates,

however, with corresponding steady state oxygen de-

mands that are restricted only by requiring (piece-

wise) monotonicity and that can be parametrized by

a set of six parameters, the same number as in this

contribution.

With such an approach, we expect a better data

ﬁtting. It remains open whether also the predic-

tive power is better than for our current model and

whether the results can be interpreted in harmony

with the current understanding of sport physiology

and sport medicine.

REFERENCES

Beaver, W. L., Wasserman, K., and Whipp, B. J. (1986). A

new method for detecting anaerobic threshold by gas

exchange. Journal of applied physiology, 60(6):2020–

2027.

Dahmen, T. (2012). Optimization of pacing strategies

for cycling time trials using a smooth 6-parameter

endurance model. In Proceedings Pre-Olympic

Congress on Sports Science and Computer Science in

Sport (IACSS), Liverpool, England, UK, July 24-25,

2012.

Dahmen, T., Byshko, R., Saupe, D., R

oder, M., and

Mantler, S. (2011). Validation of a model and a simu-

lator for road cycling on real tracks. Sports Engineer-

ing, 14(2-4):95–110.

Gaesser, G. A. and Poole, D. C. (1996). The slow compo-

nent of oxygen uptake kinetics in humans. Exercise

and sport sciences reviews, 24(1):35–70.

Jones, A. M. and Poole, D. C. (2005). Introduction to oxy-

gen uptake kinetics and historical development of the

discipline. In Jones, A. M. and Poole, D. C., edi-

tors, Oxygen Uptake Kinetics in Sport, Exercise and

Medicine, pages 3–35. London: Routledge.

Ma, S., Rossiter, H. B., Barstow, T. J., Casaburi, R., and

Porszasz, J. (2010). Clarifying the equation for model-

ing of

V O

kinetics above the lactate threshold. Jour-

nal of Applied Physiology, 109(4):1283–1284.

Martin, J. C., Milliken, D. L., Cobb, J. E., McFadden, K. L.,

and Coggan, A. R. (1998). Validation of a mathemat-

ical model for road cycling power. Journal of Applied

Biomechanics, 14:276–291.

Poole, D. C. and Jones, A. M. (2012). Oxygen uptake ki-

netics. Comprehensive Physiology, 2:933–996.

Pringle, J. S. and Jones, A. M. (2002). Maximal lactate

steady state, critical power and emg during cycling.

European journal of applied physiology, 88(3):214–

226.

Stirling, J., Zakynthinaki, M., and Billat, V. (2008). Model-

ing and analysis of the effect of training on

V O

kinet-

ics and anaerobic capacity. Bulletin of Mathematical

Biology, 70(5):1348–1370.

Svedahl, K. and MacIntosh, B. R. (2003). Anaerobic thresh-

old: the concept and methods of measurement. Cana-

dian Journal of Applied Physiology, 28(2):299–323.

Vautier, J., Vandewalle, H., Arabi, H., and Monod, H.

(1995). Critical power as an endurance index. Applied

ergonomics, 26(2):117–121.

Whipp, B. J., Ward, S. A., Lamarra, N., Davis, J. A., and

Wasserman, K. (1982). Parameters of ventilatory and

gas exchange dynamics during exercise. Journal of

Applied Physiology, 52(6):1506–1513.

Modeling Oxygen Dynamics under Variable Work Rate

207