Effect of Dispersive Reflectivity on the Stability of Gap Solitons in
Systems with Separated Bragg Grating and Nonlinearity
Tanvir Ahmed and Javid Atai
School of Electrical and Information Engineering, The University of Sydney, NSW 2006, Sydney, Australia
Keywords:
Gap Soliton, Fiber Bragg Grating, Dispersive Reflectivity.
Abstract:
The existence and stability of quiescent solitons in a dual core optical medium, where the one core has only
Kerr nonlinearity while the other has Bragg grating and dispersive reflectivity are investigated. Three spectral
gaps are identified in the systems linear spectrum, in which both lower and upper band gaps overlap with one
branch of the continuous spectrum for all values of normalized group velocity c in the linear core; and, the
central band gap remains a genuine bandgap. Soliton solutions exist only in the lower and upper gaps. In the
absence of dispersive reflectivity, stable solitons are only found in the upper bandgap. However, introduction of
dispersive reflectivity significantly alters the stability characteristics of solitons and results in the stabilization
of solitons in a portion of the lower bandgap.
1 INTRODUCTION
One of the main characteristics of fiber Bragg grat-
ings (FBGs) is that cross-coupling between forward
and backward propagating waves leads to a strong ef-
fective dispersion which may be 6 orders of magni-
tude greater than the dispersion of silica (de Sterke
and Sipe, 1994; Eggleton et al., 1997). At suffi-
ciently high intensities this effective dispersion can
be balanced by nonlinearity giving rise to the for-
mation of gap solitons (de Sterke and Sipe, 1994).
Over the past two decades, gap solitons have re-
ceived much interest and have been studied exten-
sively both theoretically (Aceves and Wabnitz, 1989;
Christodoulides and Joseph, 1989; Malomed and
Tasgal, 1994; Barashenkov et al., 1998; De Rossi
et al., 1998; Neill and Atai, 2006) and experimentally
(Eggleton et al., 1996; de Sterke et al., 1997; Eggleton
et al., 1999).
One of the main features of gap solitons is that
they can have any velocity between zero and the
speed of light in the medium. Zero velocity or very
slow solitons may be used as optical switches, opti-
cal memory or buffer elements (Krauss, 2008). As a
result, much of the experimental activity in this area
has focused on the generation of zero velocity (quies-
cent) solitons. Thus far, gap solitons with a velocity
of 23% of the speed of light in the medium have been
observed (Mok et al., 2006).
Gap solitons have been studied in a variety of
structures such as planar photonic crystal waveguides
(Monat et al., 2010), dual core fibers (Atai and Mal-
omed, 2000; Atai and Malomed, 2001; Mak et al.,
1998; Atai and Baratali, 2012), waveguide arrays
(Mandelik et al., 2004; Tan et al., 2009; Dong et al.,
2011), and nonuniform Bragg gratings with disper-
sive reflectivity (Atai and Malomed, 2005; Neill et al.,
2008). Since dual core fibers made of dissimilar cores
can exhibit better performance and switching charac-
teristics than the ones with identical cores (Atai and
Chen, 1992; Atai and Chen, 1993; Bertolotti et al.,
1995; Nistazakis et al., 2002), one may anticipate rich
dynamics and interesting applications if such couplers
are also equipped with Bragg gratings. Moreover, in
the case of Bragg gratings with dispersive reflectiv-
ity, it has been demonstrated that the presence of dis-
persive reflectivity has a stabilizing effect (Atai and
Malomed, 2005).
The objective of this work is to analyze the exis-
tence and stability of gap solitons in a dual core op-
tical system where one core is linear and contains a
nonuniform Bragg grating with dispersive reflectivity
and the other core has only Kerr nonlinearity.
2 THE MODEL
Starting with the model of (Atai and Malomed, 2001)
and following a similar procedure as described in
(Atai and Malomed, 2005), one can derive the fol-
Ahmed, T. and Atai, J.
Effect of Dispersive Reflectivity on the Stability of Gap Solitons in Systems with Separated Bragg Grating and Nonlinearity.
DOI: 10.5220/0005651602310235
In Proceedings of the 4th International Conference on Photonics, Optics and Laser Technology (PHOTOPTICS 2016), pages 233-237
ISBN: 978-989-758-174-8
Copyright
c
 2016 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved
233

-10
-5
0
5
10
k
-9
-6
-3
0
3
6
9
ω
(a)
-10
-5
0
5
10
k
-9
-6
-3
0
3
6
9
ω
(b)
Figure 1: Typical examples of the linear spectrum for (a)
λ = 0.5, c = 0.0 and m = 0.5 and (b) λ = 0.5, c = 4.0 and
m = 0.5.
lowing normalized model which describes the prop-
agation of light in a linearly coupled dual core sys-
tem with one core having Kerr nonlinearity and the
other one being linear and equipped with Bragg grat-
ing with dispersive reflectivity:
iu
t
+ iu
x
+

|v|
2
+
1
2
|u|
2

u+ φ = 0,
iv
t
− iv
x
+

|u|
2
+
1
2
|v|
2

v+ ψ = 0,
iφ
t
+ icφ
x
+ u + λψ+ mψ
xx
= 0
,
iψ
t
−icψ
x
+ v + λφ+ mφ
xx
= 0,
(1)
where the forward- and backward- propagating waves
are u and v in the nonlinear core, φ and ψ in the grat-
ing aided core, function evolution time is t and x is the
transverse coordinate, the coefficient of linear cou-
pling between the cores is normalized to be 1, Bragg
grating induced linear coupling coefficient is repre-
sented as λ > 0 between the left- and right- propagat-
ing waves. The group velocity in the nonlinear core is
-80 -40 0 40 80
x
0
0.1
0.2
0.3
0.4
0.5
|u|
ω =1.43
ω =1.47
(a)
-80 -40 0 40 80
x
-0.05
0
0.05
0.1
0.15
0.2
Re(u)
Im(u)
|u|
(b)
Figure 2: Examples of typical quiescent soliton solutions
in upper gap with different parameter values (a) λ = 0.8,
c = 0.0 and m= 0.1 (b) sidelobes formation with its real and
imaginary parts for λ = 0.8, c = 0.1, ω = 1.47 and m = 0.5.
set equal to 1 and c represents the relative group ve-
locity in the linear core. Real parameter m > 0 is the
dispersive reflectivity strength. Our analysis is lim-
ited to 0 < m < 0.5 as there is no practical importance
for m > 0.5 (Atai and Malomed, 2005).
Spectrum analysis is an important feature in or-
der to find the existence of the gap soliton in the
spectral gap (de Sterke and Sipe, 1994). Substituting
u, v, φ,ψ ∼ exp(ikx− iωt) into Eqs. (1) and lineariz-
ing we arrive at the following dispersion relation:
ω
4
−

2+

λ− mk
2

2
+

1+ c
2

k
2

ω
2
+

λk− mk
3

2
+

ck
2
− 1

2
= 0. (2)
The dispersion relation gives rise to a central gen-
uine gap and two gaps (one in the upper half and one
in the lower half of the spectrum). The upper and
lower gaps overlap with one branch of the continu-
ous spectrum and therefore are not genuine gaps (Atai
PHOTOPTICS 2016 - 4th International Conference on Photonics, Optics and Laser Technology
234

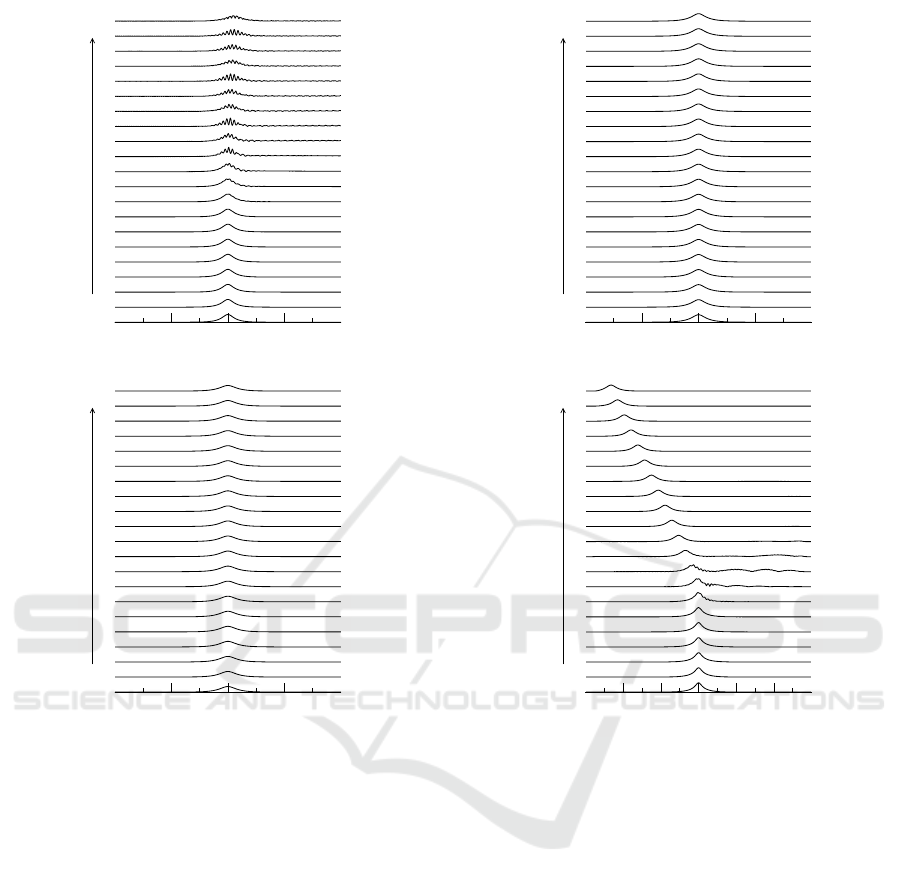
-100
-50
0
50
100
x
t
2000
0
(a)
-100
-50
0
50
100
x
t
2000
0
(b)
Figure 3: Typical examples of the stationary solitons evolu-
tion in the upper band gap (a) Unstable soliton for λ = 0.2,
c = 0.2, ω = 1.02 and m = 0.1 and (b) stable soliton for
λ = 0.2, c = 0.2, ω = 1.06 and m = 0.1. Only u compo-
nents shown here.
and Malomed, 2001). For fixed values of c and λ, the
width of the central gap varies with m. Typical exam-
ples of the bandgap structures are shown in Fig. 1.
3 STABILITY ANALYSIS
The soliton solutions are sought numerically by
means of relaxation algorithm as there are no exact
analytical solutions for m 6= 0. Stationary soliton so-
lutions are found both in the upper and lower spectral
gaps. No stationary solutions are found in the cen-
tral band gap. With higher values of λ sidelobes are
formed in the stationary soliton both in the upper and
lower band gaps over a certain value of dispersive re-
flectivity. Typical soliton solutions with and without
-100
-50
0
50
100
x
t
2000
0
(a)
-150
-100
-50
0
50
100
150
x
t
2000
0
(b)
Figure 4: Typical examples of the stationary solitons evo-
lution in the lower band gap (a) stable soliton for λ = 0.2,
c = 0.5, ω = −1.01 and m = 0.1 and (b) unstable soliton for
λ = 0.2, c = 0.5, ω = −1.07 and m = 0.1. Only u compo-
nents shown here.
sidelobes are shown in Fig. 2.
We have investigated the stability of the stationary
soliton solutions by means of systematic numerical
simulations. Figs. 3 and 4 display examples of the
evolution of stable and unstable solitons in the upper
and lower bandgaps for various values of c, λ, m and
ω. As is shown in Figs. 3(a) and 4(b) unstable soli-
tons shed some energy in the form of radiation and
subsequently they are either destroyed or evolve to a
moving soliton. This demonstrates that stable moving
solitons exist in this model.
Fig. 5 summarizes the outcomes of the stability
analysis for λ = 0.2 and m = 0.2 in the (c, ω) plane.
A noteworthy feature of the stability diagram is that
there are large regions within the upper and lower
bandgaps where solitons are stable. Additionally, it
is found that in both lower and upper bandgaps, when
Effect of Dispersive Reflectivity on the Stability of Gap Solitons in Systems with Separated Bragg Grating and Nonlinearity
235

0.905
0.945
0.99
1.035
1.08
1.105
0
0.5
1
1.5
2
2.5
3
c
-1.105
-1.08
-1.035
-0.99
-0.945
-0.905
No Soliton Solutions
Unstable
Unstable
Stable
Stable
ω
Figure 5: Stability diagram for λ = 0.2, m= 0.2 in the (c, ω)
plane.
c > 1, the stable region shrinks as c increases. On the
other hand, in the range 0.2 ≤ c < 1, increasing c re-
sults in the expansion of the stable region in the lower
bandgap.
4 CONCLUSIONS
Bragg grating solitons are investigated numerically
in a systematic way in a dual core coupled nonlin-
ear medium where one core is nonlinear that contains
Kerr nonlinearity and another core is linear and has
a Bragg grating with dispersive reflectivity. The lin-
ear spectrum of the system has three spectral gaps:
a genuine central gap and an upper and a lower gap
each overlapping with one branch of the continuous
spectrum for all values of c. Stationary soliton so-
lutions are found only in the lower and upper band
gaps. Above a certain value of dispersive reflectivity
parameter m, solitons develop sidelobes. Sidelobes
are dominant in the the soliton profile for lower val-
ues of c but for higher values of c no sidelobes are
generated.
As for the stability of solitons, unlike the model
without dispersive reflectivity, stable solitons are
found in both upper and lower gaps. We have also
identified nontrivial stability borders in the plane of
(c, ω).
REFERENCES
Aceves, A. B. and Wabnitz, S. (1989). Self-induced trans-
parency solitons in nonlinear refractive periodic me-
dia. Phys. Lett. A, 141:37–42.
Atai, J. and Baratali, B. H. (2012). Gap solitons in dual-core
bragg gratings with dispersive reflectivity. J. Opt.,
14:065202.
Atai, J. and Chen, Y. (1992). Nonlinear couplers composed
of different nonlinear cores. J. Appl. Phys., 72:24–27.
Atai, J. and Chen, Y. (1993). Nonlinear mismatches be-
tween two cores of saturable nonlinear couplers. IEEE
J. Quant. Elec., 29:242–249.
Atai, J. and Malomed, B. A. (2000). Bragg-grating soli-
tons in a semilinear dual-core system. Phys. Rev. E,
62:8713–8718.
Atai, J. and Malomed, B. A. (2001). Solitary waves in sys-
tems with separated bragg grating and nonlinearity.
Phys. Rev. E, 64:066617.
Atai, J. and Malomed, B. A. (2005). Gap solitons in bragg
gratings with dispersive reflectivity. Phys. Lett. A,
342:404–412.
Barashenkov, I. V., Pelinovsky, D. E., and Zemlyanaya,
E. V. (1998). Vibrations and oscillatory instabilities
of gap solitons. Phys. Rev. Lett., 80:5117–5120.
Bertolotti, M., Monaco, M., and Sibilia, C. (1995). Role of
the asymmetry in a third-order nonlinear directional
coupler. Opt. Comm., 116:405–410.
Christodoulides, D. N. and Joseph, R. I. (1989). Slow bragg
solitons in nonlinear periodic structures. Phys. Rev.
Lett., 62:1746–1749.
De Rossi, A., Conti, C., and Trillo, S. (1998). Stability,
multistability, and wobbling of optical gap solitons.
Phys. Rev. Lett., 81:85–88.
de Sterke, C. M., Eggleton, B. J., and Krug, P. A. (1997).
High-intensity pulse propagation in uniform gratings
and grating superstructures. IEEE J. Lightwave Tech-
nol., 15:1494–1502.
de Sterke, C. M. and Sipe, J. E. (1994). Gap solitons. Prog.
Optics, 33:203–260.
Dong, R., R¨uter, C. E., Kip, D., Cuevas, J., Kevrekidis,
P. G., Song, D., and Xu, J. (2011). Dark-bright gap
solitons in coupled-mode one-dimensional saturable
waveguide arrays. Phys. Rev. A, 83:063816.
Eggleton, B. J., de Sterke, C. M., and Slusher, R. E. (1997).
Nonlinear pulse propagation in Bragg gratings. J. Opt.
Soc. Am. B, 14:2980–2993.
Eggleton, B. J., de Sterke, C. M., and Slusher, R. E. (1999).
Bragg solitons in the nonlinear schr¨odinger limit: ex-
periment and theory. J. Opt. Soc. Am. B, 16:587–599.
Eggleton, B. J., Slusher, R. E., Krug, P. A., and Sipe, J. E.
(1996). Bragg grating solitons. Phys. Rev. Lett.,
76:1627–1630.
Krauss, T. F. (2008). Why do we need slow light? Nature
Photon., 2:448–450.
Mak, W. C. K., Malomed, B. A., and Chu, P. L. (1998).
Solitary waves in coupled nonlinear waveguides with
Bragg gratings. J. Opt. Soc. Am. B, 15:1685–1692.
Malomed, B. A. and Tasgal, R. S. (1994). Vibration modes
of a gap soliton in a nonlinear optical medium. Phys.
Rev. E, 49:5787–5796.
Mandelik, D., Morandotti, R., Aitchison, J. S., and Silber-
berg, Y. (2004). Gap solitons in waveguide arrays.
Phys. Rev. Lett., 92:093904.
PHOTOPTICS 2016 - 4th International Conference on Photonics, Optics and Laser Technology
236

Mok, J. T., de Sterke, C. M., Littler, I. C. M., and Eggle-
ton, B. J. (2006). Dispersionless slow light using gap
solitons. Nature Phys., 2:775–780.
Monat, C., de Sterke, M., and Eggleton, B. J. (2010). Slow
light enhanced nonlinear optics in periodic structures.
J. Opt., 12:104003.
Neill, D. R. and Atai, J. (2006). Collision dynamics of gap
solitons in kerr media. Phys. Lett. A, 353:416–421.
Neill, D. R., Atai, J., and Malomed, B. A. (2008). Dynam-
ics and collisions of moving solitons in bragg gratings
with dispersive reflectivity. J. Opt. A: Pure Appl. Opt.,
10:085105.
Nistazakis, H. E., Frantzeskakis, D. J., Atai, J., Malomed,
B. A., Efremidis, N., and Hizanidis, K. (2002). Mul-
tichannel pulse dynamics in a stabilized ginzburg-
landau system. Phys. Rev. E, 65:036605.
Tan, Y., Chen, F., Beliˇcev, P. P., Stepi´c, M., Maluckov, A.,
R¨uter, C. E., and Kip, D. (2009). Gap solitons in de-
focusing lithium niobate binary waveguide arrays fab-
ricated by proton implantation and selective light illu-
mination. Appl. Phys. B, 95:531–535.
Effect of Dispersive Reflectivity on the Stability of Gap Solitons in Systems with Separated Bragg Grating and Nonlinearity
237