Monocular Depth Ordering using Perceptual Occlusion Cues

Babak Rezaeirowshan, Coloma Ballester and Gloria Haro

Department of Information and Communication Technologies, Universitat Pompeu Fabra, Barcelona, Spain

Keywords:

Monocular Depth, Ordinal Depth, Depth Layering, Occlusion Reasoning, Convexity, T-junctions, Boundary

Ownership, 2.1D.

Abstract:

In this paper we propose a method to estimate a global depth order between the objects of a scene using

information from a single image coming from an uncalibrated camera. The method we present stems from

early vision cues such as occlusion and convexity and uses them to infer both a local and a global depth

order. Monocular occlusion cues, namely, T-junctions and convexities, contain information suggesting a local

depth order between neighbouring objects. A combination of these cues is more suitable, because, while

information conveyed by T-junctions is perceptually stronger, they are not as prevalent as convexity cues in

natural images. We propose a novel convexity detector that also establishes a local depth order. The partial

order is extracted in T-junctions by using a curvature-based multi-scale feature.Finally, a global depth order,

i.e., a full order of all shapes that is as consistent as possible with the computed partial orders that can tolerate

conﬂicting partial orders is computed. An integration scheme based on a Markov chain approximation of the

rank aggregation problem is used for this purpose. The experiments conducted show that the proposed method

compares favorably with the state of the art.

1 INTRODUCTION

Depth perception in humans enables a robust 3D vi-

sion even in the presence of a single view stimulus.

Such a system is desirable in computer vision mainly

due to its many applications and the abundance of

monocular cameras. Human vision harnesses monoc-

ular cues to resolve inherent ambiguity caused by 3D

to 2D projection in the image formation process and

creates a sensible 3D perception. Monocular depth

perception cues consist of dynamic cues and static

cues. Dynamic cues, such as motion occlusion and

motion parallax require multiple frames and motions

in the scene as stimuli which are out of scope of this

work. In this proposal, the focus is on static cues,

namely, convexity and T-junctions; other cues in this

category are perspective, relative dimensions, lighting

and shadow.

While physiological aspects of these cues have

been widely studied in the literature of psychophysics

and vision, there is only a handful of research works

that test these theories in a practical scenario using

computer vision methods. Most of the work related to

depth estimation in computer vision focuses on stereo

disparity or motion parallax, both of which use tri-

angulation to compute depth. While triangulation-

based methods provide absolute depth, which is de-

sirable in many applications, they require two or more

views. Monocular static cues on the other hand, can

be combined to create a depth perception in the ab-

sence of binocular and dynamic monocular cues or as

a complement to improve existing depth perception in

a much wider domain.

The goal of this paper is, given a single image

from an uncalibrated camera and its decomposition

in shapes (that are assumed to represent the projec-

tion of the 3D objects on the image plane; e.g., a seg-

mentation), to create a globally consistent depth order

of these shapes that constitute the image scene. For

this purpose, occlusion cues between objects, namely

T-junctions and convexities are used. Following the

underlying assumption for extracting depth from oc-

clusion cues, we assume that the image is composed

of objects that are fronto-parallel to the camera. This

is also referred to as the dead leaves model, a term

coined by Matheron (Matheron, 1968), which consti-

tutes a model for image formation where the image is

made by objects falling on top of each others and par-

tially occluding them. The reason for making such as-

sumption is that in the presence of non fronto-parallel

objects in the image, e.g. ﬂoor, occlusion does not

translate to depth order (see Figure 1).

Given an image that satisﬁes the dead leaves

model, the occlusion cues provide a depth order

Rezaeirowshan, B., Ballester, C. and Haro, G.

Monocular Depth Ordering using Perceptual Occlusion Cues.

DOI: 10.5220/0005726404310441

In Proceedings of the 11th Joint Conference on Computer Vision, Imaging and Computer Graphics Theory and Applications (VISIGRAPP 2016) - Volume 4: VISAPP, pages 431-441

ISBN: 978-989-758-175-5

431

Figure 1: Dead leaves model (DLM) and correctness of

convexity cues. The left image follows the DLM while the

right one doesn’t. Arrows indicate the occluding object sug-

gested by convexity cues. Bright arrows indicate a correct

depth order while dark arrows indicate a wrong one.

among neighbouring regions. However, we require

a global order to establish a rough 3D model of the

scene, which is understood here as obtaining a con-

sistent global order from a number of partial orders,

which may contain some discrepancies. This problem

is in general referred to as rank aggregation and it has

been dealt with in several ﬁelds of computer science

(Dwork et al., 2001; Basha et al., 2012). This ordering

problem appears whenever there are multiple opera-

tors providing partial orders with transitive relations.

The goal is to use the transitivity to obtain a global

robust order as consistent as possible with the partial

orders. Transitivity between orders can be stated as

the following property: if we have a partial order in-

dicating A<B and another one indicating B<C, thus

we can infer the global order A<B<C. Our approach

stems from the fact that transitivity of local orders can

be utilized to obtain a global order using rank aggre-

gation.

Our main contributions in this paper are (i) a depth

ordering system based on monocular perceptual cues

that allows reasoning without need for camera cali-

bration, multiple frames, or motion, (ii) a novel gen-

eral convexity cue detector that assigns a local depth

order based on convexity and which is based on the

convex hull of a shape, and (iii) the extraction of a

global depth order by a robust integration of the par-

tial orders.

2 RELATED WORK

3D modeling has received a signiﬁcant attention from

the computer vision community, with studies focus-

ing on various aspects of 3D perception. Due to the

vastness of the literature in this ﬁeld, we will focus

on studies conducted on monocular static cues. Com-

putational methods for depth extraction from a sin-

gle image can be categorized into supervised methods

and Gestalt-based methods. Supervised methods ei-

ther learn depth directly from training images (Saxena

et al., 2008; Eigen et al., 2014), or alternatively learn

and detect cues (Chen et al., 2013; Jia et al., 2012),

geometric (Hoiem et al., 2011) or semantic (Liu et al.,

2010) information and use them to infer an abso-

lute depth. Aforementioned approaches are aimed

at a speciﬁc type of scene (either outdoor or indoor

scenes) and require additional training or parameter

tuning to achieve high performance on the unfamil-

iar scenes. Alternatively, other approaches have been

suggested in the literature that use human perception

and vision as the basis from which to attempt to infer a

computational model simulating the known processes

of human vision. Our work falls in the latter category.

Thus, we focus on the use of T-junctions and convex-

ity cues for establishing a depth order. The role of T-

junctions as a cue for recovering surface occlusion ge-

ometry was introduced by (Guzm

an, 1968), and later

stressed by (Malik, 1987; Rubin, 2001). Moreover,

through the Gestalt school of thought in psychology,

T-junctions were described as a basis of monocular

depth perception by the work of Kanizsa (Kanizsa,

1979). Later on, more computational works demon-

strated the capability of T-junctions for depth estima-

tion; to the best of our knowledge, one of the ﬁrst

attempts at depth ordering methods using T-junctions

was performed by (Marr, 1982). Later on, an inspiring

work of Nitzberg et al. (Nitzberg and Mumford, 1990;

Nitzberg et al., 1993) proposed the so-called 2.1D

sketch through a joint segmentation and depth esti-

mation model. More recently, studies have been con-

ducted using energy minimization approaches which

use either explicit (Gao et al., 2007; Palou and Salem-

bier, 2011) or implicit (Esedoglu and March, 2003)

junction detection algorithms.

In addition to T-junctions, convexity is consid-

ered to be one of the most dominant cues for ﬁgure-

ground organization (Kanizsa, 1979). A computa-

tional model for utilizing convexity has been devel-

oped for ﬁgure-ground organization in the recent past

(Pao et al., 1999). Moreover, works on occlusion rea-

soning using Gestalt-based methods have used con-

vexity as a complementary cue to T-junctions for

a more robust relative depth estimation (Dimiccoli

et al., 2008; Calderero and Caselles, 2013; Palou

and Salembier, 2013). While it has been suggested

that convexity affects human depth perception and is

coded explicitly in the brain (Burge et al., 2010), the

literature in computational models that use convex-

ity is divided in this sense. In the works (Dimiccoli

et al., 2008; Palou and Salembier, 2013), convexity

is explicitly detected and coded, while in (Calderero

and Caselles, 2013) this is done implicitly. The pro-

posed approach shares with (Dimiccoli et al., 2008;

VISAPP 2016 - International Conference on Computer Vision Theory and Applications

432

Figure 2: Diagram of the proposed method. From left to right: The segmented image; detected convexities cues (above) and

T-junctions cues (below) and the local depth order from each cue inferred by the local cues (green areas are in front of red

ones, whereas yellow indicates an inconclusive cue); global depth order extraction by rank aggregation on a graph whose

nodes represent the different shapes and the directed edges indicate local depth orders; ﬁnal result with global depth order

illustrated as a depth map, where warmer color values indicate closer objects to the camera.

Palou and Salembier, 2013; Calderero and Caselles,

2013) the use of convexity and T-junctions cues. In

order to integrate the partial depth orders suggested

by the monocular depth cues we use a graph-based

approach. Previous works (Dimiccoli and Salembier,

2009b; Palou and Salembier, 2013) also use a graph

representation but need to reduce it to an acyclic graph

and remove conﬂicts among different cues. In con-

trast, our work can directly handle conﬂicting transi-

tive orders in the graph by using a rank-aggregation-

based method (Basha et al., 2012), and obtain a glob-

ally consistent depth order. Here, transitive order is

the order established by a path in the graph involv-

ing more than two nodes using the transitivity prop-

erty mentioned in Section 1. A very recent work on

depth layering using occlusion cues is the work of

(Zeng et al., 2015) where convexity, T-junctions and a

ground contact cue is used to obtain a depth order of

the image. An energy minimization scheme is used to

ﬁnd the correct depth order which makes their method

more complex and time consuming than our proposed

method. Moreover, they have to make more restric-

tive assumptions to obtain the correct ground contact

cue which limits their method to a smaller domain.

As the method proposed by (Zeng et al., 2015) shows

promising results and performs superior to other sim-

ilar methods (Jia et al., 2012; Palou and Salembier,

2013), it has been used as a benchmark for evaluation

of our proposed method. A comparative evaluation

using the experimental setup in (Zeng et al., 2015) is

presented in Section 4.

3 PROPOSED METHOD

We propose a method to extract a global depth or-

der from a single image from an uncalibrated cam-

era. The idea is motivated by studies showing human

vision capability to integrate monocular depth cues to

create a sensible depth perception. Given an input im-

age, let us consider the set of its (segmented) shapes -

the notion of shape used in this paper will be clariﬁed

in Section 3.1.1. Then, a global depth order can be

obtained following the steps below:

1. Determine a local depth order between each pair

of adjacent shapes by analysing the convexity of

their common boundaries.

2. Detect T-junctions and use a multi-scale feature to

determine a local depth order between the shapes

that meet at each T-junction.

3. Establish a global depth order by rank aggregation

of the previous partial local orders.

Each step of the proposed method is detailed in the

following sections. Figure 2 illustrates the different

steps of the algorithm.

3.1 Local Depth Cues Detection

Local depth cues are extracted to establish a local

depth order between neighbouring objects. In this

work, convexities (L-junctions) and T-junctions are

used for this purpose. We use a segmentation of

Monocular Depth Ordering using Perceptual Occlusion Cues

433

the image as an input to the cue detection mecha-

nism. In order to compute a local depth order in a

manner that follows the human perception based on

psychophysics studies (Kanizsa, 1979; McDermott,

2004; Burge et al., 2010), T-junctions and convexi-

ties must be treated in a different manner. Thus, an

explicit detection of such depth cues is required. In

the following, we explain how we detect both kind of

junctions.

3.1.1 Convexity Cue

In this paper we propose a global convexity decision

about each connected boundary between any two ad-

jacent (segmented) regions in the image. The aim of

this step is to determine which side of the boundary is

the occluder and which side is the occluded, thus es-

tablishing a local depth order. Given the dead leaves

model assumption, this cue can be used to infer the

local depth order of the shapes that share a boundary.

To ﬁnd the occluding region, we propose a method

to determine which side of the boundary is closer to

a convex shape. Figure 3 illustrates this process. Ini-

tially the segmented image is used to obtain the set

of all the common boundaries between any two re-

gions or objects in the image (Fig. 3, left image). For

each connected common boundary, we consider its

bounding box (shown in Fig. 3, middle-up). A con-

nected common boundary divides the bounding box

into two shapes (denoted by S and S

in Fig. 3). The

shape whose area is closer to the area of its convex

hull (i.e. smaller red area in Fig. 3, middle-down) is

considered more convex and assigned as the occluder

(S in the example of Fig. 3). On the other hand, the

complement shape (S

in Fig. 3) is assigned as the oc-

cluded.

Let us notice that there is the possibility that a

given boundary does not provide a conclusive depth

cue. In other words, the convexity cue does not pro-

vide enough information to clarify which side is the

occluder and which side is the occluded. This phe-

nomenon appears, for instance, when the common

boundary is either a straight line or a sinusoidal curve.

To deal with such cases we deﬁne a criteria based on

Figure 3: Illustration of the main steps of the convexity cue

detector and the estimated local order where green areas are

estimated to be in front of red areas.

a threshold on our proposed global convexity measure

of the connected boundary between two adjacent re-

gions. This criteria is derived from the absolute dif-

ference between the convexity defect areas (red areas

in Fig. 3) of the shapes (S and S

). If this value is not

signiﬁcant enough (i.e. it is lower than a prescribed

threshold thr

) then these boundaries are considered

inconclusive and will have no effect on the result. We

deﬁne this threshold as thr

= L · π · thr, where L is

the length of the boundary and thr is a tuning param-

eter that controls the sensitivity of the criteria and is

independent of the length of the boundary. Examples

of such inconclusive boundaries for different values

of thr can be found in Figure 4; namely, the ﬁgure dis-

plays examples for a smaller value of thr = 0.0 and a

bigger value of thr = 0.6. In order to study the effect

of this parameter, both on the local and global depth

ordering, we present in Section 4 some experiments

where the threshold thr is modiﬁed in the range of

[0.0, 0.6] with step size of 0.05.

Figure 4: Illustration of modifying the value of thr

through the parameter thr. Top row, thr = 0.5; bottom row,

thr = 0.15. Decreasing thr leads to accepting more conclu-

sive boundaries (less inconclusive boundaries in yellow).

3.1.2 T-junctions Cue

One of the pivotal depth cues used in this paper are

T-junctions. T-junctions appear at the meeting points

of three shapes boundaries and are related to occlu-

sion conﬁgurations (see Figure 2). Two of the three

regions present in the T-junction are separated by the

stem of the T; these two regions are perceived to be

partly occluded by the region which presents a larger

section or angle. The latter region is then in front

of the other two. Moreover, the angle of each object

forming the junction must satisfy some criteria to be

classiﬁed as a T-junction.

In this paper, we compute T-junctions using the

method in (Caselles et al., 1999) where the authors

gave a deﬁnition of T-junction which overcomes the

difﬁculty of computing angles in a discrete image.

VISAPP 2016 - International Conference on Computer Vision Theory and Applications

434

They proposed an efﬁcient algorithm which is mainly

based on thresholding and computes junctions di-

rectly on the image without previous preprocessing

or smoothing. The segmented image is used as an in-

put to this method and the output is the locations of

T-junctions.

The deﬁnition is based on the topographic map of

an image u : Ω ∈ R

→ R (in our case, the segmented

image), that is, the family of the connected compo-

nents of the so-called, level sets of u, [u ≥ λ] := {x ∈

Ω : u(x) ≥ λ}, and on its boundaries, the so-called

level lines. Here, λ represents the gray level of the

segmented image u. The set of level sets is invariant

to monotonic non-decreasing illumination changes, a

classical requirement in image processing and com-

puter vision (Serra, 1986), and the level lines contain

the boundaries of the parts of the physical objects pro-

jected on the image plane.In practice, the algorithm

computes the T-junctions as all the pixels p where

two level lines meet and such that the area of the con-

nected component of each of the bi-level sets [u ≤ α],

[α < u < β], [u ≥ β], with α < β, meeting at p is big

enough.

After detecting the location of T-junctions, for es-

tablishing a local depth ordering one could use some

angle or area of the regions meeting at the T-junction,

both of which have been used in the literature (Dim-

iccoli and Salembier, 2009a; Palou and Salembier,

2013). Problems arise when certain conﬁgurations of

the cue lead to an inaccurate computation. One of the

problems is related to the scale at which the depth cue

is obtained.

Noise in the image can also lead to incorrect cues,

so one could use larger scales but they are less dis-

criminative in depth. To avoid these issues, we stem

from the work by (Calderero and Caselles, 2013) to

create a reliable multi-scale measure to establish a lo-

cal depth order (according to human vision) at the lo-

cated cues. To this end, features are formulated using

the curvature of the level lines of the distance func-

tion of each connected component in the segmented

image at different scales. The features are computed

for each scale s by adding the contribution from each

connected component using the following formula:

(x) =

∑

c=1

c,s

(x)

− 1), (1)

where K

c,s

is the curvature of the level lines of the dis-

tance function to the connected component c at scale

s, nc is the number of connected components at scale

s, γ

and β

are scale-related parameters which are

ﬁxed as proposed in (Calderero and Caselles, 2013).

In order to keep these features local and avoid over-

lapping with other boundaries, the distance function

is clipped at a distance 5. In order to generate a multi-

scale local feature we combine the local features ac-

cording to (1) by computing an average of the nor-

malized features at several scales, as in (Calderero

and Caselles, 2013). In this work, we integrate the

features from scales 1 to 5. Figure 5 illustrates with

an example the behaviour of this multi-scale features.

As it can be seen in Fig. 5 right, the part of the cue

that is perceptually closer to the observer has a higher

multi-scale feature value.

Figure 5: Multi-scale features obtained after averaging the

features E

(1) of the ﬁrst ﬁve scales.

Finally, to estimate the local depth order induced

by a certain T-junction, ﬁrst a representative value of

the multi-scale depth features is computed for each re-

gion (e.g. R1, R2, and R3 in Fig. 5 right) in the neigh-

borhood of the T-junction given by a disk of radius

5. The representative value is computed by applying

either the median or max operators on the features of

the respective region (i.e. R1, R2, R3). In section 4

we compare the performance of both operators. The

region with a higher representative local feature value

is assigned to be in front of the other two neighboring

regions (R3 in front of R1 and R2 in the example of

Fig. 5).

3.2 From Local to Global Depth

In order to establish a global depth order given by the

local cues we use an approximation of rank aggre-

gation (Dwork et al., 2001) similar to the one used

in (Basha et al., 2012) for photo-sequencing. To do

so, we construct a weighted graph G(U, E) to rep-

resent the partial order between pairs of shapes (ob-

jects), which are represented in the graph by the nodes

in U . The graph is constructed by placing a direc-

tional edge e(i, j) ∈ E connecting the node i to node j

if the local cues relating the objects suggest that object

i is in front of object j (represented here by i >> j).

The weight of the edge gathers up the local depth or-

der cues. Each convexity cue indicates a depth order

relation between two nodes (e.g. i << j) and each T-

junctions indicates a relation between three nodes us-

ing two edges (e.g. i << j, i << k). The weight of

Monocular Depth Ordering using Perceptual Occlusion Cues

435

Figure 6: Experiments with synthetic images: estimated global depth ordering (brighter gray levels indicate closer objects).

The automatically detected local depth cues, convexities and T-junctions establish a local depth order (green areas are esti-

mated to be in front of red areas). Inconclusive convexity cues are marked in yellow.

the edge e(i, j) between nodes i and j is proportional

to the number of local cues indicating the local order

i >> j, which can be interpreted as proportional to

the number of votes for the local order i >> j. This

weight corresponds to the probability that i >> j. In

such a graph, a random walk after a sufﬁcient time (in

the steady state) will reach the sink of the graph (or of

a sub-graph) which represents the object (or objects)

perceptually furthest from the viewer. Repeating this

process iteratively while in each iteration removing

the sink node (or nodes) from the previous iteration

will provide us with the global depth order. In par-

ticular the iteration number in which a set of nodes is

removed reveals the global order of this set of nodes.

For illustration of this process see Figure 2- step 3.

The steady state can be computed using an eigen-

vector analysis of M, the transition state matrix asso-

ciated to the graph. The elements of M are the proba-

bilities of moving from one state (node) to another. To

construct the matrix M with non-negative entries, we

initially form a matrix V collecting the votes, where

the rows and columns indices correspond to the index

of each associated connected component. Thus, an

image with N shapes will produce an N × N matrix

V . The i, j-th element of matrix V , V (i, j), collects

the number of votes (local cues) that agree with the

partial ordering i >> j.

Once the matrix V is ﬁlled, we compute the matrix

M which speciﬁes the probability that i >> j. Firstly,

the cycles of length two which may have been intro-

duced by conﬂicting cues are removed. We follow the

method proposed in (Basha et al., 2012) to resolve

these conﬂicts. In particular, M(i, j) = 1 −

V ( j,i)

V (i, j)

, and

M( j, i) = 0 if V (i, j) > V ( j, i). The rest of the cycles

do not need to be removed since the rank aggregation

method automatically solves them. Finally, the rows

of M are normalized to 1 in order to get transition

probabilities.

4 EXPERIMENTAL RESULTS

This section presents three different experiments with

different kind of data designed to evaluate and illus-

trate various aspects of the proposed method. An ini-

tial experiment is ﬁrst presented as a proof of concept

using synthetic images with the following parameters:

thr = 0.15 for convexity cue detection, and median

as T-junction feature operator. The goal of the sec-

ond experiment is twofold: ﬁrst, to present an exper-

imental study of different parameter settings to ﬁnd

the best performance and ﬁx the parameter values for

the rest of the experiments and, second, to provide a

quantitative comparison of the proposed method and

the most recent state-of-the-art methods (Jia et al.,

2012; Palou and Salembier, 2013; Zeng et al., 2015).

This experiment is done using a dataset of 52 images

proposed by (Zeng et al., 2015). For both the ﬁrst

and second experiments the ground truth segmenta-

tion is available, whereas in the third experiment the

segmentation is done using an interactive tool (Sant-

ner et al., 2010).

Figure 6 illustrates the results of applying the pro-

posed method to a small set of synthetic images. The

ﬁrst row shows the input images and the second row

shows the global depth order images with convexity

and T-junctions cues superimposed on them, respec-

tively. The local depth order is illustrated in each

cue, where green indicates the section perceived to

be closer to the observer. As for global depth or-

der, the grey values indicate global depth order, par-

ticularly the brighter areas are closer to the observer.

As it can be seen all T-junction cues indicate a cor-

rect local depth order, whereas some of the convexity

VISAPP 2016 - International Conference on Computer Vision Theory and Applications

436

0 0.1 0.2 0.3 0.4 0.5 0.6

0.5

0.55

0.6

0.65

0.7

0.75

0.8

0.85

0.9

0.95

thr

Accuracy adj. pairs

Med TJ + CX + GRA

Max TJ + CX + GRA

CX + GRA

CX No GRA

Med TJ + GRA

Max TJ + GRA

Figure 7: Accuracy of local depth order between adjacent

pairs of shapes.

0 0.1 0.2 0.3 0.4 0.5 0.6

0.54

0.56

0.58

0.6

0.62

0.64

0.66

0.68

0.7

thr

Accuracy all pairs

Med TJ + CX + GRA

Max TJ + CX + GRA

CX + GRA

Med TJ + GRA

Max TJ + GRA

Figure 8: Accuracy of global depth order between all pairs

of shapes.

Table 1: Depth Order accuracy.

adj.

pairs

all

pairs

(Jia et al., 2012) 79.84 29.88

(Palou and Salembier, 2013) 43.85 43.56

(Zeng et al., 2015) 82.66 84.60

Our method: CX+GRA 74.83 59.21

Our method: TJ+GRA 84.39 65.3

Our method: TJ+CX+GRA 91.49 69.94

cues are incorrect or inconclusive (marked as yellow).

However, the T-junctions cues are able to compensate

these errors and create a globally consistent depth or-

der that complies with human depth perception.

In the ﬁrst part of the second experiment the pro-

posed method is evaluated under different parameter

settings with the dataset proposed by (Zeng et al.,

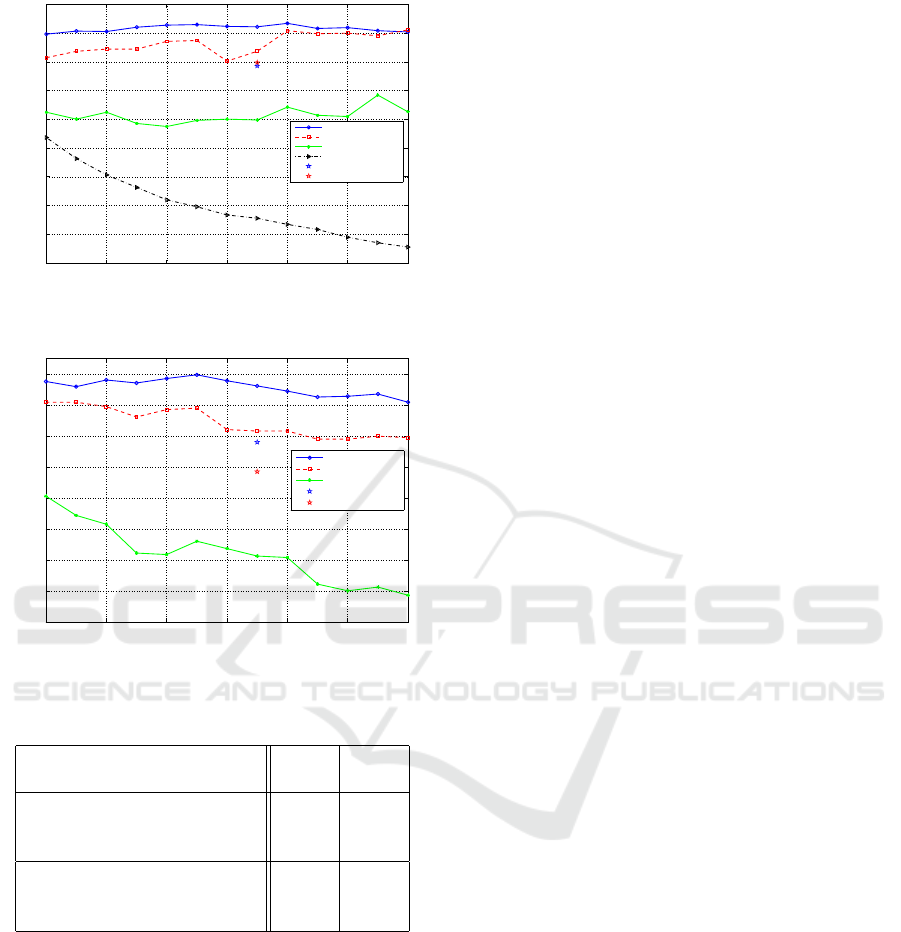
2015). Figures 7 and 8 illustrate these results. The

horizontal axis denotes the parameter thr that deﬁnes

the threshold thr

= L · π · thr applied to the differ-

ence of defect areas. Then, as the values in the hori-

zontal axis increase the threshold thr

increases and

more boundaries become inconclusive, meaning that

the sensitivity for detecting global convex boundaries

decreases (see also Fig. 4). In Figure 7 the vertical

axis indicates the accuracy as the percentage of pairs

of adjacent shapes which have been assigned a cor-

rect local depth order. Whereas in Figure 8 the ver-

tical axis indicates the accuracy as the percentage of

pairs of all shapes which have been assigned a cor-

rect global depth order. These accuracy measures are

identical to the measures of performance evaluation

in (Zeng et al., 2015). The legend of Figures 7 and

8 indicate the operator for the T-junction (median or

max), the type of local cue (T-junction (TJ), convex-

ity (CX) or both), and whether or not a global rank

aggregation was used (”GRA” or ”No GRA”). Both

Figures 7 and 8 indicate that the best performance

is achieved when the depth order induced by the T-

junction cues is computed using the median opera-

tor and is combined with the depth order induced by

the convexity cue using rank aggregation, denoted as

”Med T J +CX +GRA”. Thus, achieving a top perfor-

mance of 91.49% accuracy in local depth order esti-

mation and 69.94% in global depth order estimation.

On the other hand, using the max operator slightly

decreases the performance to 89% and 67.8% for lo-

cal and global depth estimation, respectively.The de-

crease in accuracy of the global order with respect

to the local order can be explained by the fact that

the proposed method can only infer depth relations

between objects connected by a path in the graph.

It should also be noted that the performance of the

max operator is slightly less stable. Further, it can

be seen that the contribution of T-junctions is signif-

icant for both global and local depth estimation as

they improve the performance compared to when only

convexities are used (16% increase for local depth

estimation and 19% increase in accuracy of global

depth estimation). The blue and red stars in the Fig-

ures 7 and 8 highlight the performance of using only

T-junctions (the parameter thr does not affect this

computation). As expected, T-junctions seem to be a

more reliable cue than convexities as they consistently

achieve a higher accuracy. Figure 7 illustrates how

the global integration of convexity cues using rank

aggregation improves the performance of local depth

estimation between adjacent pairs of shapes, namely,

the performance increases from 59% to 75%. Finally,

observing the two lower curves the in Figure 7 we

can see that, while the accuracy of ”CX + No GRA”

decreases as the threshold increases, the accuracy of

”CX +GRA” remains relatively stable. This indicates

that most of the convexity cues in the dataset are con-

clusive (i.e. comply with human depth perception)

and increasing the threshold will lead to less cues and

thus less accuracy. However, it is interesting to note

Monocular Depth Ordering using Perceptual Occlusion Cues

437

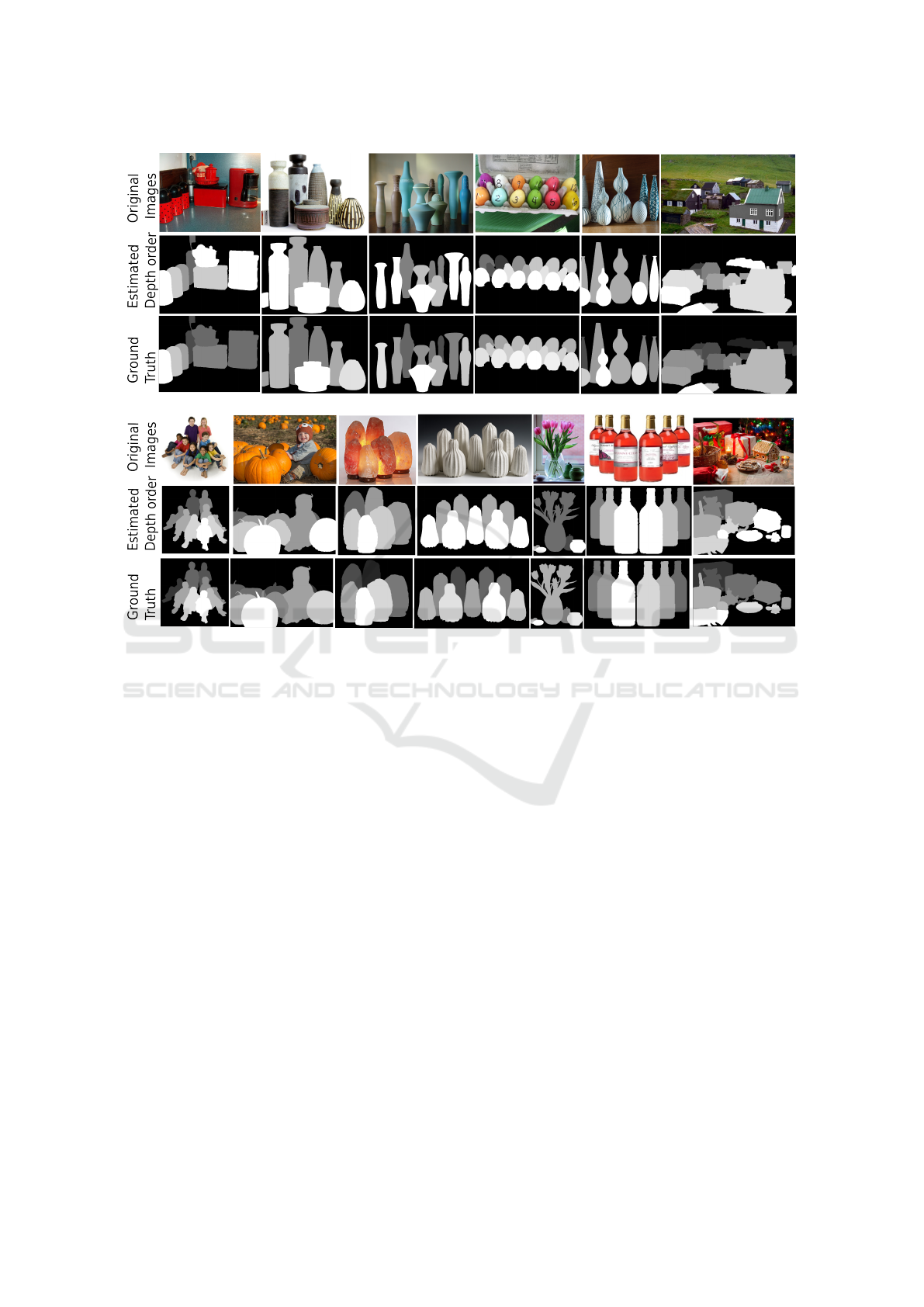
Figure 9: Depth ordering results using the proposed method on near-view scenes from the dataset by (Zeng et al., 2015).

that the global integration is able to compensate for

the removal of cues that did not satisfy the threshold

and stabilize the performance.It can be seen that the

best operation point for the threshold of the global

convexity is the mid-range value thr = 0.25, where

the average of the two accuracy measures is the high-

est. While the effect of thr is not signiﬁcant it leads

to a slight increase in the performance of the global

depth estimation (see Figure 8).

In the second part of the second experiment, the

proposed method is compared with the state-of-the-

art (Jia et al., 2012; Palou and Salembier, 2013; Zeng

et al., 2015) with the accuracy measures presented in

(Zeng et al., 2015). According to the results obtained

in the previous analysis, we ﬁx the parameters to the

following values: thr = 0.25 and median as the opera-

tor in the depth order estimated from the T-junctions.

To this end, we follow the experimental setup sug-

gested by (Zeng et al., 2015) on their proposed depth

ordering dataset. The results in Table 1 show that us-

ing a combination of T-junction and convexity cues

achieves the highest performance. As it can be seen,

the proposed method outperforms all of the state-of-

the-art methods in the adjacent pairs case and, in the

all pairs case, the proposed method performs superior

to (Jia et al., 2012) and (Palou and Salembier, 2013)

but falls short of (Zeng et al., 2015). This is mainly,as

previously noticed, due to the fact that our proposed

method cannot infer depth relations between objects

that are not are not connected with a path in our graph

i.e. there are no transitive relations to be used to infer

a global depth order. In contrast, the method by (Zeng

et al., 2015) uses the ground contact as an extra cue

to order shapes when other cues (T-junction, convex-

ity) are not present. This could be added in order to

make the constructed graph more connected. In other

words there are more transitive relations (paths) that

can be used to infer depth relations. All the previ-

ous experiments were carried out using Matlab on an

eight core 3.5GHz Core i7 processor with an average

computation time of 8.4 seconds per image.

Finally, to show how the proposed method may be

used as a real world application, the interactive seg-

mentation tool (Santner et al., 2010) has been used

to segment some images from the Berkeley dataset

(Martin et al., 2001) and the global depth order of

the segmented objects is estimated with the proposed

method. As it can be seen in Figure 10 the order of

the segmented objects is correct in most of the cases.

VISAPP 2016 - International Conference on Computer Vision Theory and Applications

438

Figure 10: Using interactive segmentation (Santner et al., 2010) and the proposed method to create a depth ordering of objects

in the scene.

5 LIMITATIONS AND

ASSUMPTIONS

Estimating depth from a single image is a very chal-

lenging and under-determined problem. It is neces-

sary to make suitable assumptions to make the prob-

lem tractable. Our ﬁrst assumption is that a good

segmentation is available where the boundaries of the

segmentation regions coincide with the actual object

boundaries. As the method is based on a convexity

cue deﬁned on boundaries and T-junctions (which are

points at the intersection of boundaries), a deﬁcient

segmentation leads to signiﬁcant depth artifacts in the

estimated depth order. A second limitation may be

noticed in one of the examples in Figure 11: the one

in box 1 of the left image. The T-junction and con-

vexity cues that are detected on the ground contact

of the object indicate incorrect depth order. In some

cases, there exist other cues that compensate for these

mistakes, either directly or indirectly using the transi-

tivity property of the graph. However, this is not the

case in the aforementioned example. Another limita-

tion is inaccuracies in our convexity detector which

can be seen in Figure 11 box 2, a misinterpretation of

convexities in cases where a long narrow shape is next

to two concavities. Figure 11 box 3 shows the bias of

the proposed method to interpret small convex objects

to be in front of their neighbouring shapes (this may

happen also in visual holes, such as windows or arch

bridges). A more general limitation is that objects in

the scene should be approximated with fronto-parallel

planes to the camera. When this assumption does not

hold it may lead to misinterpretation of local cues and

thus misestimation in the order of objects. An exam-

ple of this can be found in Fig. 11 right, box 4. In this

case, since the two objects sharing the same border

cannot be approximated with fronto-parallel planes,

the algorithm misestimates the depth order. Fortu-

nately, in some cases there are solutions to deal with

the aforementioned limitations. The non-fronto par-

allel problem can be resolved by ground separation

in simple cases. In cases where there are more than

one non-fronto parallel planes in the image, a geo-

metric context method, based for instance on surface

normal extraction, may be used to guide the depth es-

timation. The problem related to visual holes can be

addressed using a semantic labelling method that is

able to identify the visual hole; for example by classi-

fying areas like the sky which are always in the back.

6 DISCUSSION AND

CONCLUSION

Inspired by the human vision capability to perceive

depth using monocular cues, we proposed a method

for the detection and integration of T-junction and

convexity cues that is able to obtain a globally con-

sistent depth order. The proposed method computes

partial depth orders using multi-scale features, then,

integrates them using a rank aggregation method that

resolves conﬂict. This allows to simultaneously com-

pensate for incorrect partial depth orders introduced

by invalid cues and also harnesses the transitivity be-

tween the cues to obtain a global order from partial or-

ders. The proposed method is applicable to any scene

that complies with the dead leaves model and does

not require training. For future work we propose to

extend the method to images containing non fronto-

parallel objects using other monocular and binocular

cues that may be integrated in the rank aggregation

step as additional votes for partial depth orders.

ACKNOWLEDGEMENTS

The authors acknowledge partial support by MICINN

project, reference MTM2012-30772, and by GRC ref-

erence 2014 SGR 1301, Generalitat de Catalunya.

Monocular Depth Ordering using Perceptual Occlusion Cues

439

Ground

Truth

Estimated

Depth order

Original

Images

Figure 11: Due to some limitations of the proposed approach, the violation of certain assumptions leads to errors in the

estimated depth order which have been delimited with red boxes (see Section 5 for a detailed explanation of these problems).

REFERENCES

Basha, T., Moses, Y., and Avidan, S. (2012). Photo sequenc-

ing. In Computer Vision–ECCV 2012, pages 654–667.

Springer.

Burge, J., Fowlkes, C., and Banks, M. (2010). Natural-

scene statistics predict how the ﬁgure–ground cue of

convexity affects human depth perception. The Jour-

nal of Neuroscience, 30(21):7269–7280.

Calderero, F. and Caselles, V. (2013). Recovering Relative

Depth from Low-Level Features Without Explicit T-

junction Detection and Interpretation. International

Journal of Computer Vision, 104:38–68.

Caselles, V., Coll, B., and Morel, J. (1999). Topographic

maps and local contrast changes in natural images. In-

ternational Journal of Computer Vision, 33(1):5–27.

Chen, X., Li, Q., Zhao, D., and Zhao, Q. (2013). Occlusion

cues for image scene layering. Computer Vision and

Image Understanding, 117(1):42–55.

Dimiccoli, M., Morel, J.-M., and Salembier, P. (2008).

Monocular depth by nonlinear diffusion. In Com-

puter Vision, Graphics & Image Processing, 2008.

ICVGIP’08. Sixth Indian Conference on, pages 95–

102. IEEE.

Dimiccoli, M. and Salembier, P. (2009a). Exploiting

t-junctions for depth segregation in single images.

Acoustics, Speech and Signal . . . , pages 1229–1232.

Dimiccoli, M. and Salembier, P. (2009b). Hierarchical

VISAPP 2016 - International Conference on Computer Vision Theory and Applications

440

region-based representation for segmentation and ﬁl-

tering with depth in single images. Image Processing

(ICIP), 2009 16th, 1:3533–3536.

Dwork, C., Kumar, R., Naor, M., and Sivakumar, D. (2001).

Rank aggregation methods for the web. In Proceed-

ings of the 10th international conference on World

Wide Web, pages 613–622. ACM.

Eigen, D., Puhrsch, C., and Fergus, R. (2014). Depth map

prediction from a single image using a multi-scale

deep network. In Advances in Neural Information

Processing Systems, pages 2366–2374.

Esedoglu, S. and March, R. (2003). Segmentation with

depth but without detecting junctions. Journal of

Mathematical Imaging and Vision, 18(1):7–15.

Gao, R., Wu, T., Zhu, S., and Sang, N. (2007). Bayesian

inference for layer representation with mixed markov

random ﬁeld. Energy Minimization Methods in Com-

puter Vision and Pattern Recognition, 4679:213–224.

Guzm

an, A. (1968). Decomposition of a visual scene into

three-dimensional bodies. In Proceedings of the De-

cember 9-11, 1968, fall joint computer conference,

part I, pages 291–304. ACM.

Hoiem, D., Efros, A., and Hebert, M. (2011). Recovering

Occlusion Boundaries from an Image. International

Journal of Computer Vision, 91(3):328–346.

Jia, Z., Gallagher, A., Chang, Y., and Chen, T. (2012).

A learning-based framework for depth ordering. In

Computer Vision and Pattern Recognition (CVPR),

2012 IEEE Conference on, pages 294–301. IEEE.

Kanizsa, G. (1979). Organization in vision: essays on

Gestalt perception. NY, Praeger.

Liu, B., Gould, S., and Koller, D. (2010). Single image

depth estimation from predicted semantic labels. In

Computer Vision and Pattern Recognition (CVPR),

2010 IEEE Conference on, pages 1253–1260. IEEE.

Malik, J. (1987). Interpreting line drawings of curved

objects. International Journal of Computer Vision,

73403.

Marr, D. (1982). Vision: A computational approach. San

Francisco: Free-man & Co.

Martin, D., Fowlkes, C., Tal, D., and Malik, J. (2001).

A database of human segmented natural images and

its application to evaluating segmentation algorithms

and measuring ecological statistics. In Proc. 8th Int’l

Conf. Computer Vision, volume 2, pages 416–423.

Matheron, G. (1968). Mod

ele s

equentiel de partition

eatoire. Technical report, CMM.

McDermott, J. (2004). Psychophysics with junctions in real

images. Perception, 33(9):1101–1127.

Nitzberg, M. and Mumford, D. (1990). The 2.1-d sketch.

In Computer Vision, 1990. Proceedings, Third Inter-

national Conference on, pages 138–144. IEEE.

Nitzberg, M., Mumford, D., and Shiota, T. (1993). Filter-

ing, segmentation, and depth, volume 662. Lecture

notes in computer science, Springer.

Palou, G. and Salembier, P. (2011). Occlusion-based depth

ordering on monocular images with binary partition

tree. In Acoustics, Speech and Signal Processing

(ICASSP), 2011 IEEE International Conference on,

pages 1093–1096. IEEE.

Palou, G. and Salembier, P. (2013). Monocular depth or-

dering using t-junctions and convexity occlusion cues.

IEEE Transactions on Image Processing, 22(5):1926–

1939.

Pao, H., Geiger, D., and Rubin, N. (1999). Measuring con-

vexity for ﬁgure/ground separation. In Computer Vi-

sion, 1999. The Proceedings of the Seventh IEEE In-

ternational Conference on, volume 2, pages 948–955.

IEEE.

Rubin, N. (2001). Figure and ground in the brain. Nature

Neuroscience, 4:857–858.

Santner, J., Pock, T., and Bischof, H. (2010). Interactive

multi-label segmentation. In Proceedings 10th Asian

Conference on Computer Vision (ACCV), Queen-

stown, New Zealand.

Saxena, A., Chung, S. H., and Ng, A. Y. (2008). 3-d depth

reconstruction from a single still image. International

journal of computer vision, 76(1):53–69.

Serra, J. (1986). Introduction to mathematical morphology,

volume 35(3). Elsevier.

Zeng, Q., Chen, W., Wang, H., Tu, C., Cohen-Or, D.,

Lischinski, D., and Chen, B. (2015). Hallucinating

stereoscopy from a single image. In Computer Graph-

ics Forum, volume 34, pages 1–12. Wiley Online Li-

brary.

Monocular Depth Ordering using Perceptual Occlusion Cues

441