
Numerical Analysis of a New Polymer Photonic Crystal Fiber for
Sensing Applications
Issam Haddouche and Linda Cherby
Institute of Electronics and Computer Ingeneering, USTHB University, Bab Ezzouar, Algeria
Keywords: Photonic Crystal Fiber, Optical Biosensors, Polymer, Vectorial Finite Element Method.
Abstract: In this paper a new sensor design based on the enhancement of the evanescent field of the propagating
modes is presented, the sensor is a modified Photonic Crystal Fiber (PCF) with Teflon AF used as a
background material. Assessment of the sensor's performance is made by calculating Confinement loss of
the waveguide. Full-Vector Finite Element Method is used throughout the analysis. Results show a
remarkable enhancement in the evanescent field for this sensor compared with standard PCF waveguide.
1 INTRODUCTION
Over the Past decade, Photonic Crystal Fibers (PCF)
technology earned more attention in sensing
applications, Biosensing in particular (Mescia et al.,
2009; Coscelli et al., 2010). PCFs offer more design
flexibility, remote sensing and the possibility of
greater wave field profile control thanks to the
various geometry parameters, they also possess a
wide single-mode operating wavelength range and a
very interesting light dispersion properties (Saitoh
and Koshiba, 2002).
Comparing with conventional Teflon, Teflon AF
has a very interesting feature, that is, light
transmission at infrared and visible wavelength
regions with relatively low refractive index (Yang et
al., 2008).
The design of this sensor is characterized by the
intensity of the evanescent part of the optical field,
i.e. the propagating mode inside the fiber can
interact with the outer medium (Yin et al., 2008),
and thus any disturbance in this last can be detected,
which is a very interesting feature in chemical and
Bio-sensing.
The evanescent optical power is evaluated by
calculating Confinement loss of the waveguide.
A full-vector analysis is critical in order to get
accurate and reliable results (Saitoh and Koshiba,
2002). FV-FEM was used throughout this study with
edge/nodal triangular elements (Koshiba et al.,
1994) (Koshiba and Tsuji, 2000).
In this work the performance of a Teflon AF
PCF based sensor is evaluated by calculating
Confinement loss (Cl) using a FV-FEM and a
perfectly matched layer (PML) as boundary
condition (Berenger, 1993).
2 DESCRIPTION OF THE
MODEL
We consider in figure 1 a PCF with two rings of air
holes surrounded by a rectangular PML, with the
hole pitch denoted Λ, hole diameter of the inner ring
d and that of the outer ring d1. From Maxwell’s
equation the following vectorial wave equation is
derived (Saitoh et al., 2003):
0
(1)
where is the electric field, k
0
=2π/λ is the free
space wavenumber, λ is the wavelength, n is the
refractive index, [s] is complex and depends on s
i
(i
= 1...4) inside the PML region and equals the
identity matrix elsewhere (Saitoh et al., 2003):
⁄
00
0
⁄
0
00
(2)
s
x
and s
y
are given in table 1 for each PML region
with:
1
(3)
where i refers to zone 1...4 in the PML region, α
i
is
directly related to electrical conductivity and
Haddouche I. and lynda C.
NUMERICAL ANALYSIS OF A NEW POLYMER PHOTONIC CRYSTAL FIBER FOR SENSING APPLICATIONS.
Copyright
c
2016 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved
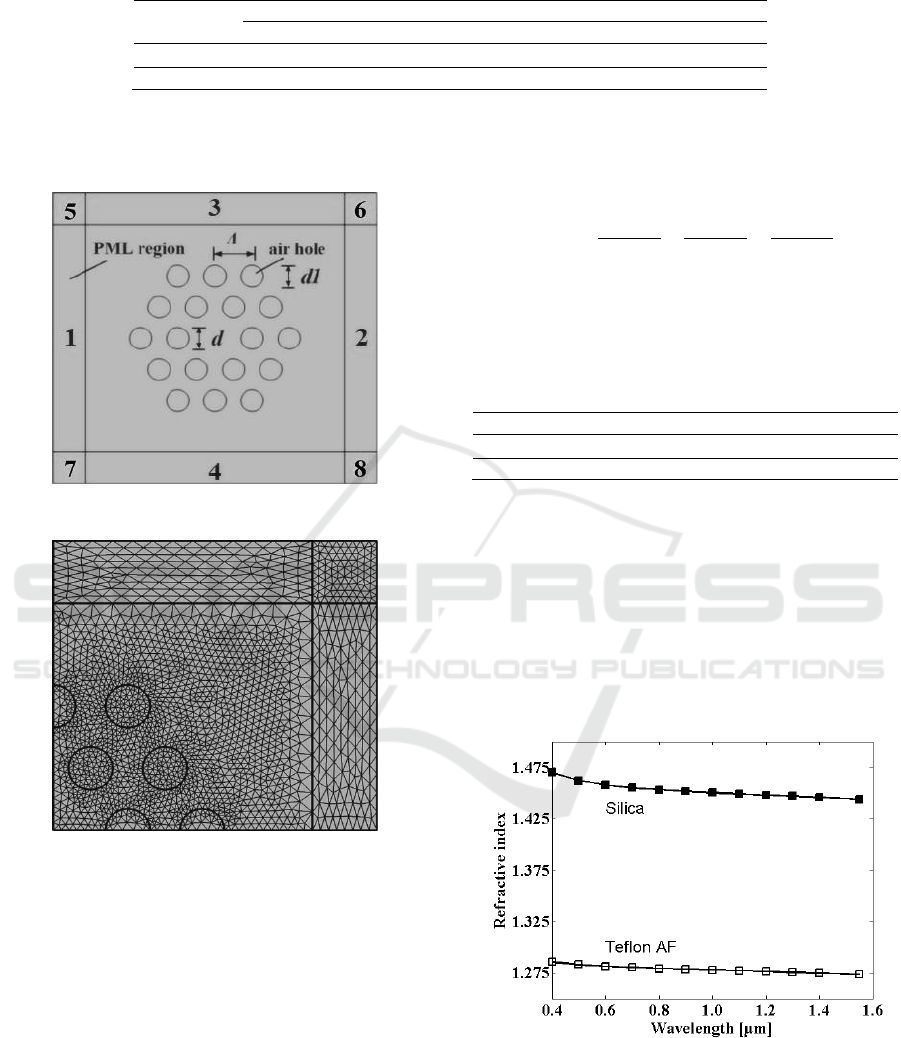
Table 1: PML parameters.
PML
parameter
PML region
1 2 3 4 5 6 7 8
s
x
s
1
s
2
1 1 s
1
s
2
s
1
s
2
s
y
1 1 s
3
s
4
s
3
s
3
s
4
s
4
reflection coefficient (Viale et al., 2005), ρ is the
distance from the beginning of the PML and t
i
is
thickness of the PML layer.
Figure 1: PCF surrounded by rectangular PML.
Figure 2: Computational window with meshing.
Taking advantage of the model’s symmetry only
one quarter is discretised into edge/nodal elements
as illustrated in figure 2, which saves some
computation time, memory and enhances accuracy.
The following eigenvalue equation is derived
(Koshiba and Tsuji, 2000):
0
(4)
where [K] and [M] are the FEM global matrices, n
eff
is the effective index of the propagating modes.
The PML layer will be used to calculate the
confinement loss [dB/m] of the propagating modes
using the following relation (Saitoh et al., 2003):
8.686
(5)
Im stands for imaginary part.
Refractive indices of silica and Teflon AF are
modeled using Sellmeier equation (Yang et al.,
2008) (Paschotta).
1
(6)
The values of B
i
and C
i
are summarized in table
2.
Material Dispersion of silica and Teflon AF are
illustrated in figure 3.
Table 2: Sellmeier Equation parameters.
material B
1
C
1
B
2
C
2
B
3
C
3
Silica 0.696 0.005 0.4 0.0135 0.897 97.93
Teflon AF 0.181 0.014 1 159.28 0 0
3 RESULTS AND DISCUSSION
The confinement loss is calculated for two kinds of
PCF, the first PCF (conventional PCF) has
d1=d=1.38 µm however the second PCF (modified
PCF) differs from the first in that d1 is smaller than
d, each waveguide is modelled for the two
background materials silica and Teflon AF
.
Figure 3: Material dispersion of silica and Teflon AF.
Figures 4 and 5 depict the x-component of the
electric field of the
mode of the Teflon AF
PCF for the two PCF models.
The mode field profile seems to be more
extended outside the core in the modified PCF, this

is due to the fact that the hole diameter of the outer
ring is smaller thus enhancing the evanescent field.
After solving eigenvalue equation (4), complex
n
eff
is obtained and relation (5) is used to calculate
confinement loss.
Figure 4: x-component of the electric field of the
HE
mode of the Teflon AF conventional PCF with: 18
air holes, Λ=2.3µm and d=d1=1.38µm.
Figure 5: x-component of the electric field of the
HE
mode for the modified Teflon AF PCF with: 18 air
holes, Λ=2.3µm, d= 1.38µm and d1=0.92µm.
Numerical Results are illustrated in figures 6 and
7, where confinement loss of the
mode was
calculated with respect to wavelength for
conventional and modified two rings PCFs
respectively and for two background materials. The
hole diameter of the outer ring was taken
d1=0.92µm in the modified PCF.
These results show that confinement loss
increases with wavelength, this can be explained by
the dependence of the mode field diameter (MFD)
on wavelength (Saitoh and Koshiba, 2005)
(Agrawal, 2002) i.e. the more MFD is important the
more mode profile is extended outside the core. We
can also note that Teflon AF PCF has a greater
confinement loss than that of silica PCF, this is due
to the lower index contrast in Teflon case providing
wider MFD (Saitoh and Koshiba, 2005)
(Agrawal,
2002).
Figure 6: Confinement loss plot of conventional PCF with
respect to wavelength with: 18 air holes, Λ=2.3µm,
d=1.38µm and d1=d.
Figure 7: Confinement loss plot of modified PCF with
respect to wavelength with: 18 air holes, Λ=2.3µm,
d=1.38µm and d1=0.92µm.
Reducing the hole diameter of the outer ring d1,
allows more energy to leave the core towards the
outer medium this is the reason why confinement
loss is more important in the modified PCF as
illustrated in figure 7.
4 CONCLUSIONS
A numerical study of a PCF for Biosensing
applications was presented in this paper, propagation
loss is a very important parameter in evanescent
wave based sensors, these sensors are simple, cheap
and easy to use unlike other types such as Surface

Plasmon Resonance (SPR) based sensors, and can be
used within interferometers to detect phase shift
resulting from disturbance in outer medium. As it is
well known PCFs are inherently lossy, the use of
some special materials with lower refractive indices
than silica such as Teflon AF will increase
confinement loss even more because of the lower
index contrast between the core and the cladding,
altering geometrical parameters values such as the
pitch Λ and the hole diameter d can also increase
confinement loss.
REFERENCES
L. Mescia, F. Prudenzano, L. Allegretti, 2009. Design of
silica based photonic crystal fiber for biosensing
applications, Journal of Non-Crystalline Solids 355.
Coscelli, E, M. Sozzi, F. Poli, 2010. Toward A Highly
Specific DNA Biosensor: PNA-Modified Suspended-
Core Photonic Crystal Fibers, IEEE Journal of
Quantum Electronics, Vol. 16, No. 4, 967–972.
Saitoh, K, M. Koshiba, 2002. Full-vectorial imaginary-
distance beam propagation method, IEEE Journal of
Quantum Electronics, Vol. 38, No. 7, 927–933.
Yang, M. K., R. H. French, E. W. Tokarsky, 2008. Optical
properties of Teflon® AF amorphous fluoropolymers,
J. Micro/Nanolith. MEMS MOEMS, Vol. 7, No. 3, 1–
9.
S. Yin, C. Zhan, P. Ruffin, 2008. Fiber optic sensors.
Koshiba, M, S. Maruyama, K. Hirayama, 1994. A vector
finite element method with the high-order mixed-
interpolation-type triangular elements, Journal of
lightwave technology, Vol. 12, No. 3, 495–502.
Koshiba, M, Y. Tsuji, 2000. Curvilinear hybrid
edge/nodal elements with triangular shape for guided-
wave problems, Journal of lightwave technology, Vol.
18, No. 5, 737–743.
Berenger, J., 1993. A perfectly matched layer for the
absorption of Electromagnetic waves, Journal of
computational physics, Vol. 114, 185–200.
Saitoh, K, M. Koshiba, T. Hasegawa, E. Sasaoka, 2003.
Chromatic dispersion control in photonic crystal
fibers: application to ultra-flattened dispersion, Optics
express, Vol. 11, No. 8, 843–852.
Viale, P, S. Février, F. Gérome, H. Vilard, 2005.
Confinement Loss Computations in Photonic Crystal
Fibres using a Novel Perfectly Matched Layer Design,
COMSOL Multiphysics User's Conference Paris.
R. Paschotta, Sellmeier Formula, RP Photonics,
https://www.rp-photonics.com/sellmeier_formula.html.
Saitoh, K, M. Koshiba, 2005.Numerical Modeling of
Photonic Crystal Fibers, Journal Of Lightwave
Technology, Vol. 23, No. 11, 3580- 3590, November.
G. P. Agrawal, 2002. Fiber-Optic Communications
Systems, Third Edition, Wiley, pp. 33-37.
