A Structural Subsumption based Similarity Measure

for the Description Logic ALEH

Boontawee Suntisrivaraporn and Suwan Tongphu

School of Information, Computer and Communication Technology,

Sirindhorn International Institute of Technology, Thammasat University, Pathumthani, Thailand

Keywords:

Similarity Measure, Description Logic, Non-standard Reasoning, Semantic Web.

Abstract:

Description Logics (DLs) are a family of logic-based knowledge representation formalisms, which can be

used to develop ontologies in a formally well-founded way. The standard reasoning service of subsumption

has proved indispensable in ontology design and maintenance. This checks, relative to the logical deﬁnitions in

the ontology, whether one concept is more general/speciﬁc than another. When no subsumption relationship

is identiﬁed, however, no information about the two concepts can be given. This work extends from an

existing work on similarity measure in ELH to the more expressive description logic ALEH . We introduce

generalizations of the notion of normalization and homomorphism in ALEH which are then employed at the

heart of our semantic similarity measure. The proposed similarity measure computes a numerical degree of

similarity between two ALEH concept descriptions despite not being in the subsumption relation.

1 INTRODUCTION

Representing knowledge base is one interesting topic

in artiﬁcial intelligence. Among various techniques of

semantic-level analysis, one commonly well-founded

way is through the help of Description Logics (DLs)

(Baader et al., 2007). Being recommended by W3C,

DLs (i.e. the logical underpinning of the Web Ontol-

ogy Language (OWL)) become a standard tool for

formally and systematically modelling a knowledge

base. Besides their unambiguous syntax and seman-

tics which are essential for ontology modelling and

sharing, DLs provide several useful reasoning ser-

vices that allow inferencing of implicit knowledge

from the one explicitly deﬁned. For example, with

a service of a subclass-superclass relation identiﬁ-

cation (concept subsumption), two deﬁned concepts

which are visually out of subsumption relation may be

logically classiﬁed into the same hierarchy. Though

seemingly useful, the classical DL reasoning service

of concept subsumption merely produces a crisp re-

sponse. The service indeed provides a positive con-

clusion if and only if all necessary and sufﬁcient con-

ditions of being in the subclass–superclass relation are

fully satisﬁed. Otherwise, alas, it will suggest that the

two concepts are irrelevant to each other.

In some concrete situation, checking for subsump-

tion relation may not be adequate. Consider for exam-

ple the case in which a new disease, which is closely

similar to the existing one, is being discovered. Since

we know that the two diseases are similar, checking

for their common characteristics would likely provide

a beneﬁcial clue to the disease etiology. Therefore,

it would be easy to suggest an appropriate treatment

from previously known diseases to another new one.

This work is an extension of an existing similarity

measures for DLs in the EL family (Suntisrivaraporn,

2013; Tongphu and Suntisrivaraporn, 2014; Tongphu

and Suntisrivaraporn, 2015) to the strictly more ex-

pressive DL ALEH . The method is based on the

known homomorphism-based structural subsumption

and produces a numerical degree of similarity be-

tween two ALEH concept descriptions despite not

being in the subsumption relation.

The rest of the paper is organized in order. The

background on the DL ALEH , unfoldable TBoxes,

and the structural subsumption algorithm is presented

in the next section. Section 3 and 4 introduce the no-

tions of homomorphism degree and ALEH seman-

tic similarity measure, respectively, and exemplify the

introduced measure by means of a small yet prototyp-

ical medical ontology. Section 5 suggests a possible

extension of similarity measure for the DL ALC H .

Related works are discussed in Section 6, and the last

section gives some concluding remarks.

204

Suntisrivaraporn, B. and Tongphu, S.

A Structural Subsumption based Similarity Measure for the Description Logic A LEH .

DOI: 10.5220/0005819302040212

In Proceedings of the 8th International Conference on Agents and Artiﬁcial Intelligence (ICAART 2016) - Volume 2, pages 204-212

ISBN: 978-989-758-172-4

2 BACKGROUND

In DLs, concept descriptions are inductively deﬁned

with the help of a set of constructors, starting with

a set CN of concept names and a set RN of role

names. ALEH concept descriptions are formed us-

ing the constructors shown in the upper part of Ta-

ble 1. An ALEH terminology or TBox is a ﬁnite

set of concept deﬁnitions and role hierarchy axioms,

of which the syntactic forms are shown in the lower

part of Table 1. A TBox is called unfoldable if it is

deﬁnitorial (i.e. containing at most one concept def-

inition for each concept name), and acyclic (i.e. not

containing cyclic dependencies). Figure 1 depicts an

example unfoldable ALEH TBox. The set CN

def

of deﬁned concepts comprises those concept names

that appear on the left hand side of a concept deﬁ-

nition. All other concept names are called primitive

concepts, denoted by CN

pri

. Since the DL ALEH al-

lows for atomic negation, for convenience, we denote

by CN

label

the set of all primitive concepts, their nega-

tions, and the bottom concept, i.e. CN

label

= { A,¬A |

A ∈ CN

pri

} ∪ {⊥}. Conventionally, r,s possibly with

subscripts are used to range over R N, A, B to range

over CN, and C, D to range over concept descriptions.

Primitive concept deﬁnitions are commonly found in

realistic terminologies to deﬁne those concepts, of

which only necessary conditions are known. For in-

stance,

HappyMan ⊑ Man ⊓ Rich ⊓ ∃child.Beautiful

(1)

Such a primitive deﬁnition B ⊑ D can easily be

transformed into a semantically equivalent full deﬁni-

tions B ≡ X ⊓ D where X is a fresh concept name.

Like other DLs, the semantics of ALEH is de-

ﬁned in terms of interpretations I = (∆

,·

), where

the domain ∆

is a non-empty set of individuals, and

the interpretation function ·

maps each concept name

A ∈ CN to a subset A

of ∆

and each role name

r ∈ RN to a binary relation r

on ∆

. The extension

of ·

to arbitrary concept descriptions is inductively

deﬁned, as shown in the semantics column of Table 1.

An interpretation I is a model of a TBox O if, for

each concept deﬁnition in O, the conditions given in

the semantics column of Table 1 are satisﬁed. The

main inference problem for ALEH is the subsump-

tion problem:

Deﬁnition 1 (Concept Subsumption). Given two

ALEH concept descriptions C, D and an ALEH

TBox O, C is subsumed by D w.r.t. O (writtenC ⊑

if C

⊆ D

in every model I of O. Moreover, C, D are

equivalent w.r.t. O (written C ≡

D) if C ⊑

D and

D ⊑

Woman ≡ Female ⊓ Person

Man ≡ ¬Female ⊓ Person

Parent ≡ Person ⊓ ∃child.Person

Mother ≡ Woman ⊓ Parent

Father ≡ Man ⊓ Parent

MotherNoSon ≡ Mother ⊓ ∀child.Woman

MotherNoDaughter ≡ Mother ⊓ ∀child.Man

FosterFather ≡ Man ⊓ ∃fchild.Person

NonFosterFather ≡ Father ⊓ ∀fchild.⊥

fchild ⊑ child

Figure 1: An example ALEH terminology O

family

;

here child and fchild are shorthands for hasChild and

hasFosterChild, respectively.

Provided that the TBox is unfoldable (i.e. acyclic and

deﬁnitional), any ALEH concept description can be

expanded to an equivalent one that may use any role

names but consists only of primitive concept names,

their negations and the bottom concept from CN

label

This can be done by repeatedly replacing a deﬁned

concept by its deﬁnition until no more deﬁned con-

cepts appear in the concept description. Consider,

for instance, the concept MotherNoSon along with its

deﬁnition ω

in Figure 1. By replacing the deﬁned

concept Mother and Woman with their correspond-

ing descriptions (see ω

and ω

), the description can

be expanded to:

Female ⊓ Person ⊓ ∃child.Person ⊓

∀child.(Female ⊓ Person)

(2)

where Person,Female ∈ CN

label

. We denote by

C the

expanded equivalence of the concept description C.

We can assume without loss of generality that an

ALEH concept C can be expanded and has the fol-

lowing form:

i=1

⊓

j=1

∃r

⊓

k=1

∀s

where L

∈ CN

label

, r

∈ RN, and D

are

ALEH concept descriptions in the same for-

mat as C. For simplicity, we assign P

,. . . , L

}, E

:= {∃r

,. . . ,∃r

}, and A

{∀s

,. . . , ∀s

}. Also, we denote by R

∃r

and

∀r

the sets of all super-roles and of all sub-roles of

r, respectively. That is, R

∃r

= { s ∈ RN | r ⊑

∗

s} and

∀r

= {t ∈ RN | t ⊑

∗

r} where where ⊑

∗

represents

the reﬂexive-transitive closure of ⊑ over role names.

However, since a normalized ALEH concept de-

scription makes implicit description explicit and yet

A Structural Subsumption based Similarity Measure for the Description Logic ALE H

205

Table 1: Syntax and semantics of the DL ALEH and DL ALCH .

Name Syntax Semantics ALEH ALC H

bottop ⊥

0 X X

top ⊤ ∆

X X

concept name A A

⊆ ∆

X X

atomic negation ¬A ∆

\A X X

concept negation ¬C ∆

\C X

concept conjunction C⊓ D C

∩ D

X X

concept disjunction C⊔ D C

∪ D

existential restriction ∃r.C {x | ∃y ∈ ∆

: (x,y) ∈ r

∧ y ∈ C

} X X

value restriction ∀r.C {x | ∀y ∈ ∆

: (x,y) ∈ r

⇒ y ∈ C

} X X

primitive deﬁnition

B ⊑ D A

⊆ D

X X

full deﬁnition B ≡ D A

= D

X X

role hierarchy r ⊑ s r

⊆ s

X X

preserves equivalence, we exhaustively apply the fol-

lowing normalization rules to the ALEH concept de-

scriptions after expansion. The normalization rules

below are modulo commutativity of conjunction:

∀s.C ⊓ ∀r.D → ∀s.C⊓ ∀r.(C⊓ D)

∀s.C ⊓ ∃r.D → ∀s.C⊓ ∃r.(C⊓ D)

∀r.⊤ → ⊤

C⊓ ⊤ → C

A⊓ ¬A → ⊥

∃r.⊥ → ⊥

C⊓ ⊥ → ⊥

where s ∈ R

∃r

. Note that the ﬁrst two normalization

rules generalize the corresponding ones in (Baader

and K¨usters, 2006) where a role hierarchy is taken

into consideration. In fact, for a super-role s of r, it is

the case that ∀s.C implies ∀r.C.

For example, let MotherNoSon be expanded and

has the form as shown in Equation 2. By applying

the above rules, a normalized concept description of

MotherNoSon can be exempliﬁed as follows:

Female ⊓ Person ⊓ ∃child.(Female ⊓ Person) ⊓

∀child.(Female ⊓ Person)

In (Baader and K¨usters, 2000; Baader, 2003),

a characterization of subsumption in ALEH w.r.t.

an unfoldable TBox using homomorphism has been

proposed. Instead of considering concept descrip-

tions directly, the characterization considers so-called

ALEH description trees that structurally correspond

to the ALEH concept descriptions. Given the ex-

panded concept descriptionC, beginning from the top

level, such a description can recursively be translated

into an ALEH description tree G

:= (V, E, v

,ℓ, ρ)

where V is a set of nodes, E ⊆ V ×V is a set of edges,

∈ V is the root, ℓ : V → 2

label

is a node labelling

function, and ρ : E → 2

is an edge labelling func-

tion. The translation can be done using the following

steps:

i. Assign P

to ℓ(v

ii. For each ∃r.X

∈ E

, introduce a new node w to

V, add an edge (v

,w) to E, and assign R

∃r

ρ(v

,w). Repeat from step (i) by treating w as v

and X as C.

iii. For each ∀r.Y

∈ A

, introduce a new node w

′

V, add an edge (v

′

) to E, and assign R

∀r

ρ(v

′

). Repeat from step (i) by treating w

′

and Y as C.

In essence, the root v

of the ALEH description tree

has P

as its label; has m existential edges, each

labeled with R

∃r

to a vertex w

; and has n universal

edges, each labeled with R

∀s

to a vertex w

′

, for 1 ≤

j ≤ m and 1 ≤ k ≤ n. Each of the child nodes w

and

′

is the root of a similar tree structure which forms

a subtree of G

Deﬁnition 2 (Homomorphism). A homo-

morphism from an ALEH description tree

G = (V, E, v

,ℓ, ρ) into an ALEH description

tree G

′

= (V

′

,ℓ

′

,ρ

′

) is a mapping h : V → V

′

such that:

i. h(v

) = v

′

ii. ℓ(v) ⊆ ℓ

′

(h(v)) for all v ∈ V,

iii. for each existential edge (v, w) ∈ E with ρ(v,w) =

∃r

, there exists (h(v), h(w)) ∈ E

′

such that

′

(h(v),h(w)) = R

∃s

and R

∃r

⊆ R

∃s

, and

ICAART 2016 - 8th International Conference on Agents and Artiﬁcial Intelligence

206

iv. for each universal edge (v, w) ∈ E with ρ(v,w) =

∀r

, there exists (h(v), h(w)) ∈ E

′

such that

′

(h(v),h(w)) = R

∀t

and either of the following

holds:

a. R

∀r

⊆ R

∀t

, or

b. h(v) = h(w) and ℓ

′

(h(v)) = { ⊥}.

Observe that this generalizes the notion of ho-

momorphism ﬁrst introduced in (Baader and K¨usters,

2006) by allowing each edge label to be a set of role

names instead of a mere role name. Moreover, it sim-

pliﬁes the condition for existential edge mapping by

omitting ⊥ since any existential successor with ⊥ as

its label must be collapsed due to the normalization.

The subsumption is then characterized by means

of an existence of a homomorphism in the reverse di-

rection.

Theorem 1 ((Baader and K¨usters, 2006)). Let C, D

be ALEH concept descriptions, and G

the cor-

responding ALEH concept description trees. Then,

C ⊑ D iff there exists a homomorphism h : G

→ G

which maps the root of G

to the root of G

Consider the normalized description for

MotherNoSon as previously mentioned and the

following normalized descriptions for Mother and

NonFosterFath er:

Female ⊓ Person ⊓ ∃child.Person

(3)

¬Female ⊓ Person ⊓ ∃child.Person ⊓ ∀fchild.⊥

(4)

Figure 2 depicts the ALEH description

trees G

NonFosterFather

(left) G

Mother

(center), and

MotherNoSon

(right). It is important to note here

that R

∀child

= {fchild,child} and R

∀fchild

= {fchild}

since ω

is the only role hierarchy axiom in the

ontology. This ﬁgure shows a homomorphism h

(dashed arrows) that maps the root u

of G

Mother

to the root v

of G

MotherNoSon

. It also demonstrates

a failed attempt to map (see the dotted arrow) the

root of G

Mother

to the root of G

NonFosterFather

. The-

orem 1 ensures that MotherNoSon ⊑

Mother and

NonFosterFath er 6⊑

Mother.

Though sharing some common features between

MotherNoSon and NonFosterFather (i.e. both are

Person ), the classical reasoning of subsumption can-

not tell how similar the two descriptions are. This

leads to an introduction of a concept similarity mea-

sure based on the structural characterization. Instead

of merely giving either positive or negative result be-

tween descriptions, the proposed measure calculates a

numerical value ranging between 0 and 1. Intuitively,

the larger the number approaching to 1, the more sim-

ilar the two concepts are.

3 HOMOMORPHISM DEGREE IN

ALEH

As suggested by Theorem 1, an existence of a homo-

morphism mapping from one ALEH description tree

to another implies a subsumption relationship in a re-

verse direction. We extend the idea to the case where

a homomorphism between the two ALEH descrip-

tion trees does not exist but there is a shared structure.

LetC, D be ALEH concept descriptions, and G

and

be the corresponding ALEH description trees.

Also, let P

, and A

be as deﬁned in

the previous section. We deﬁne the homomorphism

degree from G

to G

as follows:

Deﬁnition 3 (Homomorphism Degree). Let

ALEH

be the set of all ALEH descrip-

tion trees. The homomorphism degree function

hd : G

ALEH

× G

ALEH

→ [0, 1] is deﬁned as follows:

hd(G

) := (1− µ

− µ

) · p-hd(P

· e-set-hd(E

· a-set-hd(A

)

(5)

where | · | represents the set cardinality, µ

∪ E

∪ A

, and µ

∪ E

∪ A

;

p-hd(P

) :=

(

1 if P

0 or P

= {⊥}

∩ P

otherwise,

(6)

e-set-hd(E

) :=











1 if E

0 if E

0, E

∑

∈E

max{e-hd(ε

,ε

):ε

∈E

}

otherwise,

(7)

where ε

,ε

are existential restrictions;

e-hd(∃r.X, ∃s.Y) :=

(ν

(r) + (1− ν

(r)) · hd(G

))

(8)

where γ

∃r

∩ R

∃s

∃r

and ν

: RN → [0,1).

a-set-hd(A

) :=











1 if A

0 if A

0, A

∑

∈A

max{a-hd(α

,αj):α j∈A

}

otherwise

(9)

where α

,α

are universal restrictions; and ﬁnally

A Structural Subsumption based Similarity Measure for the Description Logic ALE H

207

: {¬Female,Person}

: {Person}w

: {⊥}

: {Female,Person}

: {Person}

: {Female,Person}

: {Female,Person} v

: {Female,Person}

∃{child}

∃{child} ∀{fchild,child}

∃{child}

∀{fchild}

Figure 2: A homomorphism h (dashed arrows) that maps the root of G

Mother

to the root of G

MotherNoSon

; a failed attempt to

identify a homomorphism (dotted arrows) that maps the root of G

Mother

to the root of G

NonFosterFather

a-hd(∀r.X, ∀s.Y) :=



if P

= {⊥},

(ν

(r) + (1− ν

(r)) · hd(G

)) otherwise

(10)

where γ

∀r

∩ R

∀s

∀r

and ν

: RN → [0, 1).

Note that since ∃r.⊥ can never occur in any nor-

malized ALEH concept description, we need not

treat this case in Equation 7 (cf. Deﬁnition of homo-

morphism in the previous section and in (Baader and

K¨usters, 2006)). Intuitively, the homomorphism de-

gree (hd) of the two given ALEH description trees

can be computed based on the degree of common

node label inclusion and the degree of common out-

going edges. Formula 6 calculates the proportion of

the matched node labels comparing to all those avail-

able in the top level. Formula 7 and 9 computes the

degrees of edge matching of an existential restriction

and a universal restriction, respectively. If there is a

shared edge label, then there is some degree of sim-

ilarity; but the successors’ labels and structures have

yet to be checked. This is done recursively by calling

the function hd(G

The parameter µ

(resp., µ

) deﬁned in Formula 5

indicates how important the existentially quantiﬁed

(resp., universally quantiﬁed) subconcepts are to be

considered for similarity measure. The use of ν

and ν

allows to indicate an importance of the role

name in an existential restriction and a universal re-

striction. It is similar to that in (Suntisrivaraporn,

2013) except that these are deﬁned as a function on

role names. This means that the importance of dif-

ferent role names and thus the discount of similarity

between nested concepts can be unequally assigned

based on their use and modelling discipline in a par-

ticular ontology. The value of γ in Formula 8 and 10

indicates a degree of inclusion between the two edge

labels. The case where γ = 0 means there is no com-

monality between two given roles, and hence further

computation for the degrees of membership between

their corresponding nested pairs should be omitted.

Example. To better understand how the algorithm

works, consider the description tree G

Mother

for

the unfolding of Mother and the description tree

NonFosterFather

for the unfolding of NonFosterFather

as shown in Figure 2. Using µ as previously described

and ﬁxing ν

⋆

(r) to 0.4 for every role name r ∈ RN, the

degrees of homomorphism from the root of G

Mother

to the root of G

NonFosterFather

can be computed as fol-

lowing steps (abbreviations are used for the sake of

simplicity):

hd(G

NFF

)

p-hd(P

NFF

) +

e-hd(E

NFF

(0)a-hd(A

NFF

)

[

] +

e-hd(ε

,ε

)

// with µ

, µ

= 0,

// ε

= ∃child.Person and ε

= ∃child.Person

[

] +

[

][

hd(G

Person

)]

[

] +

[

]]

:= 0.67

The reverse direction can be computed as follows:

hd(G

NFF

)

p-hd(P

NFF

) +

e-hd(E

NFF

a-hd(A

NFF

)

[

] +

e-hd(ε

,ε

) +

a-hd(α

,α

)

// with µ

, µ

// ε

= ∃child.Person and ε

= ∃child.Person

// α

= ∀fchild.⊥ and α

[

] +

[

][

hd(G

Person

)] +

[0]

:= 0.50

Hence, the degree of having a homomorphism

from the root of G

Mother

to G

NonFosterFather

is 0.67, and

that for the opposite direction is 0.50. The hd values

for other pairs can be obtained in an analogous man-

ner and are shown in Table 2.

Using a proof by induction, together with Theo-

rem 1 (Baader and K¨usters, 2000; Baader, 2003), it is

ICAART 2016 - 8th International Conference on Agents and Artiﬁcial Intelligence

208

Table 2: Homomorphism degrees to and from the deﬁned concepts in O

family

hd(↓, →) Woman Man Parent Mother Father MNS MND FF NFF

Woman 1.00 0.50 0.50 0.67 0.33 0.50 0.50 0.33 0.25

Man 0.50 1.00 0.50 0.33 0.67 0.25 0.25 0.67 0.50

Parent 0.50 0.50 1.00 0.67 0.67 0.43 0.43 0.50 0.50

Mother 1.00 0.50 1.00 1.00 0.67 0.68 0.68 0.50 0.50

Father 0.50 1.00 1.00 0.67 1.00 0.43 0.43 0.83 0.75

MotherNoSon (MNS) 1.00 0.50 1.00 1.00 0.67 1.00 0.85 0.50 0.60

MotherNoDaughter (MND) 1.00 0.50 1.00 1.00 0.67 0.85 1.00 0.50 0.60

FosterFather (FF) 0.50 1.00 1.00 0.67 1.00 0.43 0.43 1.00 0.75

NonFosterFath er (NFF) 0.50 1.00 1.00 0.67 1.00 0.55 0.55 0.83 1.00

not difﬁcult to obtain the correspondence between the

homomorphism degree and subsumption.

Proposition 2. Let C, D be expanded and normalized

ALEH concept descriptions, and G

, G

be their

corresponding description trees, respectively. Then,

the following are equivalent:

1. C ⊑ D,

2. hd(G

) = 1.

In fact, the closer the hd(G

) value is to 1, the

more likely the corresponding subsumption may hold.

More precisely, the label and edge constraints in G

can likely be simulated by those in G

4 ALEH SEMANTIC

SIMILARITY

The homomorphism degree function introduced in

Section 3 returns a degree that represents the sim-

ilarity of one concept description compared to an-

other concept description. As shown in the compu-

tation example, the direction of the homomorphism

degree matters, viz., hd(G

NFF

) = 0.67, whereas

hd(G

NFF

) = 0.50. Since both directions consti-

tute the degree of the two concepts being equivalent,

our similarity measure for ALEH concept descrip-

tions is deﬁned by means of these values.

Deﬁnition 4 (ALEH Concept Similarity). Let C, D

be expanded ALEH concept descriptions. The

degree of similarity between C and D is deﬁned as:

sim

ALEH

(C,D) :=

hd(G

) + hd(G

)

(11)

Intuitively, the degree of similarity between two

concepts is the average of the degree of having ho-

momorphisms in both directions, thus sim(C, D) =

sim(D,C) as required.

Note that other functions apart from average could be

applied; for instance, root mean square and multiplication

(Suntisrivaraporn, 2013).

Based on the homomorphism degree values in Ta-

ble 2, the degrees of similarity among the deﬁned

concepts in the example ontology O

family

can be ob-

tained; see Table 3. Note also that, though not in-

cluded in Table 2 and 3, the similarity involving prim-

itive concepts like Female and Person can also be

computed. Nevertheless, the pairwise similarity de-

gree between any two primitive concepts is zero by

our deﬁnition since there is absolutely no commonal-

ity between them apart from both being subsumed by

⊤.

The similarity measure sim

ALEH

generalizes sim for

the DL ELH (Suntisrivaraporn, 2013; Tongphu and

Suntisrivaraporn, 2015) in the sense that when two

given concept descriptions are restricted to ELH ,

then both measures coincide.

Proposition 3. Let C, D be two ELH concept de-

scriptions. Then,

sim

ALEH

(C,D) = sim(C, D).

This is the case since any ELH description tree is

also an ALEH description tree that does not contain

universal edges.

5 APPROXIMATING ALCH

SEMANTIC SIMILARITY

A description logic ALC H can be considered as an

extension of ALEH that supports more concept con-

structors, namely disjunction and full concept nega-

tion (see Table 1). Since DL ALEH is a language in

the family DL ALC H , in this section, we show that

the notion of ALEH similarity measure can be ex-

tended to a new notion of ALC H similarity measure.

In Section 3 we review the structural characteri-

zation of subsumption ALEH through a homomor-

phism. Alas, this characterization is not directly ap-

plicable to the more expressive language ALCH due

to disjunction. Fortunately, one can approximate an

A Structural Subsumption based Similarity Measure for the Description Logic ALE H

209

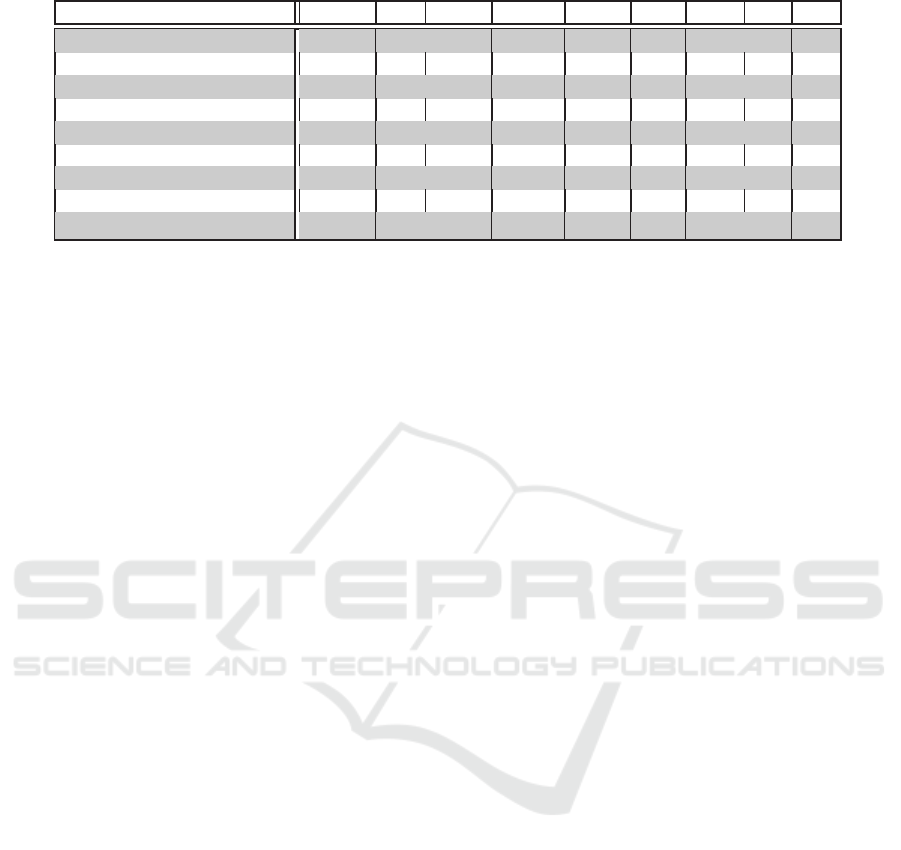
Table 3: Similarity degree between a pair of deﬁned concepts in O

family

hd(↓,→) Woman Man Parent Mother Father MNS MND FF NFF

Woman 1.00 0.50 0.50 0.83 0.42 0.75 0.75 0.42 0.38

Man 1.00 0.50 0.42 0.83 0.38 0.38 0.83 0.75

Parent 1.00 0.83 0.83 0.71 0.71 0.75 0.75

Mother 1.00 0.67 0.84 0.84 0.58 0.58

Father 1.00 0.55 0.55 0.92 0.88

MotherNoSon (MNS) 1.00 0.85 0.46 0.58

MotherNoDaughter (MND) 1.00 0.46 0.58

FosterFather (FF) 1.00 0.79

NonFosterFath er (NFF) 1.00

ALC H -concept description in the less expressive DL

ALEH . Once approximation is calculated, the simi-

larity measure introduced in this paper could be used

to obtain approximate similarity between two concept

descriptions written in ALCH .

Deﬁnition 5 (Approximation). (Baader and K

usters,

2006) Let C be an ALCH -concept description.

An ALEH -concept description D is an ALEH -

approximation of C, written ALEH -approx(C), iff

i. C ⊑ D and

ii. D ⊑ E for every ALEH -concept description E

with C ⊑ E.

Intuitively, an approximation is the most speciﬁc

concept in ALEH that subsumes the given ALC H

concept. One can approximate an ALCH con-

cept by resorting to ﬁnding commonalities among

sub-concepts in a disjunction, also known as the

least common subsumer (lcs) problem (Turhan, 2007;

Baader et al., 1998).

We deﬁne the notion of similarity measure be-

tween two ALCH concept descriptions as follows:

Deﬁnition 6 (ALC H Concept Similarity). Let X,Y

be ALC H concept descriptions. The degree of sim-

ilarity between X and Y, in symbols sim

ALCH

(X,Y),

is deﬁned as:

sim

ALC H

(X,Y) :=

sim

ALE H

(ALEH -approx(X),ALEH -approx(Y))

An analogous idea can be employed to compute

concept similarity in another DLs and yet using an-

other similarity measure. For instance, it is possible

to approximate ELU-concept descriptions (EL ex-

tended with disjunction) and then compute similar-

ity using the known measure for EL (Lehmann and

Turhan, 2012; Suntisrivaraporn, 2013). It remains

however to be shown whether this produces accept-

able similarity results in practice.

6 RELATED WORKS

The subject of concept similarity has been widely

studied. The techniques can be roughly classiﬁed into

two main groups: a structure-based approach and an

edit-distance-based approach.

In (Distel et al., 2014), the authors introduced a

new framework of concept similarity measure. This

framework is based on a counting of relaxation oper-

ations. A similarity is deﬁned by means of the dis-

tance between concept descriptions C and D, i.e. the

number of times D needs to be relaxed before it sub-

sumes C. The method is claimed to satisﬁed several

properties of concept similarity but has not yet been

implemented.

A measure proposed by (Ge and Qiu, 2008) cal-

culates a degree of similarity based on the depth of

a concept deﬁned in different levels of the ontolog-

ical hierarchy. The method considers the distance

relationship (subsumption relation) between concepts

and assigned different weights to the role depth. The

degree of similarity between two concepts was mea-

sured by means of a distance (a propagation of all la-

bel weights) to their least common subsumer. Simi-

lar approaches were proposed in (Ge and Qiu, 2003;

Giunchiglia et al., 2007). Despite their usefulness in

structural analysis, these methods were fully relied on

an ontology hierarchy and usually ignored constraints

of concept deﬁnitions in the ontology.

A simple method for similarity measure in the ba-

sic DL L

(i.e. no use of roles) was proposed in (Jac-

card, 1901), known as Jaccard Index). An extension

thereof to the DL ELH was proposed in (Lehmann

and Turhan, 2012). The extended work suggested

a new framework that satisﬁes several properties for

similarity. While the framework is deﬁned in general,

the functions and operators needed for the computa-

tion are parameterized and thus left to be speciﬁed.

Moreover, the framework does not contain implemen-

tation details.

ICAART 2016 - 8th International Conference on Agents and Artiﬁcial Intelligence

210

The notion of homomorphism degree was origi-

nally introduced in (Suntisrivaraporn, 2013) and em-

ployed as the heart of the similarity measure for the

DL EL. This has been extended to ELH and con-

tinuously studied in (Tongphu and Suntisrivaraporn,

2014; Tongphu and Suntisrivaraporn, 2015).

Racharak and Suntisrivaraporn suggested two new

notions of similarity for the DL F L

(Racharak and

Suntisrivaraporn, 2015). Both the skeptical and cred-

ulous similarity measures are derived from the known

structural characterization subsumption through in-

clusion of regular languages.

The similarity measure presented in this paper is

similar to those reported in (Tongphu and Suntisri-

varaporn, 2014; Suntisrivaraporn, 2013). It however

focuses on the strictly more expressive DL and em-

ploys generalizations of the normalization and char-

acterization from (Baader and K¨usters, 2006).

7 DISCUSSIONS AND FUTURE

WORKS

This paper presents a new notion of concept similarity

for the DL ALEH w.r.t. an unfoldable terminology

and suggests a way to approximate concept similarity

for the more expressive ALC H . At the heart of the

measure is the calculation of the degree of homomor-

phism to and from between two description trees. To

allow this, we ﬁrst review and extend the known nor-

malization and homomorphism to take into account

also role hierarchy axioms. The proposed similarity

measure can be regarded as an extension of the sim-

ilarity measure sim for the EL family (Suntisrivara-

porn, 2013; Tongphu and Suntisrivaraporn, 2015).

There are various directions for future works. One

could try to evaluate the proposed measure on appro-

priate ontologies from real-world domains. Similar to

the experiments on SNOMED CT reported in (Tong-

phu and Suntisrivaraporn, 2015), a similar setting can

be carried out. Besides, more expressive ontologies

that make use of the universal quantiﬁcation such as

GALEN could be experimented upon. It can be ex-

pected to ﬁnd out new hidden knowledge in the on-

tology that could not have been done before with the

mere standard reasoner. Another useful application

is a measure of similarity between diseases proposed

in (Mathur and Dinakarpandian, 2012). The appli-

cation has shown useful cases in similarity measure

processes underlying each disease for more accurate

unknown disease prediction.

Concerning the choice of representation lan-

guage, it is an obvious future work to explore non-

approximate similarity measure for ALC by investi-

gating under scrutiny into the original tableau algo-

rithm. Another direction for future work could be

to compare the measure presented in this paper to

those two notions of similarity for F L

introduced in

(Racharak and Suntisrivaraporn, 2015). Since F L

is a sub-logic of ALEH and as such sim

ALEH

applicable also to F L

, it is interesting to explore

whether sim

ALEH

is stronger (see (Racharak and

Suntisrivaraporn, 2015)) than the skeptical and cred-

ulous similarity measures.

ACKNOWLEDGEMENTS

This research is partially supported by Thammasat

University Research Fund under the TU Research

Scholar, Contract No. TOR POR 1/13/2558; the Cen-

ter of Excellence in Intelligent Informatics, Speech

and Language Technology, and Service Innovation

(CILS), Thammasat University; and the National Re-

search University (NRU) project of Thailand Ofﬁce

for Higher Education Commission.

REFERENCES

Baader, F. (2003). Terminological cycles in a descrip-

tion logic with existential restrictions. In Gottlob, G.

and Walsh, T., editors, Proceedings of the 18th Inter-

national Joint Conference on Artiﬁcial Intelligence,

pages 325–330. Morgan Kaufmann.

Baader, F., Calvanese, D., McGuinness, D., Nardi, D., and

Patel-Schneider, P., editors (2007). The Description

Logic Handbook: Theory, Implementation and Appli-

cations. Cambridge University Press, second edition.

Baader, F. and K¨usters, R. (2000). Matching in descrip-

tion logics with existential restrictions. In A.G. Cohn,

F. Giunchiglia, and B. Selman, editors, Proceedings of

the Seventh International Conference on Knowledge

Representation and Reasoning (KR2000), pages 261–

272, San Francisco, CA. Morgan Kaufmann Publish-

ers.

Baader, F. and K¨usters, R. (2006). Nonstandard inferences

in description logics: The story so far. In Gabbay,

D., Goncharov, S., and Zakharyaschev, M., editors,

Mathematical Problems from Applied Logic I, vol-

ume 4 of International Mathematical Series, pages 1–

75. Springer-Verlag.

Baader, F., K¨usters, R., and Molitor, R. (1998).

Computing least common subsumers in Descrip-

tion Logics with existential restrictions. LTCS-

Report LTCS-98-09, LuFG Theoretical Computer

Science, RWTH Aachen, Germany. See http://www-

lti.informatik.rwth-aachen.de/Forschung/Papers.html.

Distel, F., Atif, J., and Bloch, I. (2014). Concept dissimi-

larity with triangle inequality. In Proceedings of the

Fourteenth International Conference on Principles of

A Structural Subsumption based Similarity Measure for the Description Logic ALE H

211

Knowledge Representation and Reasoning (KR’14),

Vienna, Austria. AAAI Press. Short Paper. To appear.

Ge, J. and Qiu, Y. (2003). Concept similarity match-

ing based on semantic distance. In Gottlob, G. and

Walsh, T., editors, Proceedings of the Forth Interna-

tional Conference on Semantics, Knowledge and Grid

(SKG 2008), pages 380–383. Morgan Kaufmann.

Ge, J. and Qiu, Y. (2008). Concept similarity matching

based on semantic distance. In SKG, pages 380–383.

IEEE Computer Society.

Giunchiglia, F., Yatskevich, M., and Shvaiko, P. (2007).

Semantic matching: Algorithms and implementation.

Journal of Data Semantics, 9:1–38.

Jaccard, P. (1901).

Etude comparative de la distribution

ﬂorale dans une portion des Alpes et des Jura. Bul-

letin del la Soci´et´e Vaudoise des Sciences Naturelles,

37:547–579.

Lehmann, K. and Turhan, A.-Y. (2012). A framework

for semantic-based similarity measures for ELH -

concepts. In del Cerro, L. F., Herzig, A., and Mengin,

J., editors, JELIA, volume 7519 of Lecture Notes in

Computer Science, pages 307–319. Springer.

Mathur, S. and Dinakarpandian, D. (2012). Finding disease

similarity based on implicit semantic similarity. Jour-

nal of Biomedical Informatics, 45(2):363–371.

Racharak, T. and Suntisrivaraporn, B. (2015). Similar-

ity measures for F L

concept descriptions from an

automata-theoretic point of view. In Information and

Communication Technology for Embedded Systems

(IC-ICTES), pages 1–6. IEEE Computer Society.

Suntisrivaraporn, B. (2013). A similarity measure for the

description logic EL with unfoldable terminologies.

In International Conference on Intelligent Networking

and Collaborative Systems (INCoS-13), pages 408–

413.

Tongphu, S. and Suntisrivaraporn, B. (2014). On desirable

properties of the structural subsumption-based simi-

larity measure. In Semantic Technology - 4th Joint In-

ternational Conference, JIST 2014, Chiang Mai, Thai-

land, November 9-11, 2014. Revised Selected Papers,

pages 19–32.

Tongphu, S. and Suntisrivaraporn, B. (2015). Algorithms

for measuring similarity between elh concept descrip-

tions: A case study on SNOMED CT. Journal of Com-

puting and Informatics. (Accepted in May 2015; To

appear).

Turhan, A.-Y. (2007). On the Computation of Common Sub-

sumers in Description Logics. PhD thesis, TU Dres-

den, Institute for Theoretical Computer Science, Ger-

many.

ICAART 2016 - 8th International Conference on Agents and Artiﬁcial Intelligence

212