Hierarchical Complexity and Aging
Towards a Physics of Aging
Tarynn M. Witten
Center for the Study of Biological Complexity, Virginia Commonwealth University,
PO Box 842030, Richmond, VA 23284-2030 U.S.A.
Keywords:
Acceleration of Aging, Biodemography, Biological Time, Complexity Theory, Chronological Time, Hierar-
chy, Networks, Physics of Aging.
Abstract:
In this paper we extend the previous work of Witten and her team on defining a classical physics driven model
of survival in aging populations (Eakin, 1994; Eakin and Witten, 1995a; Eakin and Witten, 1995b; Witten and
Eakin, 1997) by revisiting the concept of a force of aging and introducing the concepts of a momentum of
aging, a kinetic energy and a potential energy of an aging. As an example of the use of these constructs, we
then explore the implications of these concepts with respect to the (Yu et al., 1982) diet restriction experiments.
1 HISTORY OF RELIABILITY
The history of the demographics of aging is tightly
bound to the field of survival analysis (Witten, 1981;
Elandt-Johnson and Johnson, 1999). Survival analy-
sis, however, emerged from the earlier discipline of
reliability theory (Abdel-Hameed et al., 1984; Ansell
and Phillips, 1994).
The constructs of reliability theory emerged from
the 1950’s gedankt experiments of the computer sci-
entist John Von Neumann. His interest (Neumann,
1956) was in how one would go about building a reli-
able biological organism out of unreliable parts. Until
the thought experiments of von Neumann, the concept
of reliability had not been well-defined.
Von Neumann’s argument proceeded as follows.
He began by defining the concept of the conditional
instantaneous failure rate, denoted by λ(t). We inter-
pret this as follows. The condition is that the failure
has not occurred at time t given that the organism has
survived until time t. With this in mind, we may then
define the reliability R(t) of an organism as the proba-
bility of no failure of the organism before time t. If we
let f (t) be the time to (first) failure (this is the same as
the failure density function), then the reliability R(t)
is given by R(t) = 1 − F(t) where F(t) =
R
t
0
f (τ)dτ
((Abdel-Hameed et al., 1984; Deshpande and Purohit,
2005; Elandt-Johnson and Johnson, 1999; Kalbfleish
and Prentice, 2002; Lawless, 2003)).
How do we actually obtain an equation for the re-
liability R(t)? We do this as follows. Suppose we ask
what is the reliability R(t + ∆t) where ∆t is a small
time increment. In other words, suppose that we know
the reliability of the organism at time t and we want
to know the organism’s reliability at a small time in-
crement ∆t later than time t. In order for the organ-
ism to be operational at time t +∆t, the organism must
have been operational until at least time t and then not
have failed in the time interval (t,t + ∆t). We can ex-
press this mathematically as follows. The reliability
R(t + ∆t) is given by
R(t + ∆t) = R(t) − λ(t)R(t)∆t (1)
Reading equation [1], we see that to be functional (op-
erational) at time t +∆t, the organisms had to be func-
tional at time t (denoted by the reliability term R(t)
on the right hand side of the equation). Next, we have
to subtract out all of the items that failed in the time
interval (t,t + ∆t) (given by the second term on the
right hand side of equation [1]). What remains after
this subtraction is all of the organisms or items that
remain functional at time t + ∆t. A bit of algebraic
rearrangement and we have
R(t + ∆t) − R(t)
∆t
= −λ(t)R(t) (2)
It follows that letting ∆t → 0 (remembering our cal-
culus), Equation [1] becomes the simple differential
equation given by
dR(t)
dt
= −λ(t)R(t) (3)
Witten, T.
Hierarchical Complexity and Aging - Towards a Physics of Aging.
In Proceedings of the 1st International Conference on Complex Information Systems (COMPLEXIS 2016), pages 143-154
ISBN: 978-989-758-181-6
Copyright
c
 2016 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved
143

Thus, if we can specify the form of the function λ(t),
we can solve for R(t)(Roberts, 2010). The literature
in these fields often uses the term “failure rate func-
tion” interchangeably with the term “hazard” func-
tion.
The earliest gerontological papers that made use
of reliability theory and its application to aging fo-
cussed on two application areas, genetic and gen-
eral network theoretic applications (Doubal, 1982;
Gavrilov and Gavrilova, 2001; Witten, 1984a; Wit-
ten, 1984b). The discipline of reliability theory, cou-
pled with network analysis/graph theory, has subse-
quently opened a number of direct conceptual appli-
cations of the field to the genetics of aging/longevity
(Carnes et al., 2006; Witten, 1984c; Witten, 2007;
Witten and Bonchev, 2007; Managbanag et al., 2008)
and more recently (Witten, 2014; Wimble and Witten,
2014). This is because concepts of reliability have
direct analogs to the longevity and lifespan of an or-
ganism. The most obvious one is that lifespan can be
thought of as ”the time to failure” of an organism. If
death can be viewed as a failure, then there is a natu-
ral linkage between survival and reliability. Thus, the
ideas of reliability mutated and the mutation became
what we now know as the field of survival theory.
2 FROM RELIABILITY TO
SURVIVAL
The field of survival analysis takes the term R(t) and
recognizes that it is describing the equivalent concep-
tual construct of “survival,” denoted S(t), where S(t)
represents the probability of a living organism surviv-
ing until at least age t (or age a). Following the argu-
ments in the previous section, equation [3] becomes
dS(t)
dt
= −µ(t)S(t) (4)
where µ(t) is the instantaneous conditional probabil-
ity of dying in the interval (t,t + ∆t) given that the or-
ganism has survived until at least time t. Because we
have a differential equation, we need an initial condi-
tion. We usually assume that S(0) = 1 because there
is no possibility of immediate death.
The solution to Equation[4] can be found in dif-
ferential equation textbooks (Roberts, 2010). The so-
lution of which becomes
S(t) = e
R
t
0
µ(τ)dτ
(5)
where, as before S(0) = 1. In the case where µ(τ) =
h
0
e
γτ
, S(t) becomes the traditional two-parameter
Gompertz survival distribution (Carnes and Olshan-
sky, 1997).
S(t) = e
h
0
γ
(1−e
γt
)
(6)
And, in the case where µ(τ) = h
0
e
γτ
+ M, we obtain
the traditional three-parameter Gompertz-Makeham
survival distribution (Carnes et al., 2006).
S(t) = e
−Mt
e
h
0
γ
(1−e
γt
)
(7)
This history and importance of these models are ex-
tensively discussed in the various works of Carnes
(see references). Thus, the tie between the constructs
of reliability theory and those of survival theory is
both well-established historically and is also concep-
tually useful.
3 SURVIVAL, AGING,
LONGEVITY AND BEYOND
Categorizing and comparing the behavior of differ-
ent demographic survival curves has long been of in-
terest to many disciplines. Life tables are important
in all manner of disciplines (Deshpande and Purohit,
2005; Chiang, 1984; Keyfitz, 1977). The literature
in this field is large and beyond the scope of this ar-
ticle to review. As with most disciplines, over time
each discipline develops its own jargon. The demog-
raphy of aging borrowed from the field of reliability
theory(Witten, 1981; Doubal, 1982). Subsequently
the fields of demography and biodemography of ag-
ing made use of terminology from mathematics and
physics to describe aging processes. Phrases like, “the
force of mortality” or “acceleration of aging” abound
in this literature. And yet, the interpretations of these
phrases bear little resemblance to their original mean-
ings in classical physics; serving more as anecdotal
descriptors rather than exactly quantifiable constructs.
Similar comments may be made around other sur-
vival/failure constructs that will be described in later
sections of this paper.
These conceptual disconnects inspired Witten and
her team (Witten, 1989) to develop variables and def-
initions that are more true to their physics origins.
By doing so, they hoped to provide a better sense of
what these quantitative metrics were describing. Con-
sider, for example, the physics concepts of velocity
and how to use it to quantify absolute and relative sur-
vival differences between species. In (Witten, 1989)
the author proposed formal classical physics rigor-
ous definitions for the terms “velocity” - denoted v(a)
and “acceleration” - denoted α(a) of aging. Witten
also defined the absolute and relative differences be-
tween two groups (species). The idea of being able to
compare between species or to extrapolate from one
species to another is an old one. For example, Carnes
COMPLEXIS 2016 - 1st International Conference on Complex Information Systems
144

et al.(Carnes et al., 1998; Carnes et al., 2003; Hoel
et al., 2005) examined the problem of lifespan extrap-
olation from nonhuman organisms to humans during
radiation exposure.
In (Eakin, 1994; Eakin and Witten, 1995a; Eakin
and Witten, 1995b; Witten and Eakin, 1997) the au-
thors extended their physics of aging definitions to in-
clude a “distance” metric of aging, denoted x(a) that
could be used to compare different species on a com-
parable time scale. This method involved comparing
nomograms based upon the x(a) for each species.
3.1 Terminology
In this paper, we will return to the question of devel-
oping a set of physically consistent constructs, draw-
ing upon the ideas of classical Newtonian physics,
motivated by the terms in the biogerontology of ag-
ing. Moreover, we want these constructs to be able to
be used to more clearly describe the dynamics of an
aging cohort of biological organisms. In this paper
we will begin by making use of a number of con-
cepts drawn from traditional classical physics; i.e.,
distance, velocity, force. In order to assist the reader
in understanding the conceptual development, we will
always attempt to provide a non-physics explanation
of each construct that we develop. In the next section
we talk about the concept of “extrinsic gerontologi-
cal distance” from which we derive the concepts of
velocity and an acceleration. We begin by discussing
the modifiers “extrinsic” vs. “intrinsic” of these and
other variables.
3.1.1 The Extrinsic vs. the Intrinsic Perspective
The challenge in understanding survival and mortality
data is that different organisms do not “experience”
the “aging” process in the same fashion. We are all
familiar with the idea of a seven year-old dog being
sixty-three in human years. This difference in the ex-
perience of the aging process is termed “extrinsic” ag-
ing; we have not scaled the experiences so that they
can be compared on a common experiential scale.
How do we know that one human year is 6 − 7 dog
years? The two organisms do not necessarily share
the same “intrinsic” aging experience. If we can find
a way to map one experience of aging onto the other,
we then have aligned the experiences in such a way
as to show how they are related. We call this common
experience an “intrinsic” relationship between the or-
ganisms. In this paper we will demonstrate one rigor-
ous methodology for constructing that mapping (re-
lationship) and we will then show you how it can be
used.
3.2 Brief Review of Past Work
The biodemographic and general aging literature of-
ten makes use of such terms as the“acceleration of ag-
ing” and the “velocity of aging.” As we have already
pointed out, these terms are loosely, not rigorously
defined constructs and serve rather as a set of con-
ceptual ideas that allow the reader to develop a sense
of how fast a population is dying off and whether or
not one population is dying off faster than another. In
fact, based upon the past literature in the field, there is
really no actual way to calculate either of these con-
ceptual constructs; they are simply nothing more liter-
ary descriptors. This motivated Witten and her team
set out to develop ways to make those calculations
and to do so in a rigorous and conceptually consis-
tent fashion. Since velocity and acceleration are just
time-derivatives of distance, we being by looking at
the problem of deriving the extrinsic organismal dis-
tance.
3.2.1 Defining the Extrinsic Organismal
Distance, Velocity and Acceleration
Witten(Witten, 1989) and her team demonstrated one
possible way of defining both an “extrinisic velocity”
and an “extrinsic acceleration” of aging in terms of
the formal physics definitions of these quantities. In
addition to the velocity and acceleration of aging, they
demonstrated how one could construct a “geronto-
logical distance.” The “gerontological distance” x(a)
can be seen as the “distance” an organism has trav-
eled down its hypothetical organismal lifeline by age
a. This set of definitions allowed them to provide a
complete initial formulaic grounding of velocity and
acceleration in terms of traditional physical variables,
not literary descriptors.
In Eakin(Eakin, 1994) and Eakin & Witten(Eakin
and Witten, 1995a; Eakin and Witten, 1995b) the au-
thors demonstrated that it was indeed possible to de-
fine an equivalent “extrinsic distance” metric so that
if we denote the extrinsic gerontological distance at
age a by x(a), then the following physical definitions
would be true:
x(a) = extrinsic gerontological distance (8)
v(a) =
dx(a)
da
= extrinsic velocity (9)
α(a) =
dv(a)
da
= extrinsic acceleration (10)
These follow because, given a “distance” measure and
remembering our basic physics definitions, the veloc-
ity is the rate of change of distance and the accelera-
tion is the rate of change of the velocity.
Hierarchical Complexity and Aging - Towards a Physics of Aging
145

3.2.2 Deriving the Organismal Distance,
Velocity and Acceleration
Once we have the extrinsic distance x(a), we can con-
struct the extrinsic velocity v(a) and the extrinsic ac-
celeration α(a). Remembering that velocity is rate of
change of distance and using the fact (see Eakin &
Witten(Eakin and Witten, 1995a; Eakin and Witten,
1995b)) that
x(a) =
Z
a
0
λ(ζ)dζ, (11)
we can show that the extrinsic velocity of aging is
given by v(a) and that the extrinsic velocity of aging
can be quantitatively measured as v(a) = λ(a) where
λ(a) is just the traditional “mortality rate” of the pop-
ulation being studied.
v(a) ≡
dx(a)
da
=
d
da
Z
a
0
λ(ζ)dζ = λ(a) (12)
Thus, the extrinsic velocity of aging now has a rigor-
ous physical meaning. In fact, the extrinsic velocity
of aging is just the traditional mortality rate λ(a) of
a population. It follows from this fact that the extrin-
sic acceleration of aging is just the rate of change of
extrinsic velocity and therefore the extrinsic acceler-
ation of aging is the rate of change of the traditional
mortality rate.
α(a) ≡
dv(a)
da
=
dλ(a)
da
(13)
We now have a formal way to calculate the “accel-
eration of aging.” The construct of a mathematical
acceleration was used in (Carnes and Witten, 2013)to
show that after a certain point in the human lifespan,
there is actually a real acceleration in the how fast a
population of individuals travels through their lifes-
pan. Throughout the remainder of this paper we will
use mortality rate and extrinsic velocity of aging in-
terchangeably.
As we have seen, given the mortality rate function
λ(a), we can calculate x(a), v(a) and α(a). Eakin &
Witten(Eakin and Witten, 1995a; Eakin and Witten,
1995b) also demonstrated that if S(a) is the extrinsic
survival fraction (denoted S(a), and if a represents the
extrinsic chronological age, then the “extrinsic geron-
tological distance” x(a) is related to the extrinsic sur-
vival fraction S(a) of the population via the following
relationship
x(a) =
Z
a
0
λ(ζ)dζ = ln

1
S(a)

= − ln [S(a)] (14)
Observe that Equation[14] is independent of the spe-
cific extrinsic survival function S(a) in that any sur-
vival distribution S(a) can be used for S(a) in the for-
mula (Weibull, Gompertz-Makeham, etc.). Moreover,
modifications to such models - for example, addition
of frailty distributions, etc. do not alter the mathemat-
ical construction of the physical variables nor do they
alter their meaning from a classical physical perspec-
tive.
This completes our core requirements for a basic
physics of survival in that the appropriate derivatives
of the gerontological distance x(a) yield the usual
physics literature definitions of both the extrinsic ve-
locity of aging and the extrinsic acceleration of ag-
ing. Finally, we note that given the extrinsic geron-
tological distance x(a) we can compute the extrinsic
survival distribution, which is given by the following
equation
S(a) = exp[−x(a)] (15)
This relationship makes conceptual sense because, the
farther down the “life distance” x(a) you travel, the
less likely you are to survive. We now address how
to actually calculate these variables and how to use
them.
3.2.3 Computing the Organismal Distance,
Velocity and Acceleration for the Masoro
& Yu Diets
It is also important to note that our physical con-
structions revolve around the use of a parametric
model for the mortality/survival curves. Parameters
for the model must be estimated using some tradi-
tional method such as maximum likelihood estima-
tion (Elandt-Johnson and Johnson, 1999; Lawless,
2003; Kalbfleish and Prentice, 2002). In order to help
us understand these new physical constructs, we will
make use of the data from the original Masoro & Yu
(Yu et al., 1982) data to illustrate the concepts.
Because the Gompertz survival model is so fre-
quently used in the Gerontological literature, we will
use that it as our exemplar parametric survival model
S(t) (any others would also work). The traditional
two-parameter Gompertz mortality and survival mod-
els are given by the following equations
λ(a) = h
0
e
γa
(16)
S(a) = exp

h
0
γ
(1 − e
γa
)

(17)
where h
0
is the mortality rate at age a = 0 and γ rep-
resents the slope of the line in the ln λ(a) vs. a plot
and is ultimately related to the mortality rate doubling
COMPLEXIS 2016 - 1st International Conference on Complex Information Systems
146

time (Table [1], (Finch et al., 1990)). We can see how
sharply the changes in acceleration are occurring by
looking at how the two functions change their curva-
ture (Thomas, 1968). The curvature, denoted κ(a) of
the Gompertz equation, at any age, can be calculated
using the following formula
κ(a) =
γ
3
λ(a)
p
1 + γ
4
λ
2
(a)
(18)
An interesting aside here is that if one were to choose
to use the Gompertz-Makeham mortality rate given
in equation [7] then, while the velocity v
GM
(a) =
v
G
(a) + M, that is the Gompertz-Makeham velocity
is shifted by an amount M, the actual accelerations
α
GM
(a) and α
G
(a) are identical. This suggests that
the addition of the baseline mortality constant M does
not affect the acceleration that the organism popula-
tion undergoes but only the organismal velocity of ag-
ing. Since the Makeham constant is added in order to
describe the “environmental” aging effects, our result
suggests that for the Gompertz-Makeham parametric
model of aging, the Makeham constant merely adds
to the speed (velocity) with which the organismal co-
hort travels across the lifespan timeline, but does not
change the acceleration the cohort undergoes.
3.2.4 Summary of AD vs. DR Results
We summarize our findings as follows. The AD or-
ganisms traverse their life path x(a) faster than do the
DR organisms. The word “faster,” unlike before, can
be quantitatively described by examining the veloc-
ity plots v(a) of the respective organisms. Moreover,
the organisms do not traverse their life paths with a
constant velocity and this suggests that there is an un-
derlying acceleration process α(a) taking place. In
addition, using the concept of curvature κ(a), we can
quantitate the way the organismal acceleration curves
bend and how sharply they bend thereby giving us in-
sight into the way (form) in which the organismal ac-
celeration curves are either similar or different with
respect to how they bend over time. Table [1] pro-
vides the reader with some traditional measures for
the two diet groups. MRD is the mortality rate dou-
bling time as defined in Finch, Pike & Witten(Finch
et al., 1990), the mean lifespan MLS and the inflec-
tion age a
inf
defined in Witten(Witten, 1989). These
will additional values will be useful in an upcoming
section.
Table 1: Parameter Values for Ad libitum(AD) and Re-
stricted Rats (DR) Using A Gompertz Survival Model.
Variable Symbol AD
Gompertz slope (per day) γ 0.010257
Gompertz intercept (per day) h
0
0.00000512
MRD (days)
ln2
γ
67.574
MLS (days) ¯a 685.197
Inflection age (days) a
inf
741.078
Variable Symbol DR
Gompertz slope (per day) γ 0.00487
Gompertz intercept (per day) h
0
0.0002344
MRD (days)
ln2
γ
142.206
MLS (days) ¯a 982.195
Inflection age (days) a
inf
1094.92
4 LOOKING FOR DIFFERENCES
BETWEEN SPECIES
LONGEVITY BEHAVIORS
Given the previous descriptors for longevity dynam-
ics, how might we go about comparing different or-
ganisms? Witten (Witten, 1989) also pointed out that
it is also natural to want to compare longevity dynam-
ics under different living conditions. Hence, we also
wish to compare the extrinsic survival, gerontological
distance, velocity, acceleration or acceleration curva-
ture of one organism Ω
1
at age a
1
against those of
another organism Ω
2
at age a
2
.
4.1 Differences in Survival, Mortality,
Velocity and Distance
In her original paper, Witten (Witten, 1989) defined
the “extrinsic velocity difference” and “extrinsic ac-
celeration difference” of aging. The “extrinsic sur-
vival difference”, for the two diet groups, was de-
fined as S
A
(a) − S
R
(a). However, now that we have
defined the extrinsic gerontological distance, we can
unify the original work of Witten with the work of
Eakin (Eakin, 1994) and Eakin & Witten (Eakin and
Witten, 1995a; Eakin and Witten, 1995b) to demon-
strate how to calculate the extrinsic values of distance
difference, velocity difference and acceleration differ-
ence between the two organisms. The necessary pa-
rameter values are given in Table [1] of this paper.
4.2 Extrinsic Crossing Points of Curves
It is also useful to know whether or not any of
our given pairs of curves cross. Crossing of sur-
vival/mortality curves can have implications in de-
mographic estimation of maximum lifespan based
Hierarchical Complexity and Aging - Towards a Physics of Aging
147

Table 2: Parameter Values for Ad libitum and Restricted
Rats Using A Gompertz Survival Model.
Symbol Value
Survival/distance crossing age (days) 394.64
Mortality/velocity crossing age (days) 282.49
Acceleration crossing age (days) 144.28
upon psycho-socio-economic variables (Hirsch et al.,
2000). The existence of a crossing point for the sur-
vival and the distance functions is a bit more complex
in that they require solving transcendental equations
(Cheney and Kincaid, 1985). For the mortality rate
crossing points, we can demonstrate that the age at
which a pair of mortality rate curves cross is given by
a
mortality
cross
=
1
γ
R
− γ
A
ln

h
0A
h
0R

(19)
Since the physics of our arguments shows that the
mortality rate is just the velocity, the variable a
mortality
cross
is just the crossing of the velocities as well. That is,
a
velocity
cross
= a
mortality
cross
The crossing of acceleration func-
tions can be shown to be given by
a
acceleration
cross
=
1
γ
R
− γ
A
ln

h
0A
γ
A
h
0R
γ
R

(20)
It is interesting to note that we can re-express
a
acceleration
cross
as follows
a
acceleration
cross
= a
velocity
cross
+

1
γ
R
− γ
A

ln

γ
A
γ
R

(21)
From our data in Table[1], it can be shown that
for our two rat groups, the crossings are illustrated in
Table[2].
5 THE PROBLEM OF THE
EXPERIENCE OF TIME
As was discussed in Eakin & Witten (Eakin and Wit-
ten, 1995a; Eakin and Witten, 1995b), the distance
function x(a) is exactly analogous to one side of a
nomogram in which we imagine a line in a two-
dimensional space. The line represents the organ-
ismal lifeline and x(a) represents how far along the
lifeline the given organism has traveled in its life by
chronological age a. When we have two organisms
Ω
1
and Ω
2
, the values x
Ω1
(a) and x
Ω2
(a) represent
two nomogram lines and it is this nomogram struc-
ture that allows us to develop a means by which to
compare the extrinsic ages of different species. This
methodology is discussed, in detail, in Eakin & Wit-
ten (Eakin and Witten, 1995a; Eakin and Witten,
1995b). With this construction in place, we now have
a means by which we can actually determine how old
a fly is, in horse years, or how old a 12 year-old hu-
man female was, in fly years.
While the previous definitions are correct from the
perspective of a physicist, there is a tacit assumption
that time (or the aging process as measured over time)
is experienced in exactly the same way by all of the
organisms being examined. In fact, this is simply not
realistic. Therefore, we need to rework our previous
definitions so that we account for this fact. The flaw
here is that the time units of one organism are not nec-
essarily those of the other organism. We need an “in-
trinsic” measure of distance
We begin by assuming that there is a population
based linear transformation that allows us to trans-
form time scales so that “chronological time” and “bi-
ological time” are linearly related. In other words, we
need to find a way to scale time so that all organisms
are using the same time scale. For now we will ig-
nore the question of chronological time vs. biological
time and assume that the underlying processes of ag-
ing are manifested along a life course trajectory that
can be measured using chronological time. In fact,
this is not an unreasonable assumption as the field of
the demography of aging is based upon this assump-
tion; consider, for example, the typical definition for
the demographic variable n(t,a) which is the number
of individuals of age a in the population at time t (see
work of Von Foerster and others who derive the de-
mographic partial differential equation that describes
n(t, a) (Witten, 1983).
There are many ways that one could approach
scaling or normalizing time. We will use the method
discussed in our work and as presented in Witten &
Eakin (Witten and Eakin, 1997). For the purposes
of discussion and illustration, from this point on we
will use the Gompertzian survival distribution defined
in Equations [16]. However, any parametric distribu-
tion (i.e., Weibull, Gompertz-Makeham), even mix-
tures can be used to replace the Gompertz and our
methodology will still apply.
5.1 Review of the Witten-Eakin
Normalization Method
In Witten & Eakin(Witten and Eakin, 1997) we in-
troduced the following temporal normalization algo-
rithm. If we call a the extrinsic age of the organism,
we can generate what we will call an intrinsic age de-
noted by a and obtained by dividing the extrinsic age
by the life expectancy of the individual at birth. Thus,
COMPLEXIS 2016 - 1st International Conference on Complex Information Systems
148

a would be given by
a =
a
R
∞
0
S(ζ)dζ
(22)
Other normalization methods, interquartile normal-
ization Carnes (Carnes et al., 2006) and inflection time
normalization Witten & Eakin (Witten and Eakin,
1997) have also been demonstrated. Each has its ben-
efits and drawbacks. However, what is interesting is
that our general methodology will work for any of
these normalization methods. Moreover, it is pos-
sible to demonstrate that there is a simple linear re-
lationship between the different normalization trans-
forms so that one can be easily transformed into the
other. Following the arguments in (Eakin and Witten,
1995a; Eakin and Witten, 1995b), we can construct
the intrinsic normalized gerontological distance χ(a)
and demonstrate the formula for a Gompertzian two
parameter distribution Equation[16]. The results are
given by
χ(a) = ln

1
S(a)

(23)
From this, we can derive the intrinsic normalized ve-
locity v(a)
v(a) =
dχ(a)
da
= λ(a) = h
0
e
γa
(24)
and the intrinsic normalized acceleration ς(a) as fol-
lows
ς(a) =
dv(a)
da
= γλ(a) (25)
Again, note that the results equations[23-25], while
applied to the two-parameter Gompertz distribu-
tion, can be applied to any parametric distribution
(Weibull, Gompertz-Makeham, etc.) and can be nor-
malized using any of the methodologies discussed.
5.2 Witten-Eakin Rectangularity
Measures
The concept of survival curve rectangularity has been
discussed by a number of authors (Demetrius, 1977;
Demongeot, 2009; Nagnur, 1986; Pflaumer, 2010).
Witten & Eakin (Witten and Eakin, 1997) demon-
strated how to construct rectangularity and drift mea-
sures for both the intrinsic and extrinsic survival
curves when one was using a parametric model to fit
to the lifespan data. Their methodology is extensively
discussed in Pflaumer (Pflaumer, 2010). Using these
methods, Witten & Eakin were able to demonstrate
that while the U.S. population survival distribution is
rectangularizing, it is also drifting with a simultane-
ous lengthening of mean survival and squaring of the
Table 3: Rectangularity Measure Values for Ad libitum and
Restricted Rats Using A Gompertz Survival Model.
Parameter Symbol
Extrinsic Modal Value (days) m
Extrinsic Elasticity (days) ε
0
Extrinsic Keyfitz Entropy (days) H
Extrinsic Prolate Angle (Degrees) θ
Prolate Rectangularity Index κ
Modified Prolate Angle (degrees) θ
0
Modified Rectangularity κ
0
Parameter AD Value
Extrinsic Modal Value (days) 741.078
Extrinsic Elasticity (days) 684.806
Extrinsic Keyfitz Entropy (days) 8.01079
Extrinsic Prolate Angle (Degrees) −21.4898
Prolate Rectangularity Index 0.930483
Modified Prolate Angle (degrees) 18.9534
Modified Rectangularity ???
Parameter DR Value
Extrinsic Modal Value (days) 1094.92
Extrinsic Elasticity (days) 976.495
Extrinsic Keyfitz Entropy (days) 11.8226
Extrinsic Prolate Angle (Degrees) −28.1145
Prolate Rectangularity Index 0.882008
Modified Prolate Angle (degrees) 25.2246
Modified Rectangularity ???
survival distribution. Table [3] provides the values of
some of these measures for the two diet populations
in this paper.
With this section, we have completed the dis-
cussion of the basic physics. To summarize, using
the survival extrinsic parametric survival distribution
S(a), we have defined an extrinsic distance of aging
x(a), an extrinsic velocity of aging v(a), and an ex-
trinsic acceleration of aging α(a). Extending these
definitions, we have defined extrinsic relative and ab-
solute differences of these functions for two differ-
ent species or diet groups. We have illustrated these
concepts using the original Masoro & Yu (Yu et al.,
1982) ad libitum/diet restriction data. We then made
the argument that the two groups might not experi-
ence “time” in the same way. Consequently, we in-
troduced a number of temporal scaling methods that
might be used to “normalize” the experience of time.
We termed the unnormalized age “extrinsic age” and
the normalized age variable “intrinsic age.” We then
illustrated how all of the previously defined variables
are modified by normalization and we subsequently
compared the normalized ad libitum and normalized
diet restricted variables.
In the upcoming discussion we will continue our
Hierarchical Complexity and Aging - Towards a Physics of Aging
149

definition of physics quantities by looking at how to
define the key concepts of a population mass, a force
of aging, a population momentum, a kinetic energy
and a total energy.
6 EXPANDING THE
POPULATION PHYSICS
DEFINITIONS
6.1 Defining the Mass of a Population
One of the challenges in defining a physics of ag-
ing is that of defining a mass quantity. Nearly ev-
ery physical quantity involves the use of a mass con-
struct; force, momentum, kinetic and potential energy
to name a few. For the moment, we will assume that
we have a single organism and that we may hypothet-
ically assign to that organism what we will call “one
omu” (organismal mass unit). It follows that if there
are N(a) organisms at time a we will denote the total
mass M(a) of the system of organisms as
M(a) = N(a) · 1omu = N
0
S(a) · 1omu (26)
Note that we are treating the population as a homo-
geneous population in which the individual variation
of the masses will not be something with which we
need to concern ourselves. This is not unreasonable
because all parametric models homogenize the popu-
lations with respect to many potentially important fac-
tors. With this mass construct in hand, we may now
extend our physics of aging definitions. The most ob-
vious first choice is to define a population force.
6.2 Defining the Force of a Population
We first consider the “force” construction because the
terminology of a force of mortality has been histori-
cally used in the field of biogerontology. The “force
of mortality” is traditionally defined as follows
µ(a) = −
S
0
(a)
S(a)
. (27)
which we have shown is equivalent to our physical
definition
λ(a) = −
S
0
(a)
S(a)
= −
d
da
ln[S(a)] =
d
da
x(a) = v(a).
(28)
Sadly, while “force of mortality” may be an excellent
conceptual description of µ(a) (old notation) or λ(a)
(new notation), it is not viable measurable “force”
quantity. Using the physics constructions we have
now developed we see that the traditional definition
of “force of mortality” is actually a velocity of aging
and not a force quantity at all.
Using our definition of a mass unit (Equation [26])
and our previous definition for acceleration (Equation
[8]), we observe that the magnitude of the population
force must satisfy F = Mα. Therefore, our force def-
inition becomes
F = M(a)
dv(a)
da
= N
0
S(a)
dλ(a)
da
(29)
Using the Gompertz distribution, as an example,
equation [29] becomes
F = N(a) · γ · λ(a) (30)
= γN
0
S(a)λ(a) (31)
Thus, for the Gompertz distribution, the “force of ag-
ing” is actually given by the following equation:
F = h
0
γN
0
e
γa
e
h
0
γ
(1−e
γa
)
(32)
We provide the complete derivation in Appendix [1].
The obvious question is whether or not the units
are correct for this force definition. We already know
that mass has the correct units. Velocity has units
of distance/time so mass times velocity has the cor-
rect units. Since γ has units of 1/time, multiply-
ing it times mass times velocity gives units of mass ·
distance/second· second which is the correct units for
force (Newtons) F = ma.
It is also possible to show that the maximum force
occurs at age a
F
where a
F
is given by
a
F
=
1
γ
ln
γ
h
0
(33)
This is nothing more than the inflection age (or in-
flection time) discussed in the work of Eakin & Wit-
ten(Eakin and Witten, 1995b). Knowing this, it
is straightforward to demonstrate that the maximum
force F
max
is given by
F
max
= γ
2
N
0
e
h
0
γ
−1
(34)
Let us continue our derivation of the different
physics quantities before we discuss their actual ap-
plication and relevance to biodemography and the bi-
ology of aging.
6.3 Defining Momentum of a Population
We also note that we can immediately obtain a defini-
tion for momentum magnitude p(a) by observing that
COMPLEXIS 2016 - 1st International Conference on Complex Information Systems
150

p = mv which is just
p(a) = M(a)v(a) = M(a)
dx(a)
da
= N
0
S(a)λ(a) (35)
For the Gompertz distribution, p(a) becomes
p(a) = M(a) · v(a) (36)
= h
0
N
0
e
γa
e
h
0
γ
(1−e
γa
)
(37)
= γF(a) (38)
6.4 Defining Kinetic Energy of a
Population
Given that we have mass and velocity, we can now
also construct a population kinetic energy KE. We
remember the traditional definition of kinetic energy
as follows
KE =
1
2
M(a) · v(a)
2
(39)
=
1
2
· N(a) ·

dx(a)
da

2
(40)
If we substitute for the Gompertz distribution vari-
ables, and given that v(a) = λ(a), our kinetic energy
KE =
1
2
N(a) [λ(a)]
2
=
N
0
2
S(a)[λ(a)]
2
(41)
=

N
0
h
2
0
2

e
h
0
γ
(1−e
γa
)
e
2γa
(42)
It is straightforward to show that the maximum value
of the kinetic energy occurs at a
KE
which is given by
a
KE
=
1
γ
ln
γ
h
0
+
1
γ
ln2 (43)
Notice that the first term in this equation is the age
at which the maximum force occurs. Thus, we could
write a
KE
as a
KE
= a
F
+
1
γ
ln2.
6.5 Defining Potential Energy of a
Population
Deriving a potential energy of a population is a bit
more difficult. We begin by hypothesizing that our
force F(a) is the gradient of the potential energy func-
tion V (a) that we wish to determine. We do this
because it would create a conservative function that
would make the physics nicer. In particular, using
our physics analogies, let us suppose that F(a) =
−∇V (a). In one dimension this becomes F(a) =
−dV (a). Thus, if we were to integrate both sides of
the equation, we would obtain a solution for V (a).
Therefore, integrating both sides, we have that
V (a) = −
Z
a
0
F(ζ)dζ +V (0) (44)
where V (0) is the population potential energy at age
a = 0. For the purposes of illustration, we will solve
for the Gompertz potential energy. From equation
[32], we have that F(a) = γN(a)h
0
e
γa
. Integrating this
from age = 0 to age = a we have that
Z
a
0
F(ζ)da = −γh
0
Z
a
0
N(ζ)e
γζ
dζ (45)
= γN
0

exp

h
0
γ
(1 − e
γa
)

− 1

(46)
from which we observe that
V (a) − V (0) = γN
0

exp

h
0
γ
(1 − e
γa
)

− 1

(47)
= −γN
0
+ γN
0
S(a) (48)
∆V = −γN
0
F(a) (49)
where F(a) is the cumulative failure rate and ∆V is
just V (a) − V (0). Solving for V (a), we have that
V (a) = −γN
0
S(a) + [V (0) − γN
0
] (50)
In fact, since V (0) is an arbitrary constant, we can
choose it’s value such that it cancels the γN
0
term
yielding the following final equation for the potential
energy is just
V (a) = γN
0
S(a) (51)
6.6 Defining Total Energy of a
Population
Once we have the kinetic energy KE and the poten-
tial energy V, we can form the total population energy
remembering that TE = KE + V which is given by
TE =
1
2
N(a)

dx(a)
da

2
+
Z
a
0
F(ζ)dζ (52)
For the Gompertz equation, using our previous calcu-
lations, we can rewrite the total energy as
TE =
N
0
2
λ
2
(a)S(a) + γN
0
S(a) = S(a)

N
0
2
λ
2
(a) + γN
0

(53)
which can be simplified to
TE = S(a)N
0

1
2
λ
2
(a) + γ

(54)
All of our derivations are summarized in Table 4.
Hierarchical Complexity and Aging - Towards a Physics of Aging
151

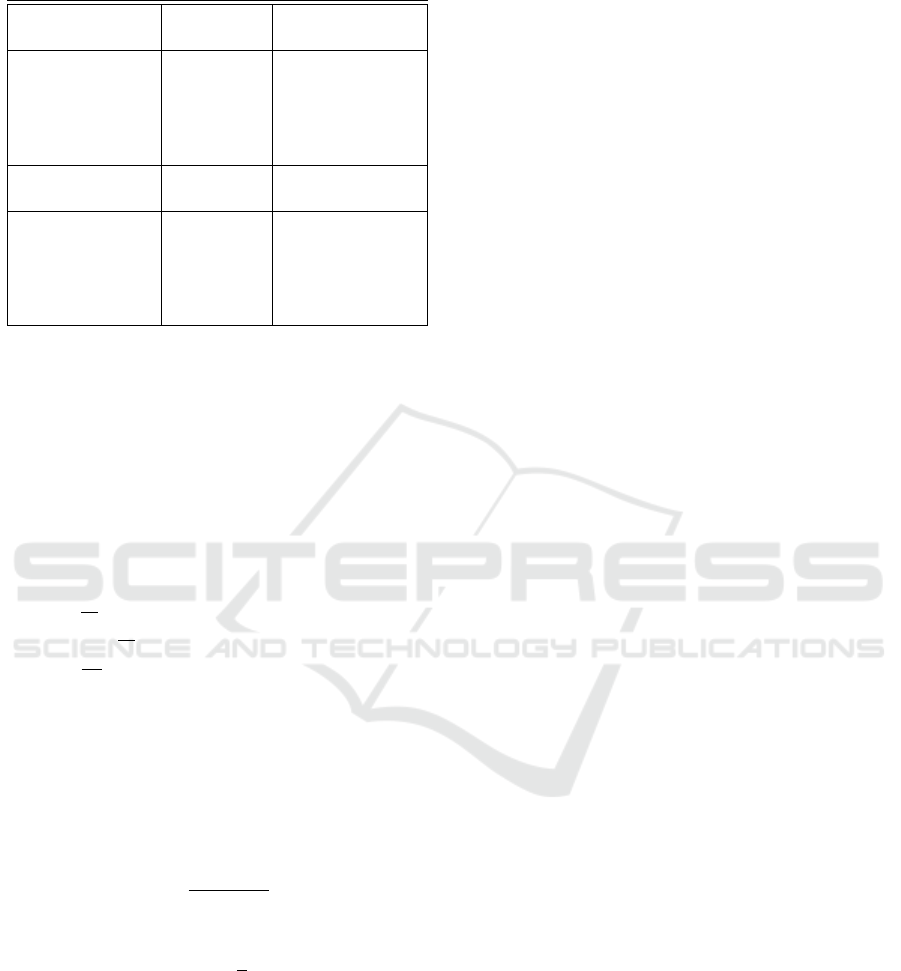
Table 4: Locations and Values of Maxima for each Function
Using the Gompertz Model.
Variable Age of AD AD
Maximum Maximum Value
Force 741.08 1.27 ∗ 10
−9
Momentum 741.08 0.004
Kinetic Energy 808.65 5.56
Potential Energy 953 0
Total Energy 953 0
Variable Age of RD RD
Maximum Maximum Value
Force 1094.92 8.78 ∗ 10
−6
Momentum 1094.92 0.002
Kinetic Energy 1237.12 0.28
Potential Energy 1435 0
Total Energy 1435 0
6.7 Addressing the Problem of Different
Initial Population Sizes N
0
Observe that the previous physical variable defini-
tions contain the parameter N
0
. This makes it diffi-
cult to compare populations with different initial pop-
ulation sizes, when working with our newly defined
physics variables. To address this problem, we de-
fine the normalized variable of interest by dividing
through by N
0
. For example, the normalized force F
would be
F
N
0
. Similarly, we define the normalized mo-
mentum P as
p
N
0
, the normalized kinetic energy K E
would be
KE
N
0
and the normalized total energy T E and
normalized potential energy V would be defined in a
similar fashion.
If we now substitute for the Gompertz distribution
equivalent variables, we have that the following re-
sults
F = γS(a)λ(a) (55)
P = S(a)λ(a) (56)
K E =
S(a)λ(a)
2
2
(57)
V = γS(a) (58)
T E = S(a)

1
2
λ
2
(a) + γ

(59)
In plotting the actual figures, it is easy to see that
not only is the kinetic energy higher in the ad libi-
tum group, but that it peaks much earlier in the ad
libitum group as well. Note that, as we did with the
arguments around survival, we can make the same ar-
guments for the newly defined variables and ask about
their intrinsic vs. extrinsic behaviors.
7 CONCLUSIONS
In this work, we have derived a large number of the
remaining basic constructs for a classical physics in
support of the biodemographics of aging. We have
demonstrated how these constructs are internally con-
sistent, from a physics perspective. Moreover, we
have also demonstrated how they provide useful addi-
tional insights into the dynamics of population biody-
namics. Lastly, using the concepts developed in this
paper, we have proposed a formal rigorous definition
for the lethality of any intervention when measured
against a control group.
ACKNOWLEDGEMENTS
One is lucky to have one’s career touched by a sin-
gle giant in the field. I have been more than lucky,
perhaps blessed would be a better choice of words,
as I have been able to interact with a number of indi-
vidual giants across multiple disciplines. Moreover, I
have been lucky to walk with some and to stand on the
shoulders of many. A number of specific individuals
have contributed the the evolution of this work effort.
When I was a young postdoctoral student at the Uni-
versity of Southern California, Bernard Strehler en-
couraged me to use my physics background to look at
assorted problems in the field of the demography of
aging. He encouraged me to use mathematical mod-
eling and computer simulation to study the dynamics
of population aging; the first person I met as a young
scientist, who believed in and published the first ar-
ticles in this series. I would especially like to thank
Edward Masoro and B.P. Yu both of whom who took
an interest in my ideas, when I was just beginning my
survival theory of aging research. They generously
shared their early mouse and rat data (Yu et al., 1982)
and for made time, in their respectively busy sched-
ules, for many hours of time spent in teaching a physi-
cist about the biology of aging. Leonard Hayflick and
George Martin spent equal amounts of time teaching
an older scientist about the ongoing research in the
biology of aging. Caleb Finch and Thomas Johnson
tuffed it out as I worked through many of my calcu-
lations, making many biological errors in my formu-
lations. Their patience is more appreciated than they
know. I would like to thank my former postdoctoral
student Tim Eakin for his contributions to the ongoing
development of this effort by introducing the scaling
methods in (Eakin, 1994) and for working with me on
(Eakin and Witten, 1995a; Eakin and Witten, 1995b;
Witten and Eakin, 1997). Marilyn Bishop has been
my right hand in debugging the Mathematica code
COMPLEXIS 2016 - 1st International Conference on Complex Information Systems
152

needed to generate the complex graphics for this pa-
per. And lastly, I would like to thank my friend, col-
league and co-author Bruce Carnes who has consis-
tently believed in my approach. Who could ask for
better friends and colleagues than that.
REFERENCES
Abdel-Hameed, M., Cinlar, E., and Quinn, J. (1984). Relia-
bility Theory and Models: Stochastic Failure Models,
Optimal Maintenance Policies, Life Testing and Struc-
tures. Academic Press, Inc., Orlando, FL.
Ansell, J. and Phillips, M. (1994). Practical Methods for
Reliability Data Analysis. Clarendon Press, Oxford,
England.
Carnes, B. and Olshansky, S. (1997). A biologically moti-
vated partitioning of mortality. Experimental Geron-
tology, 32(6):615–631.
Carnes, B. and Witten, T. (2013). How long must humans
live. J. Gerontology Biol. Sci. Med. Sci., 69A(8):965
– 970.
Carnes, B. A., Grahn, D., and Hoel, D. (2003). Mortality
of atomic bomb survivors predicted from laboratory
animals. Radiat. Res., 160(2):159 – 167.
Carnes, B. A., Holden, L. R., Olshansky, S. J., Witten,
M. T., and Siegel, J. S. (2006). Mortality partitions and
their relevance to research on senescence. Biogeron-
tology, 7(4):183 – 198.
Carnes, B. A., Olshansky, S. J., and Grahn, D. (1998). An
interspecies prediction of the risk of radiation-induced
mortality. Radiat. Res., 149(5):487 – 492.
Cheney, W. and Kincaid, D. (1985). Numerical Mathemat-
ics and Computing. Brooks Cole, Monterey, CA.
Chiang, C. (1984). The Life Table and its Applications.
Robert E. Krieger Publishing, Co., Malabar, FL.
Demetrius, L. (1977). Measures of fitness and demographic
stability. Proc. Natl. Acad. Sci. USA, 74(1):384 – 386.
Demongeot, J. (2009). Biological boundaries and biological
age. Acta Biotheoretica, 57(4):397 – 418.
Deshpande, J. and Purohit, S. (2005). volume 11 of Quality,
Reliability and Engineering Statistics. World Scien-
tific, Hackensack, NJ.
Doubal, S. (1982). Theory of reliability, biological systems
and aging. Mech. Aging and Dev., 18(4):339 – 353.
Eakin, T. (1994). Intrinsic time scaling in survival analy-
sis: Application to biological populations. Bull. Math.
Biol., 56(6):1121 – 1141.
Eakin, T. and Witten, T. (1995a). A gerontological distance
metric for analysis of survival dynamics. Mech. Aging
and Dev., 78:85 – 101.
Eakin, T. and Witten, T. (1995b). How square is the survival
curve of a given species? Experimental Gerontology,
30(1):33 – 64.
Elandt-Johnson, R. and Johnson, N. (1999). Survival Mod-
els and Data Analysis.
Finch, C. E., Pike, M. C., and Witten, T. M. (1990). Slow
mortality rate accelerations during aging in some ani-
mals approximate that of humans. Science, 249:902 –
905.
Gavrilov, L. and Gavrilova, N. (2001). The reliability theory
of aging and longevity. J. theor. Biol., 213(4):427 –
445.
Hirsch, H., Liu, X., and Witten, T. (2000). Mortality rate
crossovers and maximum lifespan in advantaged and
disadvantaged populations: Accelerated mortality and
sudden death models. J. theor. Biol., 205(2):171 – 180.
Hoel, D., Carnes, B., Dedrick, R., Fry, R., Grahn, D., Grif-
fith, W., Groer, P., and Preston, R. J. (2005). Extrap-
olation of radiation-induced cancer risks from nonhu-
man experiment systems to humans. Technical report,
National Council on Radiation Protection and Mea-
surements, Bethesda, MD.
Kalbfleish, J. D. and Prentice, R. (2002). The Statistical
Analysis of Failure Time Data. John Wiley and Sons,
New York, N.Y.
Keyfitz, N. (1977). Applied Mathematical Demography.
John Wiley and Sons, Inc., New York, N.Y.
Lawless, J. (2003). Statistical Models and Methods for Life-
time Data. John Wiley and Sons, Inc., Hoboken, N.J.
Managbanag, J., Witten, T., Bonchev, D., Fox, L.,
Tsuchiya, M., Kennedy, B., and Kaeberlein, M.
(2008). Shortest-path network analysis is a useful
approach towards identifying genetic determinants of
longevity. PLoS ONE, 3(11):e3802.
Nagnur, D. (1986). Rectangularization of the survival curve
and entropy: The Canadian experience 1921 - 1981.
Canadian Studies in Population, 13(1):83 – 102.
Neumann, J. V. (1956). Probabilistic logics and the synthe-
sis of reliable organisms from unreliable components.
Princeton University Press, Princeton, N.J.
Pflaumer, P. (2010). Measuring the rectangularization of
lifetables using the Gompertz distribution. Vancou-
ver, Canada. Proc. Joint Statistical Meetings, Social
Statistics Section.
Roberts, C. (2010). Ordinary Differential Equations: Ap-
plications, Models and Computing. Chapman and
Hall/CRC Press, Boca Raton, FL.
Thomas, G. (1968). Calculus and Analytic Geometry. Ad-
dison Wesley Publishing, Co., Reading, MA.
Wimble, C. and Witten, T. (2014). Modeling aging net-
works: Part2 - Applications. Karger Press, New York,
N.Y.
Witten, T. (1981). Investigating the aging mammalian sys-
tem: cellular levels and beyond. In Proc. 25th Annual
Meeting of the Society for General Systems Research,
pages 309 – 315.
Witten, T. (1983). On stochasticity in the von Foerster hy-
perbolic partial differential equation system. Further
applications to the modeling of an asynchronously di-
viding cellular system. Computers and Mathematics
with Applications, 9(3):447 – 458.
Witten, T. (1984a). A return to time, cells, systems and
aging: II. Relational and reliability theoretic aspects
of senescence in mammalian systems. Mech. Aging
and Dev., 27:323 – 340.
Witten, T. (1984b). Mathematics of molecular aging. Aca-
demic Press, New York, N.Y.
Hierarchical Complexity and Aging - Towards a Physics of Aging
153

Witten, T. (1984c). Time aberration in living organisms:
stochastic effects. Math. Modeling, 5:97 – 101.
Witten, T. (1989). Quantifying the concepts of rate and
acceleration/deceleration of aging. Growth, Develop-
ment and Aging, 53:7 – 16.
Witten, T. (2007). (M,R)-systems, (P,M,C)-nets, hi-
erarchical decay and biological aging: Reminis-
cences of Robert Rosen. Chemistry and Biodiversity,
4(10):2332 – 2344.
Witten, T. (2014). Modeling aging networks: Part 1 - Intro-
duction to the theory. Karger Press, New York, N.Y.
Witten, T. and Bonchev, D. (2007). Predicting
aging/longevity-related genes in the nematode C. el-
egans. Chemistry and Biodiversity, 4:2639 – 2655.
Witten, T. and Eakin, T. (1997). Multiphasic models of sur-
vival: Background and early developments. Experi-
mental Gerontology, 32(2):259 – 285.
Yu, B., Masoro, E., Murata, I., Bertrand, H., and Yu,
F. (1982). Lifespan study of SPF Fisher 344 male
rats fed ad libitum or restricted diets: longevity,
growth, lean body mass and disease. J. Gerontology,
37(2):130 – 141.
COMPLEXIS 2016 - 1st International Conference on Complex Information Systems
154