
Suremath
User Study and Related (Re-)Implementation of a Multitouch Application for
Learning Math
Georg J. Schneider and Immanuel Ubl
Dept. of Computer Science, University of Applied Sciences Trier, Schneidershof, Trier, Germany
Keywords: User Study, e-Learning Hardware and Software, Multi-platform Multi-touch Application, e-Learning
Application for Mathematics.
Abstract: Learning of the relation between mathematical effects and the underlying formula is a huge step for learners,
which are used to execute rather basic arithmetic calculations up to this point. This usually happens in high
school and pupils are often overstrained by the amount of abstraction which is required. In order to help
students to overcome this gap, we have developed an application for a multitouch table. The pupils are able
to grasp and move function graphs and see how the parameters of the formula change immediately. We have
claimed that this approach leads to a better and faster understanding of the facts to be learned. In order to
evaluate our approach we have carried through several workshops with a focus group and a small user study
with pupils at the relevant age. In this paper we will describe the findings and we will shortly sketch the
resulting (re-) implementation of our system.
1 INTRODUCTION
Learning mathematical facts is a hard task for many
students. Whereas basic mathematic knowledge more
or less still reflects the daily life. More complex and
abstract features appearing in the pre-calculus
curriculum are hard to match with daily topics.
To help learners to get a better understanding of
the more theoretical concepts we have developed a
multitouch table application, where they can interact
with the mathematical concepts in a natural way,
nevertheless providing a link to the underlying
concept. They can move function graphs using their
fingers by touching and dragging on that table. In
parallel they can observe the changes of the
parameters of the belonging equations in real time
(Blanke and Schneider 2011). Furthermore the
application provides an integrated exercise module.
The system has two application scenarios. First, it
shall add another view and another media to the
regular class. There should be a change between
regular teaching methods with pen and paper and the
multitouch application. We also imagine that pupils
should have time to experiment with the application
on their own for a certain period of time in order to
verify their concepts or to discover correlations.
Second, the pupils shall work independently with the
application at home using the built-in exercise mode
for exam training.
The evaluation of our application in a real world
setting in order to figure out if we can prove that the
application leads to better results in learning these
specific topics was another goal. Therefore we have
carried out several workshops with a focus group and
a small user study with pupils and their teacher.
Having only a relatively small group of participants,
we have focussed on finding hints for improving our
system and its acceptance among learners, which we
wanted to integrate afterwards.
In the following we will describe the study and the
workshop we have carried through as well as the
results.
Finally we have deduced different factors to
support the aspects identified in the studies and
incorporated the results into our application. This has
led to a complete reimplementation of the system
since these features could not be incorporated into the
current system.
Accordingly, we will describe our new approach
at the end of this paper.
198
Schneider, G. and Ubl, I.
Suremath - User Study and Related (Re-)Implementation of a Multitouch Application for Learning Math.
In Proceedings of the 8th International Conference on Computer Supported Education (CSEDU 2016) - Volume 1, pages 198-204
ISBN: 978-989-758-179-3
Copyright
c
2016 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved

2 RELATED WORK
First of all, we have to ask the question if at all, there
is a hint that computer supported learning leads to
better results. (Means et. Al. 2009) conducted a meta-
analysis of 46 studies in the area of online learning,
incorporating different learning techniques, like
blended learning. One of the main effects they
discovered was, that classes with online learning
(including blended learning) produce stronger student
learning outcomes than classes with solely face-to-
face instruction.
Novel user interfaces based on multitouch tables
in the field of mathematics are presented in (Zeleznik
et al 2010). The application supports the manipulation
of formulas using gestures on the device. A small
prototype evaluation with students of their university
indicated acceptance of the prototype.
Similar applications like our system are
"GeoGebra" (Hohenwarter, Fuchs, 2004) and
"Cinderella" (Kortenkamp, Richter-Gebert, 2002).
However the interaction is more complicated
compared to our system and we strongly believe that
integrated exercises are a very important feature for
learners for self-assessment.
(Iijima 2012) presents a math application based
on HTML5, which hence runs on many output
devices. Therefore popular devices for students as
tablets can be supported as well.
Even though the systems above reflect different
aspects of the field of interactive multitouch math
learning applications there is not yet a clear picture,
which features must be integrated in order to build a
successful application.
3 THE APPLICATION
Our multitouch application consists of a display area,
where the function graph is displayed (see fig. 1 right
part), an equation area, where the equation is
displayed (see fig. 1 left upper part) and an exercise
area, where the exercises are displayed (see fig. 1 left
lower corner).
The students can create mathematical objects by
drawing the shape of the object with their fingers on
the table. When the object is recognized, the
belonging equation is displayed as well. Afterwards
they can move the objects by touching and dragging
and observe how the parameters in the equation
change in real time. Doing so, they can explore the
topics and try to detect the interrelation on their own.
The exercises serve as a means for the students to
check on their own if the concepts have been
understood. The use of a traffic light symbol tells
them if the exercise has been completely, partially or
not at all completed correctly.
Figure 1: The user interface of the multitouch application.
Suremath - User Study and Related (Re-)Implementation of a Multitouch Application for Learning Math
199

4 STUDY DESIGN
In this section we will quickly sketch the design of
our user study and the workshops with a focus group
we have carried through.
4.1 Study Method and Participants
For the study design we have selected 9 pupils from a
local high school for girls at the age between 13 and
14 years. The topics we have selected for the study
were about quadratic equations and parabolas. The
topics have not been taught at school yet. Therefore,
all pupils have were new to the topics that were part
of the study.
Our hypotheses were that:
(A) Pupils will be able to solve the exercises
faster with the multitouch application
compared to pen and paper
(B) Pupils gain a better understanding of the
topic so that they perform better at the pen
and paper exercise compared to the group
that did not work with our application before
the test.
The study started with a short introduction to the
field, explained by one of their teachers.
Then the group has been split up into two groups,
A and B. Both groups had to perform similar tasks.
However in the first round, group A started to work
on an exercise concerning moving a parabola along
the x-axis and determine the effects on the parameters
in the equation and vice versa, using pencil and paper,
whereas group B used the multitouch table
application for this task.
Then the groups switched roles and continued to
work on the same topic. The table group used pencil
and paper and the pencil and paper group used the
application. For the second round, we have focussed
on the movement along the y-axis. This time, group
A started with pencil and paper, whereas group B
used the table. Then they switched their roles again as
in the first round. Each round consisted of 6 exercises,
3 exercises which had to be performed with pencil
and paper and 3 exercises using the multitouch
application.
Exercises looked like this:
The quadratic function f has the form
f(x) = x
2
+ r (1)
Chose a value for r in a way that f has
Two x-intercepts: r= (2)
Only one x-intercept: r= (3)
No x-intercepts: r= (4)
The groups had 20 minutes for the exercises,
independent of the working method.
After completing all exercises, we had handed out
a questionnaire asking their opinion about the
application.
At the end, we had a short discussion with the
whole group.
4.2 Focus Group Workshops
The workshops consisted of a focus group of pupils
from another high school. They all were part of a
STEM special interest course. The number of pupils
varied during the several meetings between 6 and 11.
Their age was between 15 and 17, both male and
female. All of them were familiar with the
mathematical background.
The kick-off of the workshops has been an
introduction into our system. Afterwards the pupils
have met during about 9 months for open discussions.
Altogether, there were approximately 6 general
meetings with all participants. The goal of the
workshops has been to suggest further features, that
the students would find useful for an interactive math
application, having in mind their recent learning
experience.
5 RESULTS OF THE STUDY
Whereas the user study tried to find a hint to support
our hypotheses. Especially we were keen to know if
the pupils perform better, when they use an
interactive application compared to a group with
pencil and paper.
The focus group workshop was targeted at
improving the interactive math application in general
in order to improve its acceptance among pupils in the
classroom.
5.1 Findings of the User Study
As it concerns the results of the exercises, we could
verify our first hypothesis (A): The multitouch group
was already finished with their exercises mostly in
less than half of the available time, whereas the pen
and paper group needed almost the whole time slot.
Concerning hypothesis (B) there were no clear
findings if the pencil and paper group performed
better or worse than the multitouch group. The pen
and paper groups even performed slightly better in
our study. However, the small number of participants
CSEDU 2016 - 8th International Conference on Computer Supported Education
200

and the small number of exercises is a weakness of
this study. Hence, we do not see a clear hint that one
of the methods would be better for learning the
concept than the other.
We made several interesting discoveries, when we
met after the exercise to discuss the application.
The teacher made a comment that she could
introduce the concept of the quadratic equation and
the displacement of the function graph in half an hour
compared to a week (about 4 teaching hours) using
the traditional way. This comment points toward a
high potential of the application for the use in a
classroom from the teacher’s point of view.
Afterwards she encouraged the pupils to use the
application for further experimentation and asked
more in depth question. Although the pupils have
performed very well in both exercises, they had not
yet profoundly acquired the underlying concepts. For
several questions, they selected wrong parameters
(especially the wrong sign). Experimenting with the
application, they quickly discovered their mistake and
corrected the parameters. This observation together
with the fact that the pupils executed the exercises
much quicker using the application led us to the belief
that the use of a careful didactic instruction and
especially careful selection of exercises is needed.
Learners may be entrapped by the application to make
faster but not always carefully elaborated decisions.
Further results have been discovered by
evaluating the questionnaires. On a 7 point likert scale
the students had to answer several questions, where
“1” was “strongly agree” and “7” was “strongly
disagree”. In the following, we highlight the most
interesting results. In general, there was a great
acceptance of the multitouch application and the
worst results for individual questions has been an
average of 2.44.
We had asked questions concerning the topics
ease of use, work effort, maturity of the hard- and
software, usage scenarios (basic introduction,
repetition, in-depth studies) and general opinion.
In the following, we mention only some selected
answers that we found the most useful.
The question “The interaction with the table was
obvious” has been rated 1.0. Asking if “The
application motivates to deepen the topics
autonomous” has been rated 2.11. “The critical
examination of the topics is stimulated” scores 2.33
and the question “The examples of the multitouch
application improve my interest in the topic” scores
with 1.66. Most students were convinced that the
application helps them to determine their individual
learning pace (1.55). The pupils wanted to use the
application to get an overview of the related topic
(1.67), acquire basic knowledge (1.62), evaluate
theoretical knowledge (1.56) and repeat and deepen
(1.67) the content. In general the pupils felt well
prepared for the math course at school using the
application (1.72). Summing up they gave a grade of
1.67 for the application and they would recommend
the application to their fellow students with a value of
1.22.
There was an additional possibility to write free-
form text concerning advantages and drawbacks of
the application. As advantages the students
mentioned at first “Having more fun to learn the
topics”, which has been mentioned 5 times. Working
with the multitouch application means spending less
amount of work for the same result has been
mentioned 5 times as well. Three positive comments
relate to the integrated exercises. Two utterances
relate to the positive experience with experimental
learning.
On the other site the pupils mentioned as
drawbacks that the output device is not well suited for
their learning environment (too expensive, too hard to
transport, limited number of users at the same time).
4 comments related to this point. One student pointed
out, that the tool does not replace the explanation of a
teacher.
5.2 Results of the Workshops
The participants of the focus group analysed the
multitouch application and elaborated several topics
in their meetings concerning different parts of the
application and the learning process with the
application in general. The following sections sketch
their proposals.
5.2.1 Support of the Introduction Phase
There should be an introduction into the specific
topics, which is integrated in the application in a way
that the topics are self-contained and do not need an
external explanation.
5.2.2 Different Learning Modi
There should be different ways for working with the
exercises. A learning mode could be used to focus on
specific subjects only.
Assigning difficulty levels to exercises should
help students to deepen their knowledge in a way that
only exercises related to a specific level will be
selected.
An exam training mode should present different
questions of the topic field, possibly also varying in
Suremath - User Study and Related (Re-)Implementation of a Multitouch Application for Learning Math
201

the difficulty.
A self- assessment mode should only go from one
topic to another if a certain number of exercises has
been solved correctly.
Furthermore the participants suggested to
incorporate some kind of gamification e.g. using high
scores so that pupils of a class could compete against
each other.
5.2.3 Misconception Detection
The application should be able to discover a
misconception and to actively help the learner to
overcome the error. E.g. if a student drags the
parabola in the wrong direction, the application
should detect this (common) mistake and remind the
user to rethink her approach and possibly give her
relevant information that is needed in this situation.
6 OPERATIONALIZATION OF
THE RESULTS
The results of the study and the workshops require to
integrate certain concepts that are not part of the
system yet.
As it concerns the exercises, right now they are
“hard-wired” into our application. In order to
guarantee a high amount of exercises to make the
application attractive to use, it is necessary to provide
an authoring tool which can be easily used by teachers
and pupils. Hence there must be a possibility to load
the exercises into the system at run-time.
We have decided to integrate a web based
authoring tool and to store the exercises on a server in
order to build-up a pool of exercises that everybody
can use.
A further requirement was the dynamic
compilation of exercises.
Therefore each exercise can be associated with
metadata, which will be used form the system to
select exercises at run-time. Accordingly different
selection criteria have to be integrated into the
application for supporting the different learning modi
(Section 5.2.2).
Concerning the remarks about the poor
availability of the system in schools, we have decided
to port the implementation to a platform that supports
several clients, which are more commonly available
at schools, like whiteboards, PCs or tablets (Section
5.1).
We are about to make it possible to display text,
sound and animation. This enables possibilities for
the introduction phase (Section 5.2.1), like explaining
mathematical concepts and instructions how to work
with the system. Especially concerning the
animations, we will offer the possibility to control the
display of graphs, formula text and sound using a
simple scripting language.
Finally, the detection of mistakes (Section 5.2.3)
is supported by the system through attaching events
to the mathematical objects. If a user has to move a
parabola to the right in order to correctly solve the
exercise, it is possible to attach an event to the
parabola so that moving the parabola to the left will
trigger an event displaying hints or pointing again to
the theoretical foundations.
7 SYSTEM DESIGN
The program is implemented in the C# language,
using the Monogame framework (Monogame 2016).
This framework evolved from Microsofts XNA
Game Studio (Microsoft 2016) and can support
multiple operating systems like Microsoft Windows,
Linux, OS X, Android, iOS and more. For
implementing applications for Android and iOS, the
Mono implementation from Xamarin (Xamarin 2016)
is necessary. The programming is mostly done loop
based like a video game rather than event based. This
allows easier programming and smoother handling
like moving the function graphs on the drawing area.
Each frame, the input is read and the program reacts
on changes.
7.1 Input
Suremath has input handling for mouse, touch and
pixelsense (SUR40 (Samsung 2016). Input from most
interactive whiteboards is possible as mouse input
simulation. As long as the input is not the mouse
device (or its emulation) the program can handle
multitouch.
7.2 Gesture Recognition
The recognition of the mathematical functions drawn
on the grid is realized using the Protractor algorithm
(Li 2010). This algorithm is a geometric template
matcher that is based on the angular distance between
the template and the drawn input. This allows very
easy and fast recognition, but some additional work
on the templates is needed. For example a normal
parabola (5) and one with a negative leading
coefficient (6) have different angular sums, and hence
need two separate templates.
CSEDU 2016 - 8th International Conference on Computer Supported Education
202
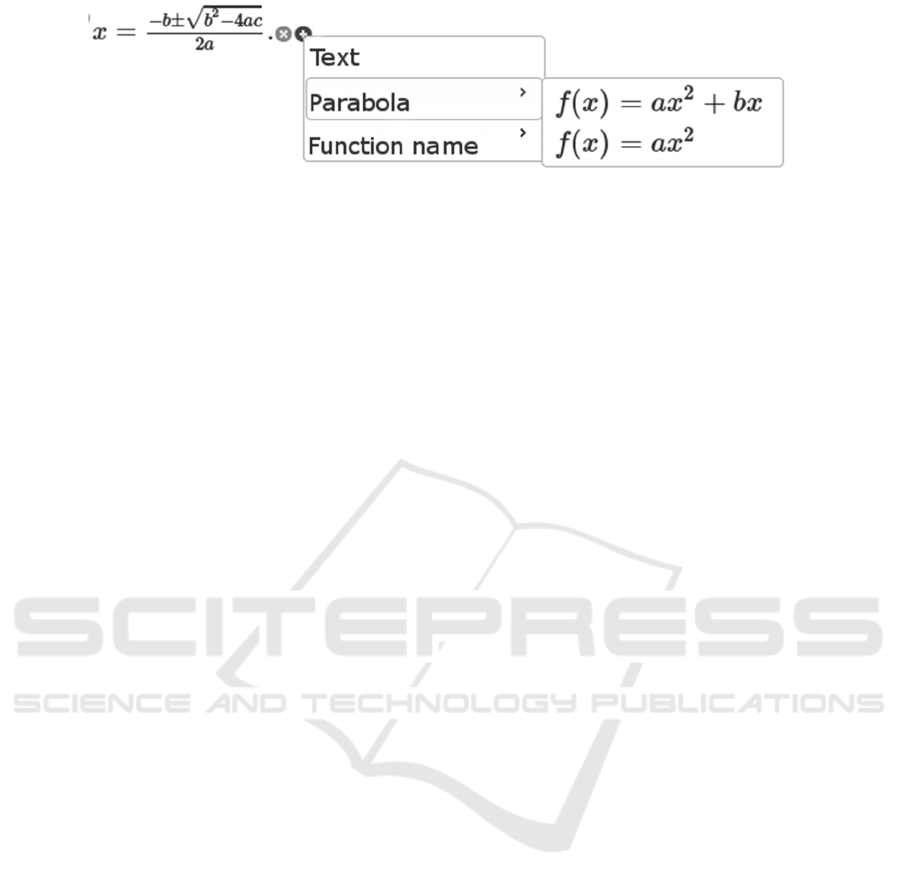
Figure 2: Formula creation using the authoring tool.
y = x
2
(5)
y = -x
2
(6)
However the algorithm is so flexible that new
functions can be recognized by simply adding the
corresponding templates. The templates are stored in
an XML file. This guarantees a user friendly way to
easily extend the set of gestures.
7.3 UI Elements
Since XNA/Monogame does not have any GUI
elements included, all UI elements like windows,
buttons, slider or textboxes have to be implemented
separately. In our implementation the required images
are generated programmatically on the fly.
7.4 Exercises
Our authoring tool allows to create exercises and to
store them on a server. The exercises can be retrieved
over the internet by entering a code in an input field
on the exercises menu. A set of several exercises is
stored in a ZIP file that contains a SQLite database
file together with the metadata of the exercises and
the images that contain the instructions. The use of
images instead of text is necessary since complex
formulas have to be displayed.
Exercises can have attached events, text or sound
hints and animations. Giving an example, it is
possible to add a text hint that is shown when a
parabola is moved to the left instead of to the right.
This allows the creation of self-explaining exercises.
Furthermore the integration of sound and
animation can be used for an introduction into the
subject. Using evented math objects it is even
possible to integrate a mixed initiative introduction
where the user is required to take some action before
an animation continues.
7.5 Authoring Tool
The authoring tool is a web-based tool, which is
integrated in a web page. It allows creating exercises
as a static and/or dynamic set of exercises. Static sets
are a fixed list of exercises, created by the author.
These sets are presented to the learner in the sequence
as they have been created. Dynamic exercise sets can
be compiled on the fly, based on metadata like
difficulty, or topic.
The creation of the individual exercises in the web
page is done using a very simple WYSIWIG LaTeX
editor, based on tokens. Therefore it is possible to
create professionally looking instructions for
exercises without the need of knowing LaTeX or any
other language to render equations.
Every token has two icons on the right hand side
(see fig 2.).
A little “x” in a circle, used to delete this token.
A “+” in a circle adds a new token right to this
token.
This tool uses conventional web technologies like
Javascript and jQuery on the client side. For the user
interface jQueryUI is used. The rendering of the
equations is done with MathJax.
On the server-side asp.net and LaTeX are used.
The application runs on a Linux system to render the
text into images and create ZIP files and SQLite
databases for deploying exercise-sets to the clients.
8 SUMMARY
In this paper, we have presented the evaluation of our
multitouch application for learning math and the
improved implementation based on the results we
have gathered through our evaluation with pupils and
workshops with a focus group.
First we have described the setup of our study and
the approach for the workshops.
In the user study, we could show that learners can
work faster with our application, compared to
working with pen and paper.
Based on the findings of the comments from the
user study and the remarks from the focus group, we
have formulated the belonging features and changes
of our application. These changes had to be integrated
in order to support the learner and to improve the
Suremath - User Study and Related (Re-)Implementation of a Multitouch Application for Learning Math
203

acceptance and usability of our system. These were
concepts like providing a kind of scripting to create
an introduction to the domain. We have introduced
meta data, e.g. in form of difficulty levels and
different learning modes like competition, exam
training etc.. Additionally we have introduced events
to react flexibly on the user interaction.
Finally, we have shortly sketched our system (re)
implementation, based on a video game based
concept with an independent authoring tool, which is
browser based.
In the future, we want to extend the use of our
system. We have it already installed in one of the high
schools running on a whiteboard for being used in the
classroom. We also plan to make the Android version
available in the app store soon. We hope to receive
much more input from pupils and teachers over this
channel. Additionally we want to carry through a
more profound user study with our improved system.
As a further step, we plan to extend the
functionality of our application to fields with similar
challenges, e.g. trigonometric functions. More
specifically, we want to extend the system to
exercises of the type: “At a point 15 feet from the base
of a church, the angle of elevation of the top of the
church is 43°. Find the height of the church to the
nearest foot.” We imagine that moving objects back
and forth and changing the angle of lines interactively
while displaying the values of angles, lengths and
trigonometric functions can help to grasp the concept
behind relatively abstract functions like sine or
cosine.
ACKNOWLEDGEMENTS
We especially want to express our gratitude to the
Angela Merici Gymnasium Trier, Germany, for
supporting our research, especially Miss Daniela
Kiefer and her pupils who participated in the user
study. Furthermore we want to thank the Auguste-
Viktoria-Gymnasium, Trier, Germany especially
Miss Karin Brezina and Miss Anne Bläsius and her
pupils who supported our work by participating in our
workshops and still continue working with us on this
topic.
REFERENCES
Blanke, D., Schneider, G., 2011. TOM A multi-touch
System for learning math, In Proceedings of
the 3rd International Conference on Computer
Supported Education CSEDU 2011, 6. - 9. 5. 2011
Noordwijkerhout, The Netherlands.
Hohenwarter, M., Fuchs, K. (2004). Combination of
Dynamic Geometry, Algebra and Calculus in the
Software System GeoGebra. Computer Algebra
Systems and Dynamic Geometry Systems in
Mathematics Teaching Conference 2004. Pecs,
Hungary.
Iijima, Y. 2012. GC/HTML5: Dynamic Geometry Software
which can be Used with Ipad and PC - Feature of
Software and Some Lessons with it, In: Proceedings
ICME 12, Seoul, Korea.
Kortenkamp U., Richter-Gebert, J. (2002). Making the
move: The next version of Cinderella. Mathematical
Software, Proceedings of the First International
Congress of Mathematical Software, 208–216, World
Scientific.
Li, Y., 2010. Protractor: a fast and accurate gesture
recognizer, In: Proceedings CHI '10 Proceedings of the
SIGCHI Conference on Human Factors in Computing
System, ACM New York, NY, USA.
Means, B., Toyama, Y., Murphy, R., Bakia, M., Jones, K.
2009. Evaluation of Evidence-Based Practices, In
Online Learning: A Meta-Analysis and Review of
Online Learning Studies, U.S. Department of
Education, Office of Planning, Evaluation, and Policy
Development, Washington, DC, USA.
Microsoft 2016. https://www.microsoft.com/en-us/down
load/details.aspx?id=23714, download: 1/11/2016.
Monogame 2016. http://www.monogame.net, download:
1/11/2016.
Samsung 2016. http://www.samsung.com/uk/business/
business-products/smart-signage/professional-
display/LH40SFWTGC/EN, download: 1/11/2016.
Xamarin 2016. https://xamarin.com, download: 1/11/2016.
Zeleznik, R., Bragdon, A., Adeputra, F., and Ko. H., 2010.
Hands-on math: a page-based multi-touch and pen
desktop for technical work and problem solving In:
Proceedings UIST '10 Proceedings of the 23nd annual
ACM symposium on User interface software and
technology, ACM New York, NY, USA.
CSEDU 2016 - 8th International Conference on Computer Supported Education
204
