Design and Analysis of Cascaded Variable Buoyancy Systems for

Selective Underwater Deployment

Thiyagarajan Ranganathan and Asokan Thondiyath

Robotics Laboratory, Department of Engineering Design, Indian Institute of Technology Madras, Chennai, India

Keywords:

Variable Buoyancy, Selective Deployment, Bellow with Linear Actuator.

Abstract:

Variable Buoyancy systems for selective deployment has been designed and the analysis of dynamics is dis-

cussed in this paper. Multiple interconnected VB modules have speciﬁc advantages in positioning the payloads

like sensors and communication equipment at various depths to collect strategically important subsea data. The

design of metallic bellow based Variable Buoyancy Systems (VBS) is presented along with the dynamic anal-

ysis of the module. The dimensions of the VB module are optimised to give best performance at the desired

depth of operation. Effect of anchoring the module to a base station and cascading multiple modules for de-

ploying at various depths have been studied in detail. Simulation results show that the cascaded VB modules

can be successfully deployed for selective applications under various operating conditions.

1 INTRODUCTION

A variable buoyancy system is a device which can

change its mass (keeping volume unaltered) or vol-

ume (keeping mass unaltered) during its operation,

thereby varying the net buoyancy (difference between

weight and buoyancy) of the system. When the

weight of the system is equal to the buoyancy, the

system is Neutrally buoyant and based on the sign of

difference between weight and buoyancy, the system

is either positively or negatively buoyant. Variable

Buoyancy Systems for different underwater applica-

tions either for underwater systems or as stand-alone

system, is one of the main research interests in the

ﬁeld of underwater vehicles (Sumantr and Teknologi,

2008; Tangirala and Dzielski, 2007; Wen-de Zhao

et al., 2010; Worall et al., 2007; Ranganathan et al.,

2015; Wu et al., 2011). Such systems dive across

the water column by varying the buoyancy and they

are used in underwater vehicles to get depth varia-

tion during manoeuvres. VBS, being one of the ef-

ﬁcient ways to achieve various depths, has potentials

much more than just using them as add-ons to under-

water vehicles. There may be situations where we

need to maintain the depth of a sensor suite under-

water to collect information. Depending on the appli-

cation, sensor suite may need to change the depth at

regular intervals to meet speciﬁc requirements. Under

such circumstances, a VBS can be positioned at the

desired depth by anchoring and when required, it can

be deployed to the desired depths. Hence VBS can

be used as standalone systems which have the ability

to achieve required vertical single degree of motion

efﬁciently. However when the depth of operation in-

creases, the length of cable connected to VBS to an-

chor will increase which may result in couple of is-

sues. First, if the cable is not neutrally buoyant, after

a certain length, the self-weight will become higher

the maximum positive buoyancy that can be achieved

by the VBS and this will lead to system malfunc-

tioning. Secondly, the cable may get entangled and

handling the cable will be difﬁcult. In these situa-

tions, there is a need for multiple interconnected VBS

which will avoid the issues discussed. Furthermore, if

the considered scenario is extended in a way that the

surrounding environment has to be monitored at var-

ious depths simultaneously, the same interconnected

VBS can be used. Hence selective deployment can

be achieved by having such multiple interconnected

modules.

Different types of VBS are available in literature

and based on the requirement of selective deploy-

ment, a suitable method has to be chosen. Most of

variable buoyancy systems use water ballast in which

either the weight or volume is changed witha suit-

able mechanism (Tangirala and Dzielski, 2007; Wen-

de Zhao et al., 2010). (Wu et al., 2014) proposed a

ballast based VB mechanism to position the system at

a depth. The surrounding water is taken in to increase

the mass and vice versa. This may result in corro-

Ranganathan, T. and Thondiyath, A.

Design and Analysis of Cascaded Variable Buoyancy Systems for Selective Underwater Deployment.

DOI: 10.5220/0005979903190326

In Proceedings of the 13th International Conference on Informatics in Control, Automation and Robotics (ICINCO 2016) - Volume 2, pages 319-326

ISBN: 978-989-758-198-4

319

sion of the system when it is operated for longer du-

ration. (Worall et al., 2007) developed a bellow based

VB system which uses reservoir with oil and a pump

to ﬁll oil in and out of the bellow so that the overall

weight of the system is varied. In this case the size

of the system becomes large because of the support-

ing components and the maintenance becomes difﬁ-

cult. Spermaceti oil hypothesis based VB modules

are also used in some systems. Parafﬁn wax with a

heating element are used to change the volume of a

system (Shibuya and Kawai, 2009). Such response

time of such systems are low. A metallic bellow based

VB system is reported in (Shibuya and Yoshii, 2013)

which uses a peltier element to change the phase of a

parafﬁn wax which can be used to expand and com-

press the bellow. Some methods use the gas produced

by microbes to change volume and thereby achieving

variable buoyancy. These systems do not require any

external power (Wu et al., 2011).

Considering the need for a controlled buoyancy

variation, a VBS with metallic bellows and actuated

by linear actuators is discussed in this paper. The nec-

essary variation in buoyancy can be obtained by con-

tracting or expanding the metallic bellow using a suit-

able linear actuator. A system with a metallic bellow

with linear actuator and necessary supporting struc-

tures/devices becomes a VB module. Multiple of such

modules can be interconnected to selectively deploy

at different depths to simultaneously monitor/sense at

different depths. The design of a cascaded VB system

for selective deployment and intermittent actuation is

analysed in the following sections.

2 SYSTEM DESIGN

As discussed in the previous section, selective deploy-

ment uses multiple modules cascaded in such a way

that each of these modules can be actuated individu-

ally or in combination to achieve desired movement or

depth. One such cascaded schematic with two mod-

ules is shown in ﬁgure 1.

Each of these VB modules is based on the concept

of bellow with linear actuators. The conceptual de-

sign of a bellow based VB module is shown in ﬁgure

2. It has a hull and two bellows. The hull and bellows

are connected using ﬂanges with bolts and nuts. The

hull provides a platform to hold the linear actuators as

well as the electronics required to control the move-

ment of linear actuators. Two linear actuators are used

to individually actuate the two bellows. Provisions are

made for connectors for power and communication

signal transmission. To understand the behaviour of

the system, initially, bellow with linear actuator based

Figure 1: Cascaded modules for selective deployment.

(a) CAD model

(b) Internal view

Figure 2: Conceptual design of a metallic bellow based VB

module.

VBS is modelled mathematically and few open loop

simulations are carried out. Then, multiple of such

systems are cascaded and simulated.

These modules are designed based on the require-

ments that they should be able to operate at a par-

ticular depth and it is desired that these systems are

portable and light weight. To achieve these require-

ICINCO 2016 - 13th International Conference on Informatics in Control, Automation and Robotics

320

ments the dimensions of the module are optimised to

minimize the weight of the module and to minimize

the force experienced by the end plate of the bellow,

subject to constraints on length and neutral buoyancy.

Hence with all these objectives, the design parame-

ters like the radius and length of the bellow and the

hull were optimized. These dimensions of the system

are optimized for it to be operated up to a depth of

10m and are shown below. For a different depth of

operation, the dimensions are to be optimized again

and the speciﬁcation of linear actuator will vary.

= 110.5mm, r

= 108.5mm,

= 130mm, L

= 193.5mm

3 MATHEMATICAL MODEL

The system is assumed to be cylindrical with body

ﬁxed coordinate frame X

and the earth-ﬁxed co-

ordinate frame is represented as XY Z as shown in ﬁg-

ure 3. The six-degrees of Freedom (DoF) dynamics

of the underwater system is derived using Newton-

Euler equations in body ﬁxed co-ordinate frame as

shown in equation (1) (Fossen, 1994; Gianluca An-

tonelli, 2006).

τ = M

v +C

C(v

v)v

v +D

D(v

v)v

v +g

g(η

η) (1)

Here, v is the vector representing linear and an-

gular velocites of the system. η

η is the pose vector in

earth ﬁxed co-ordinate frame obtained by transferring

the body - velocities to velocities in earth frame using

J(η

η) and integrating the velocities in earth frame. τ

is the external forces and moments vector. M is the

matrix comprising of mass and inertia of the system

along the principle axes, D(v

v) is the damping forces

and moments matrix, C(v

v) is the Coriolis and Cen-

tripetal forces and moments matrix, and g(η

η) is the

Restoring forces and moments vector which governs

the forces and moments due to difference in Buoy-

ancy (W) and weight (W) of the system and also the

position of CoG and Centre of Buoyancy (CoB).

The underwater module will experience force on

the end plate due to external hydrodynamic pressure,

and internal pressure due to expansion and compres-

sion. The spring force of the bellow also inﬂuences

the dynamics of the actuation of the bellows. The

variation of the internal forces as a function of the bel-

low length variation is plotted in ﬁgure 4 .The max-

imum internal force due to internal pressure for the

designed module was found to be +/-350N for an 8%

change in length of bellows. The bellow stiffness is

taken as 54N/mm. The force on the endplate due

Figure 3: Co-ordinate frame representation.

Figure 4: Percentage change in length vs force acting on the

endplate.

to external hydrodynamic pressure will linearly vary

with the depth at the rate of 380N/m.

These forces were also modelled and incorporated

in the above discussed mathematical model while

analysing the dynamic performance of the module.

3.1 Open Loop Simulation Studies

Simulations are carried out with the mathematical

model developed. No external disturbances are con-

sidered during the simulation and the pressure vari-

ation along the water column is assumed to be uni-

form. Some studies were carried out to understand

the behaviour of the system when it has some initial

orientation. When the system is left with an initial ori-

entation of 10

◦

and 20

◦

of roll and pitch respectively,

it rolls back to the stable equilibrium position with

both becoming 0

◦

. It is because of the restoring mo-

ment created due to non-alignment of CoG and CoB.

Oscillations are noticed which dies down slowly as

shown in ﬁgure 5.

When the system is oriented in roll in such a way

that the CoG is above the CoB, it is observed that with

even small numerical disturbances, it rolls back again

to the stable position and oscillates around the sta-

ble position. This oscillation dampens down progres-

sively. The net positive buoyancy ‘b’of the system is

varied from 0.5N to 3N and the velocity at which the

system travels along the water column was studied. It

Design and Analysis of Cascaded Variable Buoyancy Systems for Selective Underwater Deployment

321

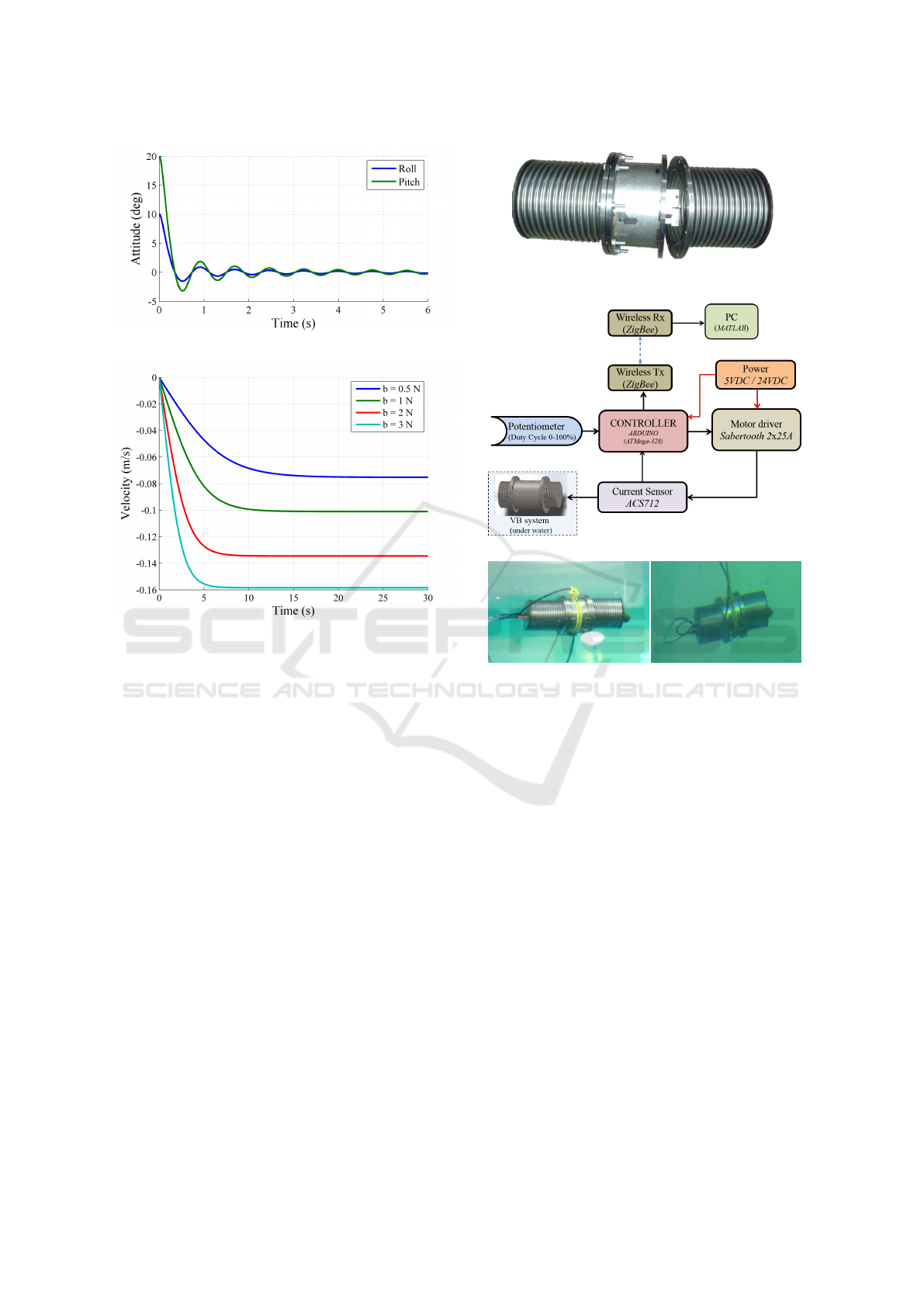
Figure 5: Attitude of the module.

Figure 6: Velocity at various positive buoyancy.

was noticed that the system accelerates initially and

after some time it starts moving with constant veloc-

ity (saturation velocity). At different levels of positive

buoyancy, the velocities achieved by the system are

shown in 6.

4 EXPERIMENTAL SETUP

The objective of the experimentation is to validate

the mathematical model and to understand the power

consumed by the system while operating at various

depths. To validate the model, a prototype was de-

signed and fabricated based on the dimensions ob-

tained from optimization. The material used for the

prototype is stainless steel; ﬁgure 7 shows the fabri-

cated system. Two linear actuators are chosen based

on the force analysis to expand and compress the bel-

lows. Underwater connectors and cables are used for

power and communication signals.

The electrical and electronic setup consists of a

controller, motor drivers for linear actuators, and sen-

sors. The role of controller is mainly to get user com-

mands, communicate them to the linear actuator ac-

cordingly and collect some vital information from the

system, communicate them to the user. A current sen-

Figure 7: Fabricated prototype.

(a) Schematic of experimental setup.

(b) VB system during operation.

Figure 8: Experimental setup.

sor is used to sense the current consumed in the sys-

tem during its operation, which can help us estimate

the overall power consumed by the system per cy-

cle of operation. The controller will generate Pulse

Width Modulated (PWM) signals and send it to mo-

tor drivers. A ZigBee based wireless communication

is used to communicate the parameters like time, cur-

rent and commanded velocity, instantly to a remote

PC in which the data can be recorded and visualised

in real time. A 24V 6A DC power source is used to

power the entire system and source is stepped down

wherever required. The schematic of the experimen-

tal setup and some pictures during the operation are

shown in ﬁgure 8. The entire setup, except the VB

module, will act as the ground control station.

4.1 Results and Discussion

Ground experiments were conducted to measure the

velocity of the linear actuator at different Duty cy-

cles of PWM. The speed of the linear actuator with

respect to PWM duty cycle is shown in ﬁgure 9. The

ICINCO 2016 - 13th International Conference on Informatics in Control, Automation and Robotics

322

Figure 9: Bench test : Velocity of LA Vs. Duty cycle.

percentage of duty cycle of PWM signal corresponds

to the input voltage to the linear actuator at the rate

of 0.24V/% of duty cycle. A bench test on the sys-

tem with different duty cycle input to the system was

conducted. It can be seen that the velocity during ex-

pansion is faster than the velocity during compression

in the bellows.

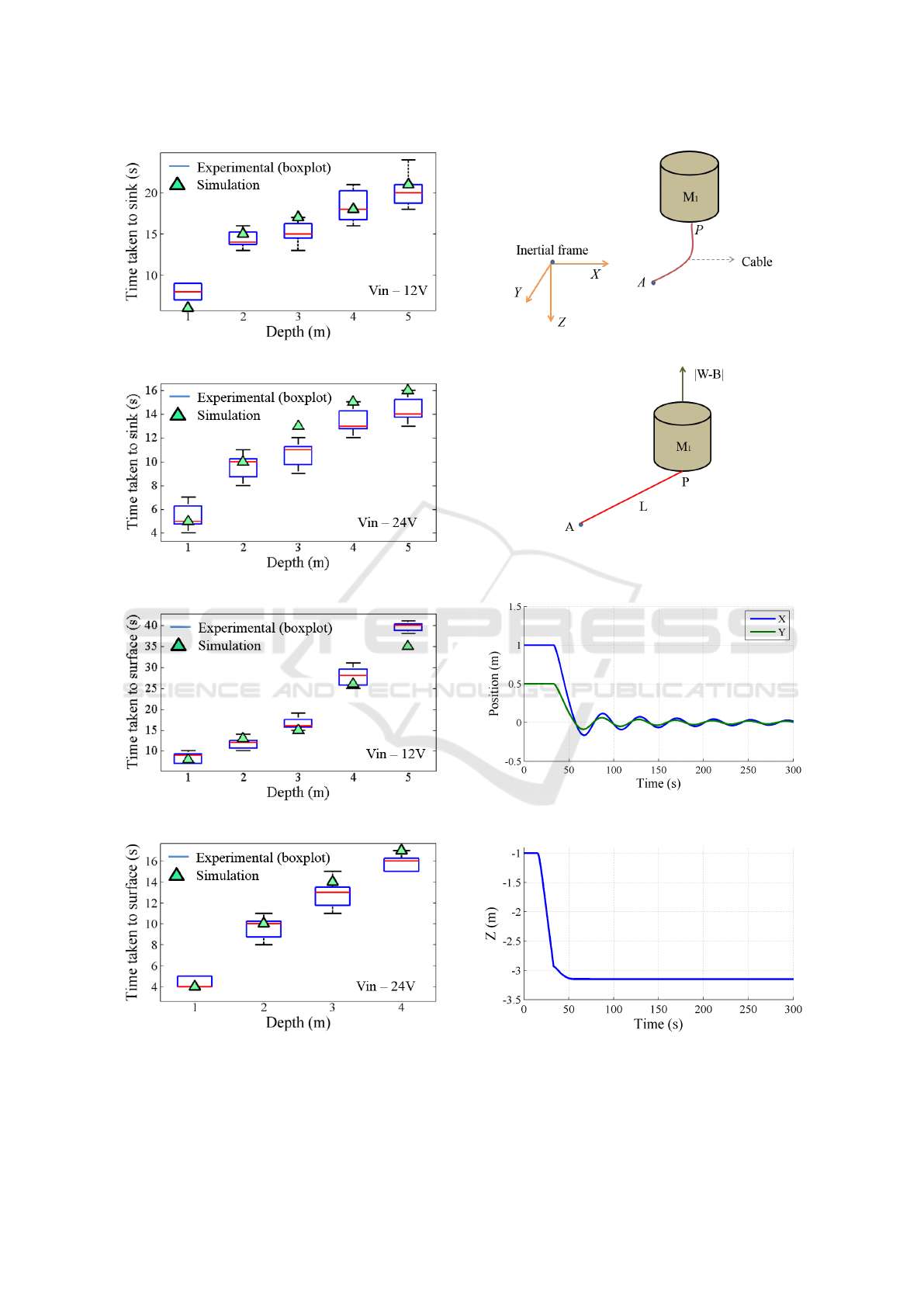
The following are the results during experiments

underwater. Experiments were conducted up to a

depth of 5m. The objective of the experiments is to

understand the time taken to surface and sink by the

system from/to different depths at different rates of

change of buoyancy. The rate of change of buoyancy

is directly proportional to the velocity of the linear ac-

tuator which is commanded by the input voltage to the

linear actuator. Experiments were repeated six times

at each depth and the mean time taken by the sys-

tem to sink/surface to a particular depth with different

duty cycle is shown in ﬁgure 10.

It can be observed that the time taken to surface is

slower than the time taken to sink to the same depth.

This is mainly due to the external pressure variations

during sinking and surfacing. The experimental re-

sults are compared with the simulation results for a

particular buoyancy variation. The experimental and

simulation results of time taken to sink and surface at

12V and 24V are shown in ﬁgure 11.

Simultaneously, the current consumed for surfac-

ing and sinking to a depth by expanding or contract-

ing is logged. It was found that the expansion from

a depth consume more current than compression ini-

tially since the linear actuator has to overcome the

force due to external pressure to expand. On the other

hand, the force required to compress is comparatively

lesser since the external pressure itself aids in com-

pression.

5 ANCHORED AND CASCADED

SYSTEMS

Simulation studies were conducted to understand the

(a) Time taken to sink.

(b) Time taken to surface.

Figure 10: Time taken to sink and surface at different rate

of change of buoyancy.

behaviour of the system when the VB module is an-

chored at a particular depth as shown in ﬁgure 12. The

point to which the cable is connected to the system is

P and the length of the cable is L. For this simulation,

the cable length is assumed to be 3m, anchoring point

A is [0,0,0] and the initial point at which the system

is positioned is [1,0.5,-1] with respect to the inertial

frame. The behaviour when the module travel along

Z is shown in ﬁgure 13. It can be seen from the plot

that it the system travels up and it oscillates when the

cable is stretched completely. The dynamics of the

cable is not considered in this simulation.

Now another module is cascaded on top of the

anchored module as shown in ﬁgure 1. Initially the

buoyancy of both the modules are kept neutral. The

cascaded module is made positively buoyant by 1N

after 50th second of simulation.

The modules are initially located at [0.5,0,-0.5]

and [1,0,-0.5] and the point to which the ﬁrst mod-

ule is anchored is at [0,0,0] with respect to the earth

frame. Cable lengths are 2m and 5m for the ﬁrst and

Design and Analysis of Cascaded Variable Buoyancy Systems for Selective Underwater Deployment

323

(a) Time taken to sink at V

=12V.

(b) Time taken to sink at V

=24V.

=12V.

(d) Time taken to surface at V

=24V.

Figure 11: Comparison of experimental and simulation re-

sults.

(a) Initial position.

(b) When cable is stretched.

Figure 12: An anchored system.

Figure 13: Variation in XY position of the VB module when

anchored.

Figure 14: Variation in Z of an anchored module.

second modules respectively. It can be seen that, as

the second system moves up, when the cable is com-

pletely stretched, the positive buoyancy of cascaded

ICINCO 2016 - 13th International Conference on Informatics in Control, Automation and Robotics

324

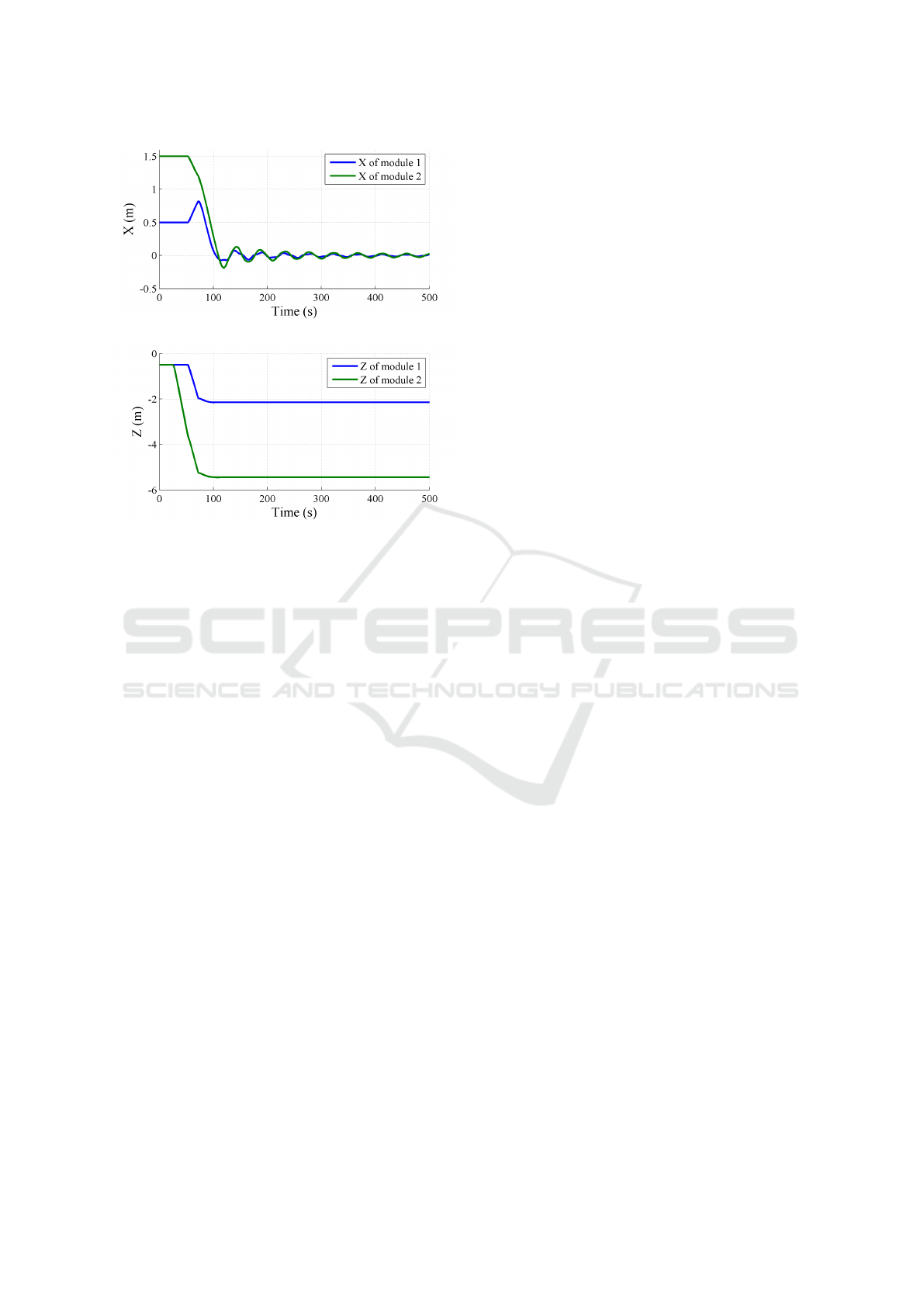
(a) Variation of X for both modules.

(b) Variation of Z for both modules.

Figure 15: Variation of position of both the modules.

module acts on the anchored module which inﬂuences

the anchored module also to travel up. Both the mod-

ules oscillate with the oscillations of cascaded module

inﬂuencing the oscillations of anchored module. The

travel of both the modules along the water column can

be seen in ﬁgure 15.

6 SUMMARY OF THE WORK

A metallic bellow based variable buoyancy system is

designed with dimensions optimally chosen based on

system requirements. The system is mathematically

modelled and the basic simulation studies shows that

the system is capable of diving to the desired depth

and surface by expanding or compressing the linear

actuator. The simulation studies also showed that the

system with some orientation in roll and pitch because

of any external disturbance will re-orient itself back

because of the restoring force created due to positions

of CoG and CoB. The terminal velocities of the sys-

tem at different positive buoyancies were studied and

it was found that at 3N positive buoyancy, the sys-

tem travels with a velocity of 0.32m/s. Experimental

results show that the mathematical model closely ap-

proximates the real system. The power consumption

study at different velocities of linear actuator shows

that, at maximum tested depth of 5m, with the max-

imum speed of linear actuator, the system consumes

an instantaneous power of about 24W. With the math-

ematical model, further simulations are carried out by

anchoring single system at a depth and by cascading

multiple such systems. The simulation studies show

that, when the system is positively buoyant, irrespec-

tive of the initial position at which it is, because of

the cable getting taught, it oscillates around the an-

chor point. When the system is at neutrally buoyant

state, the system remains at the same position unless

there is external disturbance acting on it.

7 CONCLUSIONS

The study reveals that the system is well suited for the

selective deployment applications and the ability of

them to be used as a single degree of freedom system

to have motion along heave. The study also shows

that the velocity of the system can also be controlled

by controlling the rate of change of buoyancy. Multi-

ple of such systems with individual depth control can

be cascaded and these systems can be deployed at dif-

ferent depths in the designed range.

Some issues were noticed while experiments like,

maintaining the symmetry of the bellows which re-

sulted in an unwanted tilt in the system. Also,

since two linear actuators were used, positioning them

maintaining the centre of actuation of both the bel-

lows is not possible. This can be avoided by using a

single vertical bellow based VBS. Open loop response

of the system is studied and further, the system can

be analyzed with a model based closed loop control

strategy to precisely control the depth using suitable

sensing. The depth control capabilities and perfor-

mances can be analyzed which may explore various

other applications.

REFERENCES

Fossen, T. I. (1994). Guidance and Control of Ocean Vehi-

cles.

Gianluca Antonelli (2006). Underwater Robots.

Ranganathan, T., Pattery, J., and Thondiyath, A. (2015). De-

sign and analysis of cable-connected metallic bellows

as Variable Buoyancy Modules. In 2015 IEEE Under-

water Technology (UT), pages 1–5. IEEE.

Shibuya, K. and Kawai, K. (2009). Development of a

new buoyancy control device for underwater vehicles

inspired by the sperm whale hypothesis. Advanced

Robotics, 23(7-8):831–846.

Shibuya, K. and Yoshii, S. (2013). New Volume Change

Mechanism Using Metal Bellows for Buoyancy Con-

trol Device of Underwater Robots. International

Scholarly Research Notices Robotics, 2013:1–7.

Design and Analysis of Cascaded Variable Buoyancy Systems for Selective Underwater Deployment

325

Sumantr, B. and Teknologi, U. (2008). Development of

Variable Ballast Mechanism for Depth Positioning

of Spherical URV. In International Symposium on

Information Technology (ITSim), pages 1–6, Kuala

Lumpur, Malaysia. IEEE.

Tangirala, S. and Dzielski, J. (2007). A Variable Buoyancy

Control System for a Large AUV. IEEE Journal of

Oceanic Engineering, 32(4):762–771.

Wen-de Zhao, Xu, J.-a., and Zhang, M.-j. (2010). A Vari-

able Buoyancy System for Long Cruising Range AUV

Wen-de Zhao, Jian-an Xu and Ming-jun Zhang. In

Internation Conference on Computer, Mechatronics,

Control and Electronic Engineering, pages 585–588.

Worall, M., Jamieson, A. J., Holford, A., and Neilson, R. D.

(2007). A variable buoyancy system for deep ocean

vehicles. In OCEANS 2007 - Europe, volume 44,

pages 1–6. IEEE.

Wu, J., Liu, J., and Xu, H. (2014). A variable buoyancy

system and a recovery system developed for a deep-

sea AUV Qianlong I. In Oceans 2014 - Taipei, pages

1–4. IEEE.

Wu, P. K., Fitzgerald, L. a., Bifﬁnger, J. C., Spargo, B. J.,

Houston, B. H., Bucaro, J. a., and Ringeisen, B. R.

(2011). Zero-power autonomous buoyancy system

controlled by microbial gas production. The Review

of scientiﬁc instruments, 82(5):055108.

ICINCO 2016 - 13th International Conference on Informatics in Control, Automation and Robotics

326