
Swing Leg Trajectory Optimization for a Humanoid Robot
Locomotion
Ramil Khusainov, Alexandr Klimchik and Evgeni Magid
Intelligent Robotic Systems Laboratory, Innopolis University, Innopolis City, Russian Federation
Keywords: Humanoids, Bipedal Walking, Swing Phase, Optimal Trajectory Planning, Dynamic Programming.
Abstract: The problem of walking trajectory optimisation for bipedal humanoid robots attracts many researchers
because of excessive interest to bipedal locomotion. The main focus is usually on robot dynamics and
trajectory planning for predefined walking primitives. In contrast to other works, our paper targets to obtain
optimal walking primitive for swing leg trajectory of bipedal humanoid robot walking. Optimal walking
primitives are obtained taking into account velocity and acceleration physical limitations of each joint and are
derived for different walking parameters such as step size and hip height. To obtain a desired time-optimal
trajectory dynamic programing approach is used. It is shown that a new trajectory is performed within a
shorter time comparing with commonly used locomotion trajectories for bipedal robots control. The results
allow us to assign walking parameters and corresponding walking primitive that maximize robot velocity for
predefined environment constraints.
1 INTRODUCTION
To create a robot that could successfully operate in
environments that were originally designed for
humans, research community addresses to human-
like robots also known as humanoids. The growing
interest in the development of humanoids has been
observed for more than several decades (Channon et
al., 1992; Goswami, 1999; Katić and Vukobratović,
2003). The objective of research community is to
create a robot, which will operate with a human or
instead of him/her in dynamic environments,
including offices, factories, private and public
compartments, and be able to help in performing
various operations originally adapted for a human as
an actor.
Bipedal walking is one of the most natural and
attractive types of humanoid locomotion, since
bipedal robots provide more potential and flexibility
to move through rugged terrains and complex
environments, which create considerable difficulties
for wheeled or tracked robots due to their limited
locomotion capacities. On the other hand, bipedal
robots are less stable and may fall down while
performing even simple activities in rather standard
for a human environment where we efficiently
operate on a daily basis. Therefore, bipedal
locomotion stability related research keeps attracting
many researchers nowadays in order to propose good
robot locomotion control algorithms and to prevent a
biped robot from falling down (Akhtaruzzaman and
Shafie, 2010, Escande et al., 2013).
Among the variety of open research questions in
humanoids, robot body motion gains significant
attention of research community (e.g., (Hofmann et
al., 2009, Ude et al., 2004)), since it has a direct
impact on robot dynamics and stability. Here, the key
interest of researchers is related to dynamics and
trajectory planning for predefined walking primitives
(Kajita et al., 2001). However, the leg trajectory
optimality in a swing phase - a state in which the lifted
leg has no contact with a supporting plane and swings
forward - has not been studied in details yet. Similar
problems arise in robotic manipulators, when time-
optimal trajectory for a particular technological task
should be obtained (Pashkevich et al., 2002). In
humanoids, researchers usually use predefined
smooth trajectories for swing foot motions, such as
cycloids or third order polynomials (Ha and Choi,
2007). Because during the walking process a swing
foot has a maximal Cartesian speed among all
humanoid limbs, leg joint limitations play a key role
in a humanoid robot overall walking speed. In our
paper we propose a simple and effective method for
searching of an optimal swing leg trajectory within
130
Khusainov, R., Klimchik, A. and Magid, E.
Swing Leg Trajectory Optimization for a Humanoid Robot Locomotion.
DOI: 10.5220/0006011401300141
In Proceedings of the 13th International Conference on Informatics in Control, Automation and Robotics (ICINCO 2016) - Volume 2, pages 130-141
ISBN: 978-989-758-198-4
Copyright
c
2016 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved

physical joint limits using dynamic programming
approach. Optimality of the trajectory is estimated
with a step traveling time criterion and also takes into
account velocity and acceleration limits of leg joints.
The obtained walking primitives with different
walking parameters (hip height, step size and time)
are used further to obtain optimal desired walking
primitive with a maximal robot velocity for
predefined environment constraints.
2 PROBLEM STATEMENT
There exist numerous approaches that are used for
bipedal robot locomotion control such as Central
Pattern Generation (Khusainov et al., 2015, Righetti
and Auke Jan, 2006), Neural networks, rule based
algorithms (Wright and Jordanov, 2014), gradient
optimisation technique (Ratliff et al., 2009) and
others. One of the most challenging strategies is so-
called passive-walkers (Collins et al., 2005, Collins et
al., 2001), which in some cases could be controlled
by a single actuator (Hera et al., 2013). The pioneer
method in modelling of a bipedal robot walking and
the most popular one is an analytical approach, which
defines equations for the robot locomotion under
some constraints induced by humanoid stability. This
approach has been used since 1970, when Miomir
Vukobratović proposed a so-called Zero Moment
Point (ZMP) stability constraint (Vukobratović and
Stepanenko, 1973). Currently, ZMP controller based
walking is the most popular approach, which
generates target trajectory of a humanoid robot in a
way that ZMP lies inside support polygon
(Vukobratović and Borovac, 2004, Sardain and
Bessonnet, 2004). Here, ZMP is a specific point on a
moving surface, where superposition of contact and
inertia forces does not produce horizontal moment. In
practice, the robot remains in a stable configuration
only if ZMP remains inside of a support polygon
(Erbatur and Kurt, 2009). ZMP stability constraint
equations are used to determine trajectory of Center
of Mass (CoM) for the robot (Mitobe et al., 2000).
ZMP approach is also used for trajectory generation
based on Inverted Pendulum Model (Majima et al.,
1999, Khusainov et al., 2016b) and Preview Control
(Kajita et al., 2003, Park and Youm, 2007). In these
methods CoM trajectory is a result of analytical
solution of dynamic equations for minimisation of
ZMP error in feedback control.
To the best of our knowledge, all algorithms for
bipedal robot stable walking select motion of leg
joints, which determine a swing leg movement,
without considering optimality of a trajectory. For
example, for NAO robot locomotion Motoc et
al.(Motoc et al., 2014) use a cycloid trajectory to
generate smooth motion, which is characterized with
zero velocity at the beginning and at the end of the
motion. Rai and Tewari (Rai and Tewari, 2014) use a
polynomial interpolation to obtain a swing leg
trajectory, assuming that robot’s CoM movement is
given. These approaches do not take into account
joint constraints and obtained trajectories do not
satisfy optimality from energy consumption point of
view. Therefore, the calculated trajectories of a swing
leg may be unattainable in practice because of
velocity/acceleration/jerk limits in joints and thus
would lead to wrong foot positioning, i.e. desired and
real trajectories would have a weak correspondence.
As a result, positioning errors accumulate with each
new step, which is a critical issue for autonomous
robots without global positioning feedback.
Figure 1: Full-size humanoid AR-601M (left) and its
kinematic structure in Matlab/Simulink environment
(right).
For our research we use anthropomorphic robot
AR-601M (Fig. 1) with 41 active degrees of freedom
(DoF), although we currently involve only 12 joints
(6 in each pedipulator) in the walking process.
Inverted Pendulum Model (Majima et al., 1999) with
ZMP stability constraint is used to generate CoM
trajectory. Here, six DoF of the supporting leg are
fully defined by CoM movement. For straightforward
motion it is assumed that in a frontal plane a swing
leg is perpendicular to the ground (or support plane)
at each time instance, a swing foot is parallel to the
ground, and a hip height remains constant during the
locomotion. These assumptions arise from human
natural walk analysis (Gabbasov et al., 2015) and are
widely applied in experimental works for biped
locomotion (Yussof et al., 2008). Taking into account
that a desired trajectory of a swing leg lies in a sagittal
plane, all joint coordinates of the swing leg are
uniquely defined for any foot and hip locations. In
this case the optimal trajectory problem is reduced to
2DoF system. Thus, the swing leg could be
Swing Leg Trajectory Optimization for a Humanoid Robot Locomotion
131

represented as a simple two-link system with hip and
knee joints. The corresponding to AR-601M robot leg
parameters link lengths are equal to 280 mm each.
Since in our models robot’s body moves at a constant
speed, for simplicity, we ignore body movement and
we assume a fixed position of the hip joint. This
means that the considered problem is represented in a
moving coordinate system. The trajectory of the
swing leg (solid line), support leg placement (dashed
line) and model parameters are shown in Fig. 2.
y
hip
0
0.5
ma x
x
ma x
y
x
ϕ
1
ϕ
2
Hip
Knee
L
2
L
1
Figure 2: Step motion of the swing leg in sagittal plane.
The primary research problem can be formulated
as follows: for given start and end points of a swing
leg and hip location, find an optimal trajectory, which
minimizes a selected cost function. Moreover, joint
angular velocities and angular accelerations during
locomotion should not exceed their maximal values.
The secondary research problem could be formulated
as follows: for a given robot and predefined obstacles
(which could be stepped over by the robot), obtain
optimal walking primitive that maximizes robot
speed in a given environment.
3 OPTIMISATION CRITERIA
There are different approaches to define cost function
in optimal trajectory search problem. For example,
Nakamura et.al. in (Nakamura, 2004) minimized
energy consumption, which can be written in the
form:
2
2
1
ii i
i
dt
τθ γτ
=
+
(1)
where
i
τ
is joint i torque,
i
θ
is joint i velocity,
γ
is
an empirical constant. The first term in (1)
corresponds to mechanical work, which is performed
to move dynamic system. The second term
corresponds to heat emission in each joint due to
torque generation. It was shown, that optimal
trajectory which could be found in such a way well
agrees with experimental data of human locomotion.
Yet, while obtaining a swing leg trajectory, the
authors do not take into account maximum joint
velocity and acceleration limitations, which actually
provide critical constraints for a real robot. A selected
trajectory could be energy optimal in theory, but if the
robot’s motors could not supply required by such
trajectory torques, the physical robot will fail to
perform such trajectory (Khusainov et al., 2016b).
In practice, walking speed is one of the most
important performance measures for bipedal robots.
Walking speed could be unambiguously calculated,
while energy consumption calculation is not that
obvious as it depends on many factors; e.g., energy is
mainly consumed in supporting leg joints, since they
have much higher actuating torques than swing leg
joints (in our work only swing leg motion is
considered). In addition, energy consumption
strongly correlates with step time: the faster a swing
leg moves for a given step length, the lower is its
energy consumption. Therefore, minimization of
each step time is a critical issue to be considered, and
it is the core contribution of our paper. Step time can
be evaluated as
()
1/
S
tVdS=
(2)
where V is a foot speed in Cartesian space and
dS is
the foot path. Time t is calculated numerically by
dividing the trajectory into a finite number of
intervals and further summing up over all intervals.
4 OPTIMAL TRAJECTORY
SEARCH ALGORITHM
Optimal path for a bipedal robot swing leg could be
found with regard to different optimization
techniques. For example, in (Nakamura, 2004) spline
genetic algorithm (GA) was used to determine joint
torques for several points on the trajectory, which
were further used to interpolate joint torques for
remaining trajectory points introducing third order
splines. The main drawback of this approach is the
fact that genetic algorithms in general are not efficient
in finding a global minimum for continues functions
with multiple local minima (Renders and Flasse,
1996). An alternative approach for 6 DoF
manipulator has been used by Tangpattanakul and
Artrit (Tangpattanakul and Artrit, 2009)who utilised
a heuristic optimization method to find optimal
trajectory with Harmony Search algorithm. The
ICINCO 2016 - 13th International Conference on Informatics in Control, Automation and Robotics
132

obtained trajectories were smooth and complied all
kinematic constraints (both for velocity and
acceleration). However, in this approach only 6 via-
points have been used along the trajectory, which
limited its utilisations because of potential problems
between via points.
To overcome the above mentions limitations, in
this study, an optimal path is calculated using
dynamic programming approach (Si et al., 2009). The
key idea of this approach is to divide a large problem
into sub-problems of lower dimensions
corresponding to a transition between two via points,
to solve each of these sub-problems once and to store
the solutions. Advantages of this method are
robustness and computational efficiency compared to
other methods. Illustration of this approach for a
simple case with three via points is shown in Fig. 3.
To find an optimal path from node (1,1) to node (5,1)
with a minimum total weight (time in the case of
optimisation walking trajectory) it is required to
examine all possible connections between these
points. Dynamic programing approach feature is that
via points are not specified exactly and can be
assigned to any node point of the row. Starting from
the left, for every node minimal total weight W is
computed and saved together with the node on the
previous layer, transition from which is optimal
(green lines in Fig. 3). For example, for node (2,2) the
minimal weight is 5 and the only transition from node
(1,1) is possible. For node (3, 1) minimal weight is 8
(5+3), which corresponds to transition from the node
(2,2). For node (3, 3) minimal weight is also 8 (5+3)
and corresponds to transition from the same node
(2,2) as for note (3, 1). For node (4, 3) minimal weight
is 12 (8+4), which corresponds to transition from the
node (3,1). Finally, we look at end point and find its
optimal transition. After that, to get the optimal path,
the optimal path from the last layer to the first layer is
constructed. For the provided example the optimal
path corresponds to the following path (red bold line
in the Fig. 3): (5,1), (4,3), (3,1), (2,2), (1,1).
To transform optimal path search problem for a
bipedal robot from continues optimisation problem
into a directed graph it is required to assign evenly
distributed nodes
,ij
p
within the search space, which
cover all possible trajectory paths. Here, to cover all
leg locations in x and y coordinates, it is necessitated
to create a two dimensional grid with
1
x
n +
points in
x direction and
1
y
n +
points in y direction (see
Fig. 4). Since the foot trajectories are considered with
a certain defined height limit
max
y
and step length
max
x
, the desired search space size is equal to
max max
x
y×
area, which contains
1
x
n −
via points to
be assigned (a single via point per each
2: 1
x
xn=−
, while y locations are constrained by potentially
traversable by the robot obstacles only).
1
2
3
1234
START
W=4
K=1
W=9
K=2
W=12
K=3
W=16
K=3
W=12
K=4
5
3
8
4
9
7
6
3
5
5
7
6
9
3
4
5
W=9
K=1
W=5
K=1
W=8
K=1
W=9
K=1
W=9
K=1
W=8
K=2
8
5
4
9
8
6
3
5
8
4
7
6
3
5
1
1
4
5
3
4
2
4
6
4
4
1
W=8
K=2
W=12
K=1
Figure 3: A directed graph for optimal path selection using
dynamic programming approach.
Figure 4: Building directed graph in search area by creating
2D grid.
The algorithm for finding the optimal path works
as follows:
• For each node point
,ij
p
,
1i =
,
1, 1jny=+
calculate the weight (i.e., the cost), which
corresponds to the minimal time of the transition
to that node from the start point
0,0
p
. For each
node save the weight and joints angular
velocities at the end point of the corresponding
trajectory.
• For each node
,ij
p
where
2,inx=
,
1, 1jny=+
calculate the weight of transition from
1,ki
p
−
node, where
0, 1kny=+
. Find
min
k
, for which
Swing Leg Trajectory Optimization for a Humanoid Robot Locomotion
133

the sum of the calculated weight for a transition
from
min
,ki
p
and total weight of
min
,ki
p
node is
minimal. For each node
,ij
p
save
min
k , the total
weight and joints angular velocities, which are
calculated for the transition from
min
,ki
p
to
,ij
p
• For node
nx 1,0
p
+
(the end point) calculate the
weight of the transition from
nx,k
p
, where
0, 1kny=+
. Find
min
k
, for which the sum of the
calculated weight and the total weight of
min
nx,k
p
node is minimal.
• Obtain an optimal trajectory by tracking
backward k
min
values for each node:
1
min min
2
min
1, 1
,1,
0,0
1,
...
...
nx nx
nx ny
nx k nx k
k
ppp
pp
+
++
−
→→ →
→→
(3)
where
1
min
nx
k
+
is the optimal track for
1, 1nx ny
p
++
node.
Since the transition time between two node points
is used as a cost function, it is required to calculate
minimal traveling time from node
1,ki
p
−
to node
,ij
p
taking into account velocity and acceleration limits.
First, joint angles’ increments are calculated for each
transition between two adjacent nodes. Then we mark
a joint as active if it has larger absolute value of
angular increment for a given transition. Without loss
of generality let’s assume that joint 1 is active and
joint 2 is passive. Assuming that an active joint for
each interval moves either with a constant speed or a
constant acceleration (depending whether it reaches
the maximum velocity on previous interval), the joint
movements could be described as follows:
() () () 2
0.5
ii i
s
tat
ϕω
Δ= +
(4)
() () ()iii
es
at
ωω
=+
(5)
where
i=1 for an active joint and i=2 for a passive
joint,
ϕ
Δ is an angular increment,
s
ω
and
e
ω
are
angular velocities at start and end of the interval
respectively,
a is an angular acceleration (assumed to
be constant within the interval) and t is a transition
time.
In order to describe all possible relations between
active joints on adjacent intervals, three different
cases for calculating transition time should be
considered:
Case 1: Maximal Velocity. If an absolute angular
velocity of an active joint in
1,ki
p
−
node is equal to
maximum value
max
ω
and its sign is equal to the sign
of angular increment
ϕ
Δ , then
(1)
max
/t
ϕ
ω
=Δ
,
(1)
0a = ,
(1) (1)
es
ωω
=
. Substituting t into equations (4)
and (5) we obtain
(2)
a ,
(2)
e
ω
. It should be emphasized
that if the sign of angular increment
ϕ
Δ is opposite
to the current velocity sign at the interval beginning
than either Case 2 or Case 3 should be applied.
Case 2: Maximal Acceleration. If an absolute
angular velocity of an active joint in
1,ki
p
−
node is
below its maximum value
max
ω
or its sign is opposite
to the sign of angular increment
ϕ
Δ
, then we
substitute
(1)
ϕ
Δ
into equation (2) with
(1) (1)
max
()asign a
ϕ
=Δ
and solve the second order
equation with respect to
t:
(1) (1) 2 (1) (1)
(1)
()2
ss
ww a
t
a
ϕ
−± + Δ
=
(6)
Next, we select the lower positive root of the above
equation and calculate
(1) (1) (1)
es
at
ωω
=+
. If
(1)
e
ω
is
less than or equal to
max
ω
, than
(1)
a and
(1)
e
ω
are equal
to the calculated values. Finally, we substitute
t into
equations (4)-(5) to obtain
(2)
a ,
(2)
e
ω
.
Case 3: Reaching Maximal Velocity. If Case 1
condition is not satisfied and
(1)
e
ω
in Case 2 is greater
than
max
ω
, than
(1) (1)
max
()
e
sign
ω
ϕ
ω
=Δ
,
(1) (1) (1)
2/( )
es
t
ϕωω
=Δ +
,
(1) (1) (1)
()/
es
at
ωω
=−
. We
substitute t into equation (4)-(5) to obtain
(2)
a ,
(2)
e
ω
.
Figure 5 demonstrates all three cases which are
described above. For all cases we verify if the
calculated joint angular accelerations and velocities
are below their maximal values. If this condition
cannot be satisfied, such transition is excluded from a
possible path of the swing leg.
Figure 5: Three cases of angular velocity behavior: (1)
maximal velocity; (2) maximal acceleration; (3) reaching
maximal velocity
ICINCO 2016 - 13th International Conference on Informatics in Control, Automation and Robotics
134

5 SELECTING OPTIMAL
WALKING PRIMITIVE
It should be mentioned that for each given step length
and hip height a unique optimal walking primitive is
obtained. These primitives will have different weight
(traveling time) even in a case of an identical step
length but unequal hip heights. As a result, assigning
particular initial walking parameters affects robot
speed capacity. Therefore, considering different step
size and hip height it is possible to estimate robot’s
potential and to select the most appropriate primitive,
which ensures the best performance in terms of
robot’s speed. Another important issue that should be
also considered addresses particular characteristics of
the environment, i.e. presence of different obstacles
on the robot’s way, which could not be easily
circumambulated. To obtain an appropriate walking
primitive for negotiating a traversable obstacle (i.e.
stepping over such obstacle) the following algorithm
could be utilized.
Step 1: Specifying Constrains. Based on a
visible and technically traversable obstacle that
appears on the robot’s way, define feasible legs
locations for bipedal robot. The obstacle can be
approximated with rectangles and triangles that cover
all unreachable locations – as we address only 2D
case within a sagittal plane, only obstacle’s height and
length in robot’s walking direction are considered.
Step 2: Define Walking Parameter Limits.
Based on the robot geometry and current obstacle to
be negotiated, it is required to obtain reasonable
search intervals for step length and hip rising height,
i.e. their minimal and maximal values. Parameter
limits should be selected in such a way that at least
one trajectory with a continuous solution of inverse
kinematic problems exists between start and end
locations of a swing leg. Step size should be small
enough to detect extremum points.
Step 3: Finding Optimal Primitives for Each
Case.
For each combinations of a step length and a
hip rising height obtain optimal walking primitive and
traveling time using dynamic programming approach
presented in Section 4. If there is no solution of
inverse kinematic problem for at least one via point,
it is required either to decrease step size.
Step 4: Selection of an Optimal Walking
Primitive.
For each combination of walking
parameters estimate walking speed (in Cartesian
space) and select the optimal combination with regard
to a particular optimization criterion (i.e., the one with
the highest walking speed).
The above presented algorithm could be applied
for selecting optimal walking primitives for
humanoid robots with at least two DoFs within a
sagittal plane for each leg or could be further
extended for more DoF within a sagittal plane. It
gives a unique solution for the assigned initial
parameters of the robot and particular obstacle
properties, but the results are not being directly
scalable both between different robot models and
obstacles because of obstacles variety and physical
properties of various robots (link lengths, joint limits
for velocity and acceleration etc.). Thus, particularity
of robot kinematics always requires re-computing
optimal primitives for each specific model’s
parameters and each obstacle. Nevertheless, once
computed set of primitives for typical environment
conditions (i.e. different traversable obstacles) for a
particular robot model could be further applied for the
robot control for a whole set of robot motions.
6 SIMULATION RESULTS
The simulation of the algorithm was performed
within MATLAB/Simulink environment. The
acceleration and velocity limits were assigned to 1
rad/s
2
and 1 rad/s respectively for each joint. These
values correspond to technical characteristics of the
motors, which are used in AR-601M robot. First, let
us obtain optimal walking primitives for the fixed hip
rising height and step length and then compare results
with optimal parameters settings.
For the first case hip height was fixed to 0.5 m in
order to ensure optimal locomotion speed of the robot
based on our previous empirical studies (Khusainov
et al., 2016b, Khusainov et al., 2016a). According to
joint limits and link parameters we selected the robot
step length to be 0.4 m. For these parameters hip and
knee joint angles in their starting position were set to
0.1 and 0.55 rad correspondingly; at the end of the
trajectory (goal position) hip and knee joint angles
were set to -0.66 and 0.55 rad correspondingly. These
angles define
,ij
p
and
,ij
p
nodes.
Next, three different cases were analysed:
(i)
movement without any trajectory
constraints, i.e. in an ideal case without
velocity/acceleration limits the foot may
move straightforwardly from a start point to
an end point (Fig. 6);
(ii)
movement with 0.1 m barrier (with
negligible small size in the robot walking
direction) in the middle of the trajectory
(Fig. 7);
Swing Leg Trajectory Optimization for a Humanoid Robot Locomotion
135

Figure 6: Optimal trajectory without obstacles for hip
height 0.5 m and step length 0.4 m: (a) foot trajectory in
Cartesian space; (b) angular velocity of joints; (c) angular
acceleration of joints.
Figure 7: Trajectories with 0.1 m barrier in the middle for
hip height 0.5 m and step length 0.4 m: (a) optimal foot
trajectory in Cartesian space and cycloid trajectory; (b)
angular velocity of joints; (c) angular acceleration of joints.
(i) movement with 0.05×0.2 m box barrier in
the middle (Fig. 8).
Figure 8: Optimal trajectory with 0.05×0.2 m box barrier in
the middle for hip height 0.5 m and step length 0.4 m: (a)
optimal foot trajectory in Cartesian space; (b) angular
velocity of joints; (c) angular acceleration of joints.
The results demonstrated that for all cases the
obtained Cartesian trajectories of a swing leg do not
correspond to the shortest path and differ from a
cycloid path, which is traditionally used in bipedal
robot locomotion control. To compare our results
with a cycloid path approach, we built the cycloid
trajectory for (ii) case and ensured the same travelling
time as for our optimal trajectory (Fig. 7a). The
corresponding angular velocities are presented in
Fig. 9. The simulation demonstrated that for the
cycloid trajectory, the knee angular velocity exceeds
maximum value and the accelerations at the
beginning and at the end of the trajectory are very
high. That means that in practice it is impossible to
perform such trajectory within the specified time. In
order to move along a cycloid trajectory, it is required
to scale (increase) travelling time according to the
velocity limits. Hence, the proposed algorithm
succeeds to suggest a foot trajectory with a shorter
time interval comparing to a typical cycloid
trajectory.
In the case without trajectory constraints, the foot
rises up to 0.04 m, which is caused by joint
velocity/acceleration limits. It is evident that travel
time in such case is minimum. The particularity of
this trajectory is that the joint velocity limits are not
reached (see Fig. 6b).
ICINCO 2016 - 13th International Conference on Informatics in Control, Automation and Robotics
136
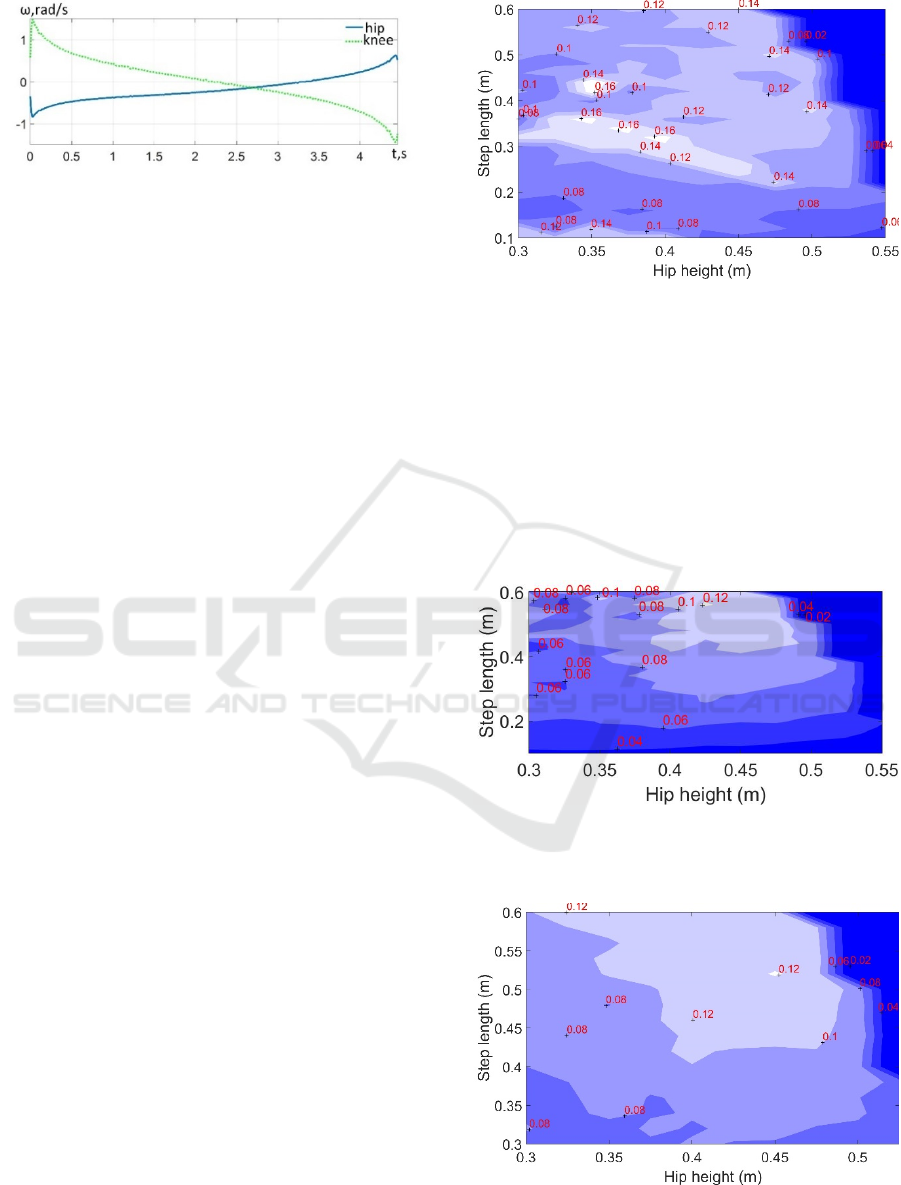
Figure 9: Angular velocities of joints for cycloid trajectory
movement with 0.1 m barrier, case of for hip height 0.5 m
and step length 0.4 m.
Barrier profile essentially effects optimal
trajectory (see Fig. 7a and Fig. 8a). Although the (ii)
case barrier is two times lower than for case (iii), the
height of the optimal trajectory for case (iii) and its
travel time are higher.
To compare efficiency of the obtained walking
primitives with conventional cycloids, the joint speed
and acceleration profiles have been obtained for
cycloids as well. It should be stressed, that these
profiles do not satisfy velocity and acceleration
limits, and to ensure such trajectory implementation
it is required to increase traveling time. In particular,
for free motions without obstacles the acceleration
limits have been exceeded by the factor 6, and to
remain within the limits traveling time should be over
10 s, which is twice higher that for the obtained
optimal trajectory. Another limitation of conventional
trajectory planning approach is its sensitivity to a
swing height and request to provide traveling time as
an input parameter. Our proposed approach does not
have these limitations and automatically estimates
minimal traveling time and an optimal swing leg
height.
Now, let us obtain optimal hip heights and step
length for all cases considered above and compare
robot performance. Speed maps for different step
length and hip height are presented in Fig. 10-12.
Here, lighter colour corresponds to higher speed and
are preferable for robot locomotion. It is shown that
Cartesian speed highly depends on the step length and
hip height and varies from one case to another. It is
also shown that optimal step parameters highly
depend on the size of the obstacle, which appears on
the robot path. In particular, for the case without
obstacles optimal settings are hip height of 0.4 m and
step length of 0.32 m, while for the case of box barrier
the optimal step length is much higher (0.52 m) and
hip height is almost the same (0.45 m).
Figure 10: Robot AR601M speed map for different step
length and hip height for the trajectory without obstacles.
Optimisation results are summarised in Table 1,
where for the three cases an optimal hip height, a step
length and a corresponding robot speed are given. For
comparison purposes it also contains robot speed for
the case of a fixed hip height and step length studied
above. It is shown that for the case of optimal hip and
step size parameters robot speed increases by 10-
23%, depending how far initial parameters were from
the optimal ones.
Figure 11: Robot AR601M speed map for different step
length and hip height for the trajectory with 0.1 m barrier in
the middle.
Figure 12: Robot AR601M speed map for different step
length and hip height for the trajectory with 0.05×0.2 m box
barrier in the middle.
Swing Leg Trajectory Optimization for a Humanoid Robot Locomotion
137

Table 1: Optimal walking parameters for locomotion of
bipedal humanoid robot AR-601M.
Case
Step
length
(m)
Hip
height
(m)
Speed (m/s)
Fixed
param.*
Optimal
param.**
(i) without
obstacles
0.32 0.40 0.13 0.16
(ii) with 0.1
m barrier
0.56
0.43 0.09 0.12
(iii) with
0.05×0.2 m
box barrier
0.52
0.45 0.11 0.12
*Fixed hip and step length parameters are 0.5 m and 0.4 m
respectively
**Optimal hip height and step length parameters
For comparison purposes Fig. 13-15 contain
walking primitives with joint velocities and
accelerations. It is shown that in the cases (ii) and (iii)
acceleration oscilations are essential (see Fig. 14c-
15c), which is mainly caused by problem
discretization and numerical calculation effects.
Nevertheless, these vibrations do not overcome
acceleration limits and will not affect robot
performance.
,
y
m
,/rad s
ω
,
x
m
,ts
,ts
2
,/arad s
a)
b)
c)
Figure 13: Optimal trajectory without obstacles for 0.4 m
hip height and 0.32 m step length: (a) foot trajectory in
Cartesian space; (b) angular velocity of joints; (c) angular
acceleration of joints.
A rather evident fact that for an optimal robot speed
without no obstacles, an optimal trajectory should be
close to the ground level was confirmed by simulation
results. It is clear that in practice such trajectory could
be hardly implemented (in fact, it is not possible to
have zero step height while locomotion), while it
demonstrates efficiency of the proposed approach.
With such moving primitive the robot can move with
0.16 m/s velocity instead of 0.13 that is maximal for
0.5 m hip height and 0.4 m step length. Similar
tendencies are observed for all considered cases.
,
y
m
,/rad s
ω
,
x
m
,ts
,ts
2
,/arad s
a)
b)
c)
Figure 14: Trajectories with 0.1 m barrier in the middle for
hip height 0.43 m and step length 0.56 m: (a) an optimal
foot trajectory in Cartesian space; (b) angular velocity of
joints; (c) angular acceleration of joints.
,
y
m
,/rad s
ω
,
x
m
,ts
,ts
2
,/arad s
a)
b)
c)
Figure 15: Optimal trajectory with 0.05×0.2 m box barrier
in the middle for hip height 0.45 m and step length0.52 m:
(a) an optimal foot trajectory in Cartesian space; (b) angular
velocity of joints; (c) angular acceleration of joints.
ICINCO 2016 - 13th International Conference on Informatics in Control, Automation and Robotics
138

7 DISCUSSIONS
In spite of numerous advantages, the proposed
walking trajectory optimization approach has several
apparent limitations, and the most significant one
among them is ignoring of dynamic and static effects
within optimization procedure. In fact, static effects
(compliance errors) are not critical for the trajectory
optimization since they are relatively small and could
be easily compensated by integrating a feedback
control from the feet force sensors. In this case the
main limitation for the moving primitive is avoiding
joint coordinates limits, which may not allow the
robot to compensate induced compliance errors. From
another side, if feedback control is not available
compliance errors should be computed using linear or
non-linear stiffness modelling (Klimchik et al., 2012,
Klimchik et al., 2014b) and control algorithm should
rely on the elasto-geometric model (Klimchik et al.,
2013, Klimchik et al., 2014a). It should be stressed
that stiffness parameters for real robot can be
obtained from the dedicated experimental study only
(Klimchik et al., 2015). So, statics effects the control
algorithm, but is not critical for optimization walking
primitives.
On the other side dynamic effects directly
influence robot stability (Majima et al., 1999, Mitobe
et al., 2000) and can be hardly compensated, since
this will directly affect walking primitive profile.
Since walking profile contains only foot coordinate,
humanoid torso and arms could be used to
additionally increase robot balance (Ude et al., 2004,
Yamaguchi et al., 1999). From another side,
integrating dynamic model into optimization
procedure may provide additional tool for trajectory
optimization. It may lead to faster robot movements
in the case when joint acceleration will be induced not
only because of actuation forces, but also by dynamic
forces. However, this approach essentially
complicates computations and may be hardly
implemented for robot control through joint angles
instead of demanded force level control. Besides,
swing leg does not contribute a lot in robot dynamics
since it does not effect robot body motion, which
mostly defining robot stability. In contrast, it is a
supporting leg (which is not considered in this work)
that mainly defines CoM trajectory and,
consequently, robot stability. From that point of view
supporting leg trajectory could be unambiguously
determined from stability condition while swing leg
coordinates are redundant variables that might be
optimised while step trajectory planning is proposed
in this work. So, the suggested in this paper approach
is a trade-off between a model complexity and
utilization of robot total capacities. In practice, to
avoid unpredictable robot behaviour, it is reasonable
not to use upper velocity/acceleration boundaries in
the optimization procedure since they may be higher
in real model because of a presence of dynamic forces
and errors in the model parameters.
The most essential limitation of the provided in
our paper results is related to kinematic constraints
induced to a hip location. It was strictly assumed that
the hip height remains the same along the trajectory,
while it is obvious that the best robot speed will be
achieved when the height varies along the trajectory.
In our approach, we separate swing and supporting
leg movements and consider only a swing leg
trajectory. Since a swing leg travels longer distances
in walking, its joints should apply higher speeds and
accelerations. Therefore, optimality due to kinematic
limits is more important for a swing leg. We consider
swing leg movement in coordinate system of a hip
where the hip is fixed. Another direction for
enhancing optimization efficiency is considering hip
speed as an additional optimization parameter, which
may vary from one via point to another. Providing
reasonable solutions of the above-mentioned
drawbacks and their integration into the optimization
algorithm will apparently lead to robot speed
increase. These issues will be addressed in details in
our future work.
8 CONCLUSIONS
In this paper we considered a problem of searching
optimal primitives for a swing leg trajectory, which
minimizes its travel time under joint angular velocity
and acceleration limits. Effective dynamic
programming approach was used to obtain a desired
optimal trajectory. It is shown that the obtained
optimal trajectory enables to decrease step time, i.e.
to increase robot speed compared to trajectories,
which had been traditionally used to control a swing
leg motion in bipedal robot locomotion. It is also
shown that the presented trajectory optimization
approach essentially increases speed of humanoid
robot AR-601M. The developed approach will be
further applied for optimization of a swing leg
trajectory with regard to a support leg and joint range
limits. Next, a set of optimal walking primitives will
be extended to the case of walking on the surface of
variable height (stairs and incline) as well as curved
paths that will bypass insurmountable obstacles and
walking in any direction. Besides, walking primitives
with variable hip height and hip speed will be
Swing Leg Trajectory Optimization for a Humanoid Robot Locomotion
139

considered as an objective for further optimization
algorithm enhancement.
ACKNOWLEDGEMENTS
This research has been supported by Russian Ministry
of Education and Science as a part of Scientific and
Technological Research and Development Program
of Russian Federation for 2014-2020 years (research
grant ID RFMEFI60914X0004) and by Android
Technics company, the industrial partner of the
research.
REFERENCES
Akhtaruzzaman, M. & Shafie, A. A. (2010) Evolution of
Humanoid Robot and contribution of various countries
in advancing the research and development of the
platform. Control Automation and Systems (ICCAS),
2010 International Conference on.
Channon, P., Hopkins, S. & Pham, D. (1992) Derivation of
optimal walking motions for a bipedal walking robot.
Robotica, 10 (02), 165-172.
Collins, S., Ruina, A., Tedrake, R. & Wisse, M. (2005)
Efficient Bipedal Robots Based on Passive-Dynamic
Walkers. Science, 307 (5712), 1082-1085.
Collins, S. H., Wisse, M. & Ruina, A. (2001) A three-
dimensional passive-dynamic walking robot with two
legs and knees. The International Journal of Robotics
Research, 20 (7), 607-615.
Erbatur, K. & Kurt, O. (2009) Natural ZMP Trajectories for
Biped Robot Reference Generation. IEEE Transactions
on Industrial Electronics, 56 (3), 835-845.
Escande, A., Kheddar, A. & Miossec, S. (2013) Planning
contact points for humanoid robots. Robotics and
Autonomous Systems, 61 (5), 428-442.
Gabbasov, B., Danilov, I., Afanasyev, I. & Magid, E.
(2015) Toward a human-like biped robot gait:
Biomechanical analysis of human locomotion recorded
by Kinect-based Motion Capture system. Mechatronics
and its Applications (ISMA), 2015 10th International
Symposium on.
Goswami, A. (1999) Postural Stability of Biped Robots and
the Foot-Rotation Indicator (FRI) Point. The
International Journal of Robotics Research, 18 (6),
523-533.
Ha, T. & Choi, C.-H. (2007) An effective trajectory
generation method for bipedal walking. Robotics and
Autonomous Systems, 55 (10), 795-810.
Hera, P. X. M. L., Shiriaev, A. S., Freidovich, L. B., Mettin,
U. & Gusev, S. V. (2013) Stable Walking Gaits for a
Three-Link Planar Biped Robot With One Actuator.
IEEE Transactions on Robotics, 29 (3), 589-601.
Hofmann, A., Popovic, M. & Herr, H. (2009) Exploiting
angular momentum to enhance bipedal center-of-mass
control. Robotics and Automation, 2009. ICRA '09.
IEEE International Conference on.
Kajita, S., Kanehiro, F., Kaneko, K., Fujiwara, K., Harada,
K., Yokoi, K. & Hirukawa, H. (2003) Biped walking
pattern generation by using preview control of zero-
moment point. Robotics and Automation, 2003.
Proceedings. ICRA '03. IEEE International Conference
on.
Kajita, S., Kanehiro, F., Kaneko, K., Yokoi, K. & Hirukawa,
H. (2001) The 3D linear inverted pendulum mode: a
simple modeling for a biped walking pattern generation.
Intelligent Robots and Systems, 2001. Proceedings. 2001
IEEE/RSJ International Conference on.
Katić, D. & Vukobratović, M. (2003) Survey of Intelligent
Control Techniques for Humanoid Robots. Journal of
Intelligent and Robotic Systems, 37 (2), 117-141.
Khusainov, R., Afanasyev, I. & Magid, E. (2016a)
Anthropomorphic robot modelling with virtual height
inverted pendulum approach in Simulink: step length
and period influence on walking stability. The 2016
International Conference on Artificial Life and
Robotics (ICAROB 2016). Japan.
Khusainov, R., Sagitov, A., Afanasyev, I. & Magid, E.
(2016b) Bipedal robot locomotion modelling with
virtual height inverted pendulum in Matlab-Simulink
and ROS-Gazebo environments. Journal of Robotics,
Networking and Artificial Life, 3 (1).
Khusainov, R., Shimchik, I., Afanasyev, I. & Magid, E.
(2015) Toward a human-like locomotion: Modelling
dynamically stable locomotion of an anthropomorphic
robot in simulink environment. Informatics in Control,
Automation and Robotics (ICINCO), 2015 12th
International Conference on.
Klimchik, A., Bondarenko, D., Pashkevich, A., Briot, S. &
Furet, B. (2014a) Compliance Error Compensation in
Robotic-Based Milling. In Ferrier, J.-L., Bernard, A.,
Gusikhin, O. & Madani, K. (Eds.) Informatics in
Control, Automation and Robotics: 9th International
Conference, ICINCO 2012 Rome, Italy, July 28-31,
2012 Revised Selected Papers. Cham, Springer
International Publishing.
Klimchik, A., Chablat, D. & Pashkevich, A. (2014b)
Stiffness modeling for perfect and non-perfect parallel
manipulators under internal and external loadings.
Mechanism and Machine Theory, 79, 1-28.
Klimchik, A., Furet, B., Caro, S. & Pashkevich, A. (2015)
Identification of the manipulator stiffness model
parameters in industrial environment. Mechanism and
Machine Theory, 90, 1-22.
Klimchik, A., Pashkevich, A., Caro, S. & Chablat, D.
(2012) Stiffness Matrix of Manipulators With Passive
Joints: Computational Aspects. IEEE Transactions on
Robotics, 28 (4), 955-958.
Klimchik, A., Pashkevich, A., Chablat, D. & Hovland, G.
(2013) Compliance error compensation technique for
parallel robots composed of non-perfect serial chains.
Robotics and Computer-Integrated Manufacturing, 29
(2), 385-393.
Majima, K., Miyazaki, T. & Ohishi, K. (1999) Dynamic
gait control of biped robot based on kinematics and
ICINCO 2016 - 13th International Conference on Informatics in Control, Automation and Robotics
140

motion description in Cartesian space. Electrical
Engineering in Japan, 129 (4), 96-104.
Mitobe, K., Capi, G. & Nasu, Y. (2000) Control of walking
robots based on manipulation of the zero moment point.
Robotica, 18 (06), 651-657.
Motoc, I. M., Sirlantzis, K., Spurgeon, S. & Lee, P. (2014)
Zero Moment Point/Inverted Pendulum-Based Walking
Algorithm for the NAO Robot. Emerging Security
Technologies (EST), 2014 Fifth International
Conference on.
Nakamura, M. (2004) Trajectory planning for a leg swing
during human walking. IEEE International Conference
on Systems, Man and Cybernetics.
Park, J. & Youm, Y. (2007) General ZMP Preview Control
for Bipedal Walking. Proceedings 2007 IEEE
International Conference on Robotics and Automation.
Pashkevich, A., Dolgui, A. & Chumakov, O. (2002)
Optimal control of robotic manipulator for laser cutting
applications. 15th Triennial World Congress of the
International Federation of Automatic Control.
Barcelona, SPAIN, 21th–26th July.
Rai, J. K. & Tewari, R. (2014) Quintic polynomial
trajectory of biped robot for human-like walking.
Communications, Control and Signal Processing
(ISCCSP), 2014 6th International Symposium on.
Ratliff, N., Zucker, M., Bagnell, J. A. & Srinivasa, S. (2009)
CHOMP: Gradient optimization techniques for
efficient motion planning. Robotics and Automation,
2009. ICRA '09. IEEE International Conference on.
Renders, J. M. & Flasse, S. P. (1996) Hybrid methods using
genetic algorithms for global optimization. IEEE
Transactions on Systems, Man, and Cybernetics, Part
B (Cybernetics), 26 (2), 243-258.
Righetti, L. & Auke Jan, I. (2006) Programmable central
pattern generators: an application to biped locomotion
control. Proceedings 2006 IEEE International
Conference on Robotics and Automation, 2006. ICRA
2006.
Sardain, P. & Bessonnet, G. (2004) Forces acting on a biped
robot. Center of pressure-zero moment point. IEEE
Transactions on Systems, Man, and Cybernetics - Part
A: Systems and Humans, 34 (5), 630-637.
Si, J., Yang, L., Chao, L., Jian, S. & Shengwei, M. (2009)
Approximate dynamic programming for continuous
state and control problems. Control and Automation,
2009. MED '09. 17th Mediterranean Conference on.
Tangpattanakul, P. & Artrit, P. (2009) Minimum-time
trajectory of robot manipulator using Harmony Search
algorithm. Electrical Engineering/Electronics,
Computer, Telecommunications and Information
Technology, 2009. ECTI-CON 2009. 6th International
Conference on.
Ude, A., Atkeson, C. G. & Riley, M. (2004) Programming
full-body movements for humanoid robots by
observation. Robotics and Autonomous Systems, 47 (2–
3), 93-108.
Vukobratović, M. & Borovac, B. (2004) Zero-moment
point — thirty five years of its life. International
Journal of Humanoid Robotics, 01 (01), 157-173.
Vukobratović, M. & Stepanenko, J. (1973) Mathematical
models of general anthropomorphic systems.
Mathematical Biosciences, 17 (3), 191-242.
Wright, J. & Jordanov, I. (2014) Intelligent Approaches in
Locomotion - A Review. Journal of Intelligent &
Robotic Systems, 80 (2), 255-277.
Yamaguchi, J., Soga, E., Inoue, S. & Takanishi, A. (1999)
Development of a bipedal humanoid robot-control
method of whole body cooperative dynamic biped
walking. Robotics and Automation, 1999. Proceedings.
1999 IEEE International Conference on.
Yussof, H., Ohka, M., Yamano, M. & Nasu, Y. (2008)
Analysis of Human-Inspired Biped Walk
Characteristics in a Prototype Humanoid Robot for
Improvement of Walking Speed. Modeling &
Simulation, 2008. AICMS 08. Second Asia
International Conference on.
Swing Leg Trajectory Optimization for a Humanoid Robot Locomotion
141
