Mueller Matrix Polarimetry by Means of Azimuthally Polarized Beams
and Adapted Commercial Polarimeter
Juan Carlos Gonz
´
alez de Sande
1
, Gemma Piquero
2
and Massimo Santarsiero
3
1
ETSIS de Telecomunicaci
´
on, Universidad Polit
´
ecnica de Madrid, Campus Sur 28031, Madrid, Spain
2
Departamento de
´
Optica, Universidad Complutense de Madrid, Ciudad Universitaria 28040, Madrid, Spain
3
Dipartimento di Ingegneria, Universit
`
a Roma Tre and CNISM, Via V. Volterra 62, 00146, Rome, Italy
Keywords:
Polarimetry, Polarization.
Abstract:
A simple method for Mueller matrix polarimetry is proposed. The experimental set up is based on using an
azimuthally polarized input beam, which presents all possible linearly polarized states across its transverse
section, and an adapted commercial light polarimeter for analyzing the polarization state of the output beam.
It will be shown that by measuring the Stokes parameters at only three different positions across the output
beam section, the complete Mueller matrix of linear deterministic samples can be easily determined.
1 INTRODUCTION
A useful way to represent the polarization state of
light is by means of Stokes parameters (Goldstein,
2003; Chipman, 2010; Azzam, 2016) that can be ar-
ranged in a 4 × 1 vector. In general, the polarization
state of an incident beam impinging onto a specimen
changes due to the interaction of light with the sam-
ple. The polarization state changes in a way that can
be described by a 4 × 4 real matrix known as Mueller
matrix. Different techniques have been used for deter-
mining the Mueller matrix of a specimen. It is neces-
sary to generate light with different polarization states
and to measure the output polarization states. In gen-
eral, this process involves a set of at least 16 measure-
ments (Chipman, 2010). Generally, uniformly polar-
ized light across the transverse section is used as in-
put light on the sample and a polarization analyzer is
used to determine the output polarization state after
the specimen.
Recently, light with non uniform polarization state
across its transverse section have been proposed for
simultaneuosly generating several states of polariza-
tion. This kind of beams can be used for Mueller ma-
trix polarimetry with a reduced number of measure-
ments (Tripathi and Toussaint, 2009; Kenny et al.,
2011). However, a problem that arise when using a
general nonuniformly polarized beam is that the po-
larization distribution across the section of the in-
cident light changes after propagation and, in many
cases, it is not easy to study how it evolves (Mart
´
ınez-
Herrero and Mej
´
ıas, 2010; Santarsiero et al., 2013;
de Sande et al., 2012). In this work we propose
two improvements: the first one is to use an az-
imuthally polarized beam (APB) (Gori, 2001; Zhan,
2009; Ram
´
ırez-S
´
anchez et al., 2009) to simultane-
ously generate all possible linearly polarized states
at once; the second one is to measure the output po-
larization state at only three points of the transverse
cross section of the output beam. To our knowl-
edge, there is only an experimental determination of
Mueller matrices using an APB as polarization state
generator, but the polarization state analyzer is com-
pletely different (de Sande et al., 2017).
APB is a particular case of a more general kind
of beams, the so called spirally polarized beams that
were introduced several years ago (Gori, 2001) and
has been experimentally synthesized and applied in
different areas (Zhan, 2009; Ram
´
ırez-S
´
anchez et al.,
2009; Ram
´
ırez-S
´
anchez et al., 2010). The polariza-
tion characteristics of an APB are invariant upon free
propagation, then the sample under test can be placed
at any plane along the beam (de Sande et al., 2017).
It is important to note that, although the polarization
state map of an APB changes after passing through a
sample, the output polarization map remains invariant
in propagation for homogeneous linear and determin-
istic samples. Then, the polarization analyzer of the
output beam can be positioned at any plane beyond
the sample.
Circular or elliptical states of polarization are not
generated in an APB. However, the three probing
de Sande J., Piquero G. and Santarsiero M.
Mueller Matrix Polarimetry by Means of Azimuthally Polarized Beams and Adapted Commercial Polarimeter.
DOI: 10.5220/0006091000390043
In Proceedings of the 5th International Conference on Photonics, Optics and Laser Technology (PHOTOPTICS 2017), pages 39-43
ISBN: 978-989-758-223-3
Copyright
c
 2017 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved
39

method (Oberemok and Savenkov, 2002) can be em-
ployed for obtainig a 4 × 3 partial Mueller matrix.
This can be accomplished by measuring, by means
of a commercial light polarimeter, the Stokes param-
eters at only three different positions across the trans-
verse section of the output light. This is sufficient to
obtain a 4 × 3 partial Mueller matrix of the specimen
under test. Taking into account the symmetry con-
straints of the Mueller matrices (Hovenier, 1994) for
the case of linear deterministic samples (Simon, 1990;
Gil, 2007), it is possible to recover the complete sam-
ple’s Mueller matrix.
After this Introduction, the theoretical foundations
of the proposed method are described in the next Sec-
tion. Afterwards, an experimental application of this
method and the obtained results are presented and, fi-
nally, the main findings of this work are summarized
in the Conclusions Section.
2 THEORETICAL BASIS
The Stokes parameters are measurable quantities that
describe the polarization state of a beam propagat-
ing along a given direction (Born and Wolf, 1980;
Chipman, 2010; Goldstein, 2003; Azzam, 2016). Al-
though it is usual to deal with uniformly polarized
light, in general, the Stokes parameters are functions
of the position vector r = (r,θ) in the transverse plane
of the beam. They can be arranged in a four dimen-
sional vector as
S(r,θ) =



S
0
(r,θ)
S
1
(r,θ)
S
2
(r,θ)
S
3
(r,θ)



(1)
where S
0
(r,θ) represents the intensity of the beam at
the point r, S
1
(r,θ) is equal to the difference between
the amount of light linearly polarized at 0 and at π/2,
S
2
(r,θ) is analogous to S
1
(r,θ) but considering lin-
ear polarization states at π/4 and −π/4, and finally,
S
3
(r,θ) is the difference of right and left circular po-
larized intensity at such point.
The polarization state of light changes when it
passes through an optical system or sample. The rela-
tion between the output light Stokes vector, S
out
and
the input Stokes vector, S
in
, is described by
S
out
(r,θ) =
b
MS
in
(r,θ) , (2)
where
b
M =



m
00
m
01
m
02
m
03
m
10
m
11
m
12
m
13
m
20
m
21
m
22
m
23
m
30
m
31
m
32
m
33



(3)
is the 4 × 4 Mueller matrix representing the polariza-
tion changes produced by the interacting object.
To experimentally determine the 16 elements, m
i j
,
i, j = 0,1,2,3, of the sample’s Mueller matrix, at least
16 measurements have to be done. Usually, a polar-
ization state generator that produce at least four states
whose Stokes vectors are linearly independent is used
to modify the polarization state of the probing beam
and the projection of the output light onto 4 polar-
ization states with linearly independent Stokes vector
are measured for obtaining the complete Mueller ma-
trix (Azzam, 2016; Chipman, 2010; Goldstein, 2003).
Azimuthally polarized beams are nonuniformly
totally polarized beams, whose electric field vector at
each point is directed along the azimuthal direction.
For these beams, the Stokes vector is given by (Gori,
2001; Ram
´
ırez-S
´
anchez et al., 2009)
S
APB
= I(r)



1
− cos(2θ)
− sin(2θ)
0



, (4)
where I(r, θ) is the irradiance of the incident beam,
which must be zero at the center because the polar-
ization is not defined at that point.
By measuring the output intensity (first Stokes pa-
rameter S
out
0
(r,θ)) at different points of the output
beam cross section (at three different θ angles) and
measuring the polarization state of the input beam at
the same positions, the following set of linear equa-
tions can be written


S
out
0
(r
0
,θ
0
)
S
out
0
(r
1
,θ
1
)
S
out
0
(r
2
,θ
2
)


=
b
W


m
00
m
01
m
02


, (5)
where
b
W is the polarimetric measurement matrix
given by
b
W =


S
in
0
(r
0
,θ
0
) S
in
1
(r
0
,θ
0
) S
in
2
(r
0
,θ
0
)
S
in
0
(r
1
,θ
1
) S
in
1
(r
1
,θ
1
) S
in
2
(r
1
,θ
1
)
S
in
0
(r
2
,θ
2
) S
in
1
(r
2
,θ
2
) S
in
2
(r
2
,θ
2
)


. (6)
Note that S
in
3
(r,θ) = 0 for any point at the cross sec-
tion of an APB.
By properly selecting three different points
(r
k
,θ
k
), in such a way that the input polarization states
are represented by three linearly independent Stokes
vector, the elements m
0 j
with j = 0, 1,2 can be ob-
tained by inverting Eq. (5).
In a similar way, if the second, third, and forth
Stokes parameters of the output beam (S
out
1
, S
out
2
, and
S
out
3
, respectively) are measured at the same points of
the transverse section of the beam, the elements m
1 j
,
m
2 j
, and m
3 j
respectively, can be obtained from the
PHOTOPTICS 2017 - 5th International Conference on Photonics, Optics and Laser Technology
40

following equation:


S
out
i
(r
0
,θ
0
)
S
out
i
(r
1
,θ
1
)
S
out
i
(r
2
,θ
2
)


=
b
W


m
i0
m
i1
m
i2


, (7)
where i = 1,2,3. It must be noted that the polarimetric
measurement matrix
b
W is the same for obtaining the
four rows of the Mueller submatrix formed by the first
three columns.
Minimization of the condition number of the po-
larimetric measurement matrix gives the optimum po-
sitions for measuring the output beam Stokes param-
eters (Peinado et al., 2015; Oberemok and Savenkov,
2002; Zallat et al., 2006). The optimum posi-
tions when using input linear polarization correspond
to points where the input polarization linear states
have azimuths equally spaced by π/3 (Oberemok and
Savenkov, 2002) and where the intensity of the input
beam is close to its maximum.
Several symmetry constraints hold for the Mueller
matrix elements (Hovenier, 1994) of linear determin-
istic samples (Simon, 1990). They can be used to
recover the last column of the sample Mueller ma-
trix (Swami et al., 2013), however, the way these au-
thors propose to recover the Mueller matrix could be
cumbersome when experimental errors are accounted
for. In the present work, we minimize a cost func-
tion formed as the sum of the squares of the left hand
side of Eqs. (42), (43) and the remaining 28 relations
represented by the pictograms of Fig. 1 in Hovenier,
1994.
3 APPLICATION AND
EXPERIMENTAL RESULTS
In order to test the proposed system we have used
an APB synthesized by means of an Arcoptix crys-
tal polarization converter (PC) as shown in the set
up of Fig. 1. A He-Ne laser stabilized in intensity
and frequency is used as light source. A linear polar-
izer P
1
selects the incident state of polarization, which
must be linearly polarized along a direction parallel
to the PC axis, in order for the PC to work prop-
erly (Ram
´
ırez-S
´
anchez et al., 2009; Ram
´
ırez-S
´
anchez
et al., 2010). A microscope objective O
1
and a lens L
1
are used to expand the beam impinging onto the PC.
After the PC, the beam is spatially filtered and colli-
mated by means of a microscope objective, a 25µm
diameter pinhole, and a converging lens (O
2
, PH, and
L
2
, respectively).
The propagated light is analyzed by means of
a commercial light measuring polarimeter (Thorlabs
TXP polarimeter) that can be positioned at any plane
He-Ne Laser
P
1
MO
1
L
1
PC
MO
2
PH
L
2
S
TXP
Figure 1: Proposed experimental setup. P: linear polarizers;
MO: microscope objectives; L: lenses; PC: polarization
converter; PH: Pinhole; S: sample; T XP: light polarimeter.
after the sample due to the invariability of the polar-
ization distribution after the sample. This light po-
larimeter (T X P), mounted on a XY −micropositioner
stage, has been modified by inserting a 500 µm di-
ameter pinhole at its entrance in order to select a
small enough area where the polarization state could
be considered as nearly uniform. By means of the
T XP light polarimeter, the Stokes parameters of the
input and output beams are measured at three differ-
ent points located at three equally spaced by π/3 di-
rections and at a distance from the center of the beam
where the intensity is near to its maximum (see Table
1 and Table 2).
As homogeneous, deterministic and transparent
test sample we have used a quarter-wave plate (QWP)
with its axes at 0 and at +π/6 relative to the x axis.
The Stokes parameters of the output beam were mea-
sured by means of the modified T XP polarimeter
at the same three points than those where the input
beam has been previously characterized (see Table 1
and Table 2). By using Eq. (7) with the four mea-
sured Stokes parameters S
out
i
(r
k
,θ
k
) with i = 0,1,2,3,
at three different points k = 0, 1,2, the first three
columns of the sample’s Mueller matrix are obtained.
As it has been already commented, when dealing with
linear deterministic samples, as is the present case,
the last column can be recovered by imposing several
symmetry constraints that the sample Mueller matrix
elements obey (Hovenier, 1994).
Figure 2 shows the experimental values measured
for a QWP with its axes at two different angles, 0 and
at +π/6, relative to the x axis. As it can be noted in
Fig. 3, the absolute value of the differences between
theoretical ideal QWP Mueller matrix elements and
the corresponding experimentally obtained values are
very small (less than 0.025), so the proposed method
is suitable for characterizing linear deterministic sam-
ples.
Mueller Matrix Polarimetry by Means of Azimuthally Polarized Beams and Adapted Commercial Polarimeter
41

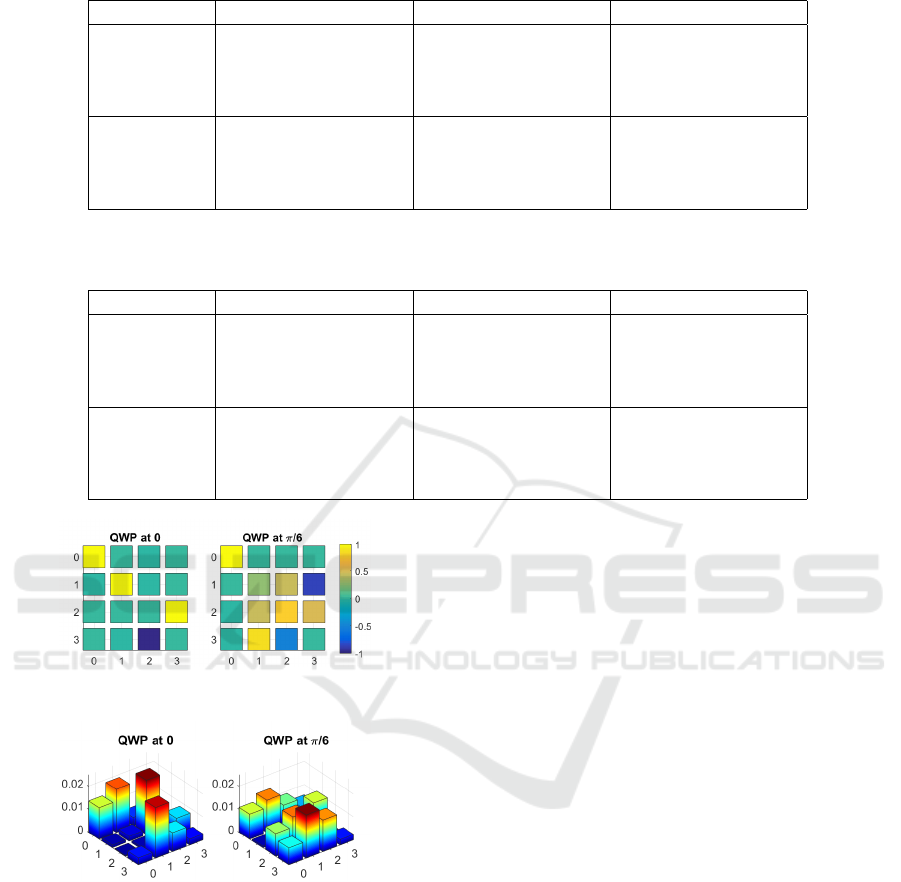
Table 1: Measured Stokes vectors, normalized to the maximum input intensity, of the input and output beam at three different
positions for the case of a QWP at 0.
Position (r
0
,θ
0
) (r
1
,θ
0
+ π/3) (r
2
,θ
0
− π/3)
Input beam S
in
=



1.0000
−0.9367
−0.0048
−0.0188



S
in
=



0.9014
0.4422
0.7676
0.0565



S
in
=



0.8280
0.4062
−0.7040
−0.0265



Output beam S
out
=



0.9841
−0.9504
0.0011
0.0477



S
out
=



0.9207
0.4345
0.0020
−0.8036



S
out
=



0.8703
0.4430
−0.0019
0.7321



Table 2: Measured Stokes vectors, normalized to the maximum input intensity, of the input and output beam at three different
positions for the case of a QWP at π/6.
Position (r
0
,θ
0
) (r
1
,θ
0
+ π/3) (r
2
,θ
0
− π/3)
Input beam S
in
=



0.9607
−0.8818
0.0003
−0.0201



S
in
=



1.0000
0.4815
0.8331
0.0153



S
in
=



0.9971
0.4841
−0.8380
−0.0234



Output beam S
out
=



0.9508
−0.2254
−0.4091
−0.7624



S
out
=



0.9767
0.4691
0.8107
−0.0004



S
out
=



0.9736
−0.2355
−0.4193
0.8265



Figure 2: Experimental Mueller matrix for a QWP with its
axes at 0 (left) and rotated π/6 (right) relative to the x axis.
Figure 3: Absolute values of the differences between the-
oretical and experimental Mueller matrix elements for a
QWP with its axes at 0 (left) and rotated π/6 (right) rela-
tive to the x axis.
4 CONCLUSIONS
An effective and easy method to obtain the Mueller
matrix of linear deterministic samples is proposed and
experimentally tested. The method is based on using
azimuthally polarized beams as a continuous polar-
ization generator and a commercial adapted polarime-
ter. This kind of beams presents invariant polariza-
tion characteristics under free propagation. Moreover,
when this kind of beams passes through a linear de-
terministic system or sample, the output polarization
distribution also remains invariant under free propa-
gation. Then, both the sample and the light measuring
polarimeter can be placed at any plane along the beam
propagation. Measurement of the Stokes parameters
at only three different points suffices for obtaining the
first three columns of the sample’s Mueller matrix.
The well known constraints for the Mueller matrix el-
ements in the case of linear deterministic samples are
exploited to recover the complete Mueller matrix. Ex-
perimental results confirm the validity of the proposed
method.
ACKNOWLEDGEMENTS
This work has been supported by Spanish Minis-
terio de Econom
´
ıa y Competitividad under projects
FIS2013-46475 and FIS2016-75147.
REFERENCES
Azzam, R. M. A. (2016). Stokes-vector and Mueller-matrix
polarimetry [Invited]. J. Opt. Soc. Am. A, 33(7):1396–
1408.
Born, M. and Wolf, E. (1980). Principles of Optics. Cam-
bridge University Press, sixth (corrected) edition.
PHOTOPTICS 2017 - 5th International Conference on Photonics, Optics and Laser Technology
42

Chipman, R. A. (2010). Polarimetry, volume I, chapter 15,
pages 1 – 46. McGraw-Hill Companies, third edition.
de Sande, J. C. G., Santarsiero, M., and Piquero, G. (2017).
Spirally polarized beams for polarimetry measure-
ments of deterministic and homogeneous samples.
Optics and Lasers in Engineering, 91:97 – 105.
de Sande, J. C. G., Santarsiero, M., Piquero, G., and Gori,
F. (2012). Longitudinal polarization periodicity of un-
polarized light passing through a double wedge depo-
larizer. Opt. Express, 20(25):27348–27360.
Gil, J. J. (2007). Polarimetric characterization of light
and media. The European Physical Journal Applied
Physics, 40:1 – 47.
Goldstein, D. H. (2003). Polarized Light. Marcel Dekker,
Inc., second (revised and expanded) edition.
Gori, F. (2001). Polarization basis for vortex beams. J. Opt.
Soc. Am. A, 18(7):1612–1617.
Hovenier, J. W. (1994). Structure of a general pure Mueller
matrix. Appl. Opt., 33(36):8318–8324.
Kenny, F., Rodr
´
ıguez, O., Lara, D., and Dainty, C. (2011).
Vectorial polarimeter using an inhomogeneous po-
larization state generator. In Frontiers in Optics
2011/Laser Science XXVII, page FThQ5. Optical So-
ciety of America.
Mart
´
ınez-Herrero, R. and Mej
´
ıas, P. M. (2010). On the
propagation of random electromagnetic fields with
position-independent stochastic behavior. Optics
Communications, 283(22):4467 – 4469. Electromag-
netic Coherence and Polarization.
Oberemok, E. A. and Savenkov, S. N. (2002). Determi-
nation of the polarization characteristics of objects by
the method of three probing polarizations. Journal of
Applied Spectroscopy, 69(1):72–77.
Peinado, A., Lizana, A., Turp
´
ın, A., Iemmi, C., Kalkand-
jiev, T. K., Mompart, J., and Campos, J. (2015). Op-
timization, tolerance analysis and implementation of
a Stokes polarimeter based on the conical refraction
phenomenon. Opt. Express, 23(5):5636–5652.
Ram
´
ırez-S
´
anchez, V., Piquero, G., and Santarsiero, M.
(2009). Generation and characterization of spirally
polarized fields. Journal of Optics A: Pure and Ap-
plied Optics, 11(8):085708.
Ram
´
ırez-S
´
anchez, V., Piquero, G., and Santarsiero, M.
(2010). Synthesis and characterization of partially
coherent beams with propagation-invariant trans-
verse polarization pattern. Optics Communications,
283(22):4484 – 4489. Electromagnetic Coherence and
Polarization.
Santarsiero, M., de Sande, J. C. G., Piquero, G., and Gori,
F. (2013). Coherencepolarization properties of fields
radiated from transversely periodic electromagnetic
sources. Journal of Optics, 15(5):055701.
Simon, R. (1990). Nondepolarizing systems and degree
of polarization. Optics Communications, 77(5):349
– 354.
Swami, M., Patel, H., and Gupta, P. (2013). Conversion
of 3×3 Mueller matrix to 4×4 Mueller matrix for
non-depolarizing samples. Optics Communications,
286:18 – 22.
Tripathi, S. and Toussaint, K. C. (2009). Rapid Mueller
matrix polarimetry based on parallelized polariza-
tion state generation and detection. Opt. Express,
17(24):21396–21407.
Zallat, J., S, A., and Stoll, M. P. (2006). Optimal configura-
tions for imaging polarimeters: impact of image noise
and systematic errors. Journal of Optics A: Pure and
Applied Optics, 8(9):807.
Zhan, Q. (2009). Cylindrical vector beams: from mathe-
matical concepts to applications. Adv. Opt. Photon.,
1(1):1–57.
Mueller Matrix Polarimetry by Means of Azimuthally Polarized Beams and Adapted Commercial Polarimeter
43