Moving Bragg Grating Solitons in a Grating-assisted Coupler with
Cubic-Quintic Nonlinearity
Md. Jahirul Islam and Javid Atai
School of Electrical and Information Engineering, The University of Sydney, NSW 2006, Sydney, Australia
Keywords:
Gap Soliton, Fiber Bragg Grating, Cubic-Quintic Nonlinearity.
Abstract:
We analyze the existence of moving Bragg grating solitons in a semilinear coupled system where one core is
equipped with a Bragg grating and has cubic-quintic nonlinearity and the other is linear. The system’s linear
spectrum contains three bandgaps, namely the upper, lower and central gaps. The bandgap edges shift with
the soliton velocity (s) and group velocity mismatch term (c) for a given coupling coefficient (κ), and result
in change in the spectral widths. Two families of moving Bragg grating solitons (referred to as Type 1 and
Type 2) are found that fill the upper and lower gaps only. No moving solitons are found in the central gap.
The border separating the two families depends on both c and s, and is determined numerically. We carried
out systematic numerical stability analysis of the moving solitons and identified non-trivial stability borders in
their parametric plane. The analysis also reveals that vast areas of stable Type 1 solitons exist in the system’s
parametric plane and that all Type 2 solitons are unstable.
1 INTRODUCTION
It is well known that the coupling between forward-
and backward-propagating waves gives rise to a
strong dispersion in fiber Bragg gratings (FBGs) that
can be up to six orders of magnitude greater than that
of the silica fiber (Desterke and Sipe, 1994). At suffi-
ciently high intensities, the grating induced dispersion
can be counterbalanced by nonlinearity resulting in
the formation of Bragg grating (BG) solitons. One of
the main features of these solitons is that their veloc-
ity can range from zero to the velocity of light in the
medium (Aceves and Wabnitz, 1989; Christadoulides
and Joseph, 1989; Neill and Atai, 2007; Mak et al.,
2003). As a result, such solitons are considered as
potential candidates for optical buffers, delay lines,
optical memory elements and logic gates. Thus far,
BG solitons with a velocity of 0.16c
0
(where c
0
is the
speed of light in the vacuum) have been demonstrated
experimentally (Mok et al., 2006).
Owing to their potential applications, the exis-
tence and dynamics of BG solitons have been investi-
gated extensively in a variety of settings and struc-
tures including quadratic nonlinearity (Mak et al.,
1998b; Conti et al., 1997; He and Drummond, 1997),
cubic-quintic nonlinearity (Atai and Malomed, 2001),
Bragg gratings in sign-changing Kerr media (Atai and
Malomed, 2002), semilinear dual-core system with
Kerr nonlinearity (Atai and Malomed, 2000), coupled
FBGs (Mak et al., 1998a; Mak et al., 2004; Tsofe and
Malomed, 2007; Islam and Atai, 2015), grating su-
perstructures (Mayteevarunyoo and Malomed, 2008),
waveguide arrays (Dong et al., 2011), and photonic
crystals (Skryabin, 2004; Atai et al., 2006).
Optical fiber couplers have received much atten-
tion over the past three decades due to their poten-
tial applications in signal processing and switching
(Fraga et al., 2006; Kaup and Malomed, 1998; Jensen,
1982). In particular, couplers made of dissimilar
cores (e.g. one nonlinear core coupled with a lin-
ear core) have shown to possess interesting switch-
ing characteristics (Atai and Chen, 1992; Atai, 1993).
Therefore, one may anticipate that combination of
such couplers and Bragg gratings lead to optical de-
vices with novel switching capabilities. In this paper,
we investigate the existence and stability of moving
BG solitons in a grating-assisted coupler where one
core has cubic-quintic nonlinearity and is equipped
with a Bragg grating and the other core is linear.
2 THE MODEL
We consider a semilinear dual-core system, where one
core is equipped with a Bragg grating and has cubic-
quintic nonlinearity and the other core is linear. Start-
44
Jahirul Islam M. and Atai J.
Moving Bragg Grating Solitons in a Grating-assisted Coupler with Cubic-Quintic Nonlinearity.
DOI: 10.5220/0006098800440048
In Proceedings of the 5th International Conference on Photonics, Optics and Laser Technology (PHOTOPTICS 2017), pages 44-48
ISBN: 978-989-758-223-3
Copyright
c
 2017 by SCITEPRESS – Science and Technology Publications, Lda. All rights reserved

-30 -20 -10 0 10 20 30
-20
-10
0
10
20
s = 0.1
s = 0.2
s = 0.4
k
Ω
Figure 1: Dispersion curves in the moving frames for κ =
10.0, c = 0.2 with different values of soliton velocity s.
ing with the model of (Atai and Malomed, 2000),
one can derive the following model that describes the
propagation of light in such a system:
iu
t
+ iu
x
+

|v|
2
+
1
2
|u|
2

u−
q

1
4
|u|
4
+
3
2
|u|
2
|v|
2
+
3
4
|v|
4

u+ v+ κφ = 0,
iv
t
− iv
x
+

|u|
2
+
1
2
|v|
2

v−
q

1
4
|v|
4
+
3
2
|v|
2
|u|
2
+
3
4
|u|
4

v+ u+ κψ = 0,
iφ
t
+ icφ
x
+ κu = 0,
iψ
t
−icψ
x
+ κv = 0,
(1)
where u and v are the forward- and backward-
propagating waves in the nonlinear core, and φ and ψ
are their counterparts in the linear core, respectively.
q > 0 controls the strength of the quintic nonlinear-
ity and κ denotes the coupling coefficient between the
cores. Also, c represents the relative group velocity in
the linear core (group velocity in the nonlinear core
has been set to 1).
To determine the linear bandgaps within
which moving solitons may exist, Eqs. (1) must
be transformed into the moving coordinates,
{X, T} = {x − st,t}, where s is the soliton velocity
normalized such a way that s = 1 denotes the speed
of light in the medium. Using this transformation,
one can obtain the following system of equations:
iu
T
+ i(1− s)u
X
+

|v|
2
+
1
2
|u|
2

u−
q

1
4
|u|
4
+
3
2
|u|
2
|v|
2
+
3
4
|v|
4

u+ v+ κφ = 0,
iv
T
− i(1+ s)v
X
+

|u|
2
+
1
2
|v|
2

v−
q

1
4
|v|
4
+
3
2
|v|
2
|u|
2
+
3
4
|u|
4

v+ u+ κψ = 0,
iφ
T
+ i(c− s)φ
X
+ κu = 0,
iψ
T
−i(c+ s)ψ
X
+ κv = 0.
(2)
To determine the linear bandgap, u, v, φ, ψ ∼ e
ikX−iΩT
is substituted into Eqs. (2), which results in the fol-
lowing dispersion relation:
Ω
4
+ 4ksΩ
3
−

1+ 2κ
2
+

1+ c
2
− 6s
2

k
2

Ω
2
− 2ks

1+ 2κ
2
+

1+ c
2
− 2s

k
2

Ω+

c
2
−s
2
− 2cκ
2
−
2κ
2
s
2

k
2
+ κ
4
+

c
2
−

1+ c
2

s
2
+ s
4

k
4
= 0, (3)
where k represents the wave number, and Ω is the fre-
quency in the moving frame and is related to the fre-
quency in the lab frame by Ω(k) = ω(k) − sk. Figure
1 displays the dispersion diagrams corresponding to
Eq. (3) for κ = 10.0, c = 0.20 with different soliton
velocities. The spectrum analysis reveals that similar
to the quiescent BG solitons with c 6= 0, moving spec-
trum contains three bandgaps: the upper, lower and
central gaps. However, none of these three bandgaps
are genuine as they overlap with one branch of contin-
uous spectrum. The bandgap edges strongly depend
on both c and s for a given κ. It is also found that
increasing c results in the enlargement of both the up-
per and lower gaps. On the other hand, higher soliton
velocities shrink the bandgap widths. Interestingly,
unlike the coupled BG system with Kerr nonlinearity
(Mak et al., 1998a), both the upper and lower gaps
disappear above a critical soliton velocity (s
cr
).
In order to determine the moving
BG soliton solutions, we use the ansatz

u(X, T),v(X,T), φ(X,T), ψ(X, T)

=

U(X),V(X),Φ(X), Ψ(X)

e
−iΩT
. Substitution
of this expression into Eqs. (2) leads to a system
of ordinary differential equations that is solved
numerically for different system parameters. It is
found that BG soliton solutions do not exist in the
central gap. On the other hand, in the upper and lower
gaps, similar to the case of single-core (Atai and
Malomed, 2001) Bragg grating with cubic-quintic
nonlinearity, two disjoint families of solitons (i.e.
Type 1 and Type 2) are found. Type 1 and Type 2
moving solitons differ in their amplitudes, phase
structures and parities. One noteworthy feature is
that the borders separating the two families strongly
Moving Bragg Grating Solitons in a Grating-assisted Coupler with Cubic-Quintic Nonlinearity
45

9.6
9.9
10.2
10.51
0 0.2 0.4
0.6
0.8 1
-10.51
-10.2
-9.9
-9.6
Stable(Type 1)
Unstable(Type 1)
q
Ω
No Soliton Solutions
Stable(Type 1)
Unstable(Type 1)
Unstable(Type 2)
(a)
Unstable(Type 2)
9.6
9.9
10.2
10.51
0 0.2 0.4
0.6
0.8 1
-10.51
-10.2
-9.9
-9.6
Stable(Type 1)
Unstable(Type 1)
q
Ω
No Soliton Solutions
Stable(Type 1)
Unstable(Type 1)
Unstable(Type 2)
(b)
Unstable(Type 2)
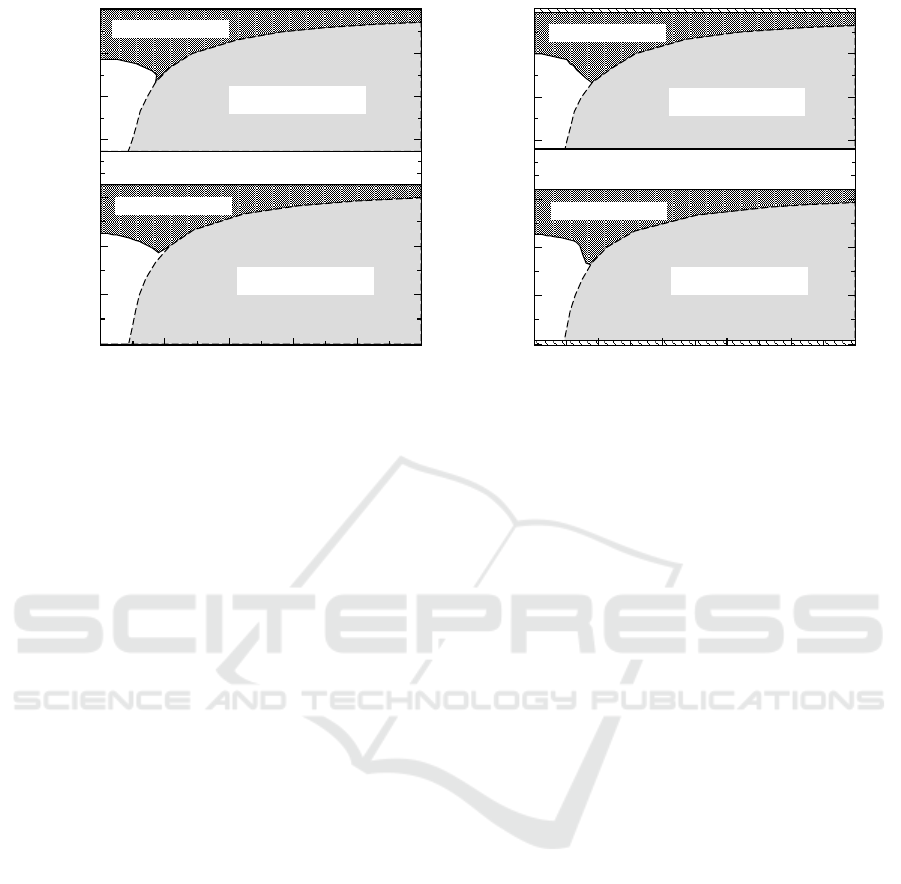
Figure 2: Stability diagrams of moving solitons plotted in the (q, Ω) plane for κ = 10.0 and c = 0.2 with velocities (a) s = 0.1
and (b) s = 0.2. The dashed curves represent the borders between Type 1 and Type 2 soliton families. Also, the regions
represented by diagonal lines indicate the regions outside the upper and lower gaps, where solitons do not exist.
depend on both c and s, and numerical approach is
used to determine the borders.
3 STABILITY OF MOVING
BRAGG GRATING SOLITONS
To determine the stability of moving solitons, we
have performed a systematic stability analysis by nu-
merically solving Eqs. (2) using a split-step Fourier
method. In all simulations, the soliton solutions of
Eqs. (2) were propagated for t = 2000. It is found
that intrinsic numerical noise was sufficient to trigger
the instability development.
The results of the stability analysis are summa-
rized in the (q, Ω) plane and a set of stability diagrams
is displayed in Figure 2. Our results demonstrate that
Type 2 moving solitons are always unstable. This is
in stark contrast to the single-core BG model where
it was shown that in certain parameter ranges stable
Type 2 solitons exist (Atai and Malomed, 2001). On
the other hand, vast regions exist where stable Type
1 moving solitons are found. The presence of quintic
nonlinearity initially results in expansion of the stabil-
ity regions. It is found that, in general, as the velocity
of solitons increases the stable Type 1 regions in the
upper and lower gaps shrink. In the examples shown
in Figures 2 (a) and (b), the stability regions shrink
by approximately 2% and 1% for the upper and lower
gaps, respectively.
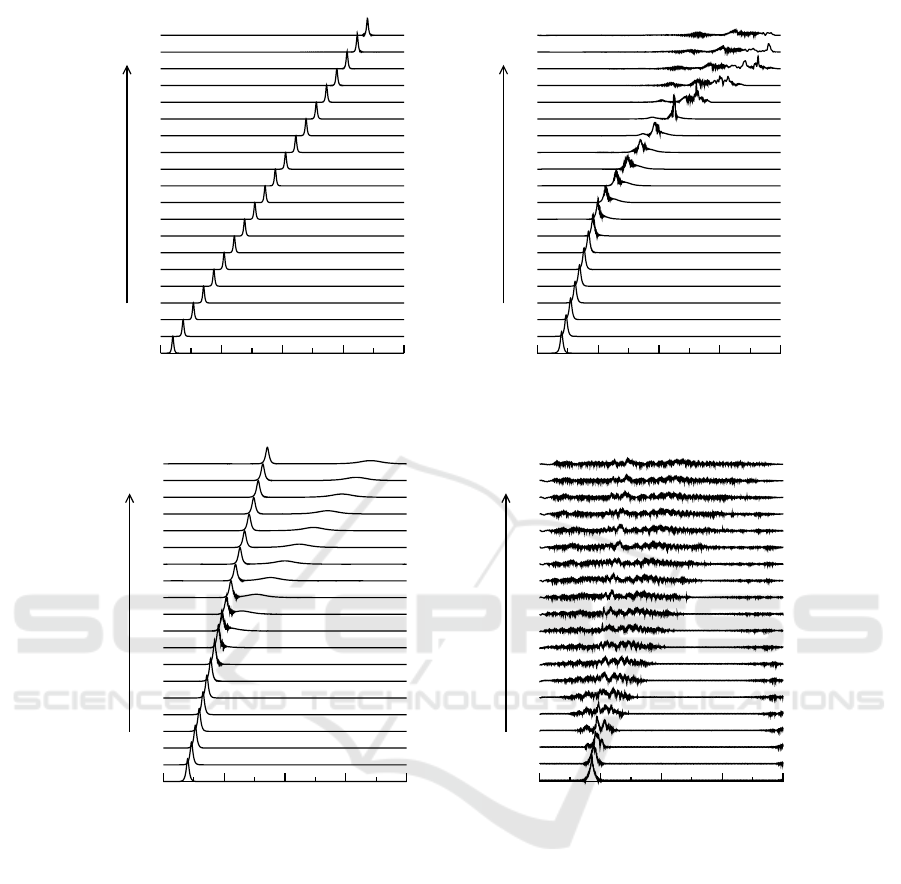
Examples of the evolution of both Type 1 and
Type 2 solitons are shown in Figure 3. The instability
development of Type 1 moving solitons can lead to
several outcomes. Solitons far from the stability bor-
der radiate some energy and decay into radiation upon
propagation (see Figure 3(b)). In certain parameter
ranges, the instability development can result in split-
ting of moving solitons and formation of two moving
solitons with different velcoties (see Figure 3(c)). An
example of stable Type 1 soliton is displayed in Fig-
ure 3(a). As for Type 2 solitons, they are unstable
and are completely destroyed upon propagation (see
Figure 3(d)).
4 CONCLUSIONS
We have numerically investigated the bandgap and
stability characteristics of moving solitons in a semi-
linear coupled system, in which one core is com-
pletely linear, and the other has cubic-quintic non-
linearity and is equipped with a Bragg grating. It
is found that the model supports three bandgaps
and moving soliton solutions exist only in the upper
and lower bandgaps. The widths and edges of the
bandgaps change with the system parameters, such as
the group velocity mismatch term (c), soliton veloc-
ity (s) and coupling coefficient (κ) between the cores.
Similar to the quiescent case, two families of solitons
known as Type 1 and Type 2 were found in the (q, Ω)
plane. The border separating the two families was de-
termined numerically and found to be dependent on
both c and s for a given κ. We have investigated sta-
bility of the moving solitons. The stability analysis
PHOTOPTICS 2017 - 5th International Conference on Photonics, Optics and Laser Technology
46

-250 -125
0
125 250
2000
t
0
X
(a)
-100
-50
0
50
100
700
t
0
X
(b)
-100
-50
0
50
100
600
t
0
X
(c)
-70
-35
0
35
70
100
t
0
X
(d)
Figure 3: Examples of moving BG soliton evolution. (a) Stable Type 1 soliton with κ = 10.0, c = 0.20, s = 0.20, q = 0.15,
Ω = 10.35; (b) unstable Type 1 soliton with κ = 5.0, c = 0.10, s = 0.10, q = 0.10, Ω = 5.05; (c) unstable Type 1 soliton with
κ = 10.0, c = 0.20, s = 0.10, q = 0.10, Ω = 10.00; and (d) unstable Type 2 soliton with κ = 1.0, c = 0.10, s = 0.20, q = 0.90,
Ω = 1.35.
demonstrates that Type 1 solitons can be stable but
Type 2 solitons are always unstable. We have de-
termined stability regions for Type 1 solitons in the
(q, Ω) plane. We have also analyzed the effect of the
variation of parameters on the size of the stable re-
gions.
REFERENCES
Aceves, A. and Wabnitz, S. (1989). Self-induced trans-
parency solitons in nonlinear refractive periodic me-
dia. Phys. Lett. A, 141(1):37–42.
Atai, J. and Chen, Y. (1992). Nonlinear couplers composed
of different nonlinear cores. J. Appl. Phys., 59(1):24–
27.
Atai, J. and Malomed, B. A. (2000). Bragg-grating soli-
tons in a semilinear dual-core system. Phys. Rev. E,
62:8713–8718.
Atai, J. and Malomed, B. A. (2001). Families of Bragg-
grating solitons in a cubic-quintic medium. Phys. Lett.
A, 284(6):247–252.
Atai, J. and Malomed, B. A. (2002). Spatial solitons
in a medium composed of self-focusing and self-
defocusing layers. Phys. Lett. A, 298(2–3):140 – 148.
Moving Bragg Grating Solitons in a Grating-assisted Coupler with Cubic-Quintic Nonlinearity
47

Atai, J., Malomed, B. A., and Merhasin, I. M. (2006). Sta-
bility and collisions of gap solitons in a model of a
hollow optical fiber. Opt. Commun., 265(1):342–348.
Atai, Javid; Chen, Y. (1993). Nonlinear mismatches be-
tween two cores of saturable nonlinear couplers. IEEE
J. Quantum Electron., 29(1):242–249.
Christadoulides, D. N. and Joseph, R. I. (1989). Slow Bragg
solitons in nonlinear periodic structures. Phys. Rev.
Lett., 62:1746–1749.
Conti, C., Trillo, S., and Assanto, G. (1997). Doubly res-
onant Bragg simultons via second-harmonic genera-
tion. Phys. Rev. Lett., 78:2341–2344.
Desterke, C. M. and Sipe, J. E. (1994). Gap Solitons.
Progress in Optics, 33:203–260.
Dong, R., R¨uter, C. E., Kip, D., Cuevas, J., Kevrekidis,
P. G., Song, D., and Xu, J. (2011). Dark-bright gap
solitons in coupled-mode one-dimensional saturable
waveguide arrays. Phys. Rev. A, 83:063816.
Fraga, W. B., Menezes, J. W. M., da Silva, M. G., Sobrinho,
C. S., and Sombra, A. S. B. (2006). All optical logic
gates based on an asymmetric nonlinear directional
coupler. Opt. Commun., 262(1):32–37.
He, H. and Drummond, P. D. (1997). Ideal soliton envi-
ronment using parametric band gaps. Phys. Rev. Lett.,
78:4311–4315.
Islam, M. J. and Atai, J. (2015). Stability of gap solitons
in dual-core Bragg gratings with cubic-quintic nonlin-
earity. Laser Phys. Lett., 12(1):015401.
Jensen, S. (1982). The nonlinear coherent coupler. IEEE J.
Quantum Electron., 18(10):1580–1583.
Kaup, D. J. and Malomed, B. A. (1998). Gap solitons in
asymmetric dual-core nonlinear optical fibers. J. Opt.
Soc. Am B, 15(12):2838–2846.
Mak, W. C. K., Chu, P. L., and Malomed, B. A. (1998a).
Solitary waves in coupled nonlinear waveguides with
Bragg gratings. J. Opt. Soc. Am. B, 15(6):1685–1692.
Mak, W. C. K., Malomed, and Chu, P. L. (1998b).
Asymmetric solitons in coupled second-harmonic-
generating waveguides. Phys. Rev. E, 57:1092–1103.
Mak, W. C. K., Malomed, B. A., and Chu, P. L. (2003).
Formation of a standing-light pulse through collision
of gap solitons. Phys. Rev. E, 68(2 Pt 2):026609.
Mak, W. C. K., Malomed, B. A., and Chu, P. L. (2004).
Symmetric and asymmetric solitons in linearly cou-
pled Bragg gratings. Phys. Rev. E, 69(6 Pt 2):066610.
Mayteevarunyoo, T. and Malomed, B. A. (2008). Gap
solitons in grating superstructures. Opt. Express,
16(11):7767–7777.
Mok, J. T., Desterke, C. M., Litler, I. C. M., and Eggleton,
B. J. (2006). Dispersionless slow light using gap soli-
tons. Nat. Phys., 2:775–780.
Neill, D. R. and Atai, J. (2007). Gap solitons in a hollow
optical fiber in the normal dispersion regime. Phys.
Lett. A, 367:73–82.
Skryabin, D. V. (2004). Coupled core-surface solitons in
photonic crystal fibers. Opt. Express, 12(20):4841–
4846.
Tsofe, Y. J. and Malomed, B. A. (2007). Quasisymmet-
ric and asymmetric gap solitons in linearly coupled
Bragg gratings with a phase shift. Phys. Rev. E, 75(5
Pt 2):056603.
PHOTOPTICS 2017 - 5th International Conference on Photonics, Optics and Laser Technology
48